Time Value of Money | Crash Course for UGC NET Commerce PDF Download
| Table of contents |

|
| Introduction |

|
| Understanding the Time Value of Money (TVM) |

|
| Relationship Between TVM and Opportunity Cost |

|
| Importance of the Time Value of Money |

|
| Inflation's Impact on TVM |

|
Introduction
The Time Value of Money (TVM) is a fundamental concept in finance that states a sum of money is worth more today than the same sum in the future due to its potential earning capacity. This is because money can generate returns when invested, so delaying a payment results in lost growth opportunities. TVM is also known as the present discounted value.
Key Points:
- Current vs. Future Value: A sum of money is more valuable today than in the future.
- Investment Potential: The principle acknowledges that money can grow over time when invested, and delaying this investment means missing out on potential gains.
- Calculation Formula: TVM is calculated using a formula that takes into account the amount of money, its future value, potential earnings, and the time period involved.
- Compounding: For savings and similar accounts, the number of compounding periods significantly impacts the outcome.
- Inflation Impact: Inflation decreases the purchasing power of money over time, negatively affecting TVM.
Understanding the Time Value of Money (TVM)
Investors prefer to receive money today rather than later because once invested, money can grow due to the power of compound interest. For example, money placed in a high-yield savings account will earn interest, which will, in turn, earn more interest over time. Conversely, if money is not invested, it can lose value due to inflation. For instance, $1,000 hidden under a mattress for three years will not only miss out on earning potential but will also lose purchasing power as prices rise.
TVM Formula
The basic formula for calculating the time value of money shows the change in a sum's value over time. It factors in:
- Present value
- Interest rate
- Number of compounding periods per year
- Number of years
This formula helps in understanding how the value of money evolves over time.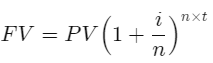
where:
FV = Future value of money
PV = Present value of money
i = Interest rate
n = Number of compounding periods per year
t = Number of years
This concept helps you understand the difference between future value and present value. Typically, the future value will be higher, which is why it's generally better to receive money now rather than later.
The TVM formula can vary depending on the specific scenario. For instance, when dealing with annuities or perpetuity payments, the general formula may include additional factors or exclude some.
The time value of money doesn't take into account any capital losses that you may incur or any negative interest rates that may apply. In these cases, you may be able to use negative growth rates to calculate the time value of money
Examples of Time Value of Money
Consider a scenario where $10,000 is invested for one year at an annual interest rate of 10%, compounded annually.
The future value of that money is: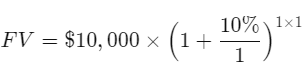
= $11, 000
The formula can also be rearranged to find the value of the future sum in present-day dollars. For example, the present-day dollar amount compounded annually at 7% interest that would be worth $5,000 one year from today is: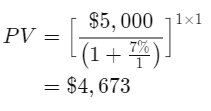
Effect of Compounding Periods on Future Value
The number of compounding periods has a dramatic effect on the TVM calculations. Taking the $10,000 example above, if the number of compounding periods is increased to quarterly, monthly, or daily, the ending future value calculations are:
- Quarterly Compounding:
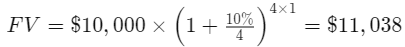
- Monthly Compounding:
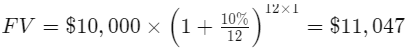
- Daily Compounding:
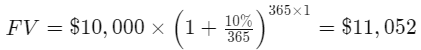
Factors Influencing Time Value of Money (TVM)
The TVM is influenced not only by the interest rate and the time horizon but also by the frequency of compounding within a given year.
Relationship Between TVM and Opportunity Cost
Opportunity cost is a fundamental aspect of the time value of money. Money can only grow if it is invested and earns a positive return over time. Money that is not invested loses value due to inflation. Therefore, any sum expected to be received in the future, regardless of the certainty of its payment, loses value over time. The opportunity cost here is the potential growth lost by not investing that money today.
Importance of the Time Value of Money
The time value of money is crucial for making informed investment decisions. For example, if a business must choose between two projects, where Project A offers a $1 million cash payout in one year and Project B offers the same amount in five years, the payouts are not equivalent. The $1 million received after one year has a higher present value than the same amount received after five years.
Application of TVM in Finance
The time value of money is a cornerstone of financial decision-making, affecting virtually every area of finance. It is central to discounted cash flow (DCF) analysis, one of the most widely used methods for evaluating investment opportunities. TVM is also critical in financial planning and risk management. For example, pension fund managers use TVM principles to ensure that account holders receive sufficient funds upon retirement.
Inflation's Impact on TVM
The value of money evolves over time, and various factors, such as inflation, influence this change. Inflation, or the general increase in the prices of goods and services, diminishes the future value of money. As prices rise, the purchasing power of money decreases, meaning that the same amount of money will buy less in the future than it does today.
Conclusion
The future value of money is different from its present value, and this difference is captured by the concept of the time value of money (TVM). Both businesses and individuals can use this concept to make more informed investment decisions.
|
237 videos|236 docs|166 tests
|
FAQs on Time Value of Money - Crash Course for UGC NET Commerce
| 1. How does the Time Value of Money (TVM) concept impact investment decisions? |  |
| 2. What is the relationship between the Time Value of Money (TVM) and opportunity cost? |  |
| 3. Why is the Time Value of Money (TVM) important in financial planning? |  |
| 4. How does inflation impact the Time Value of Money (TVM)? |  |
| 5. What are some practical examples of applying the Time Value of Money (TVM) concept in everyday financial decisions? |  |















