Vibration Isolation | Theory of Machines (TOM) - Mechanical Engineering PDF Download
Vibrations Causing Unbalanced Forces in a Mechanical Running System
1. Rotating Unbalance
(Rotors, whose C.G. is not coinciding with the axis of shaft).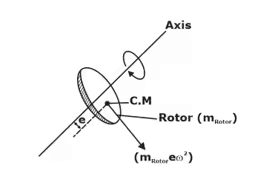 At time, t
At time, t
fun in a particular direction,
fun = (MRotor eω2)
where, f0 = (mRotor eω2) — max volume of unbalanced force.
ω — force frequency or excitation.
2. Reciprocating Unbalance: (in Piston-crank)
fun = mRrω2 sinθ
[mR—mass of Reciprocating Ports]
(mass of Piston + mass of crosshead + mass of connecting Rod)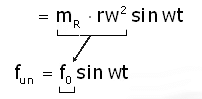
Where, ω = forced frequency and m = machine mass (whole) which is under vibrations.
3. Forced-Damped Systems (Perfect Reality)
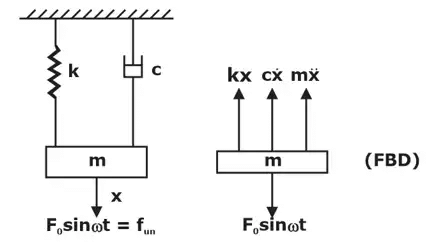 Forced vibrations of a damped spring mass system
Forced vibrations of a damped spring mass system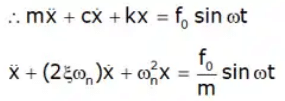
This is the equation of forced-Damped System.
∴ The solution will be, x = c · f + P · I After some time, CF = 0
After some time, CF = 0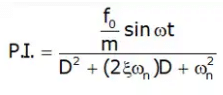
After solving,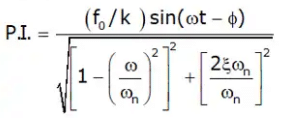
Amplitude of forced vibrations (A):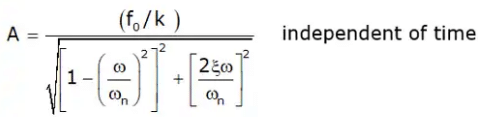
Hence, x = PI
∴ x = A sin(ωt - ϕ)
Where, A = Amplitude of steady state vibrations (independence of time) (forced vibrations)
Running system vibrations will never stop.
Every machine/mechanical running system must have one running life.
4. Magnification factor (M.F.)
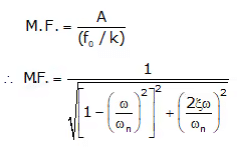
∴ M.F. depends upon:
(i) ω/ωn
(ii) ζ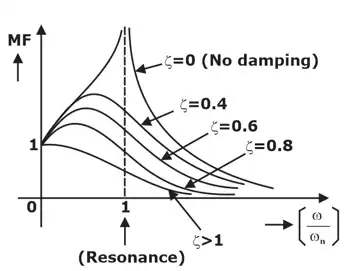 Magnification Factor
Magnification Factor
- As Underdamping Increases
⇒ ζ↓
⇒ MF ↑ ⇒ A↑
⇒ Running life decrease ↓ - At Resonance
[ω/ωn = 1]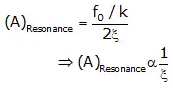
Note: At some time 't',
F = f0sin[ωt]
x = Aω cos ωt
= Aω[sin{π/2 + (ωt - ϕ)}]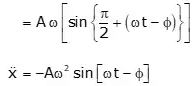
The Basic Equation was,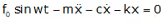
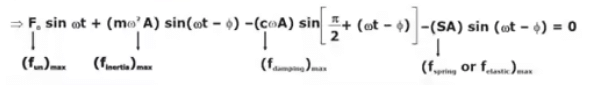
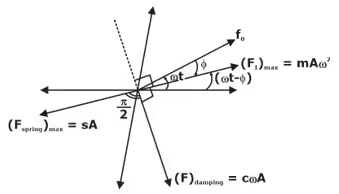
- Spring and damping force’s max values are perpendicular to each other.
- Inertia force lies exactly opposite (At 180°) To the spring force’s max value.
6. Vibration Isolations
It is used to isolate the ground from the vibrations of the Running machine so as to save other stationary m/cs from these vibration effects.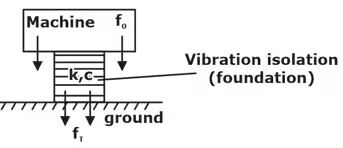 Vibration Isolation
Vibration Isolation
Fτ = force transmitted to the ground
Fτ < < < < < F0
∈ = Fτ / F0 = Transmissivity
0 < ∈ < 1
∈ → 0 (for Belts)
Now, Fτ = Resultant of forces of spring force and damping force (max values)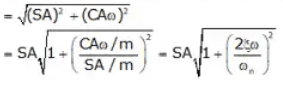
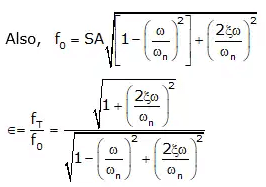
Hence, ′∈′ depends upon:
(i) ω/ωn
(ii) ζ
Note: If, w/wn = 0 w/wn = √2 ⇒ ∈ = 1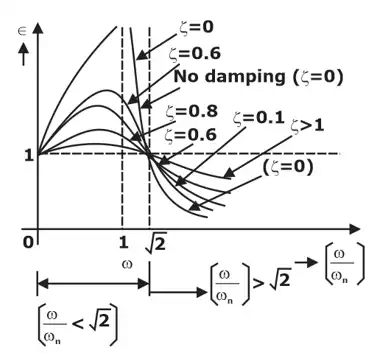
- As Underdamping ↑ ⇒ ζ↓
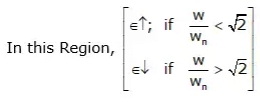
∈ will be constant, if w/wn = √2 - Vibration Isolation is Effective
When ∈ < 1
⇒ (w/wn > √2) → called "Effective Vibration-isolation-zone". - In Effective Vibration Isolation Zone:
w/wn > √2; Then At ζ = 0, e is minimum.
∴ No damping → is Best. (∵ = ∈ → 0 Best)
Hence, Damping is dangerous in this Zone.
⇒ Ass ↑ (in this zone). ∈↑ ⇒ fτ ↑
|
87 videos|75 docs|29 tests
|





















