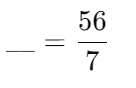Class 5 Maths - Be My Multiple I will be Your Factor - CBSE Worksheets Solutions - 1
Q1. Fill in the blanks with the next two numbers in the pattern:
(i) 2, 4, 8, 16, ____, ____.
(ii) 3, 6, 9, ____, ____.
(iii) 1, 3, 5, ____, ____.
(iv) 10, 20, 30, ____, ____.
Ans:
(i) Next two numbers are 2, 4, 8, 16, 32, 64.
The sequence is doubling at each step:
- 22=4
- 4×2=8
- 8×2=16
Following the same pattern:
- 16×2=32
- 32×2=64
Thus, the next two terms in the sequence are 32 and 64.
(ii) Next two numbers are 3, 6, 9, 12, 15.
The sequence increases by 3 at each step:
- 3+3=6
- 6+3=9
Following the same pattern:
- 9+3=12
- 12+3=15
Thus, the next two terms in the sequence are 12 and 15.
(iii) Next two numbers are 1, 3, 5, 7, 9.
The sequence increases by 2 at each step:
- 1+2=3
- 3+2=5
Following the same pattern:
- 5+2=7
- 7+2=9
Thus, the next two terms in the sequence are 7 and 9.
(iv) Next two numbers are 10, 20, 30, 40, 50.
The sequence increases by 10 at each step:
- 10+10=20
- 20+10=30
Following the same pattern:
- 30+10=40
- 40+10=50
Thus, the next two terms in the sequence are 40 and 50.
Q2. Word Problems:
(i) If you start counting by 3s starting from 0, what is the 7th number you will say?
Ans: The 7th number will be 18.
When you count by 3s starting from 0, you're repeatedly adding 3 to each number. Here's the process for each step:
- Start at 0.
- Add 3 to get the next number:
- Add 3 again:
- Keep adding 3 for each step after that:
0,3,6,9,12,15,18, ....
Now, let's count these steps:
The 1st number is 0.
The 2nd number is 3.
The 3rd number is 6.
The 4th number is 9.
The 5th number is 12.
The 6th number is 15.
The 7th number is 18.
So, the 7th number when counting by 3s starting from 0 is 18.
(ii) Maria collects stickers. She has 5 stickers and collects 5 more each week. Write the pattern of how many stickers she will have at the end of each week for 5 weeks.
Ans: The pattern is as follows:
Week 1: 10 stickers
Week 2: 15 stickers
Week 3: 20 stickers
Week 4: 25 stickers
Week 5: 30 stickers
Week 1: Maria starts with 5 stickers and collects 5 more, so:
5+5=10 stickers.Week 2: She collects another 5 stickers:
10+5=15 stickers.Week 3: She collects another 5 stickers:
15+5=20 stickers.Week 4: She collects another 5 stickers:
20+5=25 stickers.Week 5: She collects another 5 stickers:
25+5=30 stickers.
(iii) James is planting trees in rows. He plants 4 trees in each row. If he plants 5 rows, how many trees does he plant in total? Write the pattern of the total number of trees after each row.
Ans: The pattern is as follows:
After Row 1: 4 trees
After Row 2: 8 trees
After Row 3: 12 trees
After Row 4: 16 trees
After Row 5: 20 trees
Row 1:
4×1=4 trees planted.Row 2:
4×2=8 trees planted.Row 3:
4×3=12 trees planted.Row 4:
4×4=16 trees planted.Row 5:
4×5=20 trees planted.
Q3: Practice the questions given in the worksheet on factors and multiples.
(i) Find out the even numbers.
27, 36, 48, 125, 360, 453, 518, 423, 54, 58, 917, 186, 423, 928, 358
Ans: 36, 48, 360, 518, 54, 58, 186, 928, 358
To find the even numbers in the list, we need to identify the numbers that are divisible by 2.
(ii) Find out the odd numbers.
10, 45, 78, 146, 347, 543, 495, 638, 497, 968, 729, 427, 624, 572
Ans: 45, 347, 543, 495, 497, 729, 427
To find the odd numbers in the list, we need to identify the numbers that are not divisible by 2.
(iii) Write the factors of the following:
(a) 27
(b) 32
(c) 18
(d) 45
(e) 25
(f) 56
(g) 68
(h) 38
(i) 72
Ans: Factors are the numbers that can divide a number exactly.
(a) Factors are 1, 3, 9, 27
To find the factors of , we look for all the numbers that can divide without leaving a remainder.
The factors of are:
1 (since 27÷1=27)
3 (since 27÷3=9)
9 (since 27÷9=3)
27 (since 27÷27=1)
Factors of 27:
1, 3, 9, 27
(b) Factors are 1, 2, 4, 8, 16, 32
To find the factors of 32, we look for all the whole numbers that can divide 32 without leaving a remainder.
Factors of 32:
1 (since )
2 (since )
4 (since )
8 (since )
16 (since )
32 (since )
The factors of 32 are: 1, 2, 4, 8, 16, 32.
(c) Factors are 1, 2, 3, 6, 9, 18
Now, solve the remaining parts by referring to the above two examples.
(d) Factors are 1, 3, 5, 9, 15, 45
(e) Factors are 1, 5, 25
(f) Factors are 1, 2, 4, 7, 8, 14, 28, 56
(g) Factors are 1, 2, 4, 17, 34, 68
(h) Factors are 1, 2, 19, 38
(i) Factors are 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72
(iv) Write the first five multiples of the following:
(a) 4
(b) 3
(c) 7
(d) 9
(e) 5
(f) 8
(g) 12
(h) 15
Ans:
The first five multiples of a number are obtained by multiplying that number by the integers 1 to 5. Here’s a general formula:
- First multiple:
- Second multiple:
- Third multiple:
- Fourth multiple:
- Fifth multiple:
(a) First five mutiples of 4 are 4, 8, 12, 16, 20.
The first five multiples of 4 can be found by multiplying 4 by the integers to :
First five multiples of 4 : 4, 8, 12, 16, 20.
(b) First five mutiples of 3 are 3, 6, 9, 12, 15
For 3:
- 3×1=3
- 3×2=6
- 3×3=9
- 3×4=12
- 3×5=15
First five multiples of 3: 3, 6, 9, 12, 15
(c) First five multiples of 7 are 7, 14, 21, 28, 35
Now, using the above method, the rest can be found out.
(d) First five mutiples of 9 are 9, 18, 27, 36, 45
(e) First five mutiples of 5 are 5, 10, 15, 20, 25
(f) First five mutiples of 8 are 8, 16, 24, 32, 40
(g) First five mutiples of 12 are 12, 24, 36, 48, 60
(h) First five mutiples of 15 are 15, 30, 45, 60, 75
(v) Find the first three multiples of 8.
Ans: 8, 16, 24
(vi) Find the missing factors.
(a) 7 × ___ = 56
(b) 5 × ___ = 30
(c) ___ × 3 = 24
(d) 6 × ___ = 48
(e) 8 × ___ = 72
Ans:
(a) 7 × 8 = 56
To find the missing factor in the equation , you can divide by 7.
Now, calculate it:
How many times does fit into ?
If you count by
You find that goes into exactly 8 times.
So, the missing factor is 8.
(b) 5 × 6 = 30
The rest can be solved with reference to the first one.
(c) 8 × 3 = 24
(d) 6 × 8 = 48
(e) 8 × 9 = 72
(vii) Write the multiples of 6 which are greater than 20 and less than 50.
Ans: 24, 30, 36, 42, 48
List the multiples of 6:
6×1=6
6×2=12
6×3=18
6×4=24
6×5=30
6×6=36
6×7=42
6×8=48
6×9=54
Select the multiples that are greater than 20 and less than 50:
24 (greater than 20 and less than 50)
30 (greater than 20 and less than 50)
36 (greater than 20 and less than 50)
42 (greater than 20 and less than 50)
48 (greater than 20 and less than 50)
So, The multiples of 6 that are greater than 20 and less than 50 are:24, 30, 36, 42, 48.
|
31 videos|192 docs|41 tests
|
FAQs on Class 5 Maths - Be My Multiple I will be Your Factor - CBSE Worksheets Solutions - 1
| 1. What is the difference between multiples and factors? |  |
| 2. How can I find the multiples of a number? |  |
| 3. Why are learning multiples and factors important in math? |  |
| 4. Can a number be both a multiple and a factor? |  |
| 5. How can I practice finding multiples and factors? |  |