NCERT Solutions for Class 9 Maths Chapter 12 - Exercise 12.2 Heron’s Formula
Application of Heron's Formulas in Finding Areas of Quadrilaterals
Many fields are in the shape of quadrilaterals. Similarly, various surfaces are polygons. To find their areas, we divide the surface into triangular parts and then use Heron’s formula for finding areas of the triangles.

Exercise 12.2
Q1. A park, in the shape of a quadrilateral ABCD, has – C = 90°, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m. How much area does it occupy?
Ans: Let us join B and D, such that ΔBCD is a right-angled triangle.
We have area of ΔBCD = (1/2)x base x altitude
=(1/2)x 12 x 5 m2
= 30 m2
Now, to find the area of ΔABD, we need the length of BD
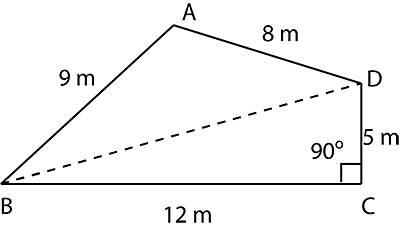
∴ In right ΔBCD, BD2 = BC2 + CD2 [Pythagoras theorem]
⇒ BD2 = 122 + 52
⇒ BD2 = 144 + 25 = 169 = 132
⇒ BD =  = 13 m
= 13 m
Now, for ΔABD, we have a = 9 m, b = 8 m, c = 13 m
∵ 
∴ Area of ΔABD

∴ Area of quadrilateral ABCD = area of ΔBCD + area of ΔABD
= 30 m2 + 35.5 m2 = 65.5 m2 (appx)
Q2. Find the area of a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 4 cm, DA = 5 cm and AC = 5 cm.
Ans: For ΔABC,
a= 3 cm, b= 4cm and c = 5 cm

∴ Area of ΔABC,

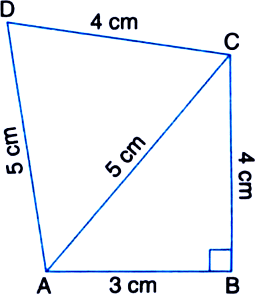
For ΔACD,
a=5 cm, b = 4cm and c= 5cm


For ΔACD,
a=5 cm, b = 4cm and c= 5cm

∴ Area of ΔACD,

Now, area of quadrilateral ABCD = area of ΔABC + area of ΔACD
= 6 cm2 + 9.2 cm2 (approx.)
= 15.2 cm2 (approx.)
Q3. Radha made a picture of an aeroplane with coloured paper as shown in Fig. Find the total area of the paper used.
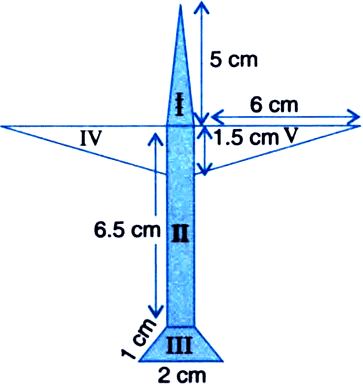
Ans: Area of surface I It is an isosceles triangle whose sides are a = 5 cm, b = 5 cm, c = 1 cm


= 0.75 x 3.3 cm2 (approx.) = 2.475 cm2 (approx.)
Area of surface II It is a rectangle with length of 6.5 cm and breadth of 1 cm.
∴ Area of rectangle II = Length x Breadth
= 6.5 x 1 = 6.5 cm2
Area of surface III It is a trapezium whose parallel sides are 1 cm and 2 cm as shown in the adjoining figure. Its height is given by


Note: The perpendicular distance between the parallel sides is called the height of the trapezium.
∵ Area of a trapezium = 1/2(sum of the parallel sides) x Height
∴ Area of trapezium III =

Area of surface IV It is a right triangle with base as 6 cm and height as 1.5 cm.
∴ Area of right triangle IV =(1/2)x base x height
=(1/2)x 6 x 1.5 cm2 = 4.5 cm2
Area of surface V
∵ Right triangle V ≌ Right triangle IV
∴ Area of right triangle V = Area of right triangle IV = 4.5 cm2
Thus, the total area of the paper used = (area I) + (area II) + (area III) + (area IV) + (area V)
= [2.475 cm2 (approx.)] + [6.5 cm2] + [1.3 cm2 (approx.)] + [4.5 cm2] + [4.5 cm2]
= 19.275 cm2 = 19.3 cm2 (approx.)
Q4. A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26 cm, 28 cm and 30 cm, and the parallelogram stands on the base 28 cm, find the height of the parallelogram.
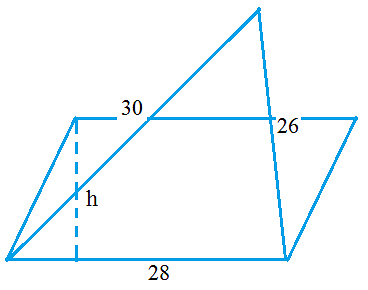
Ans: For the given triangle, we have a = 28 cm, b = 30 cm, c = 26 cm


= 6 x 7 x 2 x 4 cm2 = 336 cm2
∵ [Area of the given parallelogram] = [Area of the given triangle]
∴ [Area of the parallelogram] = 336 cm2
∴ [Base of the parallelogram] x [Height of the parallelogram] = 336 cm2
⇒ [28 cm] x [h cm] = 336 cm2
⇒ h= (336/28)cm = 12
Thus, the required height of the parallelogram = 12 cm
Q5. A rhombus shaped field has green grass for 18 cows to graze. If each side of the rhombus is 30 m and its longer diagonal is 48 m, how much area of grass field will each cow be getting?
Ans: Here, each side of the rhombus = 30 m
One of the diagonal = 48 m
Since a diagonal divides the rhombus into two congruent triangles.

Sides of first triangle are a = 30 m, b = 30 m, c = 48 m
∵

∴ Area of triangle I =

= 3 x 6 x 4 x 6 m2 = 432 m2
∴ Area of second triangle = 432 m2
⇒ Total area of both triangles = 432 cm2 + 432 m2 = 864 m2
⇒ The area of the rhombus = 864 m2
Thus, area of grass for 18 cows = 864 m2
⇒ Area of grass for 1 cow = (864/18)m2 = 48 m2
Q6. An umbrella is made by stitching 10 triangular pieces of cloth of two different colours (see figure), each piece measuring 20 cm, 50 cm and 50 cm. How much cloth of each colour is required for the umbrella?
Ans: Sides of each triangular piece are

a = 20 cm, b = 50 cm, c = 50 cm

∴ Area of each triangular piece

⇒ Area of 5 triangular pieces of one colour = 5 x 200/√6cm2 = 1000/√6 cm2 Area of 5 triangular pieces of other colour = 1000/√6 cm2
Note: Total triangular pieces are 10, i.e. five triangular pieces for each colour.
Q7. A kite in the shape of a square with a diagonal 32 cm and an isosceles triangle of base 8 cm and sides 6 cm each is to be made of three different shades as shown in the figure. How much paper of each shade has been used in it?
Ans: Area of triangle I
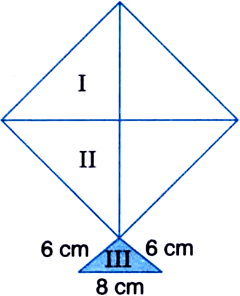
Diagonal = 32 cm
∴ Height of triangle =(1/2)(32) cm= 16 cm
∵ Area of triangle = (1/2)x base x height
∴ Area of triangle I= (1/2)x 32 x 16 cm2 = 256 cm2
Area of triangle II ∵ Diagonal of a square divides it into two congruent triangles.
∴ Area of triangle II = Area of triangle I
⇒ Area of triangle II = 256 cm2
Area of triangle III The triangle at the base is having sides as a = 8 cm, b = 6 cm, c = 6 cm
∵ 
∴ Area of triangle III

Thus, the area of different shades are: Area of shade I = 256 cm2,
Area of shade II = 256 cm2
Area of shade III = 17.92 cm2
Q8. A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9 cm, 28 cm and 35 cm (see figure). Find the cost of polishing the tiles at the rate of 50p per cm2.
Ans: There are 16 equal triangular tiles.
Area of a triangle Sides of the triangle are a = 9 cm, b = 28 cm, c = 35 cm

∵
=(72/2)cm = 36 cm
∴ Area of the triangle

Area of 16 triangles: Total area of all the triangles = 16 x 88.2 cm2 (approx.) = 1411.2 cm2 (approx.) Cost of polishing the tiles Rate of polishing = ₹ 0.5 per cm2
∴ Cost of polishing all the tiles = ₹ 0.5 x 1411.2
= ₹(5/10) x (14112/10)= ₹705.60 (approx.)
Q9. A field is in the shape of a trapezium whose parallel sides are 25 m and 10 m. The non-parallel sides are 14 m and 13 m. Find the area of the field.
Ans: The given field is in the form of a trapezium ABCD such that parallel sides AB = 10 m and DC = 25 m

Non-parallel sides are 13 cm and 14 cm.
We draw BE || AD, such that BE = 13 cm.
The given field is divided into two shapes:
(i) parallelogram ABED (ii) DBCE.
Area of ΔBCE: Sides of the triangle are a = 13 m, b = 14 m, c = 15 m

Let the height of the ΔBCE corresponding to the side 15 m be ‘h’ metres
∵ Area of a triangle = (1/2)x base x height
∴ (1/2)x 15 x h= 84
⇒ 
x m = (56/5)m
Area of parallelogram ABED
∵ Area of a parallelogram = base x height
∴ Area of parallelogram ABED = 10 x (1/2)m2 = 2 x 56 m2 = 112 m2
Thus, area of the field = Area of ΔBCE + Area of parallelogram ABED
= 84 m2 + 112 m2 = 196 m2
|
5 videos|378 docs|164 tests
|
FAQs on NCERT Solutions for Class 9 Maths Chapter 12 - Exercise 12.2 Heron’s Formula
| 1. Can Heron's Formula be used to find the area of any quadrilateral? |  |
| 2. How do you determine if a quadrilateral is cyclic? |  |
| 3. Can Heron's Formula be used to find the area of a rectangle or square? |  |
| 4. Are there any other formulas that can be used to find the area of non-cyclic quadrilaterals? |  |
| 5. How is Heron's Formula derived for finding the area of a triangle? |  |



















