NCERT Solutions for Class 8 Maths - Playing with Numbers (Exercise 3.6 and 3.7)
Exercise 3.6
 Example for calculating H.C.F.
Example for calculating H.C.F.
Q1. Find the H.C.F. of the following numbers:
(a) 18, 48
(b) 30, 42
(c) 18, 60
(d) 27, 63
(e) 36, 84
(f) 34, 102
(g) 70, 105, 175
(h) 91, 112, 49
(i) 18, 54, 81
(j) 12, 45, 75
Ans: (a) Factors of 18 = 2 x 3 x 3
Factors of 48 = 2 x 2 x 2 x 2 x 3
H.C.F. (18, 48) = 2 x 3 = 6
(b) Factors of 30 = 2 x 3 x 5
Factors of 42 = 2 x 3 x 7
H.C.F. (30, 42) = 2 x 3 = 6
(c) Factors of 18 = 2 x 3 x 3
Factors of 60 = 2 x 2 x 3 x 5
H.C.F. (18, 60) = 2 x 3 = 6
(d) Factors of 27 = 3 x 3 x 3
Factors of 63 = 3 x 3 x 7
H.C.F. (27, 63) = 3 x 3 = 9
(e) Factors of 36 = 2 x 2 x 3 x 3
Factors of 84 = 2 x 2 x 3 x 7
H.C.F. (36, 84) = 2 x 2 x 3 = 12
(f) Factors of 34 = 2 x 17
Factors of 102 = 2 x 3 x 17
H.C.F. (34, 102) = 2 x 17 = 34
(g) Factors of 70 = 2 x 5 x 7
Factors of 105 = 3 x 5 x 7
Factors of 175 = 5 x 5 x 7
H.C.F. = 5 x 7 = 35
(h) Factors of 91 = 7 x 13
Factors of 112 = 2 x 2 x 2 x 2 x 7
Factors of 49 = 7 x 7
H.C.F. = 1 x 7 = 7
(i) Factors of 18 = 2 x 3 x 3
Factors of 54 = 2 x 3 x 3 x 3
Factors of 81 = 3 x 3 x 3 x 3
H.C.F. = 3 x 3 = 9
(j) Factors of 12 = 2 x 2 x 3
Factors of 45 = 3 x 3 x 5
Factors of 75 = 3 x 5 x 5
H.C.F. = 1 x 3 = 3
Q2. What is the H.C.F. of two consecutive:
(a) numbers?
(b) even numbers?
(c) odd numbers?
Ans: (a) The HCF of two consecutive numbers is 1
Example: The HCF of 2 and 3 is 1
(b) The HCF of two consecutive even numbers is 2
Example: The HCF of 2 and 4 is 2
(c) The HCF of two consecutive odd numbers is 1
Example: The HCF of 3 and 5 is 1
Q3. HCF of co-prime numbers 4 and 15 was found as follows by factorisation : 4 = 2 × 2 and 15 = 3 × 5 since there is no common prime factor, so HCF of 4 and 15 is 0. Is the answer correct? If not, what is the correct HCF?
Ans: No. The correct H.C.F. is 1.
Exercise 3.7
Q1. Renu purchases two bags of fertiliser of weights 75 kg and 69 kg. Find the maximum value of weight which can measure the weight of the fertiliser exact number of times.
Ans: For finding maximum weight, we have to find H.C.F. of 75 and 69.
Factors of 75 = 3 x 5 x 5
Factors of 69 = 3 x 69
H.C.F. = 3
Therefore the required weight is 3 kg.
Q2. Three boys step off together from the same spot. Their steps measure 63 cm, 70 cm and 77 cm respectively. What is the minimum distance each should cover so that all can cover the distance in complete steps?
Ans: Step measure by first boy = 63 cm
Step measure by second boy = 70 cm
Step measure by third boy = 77 cm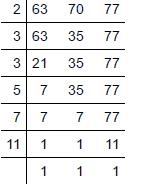
LCM = 2 × 3 × 3 × 5 × 7 × 11 = 6930
The minimum distance each should cover so that all can cover the distance in complete steps is 6930 cm.
Q3. The length, breadth and height of a room are 825 cm, 675 cm and 450 cm respectively. Find the longest tape which can measure the three dimensions of the room exactly.
Ans: The measurement of longest tape = H.C.F. of 825 cm, 675 cm and 450 cm.
Factors of 825 = 3 x 5 x 5 x 11
Factors of 675 = 3 x 5 x 5 x 3 x 3
Factors of 450 = 2 x 3 x 3 x 5 x 5
H.C.F. = 3 x 5 x 5 = 75 cm
Therefore, the longest tape is 75 cm.
Q4. Determine the smallest 3-digit number which is exactly divisible by 6, 8 and 12.
Ans: 
LCM of 6,8,12 = 2 × 2 × 2 × 3 × = 24
Hence, the smallest 3-digit number which meets the requirements must be a multiple of 24.
The smallest 3 – digit multiple of 24 is 24× 5 = 120.
Q5. Determine the greatest 3-digit number exactly divisible by 8, 10 and 12.
Ans:
LCM = 2 × 2 × 2 × 3 × 5 = 120
Now we need to find the greatest 3-digit multiple of 120
We may find 120 × 8 = 960 and 120 × 9 = 1080
Hence, 960 is the greatest 3-digit number exactly divisible by 8, 10 and 12
Q6. The traffic lights at three different road crossings change after every 48 seconds, 72 seconds and 108 seconds respectively. If they change simultaneously at 7 a.m., at what time will they change simultaneously again?
Ans: 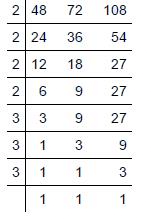
LCM = 2 × 2 × 2 × 2 × 3 × 3 × 3 = 432
Hence, lights will change together after every 432 seconds
Therefore the lights will change simultaneously at 7 minutes and 12 seconds.
Q7. Three tankers contain 403 litres, 434 litres and 465 litres of diesel respectively. Find the maximum capacity of a container that can measure the diesel of the three containers exact number of times.
Ans: The maximum capacity of container = H.C.F. (403, 434, 465)
Factors of 403 = 13 x 31
Factors of 434 = 2 x 7 x 31
Factors of 465 = 3 x 5 x 31
H.C.F. = 31
Therefore, 31 litres of the container is required to measure the quantity.
Q8. Find the least number which when divided by 6, 15 and 18, leave remainder 5 in each case.
Ans: LCM of 6, 15, 18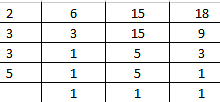
LCM = 2 × 3 × 3 × 5 = 90
Required number = 90 + 5
= 95
Hence, 95 is the required number.
Q9. Find the smallest 4-digit number which is divisible by 18, 24 and 32.
Ans: LCM of 18, 24, 32
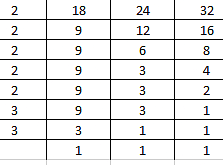
LCM = 2 × 2 × 2 × 2 × 2 × 3 × 3 = 288
Here, we need to find the smallest 4-digit multiple of 288
We find 288 × 3 = 864 and 288 × 4 = 1152
Hence, 1152 is the smallest 4-digit number which is divisible by 18, 24 and 32
Q10. Find the L.C.M. of the following numbers:
(a) 9 and 4
(b) 12 and 5
(c) 6 and 5
(d) 15 and 4
Observe a common property in the obtained L.C.Ms. Is L.C.M. the product of two numbers in each case?
Ans: (a) L.C.M. of 9 and 4
= 2 x 2 x 3 x 3
= 36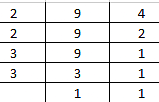
(b) L.C.M. of 12 and 5
= 2 x 2 x 3 x 5
= 60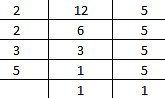
(c) L.C.M. of 6 and 5
= 2 x 3 x 5
= 30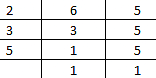
(d) L.C.M. of 15 and 4
= 2 x 2 x 3 x 5
= 60
Yes in each case the LCM of given numbers is the product of these numbers.
Q11. Find the L.C.M. of the following numbers in which one number is the factor of other:
(a) 5, 20
(b) 6, 18
(c) 12, 48
(d) 9, 45
What do you observe in the result obtained?
Ans: (a) L.C.M. of 5 and 20 = 2 x 2 x 5 = 20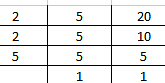
(b) L.C.M. of 6 and 18
= 2 x 3 x 3
= 18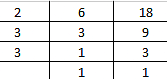
(c) L.C.M. of 12 and 48
= 2 x 2 x 2 x 2 x 3
= 48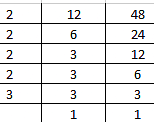
(d) L.C.M. of 9 and 45
= 3 x 3 x 5
= 45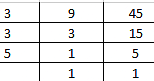
∴ Hence, in each case the LCM of given numbers is the larger number. When a number is a factor of other number then their LCM will be the larger number.
|
5 videos|378 docs|164 tests
|
FAQs on NCERT Solutions for Class 8 Maths - Playing with Numbers (Exercise 3.6 and 3.7)
| 1. What are the topics covered in Exercise 3.6 and Exercise 3.7 of NCERT Solutions Playing with Numbers? |  |
| 2. How to determine if a number is divisible by 2, 3, 4, 5, 6, 8, 9, and 10? |  |
| 3. What is the difference between HCF and LCM? |  |
| 4. Is it possible for the HCF of two numbers to be greater than the smaller number itself? |  |
| 5. How can one find the HCF and LCM of more than two numbers? |  |

|
Explore Courses for CAT exam
|

|


















