Class 9 Maths Question Answers - Areas of Parallelograms
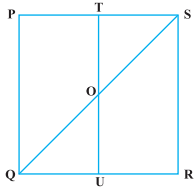
Q1. PQRS is a square. T and U are respectively, the mid points of PS and QR. Find the area of ΔOTS, if PQ = 8 cm, where O is the point of intersection of TU and OS.
We have, PS = PQ = 8 cm and TU || PQ
∵ ST = (1/2) PS
∴ ST = (1/2)× 8 = 4 cm
Also, PQ = TU = 8 cm
∴ OT = (1/2)TU = (1/2) × 8 = 4 cm
Now, Area of ΔOTS
= (1/2)× ST × OT [∵ OTS is a rt. triangle]
= (1/2)× 4 × 4 cm2
= 8 cm2
Q2. The area of the parallelogram PQRS (in the adjoining figure) is 88 cm2. Find the value of x.
PQRS is a parallelogram.
Area of a parallelogram = base x height
∴ x * 8 cm = 88 cm2
⇒ x= (88/8) cm
⇒ x= 11 cm Thus, the required value of x is 11 cm.
Q3. Find the area of ΔABC given in the adjoining figure.
∵The area of a triangle = (1/2)x base x altitude
∴ Area of ΔABC = (1/2)x 10 cm x 4 cm
= 20 cm2
Q4. In the adjoining figure, ABCD is a parallelogram and BPC is a triangle. If the area of parallelogram ABCD = 26 cm2, then find the area of triangle BPC.
Since parallelogram ABCD and ΔBPC are on the same base BC and between the same parallels BC and AD.
∴ Area of ΔBPC = (1/2) x Area of parallelogram ABCD
⇒ Area of ΔBPC =(1/2) x 26 cm2
= 13 cm2
∴ The required area of ΔBPC = 13 cm2.
Q5. In the adjoining figure, PQRS is a parallelogram and PQT is a triangle. If area of triangle PQT = 18 cm2, then find the area of the parallelogram PQRS.
∵ Parallelogram PQRS and ΔPQT are on the same base PQ and between the same parallels PQ and SR.
∴ Area of parallelogram PQRS = 2(ar ΔPQT)
⇒ ar (parallelogram PQRS) = 2(18 cm2)
⇒ ar (parallelogram PQRS)
= 36 cm2
Thus, the required area of parallelogram PQRS is 36 cm2.
Q6. In the adjoining figure. ABC is triangle and AD is a median. If the area of ΔABD is 15 cm2, then find the area of ΔABC.
Since, a median divides the triangle into two triangles of equal areas.
∴ ar ΔABD = (1/2)(ar ΔABC)
⇒ 2(ar ΔABD) = ar (ΔABC)
⇒ 2(15 cm2) = ar (ΔABC)
⇒ 30 cm2 = ar (ΔABC)
Thus, the required area of ΔABC is 30 cm2.
Q7. The area of ΔABC, in the adjoining figure, is 32 cm2. AD is a median and E is the mid-point of AD. Find the area of ΔBED.
Since, the median of a triangle, divides it into triangles of equal areas.
∴ ar (ΔABD) =(1/2)x ar (ΔABC) ...(1)
Similarly, ar (ΔBED) = (1/2) x ar (ΔABD) ...(2)
From (1) and (2), we have ar (ΔBED)
= (1/2)x (1/2)x [ar (ΔABC)]
= (1/4) ar (ΔABC)
= (1/4)x 32 cm2 = 8 cm2
Thus, the area of ΔBED is 8 cm2.
Q8. In the adjoining figure, the area of ΔBCE is 21 cm2. If CD = 6 cm, then find the length of AF.
∵ Parallelogarm ABCD and ΔBCE are on the same base and between the same parallels.
∴ ar (ΔBCE) = (1/2) x ar (parallelogram ABCD)
⇒ 21 cm2 = (1/2)x ar (parallelogram ABCD)
⇒ 21 cm2 = (1/2) [CD x AF]
⇒ 21 cm2 = (1/2)[6 x AF]
⇒ AF = ((21 x 2)/2) cm = 7 cm
Thus, the required length of AF is 7 cm.
Q9. ABCD is a parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersects DC at P, show that: ar (BPC) = ar (DPQ)
∵ The triangles on the same base and between same parallels.
∴ ar (ΔACP) = ar (ΔBPC) .. (1)
and ar (ΔADQ) = ar (ADC) ... (2)
⇒ ar (ΔADC) - ar (ΔADP)
= ar (ΔADQ) - ar (ΔADP)
⇒ ar (ΔAPC) = ar (ΔAPQ) ... (3)
From (1) and (3), we get
ar (ΔBCP) = ar (ΔDPQ)
Q10. In the adjoining figure, the area of a parallelogram ABCD is 40 cm2. If PQ is a median of ΔCDP then, find the area of ΔPDQ.
ar (ΔCDP) = (1/2) (Area of parallelogram ABCD)
= (1/2) (40 cm2) = 20 cm2
∵ PQ is a median of ΔCDP.
∴ ar (ΔPDQ) = (1/2) x ar (ΔCDP)
⇒ ar (ΔPDQ) = (1/2) x (20 cm2) = 10 cm2
Thus, the required area of ΔPDQ is 10 cm2.
Q11. In the adjoining figure, ABC is a triangle having area as 24 cm2. Find the area of (i) ΔEFD and (ii) parallelogram BDEF such that E, F and D are the mid-points of sides CA, AB and BC respectively.
Since, D, E and F are the mid-points of sides BC, CA and AB respectively.
∴ ar (ΔDEF) = (1/4) (ar ΔABC)
ar (ΔDEF) = (1/4)(24 cm2) = 6 cm2
Thus, the required area of ΔDEF = 6 cm2.
ar (parallelogram BDEF) = ar (ΔBDF) + ar (ΔDEF)
= (1/4)x ar (ΔABC) + (1/4)x ar (ΔABC)
= (1/4)x (24 cm2) +(1/4) x (24 cm2)
= 6 cm2 + 6 cm2
= 12 cm2
Thus, the required area of parallelogram BDEF = 12 cm2.
|
40 videos|471 docs|57 tests
|
FAQs on Class 9 Maths Question Answers - Areas of Parallelograms
| 1. What is the formula to calculate the area of a parallelogram? |  |
| 2. How can I find the height of a parallelogram if the area and base are given? |  |
| 3. What is the formula to calculate the area of a triangle? |  |
| 4. Can the area of a parallelogram be negative? |  |
| 5. How is the area of a triangle related to the area of a parallelogram? |  |