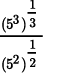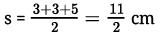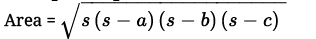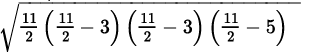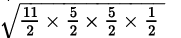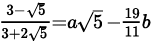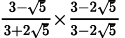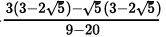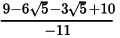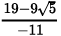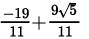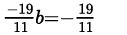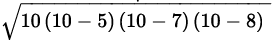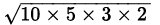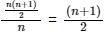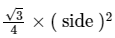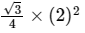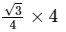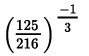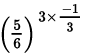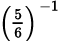Class 9 Math: Sample Question Paper Term I- 1 (With Solutions) | Mathematics (Maths) Class 9 PDF Download
| Table of contents |

|
| Class 09 Mathematics |

|
| Time: 90 Minutes |

|
| Max. Marks: 40 |

|
| Section - A |

|
| Section - B |

|
| Section - c |

|
Class 09 Mathematics
Time: 90 Minutes
Max. Marks: 40
General Instructions :
- The question paper contains three parts A, B and C
- Section A consists of 20 questions of 1 mark each. Attempt any 16 questions
- Section B consists of 20 questions of 1 mark each. Attempt any 16 questions.
- Section C consists of 10 questions based on two Case Studies. Attempt any 8 questions.
- There is no negative marking.
Section - A
Q.1: There is a number x such that x2 is irrational but x4 is rational. Then, x can be
(a) 3√2
(b) √5
(c) √2
(d) 4√2
Correct Answer is Option (d)
As (4√2)2 = √2 is irrational and (4√2)4 = 2 is rational.
Q.2: Which of the following is not a solution of 3x + 4y = 12?
(a) (4, 0)
(b) (0, 3)
(c) (8, -3)
(d) (2, 3)
Correct Answer is Option (d)
the given co-ordinate is solution of a equation if on putting the co-ordinates L.H.S = R.H.S
3x + 4y = 12
put co-ordinate (2, 3) in given equation
L.H.S
3.2 + 4.3
6 + 12 = 18
L.HS ≠ R.H.SSo we can say (2, 3) is a not a solution of 3x + 4y = 12
Q.3: n the given figure, AB CD. If ∠EAB = 50° and ∠ECD = 60°, then ∠AEB =?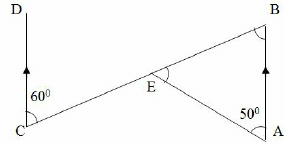
(a) 50°
(b) 60°
(c) 55°
(d) 70°
Correct Answer is Option (d)
∠BCD = ∠ABE = 60° (Vertically opposite angle)
In ΔEAB
∠EAB + ∠EBA + ∠AEB + 180 (Angle sum property)
50° + 60° + ∠AEB = 180°
∠AEB = 70°
Q.4: The perimeter of an equilateral triangle is 48 cm. Its area is
(a) 60√3 sq. cm
(b) 72√3 sq. cm
(c) 64√3 sq. cm
(d) 18√3 sq. cm
Correct Answer is Option (c)
Side = 48/3 = 16 cm
Area = √3/2 x 16 x 16 = 64√3 sq. cm
Q.5: The cube root of 125 divided by square root of 25, is
(a) None of these
(b) 1
(c) 5
(d) 1/5
Correct Answer is Option (b)
The cube root of 125 divided by square root of 25,
=
=
= 5/5
= 1
Q.6: The graph of the linear equation x – y = 0 passes through the point
(a) 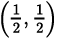
(b) (0, 1)
(c) 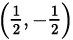
(d) (-1, 1)
Correct Answer is Option (a)
The graph of the linear equation x – y = 0 passes through the pointbecause the co-ordinate of x and y axis satisfy the given equation
x - y =0
1/2 - 1/2 = 0
0 = 0so we can say
is a solution of above equation
so we can say the value of x co-ordinate must be equal to y co -ordinate
Q.7: The point P(3, 0) lies
(a) on the y-axis
(b) on the x-axis
(c) in IV Quadrant
(d) in II Quadrant
Correct Answer is Option (b)
In Cartesian plane, any point p is written as p(x, y).
If the value of y-coordinate (ordinate) is zero then the point will lie on the x-axis.
So here point p(3, 0) will lie on positive x-axis
Q.8: In Figure, if EC || AB, ∠ECD = 70° and ∠BDO = 20°, then ∠OBD is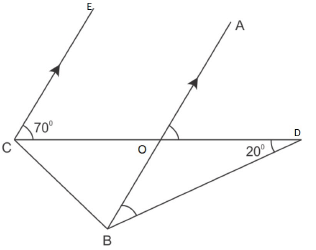
(a) 50°
(b) 50°
(c) 60°
(d) 70°
Correct Answer is Option (a)
EC || AB and CD is transverse to it.
Now ∠ECD = ∠AOD = 70° (Corresponding angles)
In ΔOBD
∠OBD + ∠BOD + ∠ODB = 180°
∠BOD = 180° - ∠AOD = 180° - 70° = 110°
∠ODB = 20° (Given)
So ∠OBD = 180° - ∠BOD - ∠ODB
= 180° - 110° - 20°
= 50°
Q.9: (625)0.16 (625)0.09 =
(a) 625
(b) 5
(c) 125
(d) 25
Correct Answer is Option (b)
(625)0.16 (625)0.09
= (625)0.16 + 0.09
= (625)0.25 or (625)1/4
But 625 = 54
So (54)1/4 = 5
Q.10: It is given that ΔABC ≅ ΔFDE and AB = 5 cm, ∠B = 40° and ∠A = 80°. Then which of the following is true?
(a) DE = 5 cm, ∠E = 60°
(b) DF = 5 cm, ∠E = 60°
(c) DF = 5 cm, ∠F = 60°
(d) DE = 5 cm, ∠D = 40°
Correct Answer is Option (b)
Given that: In ΔABC, AB = 5 cm, B = ∠40° and ∠A = 80°
Using angles sum property of triangle, we have
∠A + ∠B + ∠C = 180°
80° + 40° + ∠C = 180
120° + ∠C = 180° [∠B = 40° and ∠A = 80° ]
∠C = 180° – 120°
∠C = 60°
It is given that ABC FDE, so we have
AB = FD, BC = DE and AC = FE & ∠A = ∠F, ∠B = ∠D and ∠C = ∠E
⇒ AB = FD = 5cm and ∠C = ∠E = 60°.
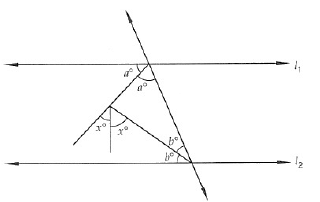
Q.11: In Fig. if l1 || l2, what is the value of x?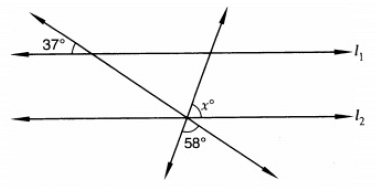
(a) 85°
(b) 75°
(c) 70°
(d) 90°
Correct Answer is Option (a)
l1‖ l2
Let transversal P and Q cuts them
∠1 = 37o
∠4 = 58o
∠5 = xo
∠1 = ∠2 = 37o (Corresponding angles) (i)
∠2 = ∠3 (Vertically opposite angle)
∠3 = 37o
∠3 + ∠4 + ∠5 = 180o (Linear pair)
37o + 58o + x = 180o
x = 85o
Q.12: An irrational number between 2 and 2.5 is
(a) √5
(b) √11
(c) √22.5
(d) √12.5
Correct Answer is Option (a)
√5 2.23606797749978969, Which is a non-terminating and non- repeating decimal therefore it is an irrational and also lies between 2 and 2,5
Q.13: (-2 - √3)(-2 + √3) when simplified is
(a) negative and irrational
(b) positive and irrational
(c) negative and rational
(d) positive and rational
Correct Answer is Option (d)
(-2 - √3)(-2 + √3)
(-2)2 - (√3)2
4 - 3
1 positive and rational
Q.14: The perpendicular distance of the point P(-3, -6) from the x-axis is
(a) 3 units
(b) 6 units
(c) -2
(d) -3
Correct Answer is Option (b)
Perpendicular distance of a point from x-axis is y-ordinate of the given point is (-3, - 6).Distance = 6 unit
Q.15: Solution of the equation 3x - y = 3 is
(a) (0, -3)
(b) (3, 6)
(c) All of these
(d) (2, 3)
Correct Answer is Option (c)
All of these. All the options satisfy the given equation.
Q.16: In the given figure, EAD ⊥ BCD. Ray FAC cuts ray EAD at a point A such that ∠EAF = 30° . Also, in ΔBAC = x° , and ∠ABC = (x + 10)°. Then, the value of x is
(a) 35
(b) 30
(c) 25
(d) 20
Correct Answer is Option (c)
In the given figure ∠CAD = ∠EAF (Vertically opposite angels)
∴ ∠CAD = 30°
In ΔABD,
∠ABD + ∠B AD + ∠ZADB = 180° (Angle sum property)
⇒ (x + 10)° (x + 30)° + 90° = 180°
⇒ 2x + 130° = 180°
⇒ 2x = 180° - 130° = 50°
⇒ x = 25
Thus, the value of x is 25.
Hence, the correct answer is 25.
Q.17: If the perimeter and base of an isosceles triangle are 11 cm and 5 cm respectively, then its area is
(a) 5/2 √11 cm2
(b) 5/4 √11 cm2
(c) 5/8 √11 cm2
(d) 5 √11 cm2
Correct Answer is Option (b)
Let each of the equal sides be x cm. Then
x + x + 5 = 11
⇒ 2x = 6
⇒ x = 3 cm
=
=
= 5/4 √11 cm2
Q.18: Observe the table given below and choose the correct alternative: The class mark for R is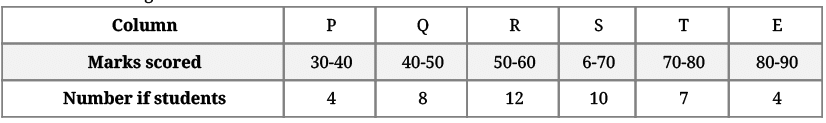
(a) 55
(b) 50
(c) 60
(d) 45
Correct Answer is Option (a)
Class mark = ( upper limit + Lower limit ) / 2
= 50 + 60 / 2
= 110 / 2
= 55
So class mark is 55
Q.19: Which of the following is an irrational number
(a) √225
(b) 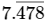
(c) √23
(d) 0.3799
Correct Answer is Option (c)
It doesn't represent the form of p/q and q ≠ 0
Q.20: If the perpendicular distance of a point P from the x-axis is 5 units and the foot of the perpendicular lies on the negative direction of x-axis, then the point P has
(a) y coordinate = – 5 only
(b) y coordinate = 5 only
(c) y coordinate = 5 or –5
(d) x coordinate = – 5
Correct Answer is Option (c)
We know that, the perpendicular distance of a point from the X-axis gives y-coordinate of that point. Here, foot of perpendicular lies on the negative direction of X-axis, so perpendicular distance can be measure in II quadrant or III quadrant. Hence, the point P has y-coordinate = 5 or -5.
Section - B
Q.21: The graph of the linear equation 2x + 5y = 10 meets the x-axis at the point.
(a) (2, 0)
(b) (5, 0)
(c) (0, 5)
(d) (0, 2)
Correct Answer is Option (b)
If the graph of the linear equation 2x + 5y = 10 meets the x-axis, then y = 0.
Substituting the value of y = 0 in equation 2x + 5y = 10, we get
2x + 5(0) = 10
⇒ 2x = 10
⇒ x = 10/2
⇒ x = 5
So, the point of meeting is (5, 0)
Q.22: In ΔABC, it is given that base = 12 cm and height = 5 cm. Its area is
(a) 60 cm2
(b) 30 cm2
(c) 15√3 cm2
(d) 30 cm2
Correct Answer is Option (b)
30 cm2
Area of triangle = 1/2 x Base Height
Area of ΔABC = 1/2 x12 x 5
= 30cm2
Q.23: The line represented by the equation x + y = 16 passes through (2, 14). How many more lines pass through the point (2, 14)
(a) 10
(b) 2
(c) many
(d) 100
Correct Answer is Option (c)
There are many lines pass through the point (2, 14).For example
x - y = -12
2x + y = 18
and many more.
Q.24: In the given figure the value of x =?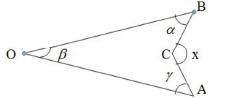
(a) α +β - γ
(b) α +γ - β
(c) α +β + γ
(d) α - β - γ
Correct Answer is Option (c)
OBCA is a quadrilateral
∠OAC + ∠BOA + ∠ACB + ∠CBO = 360°
γ + β + ACB + α = 360°∠ACB = 360° - γ- β - α
x = 360° - ∠ACB
x = α +β + γ
Q.25: If 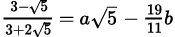 , then the value of ‘b’ is
, then the value of ‘b’ is
(a) 3
(b) 1
(c) -1
(d) 2
Correct Answer is Option (b)
taking LHS
⇒
⇒
⇒
⇒
⇒equating this with RHS,
we get,
⇒ b = 1
Q.26: The length of the sides of a triangle are 5 cm, 7 cm and 8 cm. Area of the triangle is:
(a) 100√3 cm2
(b) 10√3 cm2
(c) 300 cm2
(d) 50√3 cm2
Correct Answer is Option (b)
s == 10 cm
Area of triangle =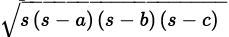
=
=
= 10√3 cm2
Q.27: The mean of first n natural numbers is
(a) 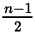
(b) 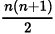
(c) 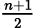
(d) 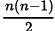
Correct Answer is Option (c)
The mean is equal to the sum of all the values in the data set divided by the number of values in the data set.
Sum of first n natural numbers is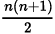
So, mean of first n natural numbers is
Q.28: If y2 = 625 then y is
(a) an irrational number
(b) a natural number
(c) a rational number
(d) neither rational nor irrational
Correct Answer is Option (c)
a rational number
y2 = 625
⇒ y = √625
⇒ y = √25 x √25
⇒ y = 25
Q.29: In Fig., if AB || CD, then the value of x is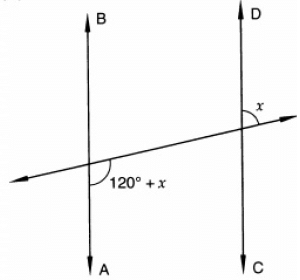
(a) 15
(b) 20
(c) 30
(d) 12
Correct Answer is Option (c)
In the given figure we have,
120° +x+x = 180°
⇒ 2x = 180° - 120°
or, x = 60/2 = 30°
Q.30: In the 'less than' type of ogive the cumulative frequency is plotted against
(a) any value of the concerned class interval
(b) the mid-value of the concerned class interval
(c) the upper limit of the concerned class interval
(d) the lower limit of the concerned class interval
Correct Answer is Option (c)
In a less than o-give we plot the points with the upper limits of the class as abscissae and the corresponding less than cumulative frequency as ordinates. It is a rising curve
Q.31: What is the area of an equilateral triangle with side 2 cm?
(a) 6 cm2
(b) 3√3 cm2
(c) √3 cm2
(d) 4√3 cm2
Correct Answer is Option (c)
Area of an equilateral triangle
Here, side = 2 cm
area ⇒
⇒
Hence the area will be √3 cm sq. unit.
Q.32: 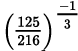 =
=
(a) 6/5
(b) 125
(c) 5/6
(d) 216
Correct Answer is Option (a)
⇒
⇒
⇒ 6/5
Q.33: An exterior angle of a triangle is equal to 100° and two interior opposite angles are equal. Each of these angles is equal to
(a) 40°
(b) 80°
(c) 75°
(d) 50°
Correct Answer is Option (d)
Let the two interior opposite angles be x° each.
Now, the exterior angle is equal to the sum of the two interior opposite angles.
x° + x° = 180°
⇒ 2x° = 100°
⇒ x° = 50°
Q.34: To analyse the election results, the data is collected from a newspapers. The data thus collected is known as
(a) raw data
(b) primary data
(c) secondary data
(d) grouped data
Correct Answer is Option (c)
Secondary data is the readily available data collected by someone else & published in newspapers or journals etc.
Q.35: In the figure, if l ║m what is the value of x.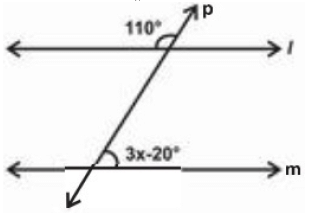
(a) 43°
(b) 37°
(c) 30°
(d) 70°
Correct Answer is Option (c)
Clearly, we have
3x - 20 + 110 = 180
3x + 90 = 180
3x = 90
x = 30
Q.36: The distance between the graphs of the equations y = -1 and y = 3 is
(a) 4
(b) 1
(c) 3
(d) 2
Correct Answer is Option (a)
Distance between the graphs of the equations y = -1 and y = 3 is = 3 – (-1) = 4 units
Q.37: In Figure, if l1|| l2, the value of x is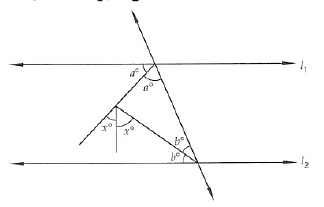
(a) 60
(b) 45
(c) 22 x 1/2
(d) 30
Correct Answer is Option (b)
From figure,
∠ACS = 180° -2b°
Also ∠ACS = ∠PAC = 2a°
⇒ 2a° = 180° -2b°
⇒ a° + b° = 90°
Now, in ΔABC
⇒ a° + b° + ∠ ABC = 180°
⇒ ∠ ABC = 180° - 2x°
⇒ a° + b° + 180° - 2x°180°
⇒x = 45°
Q.38: Which of the following is an irrational number?
(a) √20 / √5
(b) √4 / 9
(c) √64
(d) √7
Correct Answer is Option (d)
Because it cannot be express in p/q form Or fraction
Q.39: In a bar graph if 1 cm represents 30 km, then the length of bar needed to represent 75 km is
(a) 3.5 cm
(b) 2.5 cm
(c) 2 cm
(d) 3 cm
Correct Answer is Option (b)
1 cm = 30 km
So for 75 km
75/30 = 2.5 cm
Q.40: How is a histogram different from bar graph ?
(a) No difference
(b) We use class - intervals in histogram instead to variables.
(c) The histogram is same as bar graph but joined together.
(d) Both Histogram is same as bar graph but joined together and no difference are correct
Correct Answer is Option (b)
A bar chart is used in summarizing categorical data. It uses a number of rectangles, of (arbitrary) equal width, corresponding to each category and have heights equal to number of cases in each category. Bar charts can be drawn horizontally or vertically, usually with gaps between rectangles.
While a histogram is used when data are measured in intervals; discrete or continuous. It illustrates the distribution of the data in a convenient form. Usually convenient if number of observations is very large, ( > ~ 100). Here width of rectangle is decided by the range of data values and can be varied, (but fixed in a plot) and are usually drawn without gaps. Therefore the area of the rectangle is key factor in histograms. Histograms are appropriate for numerical variables only and are helpful in identifying gaps in observations.
Section - c
Question No. 41 to 45 are based on the given text. Read the text carefully and answer the questions:
There were two parallel roads AM and XY in New Delhi. Due to increasing pollution, MCD planned to get planted trees on these roads.
On the road AM, plants of Ashoka were planted by one company. While on the road XY mango trees were planted by another company.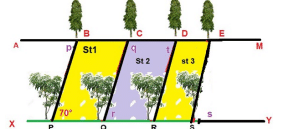
Between these roads three streets St 1, St 2 and St 3 were situated.
During the survey, ∠BPQ was measured to be 70° and other angles p, q, r, s and t were also measured.
Q.41: What is the measure of √p?
(a) 70°
(b) 100°
(c) 160°
(d) 60°
Correct Answer is Option (a)
70°
Q.42: What is the measure of √q?
(a) 160°
(b) 70°
(c) 60°
(d) 110°
Correct Answer is Option (d)
110°
Q.43: What is the value of 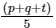 ?
?
(a) 70°
(b) 50°
(c) 160°
(d) 100°
Correct Answer is Option (b)
50°
Q.44: What is the measure of ∠EsY?
(a) 60°
(b) 70°
(c) 140°
(d) 110°
Correct Answer is Option (b)
70°
Q.45: What is value of {4p - (q + r) - (r - s)}?
(a) 160°
(b) 50°
(c) 100°
(d) 180°
Correct Answer is Option (c)
100°
Question No. 46 to 50 are based on the given text. Read the text carefully and answer the questions:
In an effort to provide high-quality and safe playgrounds for kids, our reputable manufacturers adhere to the playground safety guidelines set forth by the Indian Consumer Product Safety Commission (CPSC) and the Indian Society for Advancement of Materials and Processing Engineering (ISAMPE). These organizations set the guidelines for determining the types of playground equipment that is appropriate for kids within specific age groups: 2-3 years, 3-5 years, 5-7 years, 7-10 years, 10-15 years, and 15-17 years. A random survey of the number of children of various age groups playing in a park was found as follows:

The histogram is as given below: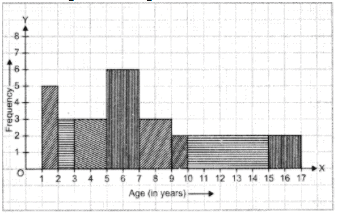
Q.46: In this question, the class sizes are different. So, calculate the adjusted frequency for each class by using the following formula:
Frequency density or adjusted frequency for class =
(a) 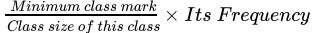
(b) 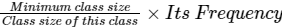
(c) 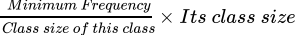
(d) 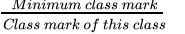
Correct Answer is Option (b)
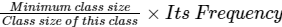
Q.47: In this question the minimum class size is
(a) 0
(b) 3
(c) 2
(d) 1
Correct Answer is Option (d)
1
Q.48: The class limits of third class interval 3-5
(a) lower limit = 5, upper limit = 7
(b) lower limit = 7, upper limit = 5
(c) lower limit = 5, upper limit = 3
(d) lower limit = 3, upper limit = 5
Correct Answer is Option (d)
lower limit = 3, upper limit = 5
Q.49: Adjusted Frequency for class interval 5-7 and 7-10
(a) 6, 6
(b) 3, 6
(c) 3, 3
(d) 6, 3
Correct Answer is Option (d)
6, 3
Q.50: Find the class mark of class 15 - 17.
(a) 25
(b) 16
(c) 2
(d) 12
Correct Answer is Option (b)
16
|
40 videos|471 docs|57 tests
|
FAQs on Class 9 Math: Sample Question Paper Term I- 1 (With Solutions) - Mathematics (Maths) Class 9
| 1. What is the format of the Class 09 Mathematics exam? |  |
| 2. How much time is allocated for each section in the Class 09 Mathematics exam? |  |
| 3. What is the maximum marks for each section in the Class 09 Mathematics exam? |  |
| 4. What topics are covered in Section A of the Class 09 Mathematics exam? |  |
| 5. What can I expect in Section C of the Class 09 Mathematics exam? |  |