Hooke’s Law & Stress-Strain Curve | Physics for JAMB PDF Download
By now, we know that the stress and strain take different forms in different situations. In this article, we will understand the relationship between stress and strain by looking at the Hooke’s law and the stress-strain curve.
The following figure shows some examples.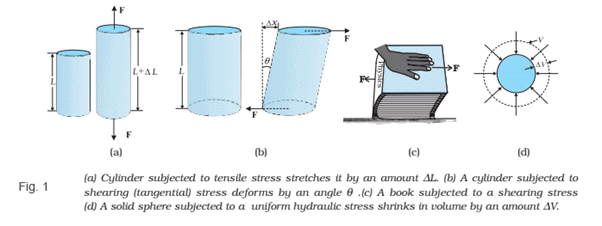
Hooke’s Law
Hooke’s Law states that for small deformities, the stress and strain are proportional to each other. Thus,
Stress ∝ Strain
Or, Stress = k × Strain … where k is the constant of proportionality and is the Modulus of Elasticity. It is important to note that Hooke’s Law is valid for most materials.
Stress-Strain Curve
To determine the relation between the stress and strain for a given material, let’s conduct an experiment. Take a test cylinder or wire and stretch it by an applied force. Record the fraction change in length (strain) and the applied force needed to cause the strain. Increase the applied force gradually, in steps, and record the readings.
Now, plot a graph between the stress (which is equal in magnitude to the applied force per unit area) and the strain produced. The graph for a typical metal looks as follows: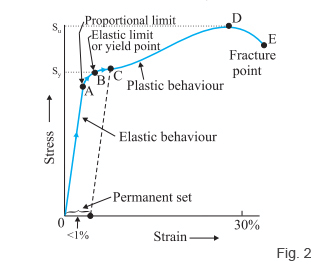
The stress-strain curves can vary with the material in question. With the help of such curves, we can understand how the material deforms with increasing loads.
Analysis of the Curve
In Fig. 2, we can see that in the region between O and A, the curve is linear. Hence, Hooke’s Law obeys in this region. In the region from A to B, the stress and strain are not proportional. However, if we remove the load, the body returns to its original dimension.
The point B in the curve is the Yield Point or the elastic limit and the corresponding stress is the Yield Strength (Sy) of the material. Once the load is increased further, the stress starting exceeding the Yield Strength. This means that the strain increases rapidly even for a small change in the stress.
This is shown in the region from B to D in the curve. If the load is removed at, say a point C between B and D, the body does not regain its original dimension. Hence, even when the stress is zero, the strain is not zero and the deformation is called plastic deformation.
Further, the point D is the ultimate tensile strength (Su) of the material. Hence, if any additional strain is produced beyond this point, a fracture can occur (point E). If,
- The ultimate strength and fracture points are close to each other (points D and E), then the material is brittle.
- The ultimate strength and fracture points are far apart (points D and E), then the material is ductile.
Exceptions
Remember, the stress-strain behavior varies from material to material. Rubber, for example, can be stretched up to several times its original length and it still returns to its original shape. The figure below shows the stress-strain curve for the elastic tissue or aorta, resent in the heart.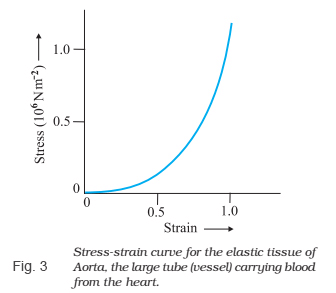
From the curve, you can observe that while the elastic region is very large, the material does not obey Hooke’s Law. Also, there is no well-defined plastic region. Materials like rubber, tissue or the aorta, etc. which can be stretched to cause large strains are called elastomers.
Solved Question
Q. Hooke’s law essentially defines
(a) Stress
(b) Strain
(c) Yield Point
(d) Elastic Limit
Ans: (d)
Solution: Hooke’s law is a principle which states that the force needed to extend or compress a spring by some distance is proportional to that distance. This proportionality constant defines the elastic limit.
|
260 videos|253 docs|230 tests
|





















