Q.1. Which of the following pairs of linear equations has unique solution, no solution, or infinitely many solutions. In case there is a unique solution, find it by using cross multiplication method.
(i) x – 3y – 3 = 0 and 3x – 9y – 2 = 0
(ii) 2x + y = 5 and 3x + 2y = 8
(iii) 3x – 5y = 20 and 6x – 10y = 40
(iv) x – 3y – 7 = 0 and 3x – 3y – 15 = 0
Solution:
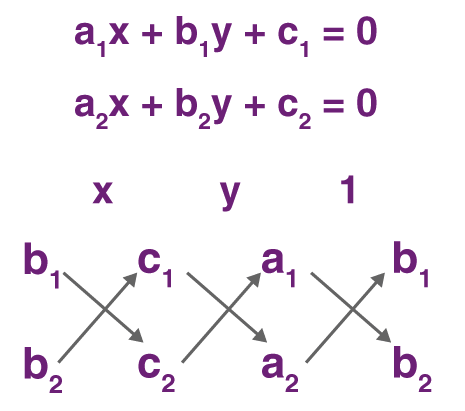 Cross Multiplication Method
Cross Multiplication Method
(i) Given, x – 3y – 3 =0 and 3x – 9y - 2 =0
a1 / a2 = 1 / 3, b1 / b2 = -3 / -9 = 1 / 3, c1 / c2 = -3 / -2 = 3 / 2
(a1 / a2) = (b1 / b2) ≠ (c1 / c2)
Since, the given set of lines are parallel to each other they will not intersect each other and therefore there is no solution for these equations.
(ii) Given, 2x + y = 5 and 3x + 2y = 8
a1 / a2 = 2 / 3, b1 / b2 = 1 / 2, c1 / c2 = -5 / -8
(a1 / a2) ≠ (b1 / b2)
Since they intersect at a unique point these equations will have a unique solution by cross multiplication method:
x / (b1c2 - c1b2) = y / (c1a2 – c2a1) = 1 / (a1b2 - a2b1)
x / (-8 - (-10)) = y / (15 + 16) = 1 / (4 - 3)
x / 2 = y / 1 = 1
∴ x = 2 and y = 1
(iii) Given, 3x – 5y = 20 and 6x – 10y = 40
(a1 / a2) = 3 / 6 = 1 / 2
(b1 / b2) = -5 / -10 = 1 / 2
(c1 / c2) = 20 / 40 = 1 / 2
a1 / a2 = b1 / b2 = c1 / c2
Since the given sets of lines are overlapping each other there will be infinite number of solutions for this pair of equation.
(iv) Given, x – 3y – 7 = 0 and 3x – 3y – 15 = 0
(a1 / a2) = 1 / 3
(b1 / b2) = -3 / -3 = 1
(c1 / c2) = -7 / -15
a1 / a2 ≠ b1 / b2
Since this pair of lines are intersecting each other at a unique point, there will be a unique solution.
By cross multiplication,
x / (45 - 21) = y / (-21 + 15) = 1 / (-3 + 9)
x / 24 = y / -6 = 1 / 6
x / 24 = 1 / 6 and y / -6 = 1 / 6
∴ x = 4 and y = 1.
Q.2. (i) For which values of a and b does the following pair of linear equations have an infinite number of solutions?
2x + 3y = 7
(a – b) x + (a + b) y = 3a + b – 2
(ii) For which value of k will the following pair of linear equations have no solution?
3x + y = 1
(2k – 1) x + (k – 1) y = 2k + 1
Solution: (i) 3y + 2x - 7 = 0
(a + b)y + (a-b)y – (3a + b -2) = 0
a1 / a2 = 2 / (a - b), b1 / b2 = 3 / (a + b), c1 / c2 = -7 / -(3a + b - 2)
For infinitely many solutions,
a1 / a2 = b1 / b2 = c1 / c2
Thus 2 / (a - b) = 7 / (3a + b – 2)
6a + 2b – 4 = 7a – 7b
a – 9b = -4 ………………….(i)
2 / (a - b) = 3 / (a + b)
2a + 2b = 3a – 3b
a – 5b = 0 …………………(ii)
Subtracting (i) from (ii), we get
4b = 4
b =1
Substituting this eq. in (ii), we get
a - 5 x 1= 0
a = 5
Thus at a = 5 and b = 1 the given equations will have infinite solutions.
(ii) 3x + y - 1 = 0
(2k - 1)x + (k - 1)y – 2k -1 = 0
a1 / a2 = 3 / (2k - 1), b1 / b2 = 1 / (k - 1), c1 / c2 = -1 / (-2k - 1) = 1 / ( 2k + 1)
For no solutions
a1 / a2 = b1 / b2 ≠ c1 / c2
3 / (2k - 1) = 1 / (k - 1) ≠ 1 / (2k + 1)
3 / (2k – 1) = 1 / (k - 1)
3k - 3 = 2k - 1
k = 2
Therefore, for k = 2 the given pair of linear equations will have no solution.
Q.3. Solve the following pair of linear equations by the substitution and cross-multiplication methods:
8x + 5y = 9
3x + 2y = 4
Solution: 8x + 5y = 9 …………………..(1)
3x + 2y = 4 ……………….….(2)
From equation (2) we get
x = (4 – 2y ) / 3 ……………………. (3)
Using this value in equation 1, we get
8(4 - 2y) / 3 + 5y = 9
32 – 16y + 15y = 27
-y = -5
y = 5 ………………………(4)
Using this value in equation (2), we get
3x + 10 = 4
x = -2
Thus, x = -2 and y = 5.
Now, Using Cross Multiplication method:
8x + 5y – 9 = 0
3x + 2y – 4 = 0
x / (-20 + 18) = y / (-27 + 32 ) = 1 / (16 - 15)
-x / 2 = y / 5 =1 / 1
∴ x = -2 and y = 5.
Q.4. Form the pair of linear equations in the following problems and find their solutions (if they exist) by any algebraic method:
(i) A part of monthly hostel charges is fixed and the remaining depends on the number of days one has taken food in the mess. When a student A takes food for 20 days she has to pay Rs.1000 as hostel charges whereas a student B, who takes food for 26 days, pays Rs.1180 as hostel charges. Find the fixed charges and the cost of food per day.
(ii) A fraction becomes 1/3 when 1 is subtracted from the numerator and it becomes 1/4 when 8 is added to its denominator. Find the fraction.
(iii) Yash scored 40 marks in a test, getting 3 marks for each right answer and losing 1 mark for each wrong answer. Had 4 marks been awarded for each correct answer and 2 marks been deducted for each incorrect answer, then Yash would have scored 50 marks. How many questions were there in the test?
(iv) Places A and B are 100 km apart on a highway. One car starts from A and another from B at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars?
(v) The area of a rectangle gets reduced by 9 square units, if its length is reduced by 5 units and breadth is increased by 3 units. If we increase the length by 3 units and the breadth by 2 units, the area increases by 67 square units. Find the dimensions of the rectangle.
Solution: (i) Let x be the fixed charge and y be the charge of food per day.
According to the question,
x + 20y = 1000……………….. (i)
x + 26y = 1180………………..(ii)
Subtracting (i) from (ii) we get
6y = 180
y = Rs.30
Using this value in equation (ii) we get
x = 1180 - 26 x 30
x = Rs.400.
Therefore, fixed charges is Rs.400 and charge per day is Rs.30.
(ii) Let the fraction be x / y.
So, as per the question given,
(x - 1) / y = 1 / 3 => 3x – y = 3…………………(1)
x / (y + 8) = 1 / 4 => 4x – y = 8 ………………..(2)
Subtracting equation (1) from (2) , we get
x = 5 ……………………………(3)
Using this value in equation (2), we get,
(4 × 5) – y = 8
y = 12
Therefore, the fraction is 5 / 12.
(iii) Let the number of right answers is x and number of wrong answers be y
According to the given question;
3x − y = 40……..(1)
4x − 2y = 50
⇒ 2x − y = 25…….(2)
Subtracting equation (2) from equation (1), we get;
x = 15 ….….(3)
Putting this in equation (2), we obtain;
30 – y = 25
Or y = 5
Therefore, number of right answers = 15 and number of wrong answers = 5
Hence, total number of questions = 20
(iv) Let x km/h be the speed of car from point A and y km/h be the speed of car from point B.
If the car travels in the same direction,
5x – 5y = 100
x – y = 20 …………………(i)
If the car travels in the opposite direction,
x + y = 100……………(ii)
Solving equation (i) and (ii), we get
x = 60 km/h………………………(iii)
Using this in equation (i), we get,
60 – y = 20
y = 40 km/h
Therefore, the speed of car from point A = 60 km/h
Speed of car from point B = 40 km/h.
(v) Let,
The length of rectangle = x unit
And breadth of the rectangle = y unit
Now, as per the question given,
(x – 5) (y + 3) = xy - 9
3x – 5y – 6 = 0……………………………(1)
(x + 3) (y + 2) = xy + 67
2x + 3y – 61 = 0…………………………..(2)
Using cross multiplication method, we get,
x / (305 + 18) = y / (-12 + 183) = 1 / (9 + 10)
x / 323 = y / 171 = 1 / 19
Therefore, x = 17 and y = 9.
Hence, the length of rectangle = 17 units
And breadth of the rectangle = 9 units
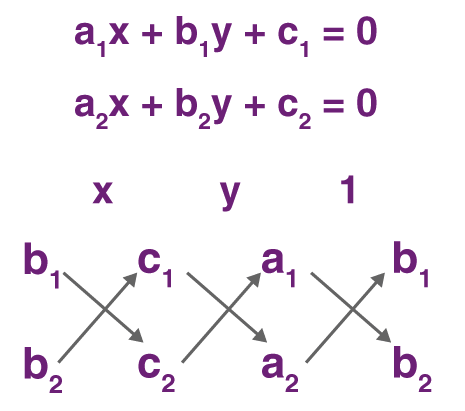 Cross Multiplication Method
Cross Multiplication Method


























