Conduction: One Dimensional - 5 | Heat Transfer - Mechanical Engineering PDF Download
Conduction: One Dimensional, Heat Transfer
2.4 Heat conduction in bodies with heat sources
The cases considered so far have been those in which the heat conducting solid is free of internal heat generation. However, the situations where the internal heat is generated are very common cases in chemical industries for example, the exothermic reaction in the solid pallet of a catalyst.
We have learnt that how the Fourier equation is used for the steady-state heat conduction through the composites of three different geometries that were not having any heat source in it. However, the heat generation term would come into the picture for these geometries. It would not be always easier to remember and develop heat conduction relations for different standard and non-standard geometries. Therefore, at this point we should learn how to develop a general relation for the heat conduction that should be applicable to the entire situation such as steady-state, unsteady state, heat source, different geometry, heat conduction in different direction, etc. Again here we will consider that the solid is isotropic in nature, which means the thermal conductivity of the material is same in all the direction of heat flow.
To get such a general equation the differential form of the heat conduction equation is most important. For simplicity, we would consider an infinitesimal volume element in a Cartesian coordinate system. The dimensions of the infinitesimal volume element are dx , dy , and dz in the respective direction as shown in the fig.2.11.
Fig.2.11. Volume element for deriving general equation of heat conduction in cartesian coordinate
The fig.2.11 shows that the heat is entering into the volume element from three different faces of the volume element and leaving from the opposite face of the control element. The heat source within the volume element generates the volumetric energy at the rate of
According to Fourier’s law of heat conduction, the heat flowing into the volume element from the left (in the x-direction) can be written as,
The heat flow out from the right surface (in the x-direction) of the volume element can be obtained by Taylor series expansion of the above equation. As the volume element is of infinitesimal volume, we may retain only first two element of the Taylor series expansion with a reasonable approximation (truncating the higher order terms). Thus,
or
The left side of the above equation represent the net heat flow in the x-direction. If we put the value of in the right side of the above equation,
In a similar way we can get the net heat flow in the y and z-directions,
and
As we know some heat is entering, some heat is leaving and some heat in generating in the volume element and as we have not considered any steady state assumption till now, thus because of all these phenomena some of the heat will be absorbed by the element. Thus the rate of change of heat energy 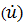 within the volume element can be written as,
within the volume element can be written as,
where, cp is the specific heat capacity at constant pressure (J/(kg·K)), ρ is the density (kg/m3) of the material, and t is the time (s).
We know all the energy term related to the above problem, and with the help of energy conservation,
On putting all the values in the above equation,
or,
or
or
As we have considered that the thermal conductivity of the solid is isotropic in nature, the above relation reduces to,
or
or
or
where is the thermal diffusivity of the material and its unit m2/s signifies the rate at which heat diffuses in to the medium during change in temperature with time. Thus, the higher value of the thermal diffusivity gives the idea of how fast the heat is conducting into the medium, whereas the low value of the thermal diffusivity shown that the heat is mostly absorbed by the material and comparatively less amount is transferred for the conduction. The
called the Laplacian operator, and in Cartesian coordinate it is defined as
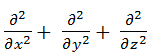
Equation 2.19 is known as general heat conduction relation. When there is no heat generation term the eq.2.19 will become,
(2.20)
and the equation is known as Fourier Field Equation.
|
55 videos|108 docs|86 tests
|
FAQs on Conduction: One Dimensional - 5 - Heat Transfer - Mechanical Engineering
| 1. What is conduction in the context of chemical engineering? |  |
| 2. How does one-dimensional conduction differ from other types of conduction? |  |
| 3. What factors affect the rate of conduction in chemical processes? |  |
| 4. How can one calculate the rate of heat conduction in a one-dimensional system? |  |
| 5. What are some practical applications of one-dimensional conduction in chemical engineering? |  |





















