Open App

Class 8 Exam > Class 8 Notes > Mathematics (Maths) Class 8 > Important Questions: Factorisation

Class 8 Maths Chapter 12 Important Question Answers - Factorisation

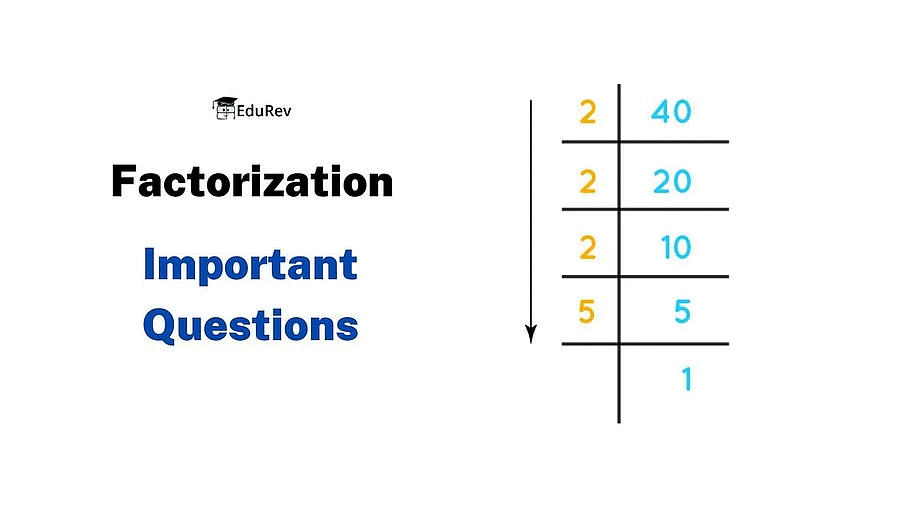
Q1: Find the common factors of the following terms.
(a) 25x²y, 30xy²
(b) 63m³n, 54mn⁴
Sol:
(a) 25x²y, 30xy²
25x²y = 5 × 5 × x × x × y
30xy² = 2 × 3 × 5 × x × y × y
Common factors are 5× x × y = 5 xy
(b) 63m³n, 54mn⁴
63m³n = 3 × 3 × 7 × m × m × m × n
54mn⁴ = 2 × 3 × 3 × 3 × m × n × n × n × n
Common factors are 3 × 3 × m × n = 9mn

Q2: Factorise the following expressions.
(a) 54m³n + 81m⁴n²
(b) 15x²y³z + 25x³y²z + 35x²y²z²
Sol:
(a) 54m³n + 81m⁴n²
= 2 × 3 × 3 × 3 × m × m × m × n + 3 × 3 × 3 × 3 × m × m × m × m × n × n
= 3 × 3 × 3 × m × m × m × n × (2 + 3 mn)
= 27m³n (2 + 3mn)

(b) 15x²y³z + 25 x³y²z + 35x²y²z² = 5x²y²z ( 3y + 5x + 7)

Q3: Factorise the following polynomials.
(a) 6p(p – 3) + 1 (p – 3)
(b) 14(3y – 5z)³ + 7(3y – 5z)²
Sol: (a) 6p(p – 3) + 1 (p – 3) = (p – 3) (6p + 1)
(b) 14(3y – 5z)³ + 7(3y – 5z)²
= 7(3y – 5z)² [2(3y – 5z) +1]
= 7(3y – 5z)² (6y – 10z + 1)

Q4: Factorise the following:
(a) p²q – pr² – pq + r²
(b) x² + yz + xy + xz
Sol: (a) p²q – pr² – pq + r²
= (p²q – pq) + (-pr² + r2)
= pq(p – 1) – r²(p – 1)
= (p – 1) (pq – r²)
(b) x² + yz + xy + xz
= x² + xy +xz + yz
= x(x + y) + z(x + y)
= (x + y) (x + z)

Q5: Factorise the following polynomials.
(a) xy(z² + 1) + z(x² + y²)
(b) 2axy² + 10x + 3ay² + 15
Sol:
(a) xy(z² + 1) + z(x² + y²)
= xyz² + xy + 2x² + zy²
= (xyz² + zx²) + (xy + zy²)
= zx(yz + x) + y(x + yz)
= zx(x + yz) + y(x + yz)
= (x + yz) (zx + y)
(b) 2axy² + 10x + 3ay² + 15
= (2axy² + 3ay²) + (10x + 15)
= ay²(2x + 3) +5(2x + 3)
= (2x + 3) (ay² + 5)

Q6: Factorise the following expressions.
(а) x² + 4x + 8y + 4xy + 4y²
(b) 4p² + 2q² + p²q² + 8
Sol: (a) x² + 4x + 8y + 4xy + 4y²
= (x² + 4xy + 4y²) + (4x + 8y)
= (x + 2y)² + 4(x + 2y)
= (x + 2y)(x + 2y + 4)
(b) 4p² + 2q² + p²q² + 8
= (4p² + 8) + (p²q² + 2q²)
= 4(p² + 2) + q²(p² + 2)
= (p² + 2)(4 + q²)

Q7: Factorise:
(a) a² + 14a + 48
(b) m² – 10m – 56
Sol:
(a) a² + 14a + 48
= a² + 6a + 8a + 48
[6 + 8 = 14 ; 6 × 8 = 48]
= a(a + 6) + 8(a + 6)
= (a + 6) (a + 8)
(b) m² – 10m – 56
= m² – 14m + 4m – 56
[14 – 4 = 10; 4 × 4 = 56]
= m(m – 14) + 6(m – 14)
= (m – 14) (m + 6)

Q8: Factorise:
(a) x⁴ – (x – y)⁴
(b) 4x² + 9 – 12x – a² – b² + 2ab
Sol: (a) x⁴ – (x – y)⁴
= (x²)² – [(x – y)²]²
= [x² – (x – y)²] [x² + (x – y)²]
= [x + (x – y] [x – (x – y)] [x² + x² – 2xy + y²]
= (x + x – y) (x – x + y)[2x² – 2xy + y²]
= (2x – y) y(2x² – 2xy + y²)
= y(2x – y) (2x² – 2xy + y²)
(b) 4x² + 9 – 12x – a² – b² + 2ab
= (4x² – 12x + 9) – (a² + b² – 2ab)
= (2x – 3)² – (a – b)²
= [(2x – 3) + (a – b)] [(2x – 3) – (a – b)]
= (2x – 3 + a – b)(2x – 3 – a + b)

Q9: Factorise the following polynomials.
(a) 16x⁴ – 81
(b) (a – b)² + 4ab
Sol: (a) 16x⁴ – 81
= (4x²)² – (9)2
= (4x² + 9)(4x² – 9)
= (4x² + 9)[(2x)² – (3)²]
= (4x² + 9)(2x + 3) (2x – 3)
(b) (a – b)² + 4ab
= a² – 2ab + b² + 4ab
= a² + 2ab + b²
= (a + b)²

Q10: Factorise:
(а) 14m⁵n⁴p² – 42m⁷n³p⁷ – 70m⁶n⁴p³
(b) 2a²(b² – c²) + b²(2c² – 2a²) + 2c²(a² – b²)
Sol:
(a) 14m⁵n⁴p² – 42m⁷n³p⁷ – 70m⁶n⁴p³
= 14m⁵n³p²(n – 3m²p⁵ – 5mnp)
(b) 2a²(b² – c²) + b²(2c² – 2a²) + 2c²(a² – b²)
= 2a²(b² – c²) + 2b²(c² – a²) + 2c²(a² – b²)
= 2[a²(b² – c²) + b²(c² – a²) + c²(a² – b²)]
= 2 × 0
= 0

Q11: Factorise:
(a) (x + y)² – 4xy – 9z²
(b) 25x² – 4y² + 28yz – 49z²
Sol: (a) (x + y)² – 4xy – 9z²
= x² + 2xy + y² – 4xy – 9z²
= (x² – 2xy + y²) – 9z²
= (x – y)² – (3z)²
= (x – y + 3z) (x – y – 3z)

(b) 25x² – 4y² + 28yz – 49z²
= 25x² – (4y² – 28yz + 49z²)
= (5x)² – (2y – 7)²
= (5x + 2y – 7) [5x – (2y – 7)]
= (5x + 2y – 7) (5x – 2y + 7)

Q12: If one of the factors of (5x² + 70x – 160) is (x – 2). Find the other factor.
Sol: Let the other factor be m.
(x – 2) × m = 5x² + 70x – 160

Q13: Express the following as in the form of (a+b)(a-b)
(i) a² – 64
(ii) 20a² – 45b²
(iii) 32x²y² – 8
(iv) x² – 2xy + y² – z²
(v) 49x² – 1
Sol: For representing the expressions in (a+b)(a-b) form, use the following formula
a² – b² = (a+b)(a-b)
(i) a² – 64 = a² – 8²= (a + 8)(a – 8)
(ii) 20a² – 45b² = 5(4a² – 9b²) = 5(2a + 3b)(2a – 3b)
(iii) 32x²y² – 8 = 8( 4x²y² – 1) = 8(2xy + 1)(2xy – 1)
(iv) x² – 2xy + y² – z² = (x – y)² – z²= (x – y – z)(x – y + z)
(v) 49x² – 1 = (7x)² – (1)² = (7x + 1)(7x – 1)

Q14: Verify whether the following equations are correct. Rewrite the incorrect equations correctly.
(i) (a + 6)² = a² + 12a + 36
(ii) (2a)² + 5a = 4a + 5a
Sol:
(i) (a + 6)² = a² + 12a + 36
Here, LHS = (a + 6)² = a² + 12a + 36
Now, RHS = a² + 12a + 36
Hence, LHS = RHS.
(ii) (2a)² + 5a = 4a + 5a
Here, LHS = (2a)² + 5a = 4a² + 5a
Now, RHS = 4a + 5a
So, LHS ≠ RHS
Correct equation: (2a)² + 5a = 4a² + 5a

Q15: For a = 3, simplify a² + 5a + 4 and a² – 5a
Sol:
Substitute the value of a = 3 in the given equations.
a² + 5a + 4 = 3² + 5(3) + 4 = 9 + 15 + 4 = 28
And,
a² – 5a = 3² – 5(3) = 9 – 15 = -6

Q16: Find the common factors of the following:
(i) 6 xyz, 24 xy² and 12 x²y
(ii) 3x² y³, 10x³y² and 6x² y² z
Sol:
(i) 6 xyz = 2 × 3 × x × y × z
24 xy² = 2 × 2 × 2 × 3 × x × y × y
12 x²y = 2 × 2 × 3 × x × x × y
Thus, the common factors are common factors of 6 xyz, 24 xy² and 12 x²y are 2, 3, x, y and, (2 × 3 × x × y) = 6xy
(ii) 3x² y³ = 3 × x × x × y × y × y
10x³ y² = 2 × 5 × x × x × x × y × y
6 x² y² z = 3 × 2 × x × x × y × y × z
Now, the common factors of 3x² y³, 10x³y² and 6x² y² z are x², y² and, (x² × y²) = x² y²

Q17: Factorize the following expressions:
(i) 54x³y + 81x⁴y²
(ii) 14(3x – 5y)³ + 7(3x – 5y)²
(iii) 15xy + 15 + 9y + 25x
Sol:
(i) 54x³y + 81x⁴y²
= 2 × 3 × 3 × 3 × x × x × x × y + 3 × 3 × 3 × 3 × x × x × x × x × y × y
= 3 × 3 × 3 × x × x × x × y × (2 + 3 xy)
= 27x³y (2 + 3 xy)

(ii) 14(3x – 5y)³ + 7(3x – 5y)²
= 7(3x – 5y)² [2(3x – 5y) +1]
= 7(3x – 5y)² (6x – 10y + 1)

(iii) 15xy + 15 + 9y + 25x
Rearrange the terms as:
15xy + 25x + 9y + 15
= 5x(3y + 5) + 3(3y + 5)
Or, (5x + 3)(3y + 5)

Q18: Factorize (x + y)² – 4xy
Sol:
To solve this expression, expand (x + y)²
Use the formula:
(x + y)² = x² + 2xy + y²
(x + y)² – 4xy = x² + 2xy + y² – 4xy
= x² + y² – 2xy
We know, (x – y)² = x² + y² – 2xy
So, factorization of (x + y)² – 4xy = (x – y)²

Q19: When we factorise an expression, we write it as a ______ of factors.
Sol: When we factorise an algebraic expression, we write it as a product of factors. These factors may be numbers, algebraic variables or algebraic expressions.

Q20: Find the common factors of 12x, 36
(a) 12
(b) 36
(c) x
(d) 12x
Ans: (a)
Sol: Here,
$12x = 2 \times 2 \times 3 \times x$
$36 = 2 \times 2 \times 3 \times 3$
So, common factor = $2 \times 2 \times 3$
$\Rightarrow 4 \times 3 = 12$
$\Rightarrow 4 \times 3 = 12$
Hence the correct option is (a).

Question for Important Questions: Factorisation

Try yourself:Q21: Factorise 49p²- 36

A.
(7p + 6)(7p + 6)
B.
(7p - 6)(7p - 6)
C.
(7p - 6)(7p + 6)
D.
(6p - 7)(7p - 6)

View Solution

Q22: Factorise using identity $x^{2} +10x+25$
Sol:

x^{2} +10x+25 = x^{2} +2 \times 5 \times x+ 5^{2}

Using the identity,

{(a + b)}^{2} = a^{2} + 2 a b + b^{2}

= x^{2} +2 \times 5 \times x+ 5^{2}

= (x+5)^{2}

= (x+5)(x+5)

Q23: Simplify $[1 0^{2} -18 \times 10+81]$

Sol: $[1 0^{2} -18 \times 10+81] = [10^{2} - 2 \times 9 \times 10 + 9^{2}]$
Using the identity, ${(a - b)}^{2} = a^{2} - 2 a b + b^{2}$
$= [10^{2} - 2 \times 9 \times 10 + 9^{2}]$
$= {[10 - 9]}^{2}$
$= {[1]}^{2}$
$= 1$

Q24: Simplify
Sol:
Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Using the identity, x²−y² = (x + y)(x − y)

Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Q25: Find x if
Sol: Ans: Taking 4 common from the numerator we get

Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Using the identity, x² − y² = (x + y)(x − y) in the numerator
Class 8 Maths Chapter 12 Important Question Answers - Factorisation

On comparing both side we can see that x = 4.

Q26: Find the remainder in the following (x⁴ - a⁴) ÷ (x² + a²)
Sol: For dividing both the equation first we have to simplify it.
So, (x⁴ − a⁴) can be written as (x²)²−(a²)².
Now by applying the identity a² − b² = (a + b)(a − b)
Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Hence the remainder is (x² - a²).

Q27: Using identity (a - b)² = a² - 2ab + b² find the value of (98)² .
Sol: (98)² can be further written as (100 - 2)²
By comparing the above equation with the identity, we get
a = 100 and b = 2
(98)2 = (100)² − 2(100)(2) + (2)²
=10000−400+4
=9604

Q28: Simplify
Sol: Identities to be used in the question are;
a²− b² = (a + b)(a − b)
Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Q29: The area of a rectangle is $6 a^{2} + 36 a$ and its width is 36a. Find its length.
Sol: Let the length of the rectangle is x
Breath = 36a
$Area of rectangle = Length \times Breath$
$6 a^{2} +36a = x \times 36a$
Class 8 Maths Chapter 12 Important Question Answers - Factorisation
Hence the length of rectangle is

Q30: The combined area of two squares is $20c m^{2}$ . Each side of one square is twice as long as a side of the other square. Find the length of the sides of each square.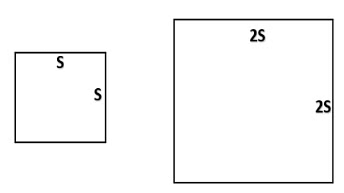

Sol: Let the side of the smaller square be S and that of the bigger square be 2S.
Combined area of the two squares = $20c m^{2}$
$S^{2} + {(2 S)}^{2} = 20$
$S^{2} + 4 S^{2} = 20$
$5 S^{2} = 20$
$S^{2} = 4$
$S = 2 c m$
Hence the side of the smaller square is 2cm and that of the bigger square is 4cm.

Q31: Find the factors of $25 x^{2} -4 y^{2} +28yz-49 z^{2}$
Sol:
$25 x^{2} -4 y^{2} +28yz-49 z^{2} = 25 x^{2} -(4 y^{2} -28yz+49 z^{2})$
$= 25 x^{2} - [(2y)^{2} -2 \times 2 y \times 7z+(7z)^{2}]$
Using the identity , ${(a - b)}^{2} = a^{2} - 2 a b + b^{2}$
${(5 x)}^{2} - {(2 y - 7 z)}^{2}$
Now using the identity , $a^{2} - b^{2} = (a + b) (a - b)$
$(5 x + 2 y - 7 z) (5 x - 2 y + 7 y)$

Q32: Factorise 6xy – 4y + 6 – 9x
(a) (3x – 2)
(b) (3x – 2)(2y – 3)
(c) (2y – 3)
(d) (2x – 3)(3y – 2)
Ans: (b)
$6 x y - 4 y + 6 - 9 x = 2 \times 3 \times x \times y - 2 \times 2 \times y + 2 \times 3 - 3 \times 3 \times x$
$= 2 y (3 x - 2) - 3 (3 x - 2)$
$= (3 x - 2) (2 y - 3)$
Hence the correct option is (b).

Q33: Simplify
Sol:
$(p^{2} +11p+28) = (p^{2} +7p+4p+28)$
$= p (p + 7) + 4 (p + 7)$
$= (p + 7) (p + 4)$
$= (p + 7) (p + 4)$
Class 8 Maths Chapter 12 Important Question Answers - Factorisation
= (p + 7)

The document Class 8 Maths Chapter 12 Important Question Answers - Factorisation is a part of the Class 8 Course Mathematics (Maths) Class 8.

All you need of Class 8 at this link: Class 8

	Mathematics (Maths) Class 8 79 videos\|408 docs\|31 tests

Mathematics (Maths) Class 8

79 videos|408 docs|31 tests

Join Course for Free

Top Courses for Class 8

View all

FAQs on Class 8 Maths Chapter 12 Important Question Answers - Factorisation

1. What is factorisation in mathematics?

Ans.Factorisation is the process of breaking down an expression into a product of its factors. For example, the factorisation of the quadratic expression $x^2 - 5x + 6$ is $(x - 2)(x - 3)$.

2. Why is factorisation important in solving equations?

Ans.Factorisation is crucial for solving equations because it allows us to transform complex expressions into simpler forms. By setting each factor to zero, we can find the solutions to the equation more easily.

3. What are some common methods of factorisation?

Ans.Common methods of factorisation include taking out the common factor, using the difference of squares, grouping, and applying the quadratic formula for quadratic expressions. Each method suits different types of expressions.

4. How can I factorise a polynomial expression?

Ans.To factorise a polynomial expression, first look for any common factors among the terms. Next, apply methods like grouping or using special products (like square of a binomial) to break down the expression further.

5. Can factorisation be used in real-life applications?

Ans.Yes, factorisation can be used in various real-life applications, such as in areas of physics for solving motion equations, in economics for optimizing functions, and in computer science for algorithm design and analysis.

Related Exams

Class 8

About this Document

	12.3K Views
	4.90/5 Rating
	Dec 22, 2024 Last updated

Document Description: Important Questions: Factorisation for Class 8 2024 is part of Mathematics (Maths) Class 8 preparation. The notes and questions for Important Questions: Factorisation have been prepared according to the Class 8 exam syllabus. Information about Important Questions: Factorisation covers topics like and Important Questions: Factorisation Example, for Class 8 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Important Questions: Factorisation.

Introduction of Important Questions: Factorisation in English is available as part of our Mathematics (Maths) Class 8 for Class 8 & Important Questions: Factorisation in Hindi for Mathematics (Maths) Class 8 course. Download more important topics related with notes, lectures and mock test series for Class 8 Exam by signing up for free. Class 8: Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Description

Full syllabus notes, lecture & questions for Class 8 Maths Chapter 12 Important Question Answers - Factorisation - Class 8 | Plus excerises question with solution to help you revise complete syllabus for Mathematics (Maths) Class 8 | Best notes, free PDF download

Information about Important Questions: Factorisation

In this doc you can find the meaning of Important Questions: Factorisation defined & explained in the simplest way possible. Besides explaining types of Important Questions: Factorisation theory, EduRev gives you an ample number of questions to practice Important Questions: Factorisation tests, examples and also practice Class 8 tests

	Mathematics (Maths) Class 8 79 videos\|408 docs\|31 tests

Mathematics (Maths) Class 8

79 videos|408 docs|31 tests

Join Course for Free

Download as PDF

Explore Courses for Class 8 exam

Top Courses for Class 8

Explore Courses

Signup for Free!

Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

Start learning for Free

10M+ students study on EduRev

Semester Notes

Objective type Questions

past year papers

Class 8 Maths Chapter 12 Important Question Answers - Factorisation

Previous Year Questions with Solutions

video lectures

Important questions

shortcuts and tricks

pdf

Free

MCQs

Viva Questions

Extra Questions

Exam

Sample Paper

Summary

ppt

mock tests for examination

Class 8 Maths Chapter 12 Important Question Answers - Factorisation

study material

practice quizzes

;

Additional Information about Important Questions: Factorisation for Class 8 Preparation

Important Questions: Factorisation Free PDF Download

The Important Questions: Factorisation is an invaluable resource that delves deep into the core of the Class 8 exam. These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective. With the help of these notes, you can grasp complex subjects quickly, revise important points easily, and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner, allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material, or toppers' notes, this PDF has got you covered. Download the Important Questions: Factorisation now and kickstart your journey towards success in the Class 8 exam.

Importance of Important Questions: Factorisation

The importance of Important Questions: Factorisation cannot be overstated, especially for Class 8 aspirants. This document holds the key to success in the Class 8 exam. It offers a detailed understanding of the concept, providing invaluable insights into the topic. By knowing the concepts well in advance, students can plan their preparation effectively. Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.

Important Questions: Factorisation Notes

Important Questions: Factorisation Notes offer in-depth insights into the specific topic to help you master it with ease. This comprehensive document covers all aspects related to Important Questions: Factorisation. It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation. Practice papers and question papers enable you to assess your progress effectively. Additionally, the paper analysis provides valuable tips for tackling the exam strategically. Access to Toppers' notes gives you an edge in understanding complex concepts. Whether you're a beginner or aiming for advanced proficiency, Important Questions: Factorisation Notes on EduRev are your ultimate resource for success.

Important Questions: Factorisation Class 8

The "Important Questions: Factorisation Class 8 Questions" guide is a valuable resource for all aspiring students preparing for the Class 8 exam. It focuses on providing a wide range of practice questions to help students gauge their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation. The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level. Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.

Study Important Questions: Factorisation on the App

Students of Class 8 can study Important Questions: Factorisation alongwith tests & analysis from the EduRev app, which will help them while preparing for their exam. Apart from the Important Questions: Factorisation, students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc. The EduRev App will make your learning easier as you can access it from anywhere you want. The content of Important Questions: Factorisation is prepared as per the latest Class 8 syllabus.

Education Revolution