NCERT Solutions for Class 8 Maths Chapter 5 - Squares and Square Roots (Exercise 5.3)
Exercise 5.3
Q1. What could be the possible ‘one’s’ digits of the square root of each of the following numbers?
(i) 9801
(ii) 99856
(iii) 998001
(iv) 657666025
Ans: The possible digit at one’s place of the square root of:
(i) 9801 can be 1 or 9.
[∵ 1 *1 = 1 and 9 * 9 = 81]
(ii) 99856 can be 4 or 6.
[∵ 4 * 4 = 16 and 6 * 6 = 36]
(iii) 998001 can be 1 or 9.
[∵ 1 *1 = 1 and 9 * 9 = 81]
(iv) 657666025 can be 5.
[∵ 5 * 5 = 25]
Q2. Without doing any calculation, find the numbers which are surely not perfect squares
(i) 153
(ii) 257
(iii) 408
(iv) 441
Ans: We know that the ending digit of perfect square is 0, 1, 4, 5, 6, and 9.
∴ A number ending in 2, 3, 7 or 8 can never be a perfect square.
(i) 153, cannot be a perfect square.
(ii) 257, cannot be a perfect square.
(iii) 408, cannot be a perfect square.
(iv) 441, can be a perfect square.
Thus, (i) 153, (ii) 257 and (iii) 408 are surely not perfect squares.
Q3. Find the square roots of 100 and 169 by the method of repeated subtraction.
Ans: (i) 100
100 – 1 = 99
99 – 3 = 96
96 – 5 = 91
91 – 7 = 84
84 – 9 = 75
75 – 11 = 64
64 – 13 = 51
51 – 15 = 36
36 – 17 = 19
19 – 19 = 0
Here, we have performed subtraction ten times.
∴ √100 = 10
(ii) 169
169 – 1 = 168
168 – 3 = 165
165 – 5 = 160
160 – 7 = 153
153 – 9 = 144
144 – 11 = 133
133 – 13 = 120
120 – 15 = 105
105 – 17 = 88
88 – 19 = 69
69 – 21 = 48
48 – 23 = 25
25 – 25 = 0
Here, we have performed subtraction thirteen times.
∴ √169 = 13
Q4. Find the square roots of the following numbers by the Prime Factorisation Method.
(i) 729
(ii) 400
(iii) 1764
(iv) 4096
(v) 7744
(vi) 9604
(vii) 5929
(viii) 9216
(ix) 529
(x) 8100
Ans:
(i)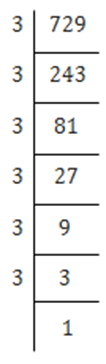
729 = 3 × 3 × 3 × 3 × 3 × 3 × 1
⇒ 729 = (3 × 3) × (3 × 3) × (3 × 3)
⇒ 729 = (3 × 3 × 3) × (3 × 3 × 3)
⇒ 729 = (3 × 3 × 3)2
⇒ √729 = 3 × 3 × 3 = 27
(ii)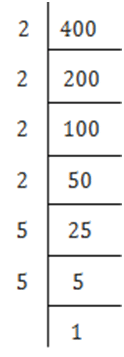
400 = 2 × 2 × 2 × 2 × 5 × 5 × 1
⇒ 400 = (2 × 2) × (2 × 2) × (5 × 5)
⇒ 400 = (2 × 2 × 5) × (2 × 2 × 5)
⇒ 400 = (2 × 2 × 5)2
⇒ √400 = 2 × 2 × 5 = 20
(iii)
1764 = 2 × 2 × 3 × 3 × 7 × 7
⇒ 1764 = (2 × 2) × (3 × 3) × (7 × 7)
⇒ 1764 = (2 × 3 × 7) × (2 × 3 × 7)
⇒ 1764 = (2 × 3 × 7)2
⇒ √1764 = 2 × 3 × 7 = 42
(iv)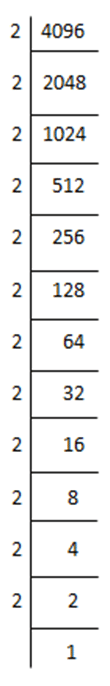
4096 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2
⇒ 4096 = (2 × 2) × (2 × 2) × (2 × 2) × (2 × 2) × (2 × 2) × (2 × 2)
⇒ 4096 = (2 × 2 × 2 × 2 × 2 × 2) × (2 × 2 × 2 × 2 × 2 × 2)
⇒ 4096 = (2 × 2 × 2 × 2 × 2 × 2)2
⇒ √4096 = 2 × 2 × 2 × 2 × 2 × 2 = 64
(v)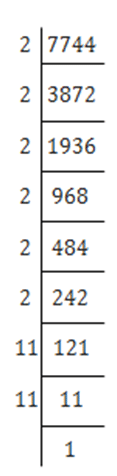
7744 = 2 × 2 × 2 × 2 × 2 × 2 × 11 × 11 × 1
⇒ 7744 = (2 × 2) × (2 × 2) × (2 × 2) × (11 × 11)
⇒ 7744 = (2 × 2 × 2 × 11) × (2 × 2 × 2 × 11)
⇒ 7744 = (2 × 2 × 2 × 11)2
⇒ √7744 = 2 × 2 × 2 × 11 = 88
(vi)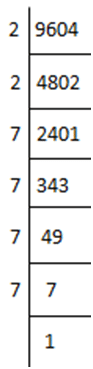
9604 = 62 × 2 × 7 × 7 × 7 × 7
⇒ 9604 = ( 2 × 2 ) × ( 7 × 7 ) × ( 7 × 7 )
⇒ 9604 = ( 2 × 7 ×7 ) × ( 2 × 7 ×7 )
⇒ 9604 = ( 2×7×7 )2
⇒ √9604 = 2×7×7 = 98
(vii)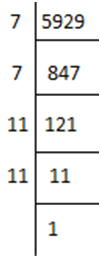
5929 = 7 × 7 × 11 × 11
⇒ 5929 = (7 × 7) × (11 × 11)
⇒ 5929 = (7 × 11) × (7 × 11)
⇒ 5929 = (7 × 11)2
⇒ √5929 = 7 × 11 = 77
(viii)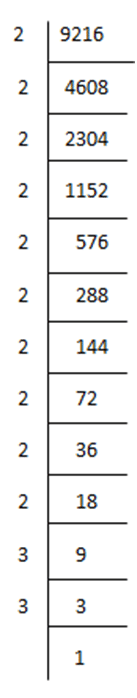
9216 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 1
⇒ 9216 = (2 × 2) × (2 × 2) × ( 2 × 2 ) × ( 2 × 2 ) × ( 2 × 2 ) × ( 3 × 3 )
⇒ 9216 = ( 2 × 2 × 2 × 2 × 2 × 3) × ( 2 × 2 × 2 × 2 × 2 × 3)
⇒ 9216 = 96 × 96
⇒ 9216 = ( 96 )2
⇒ √9216 = 96
(ix)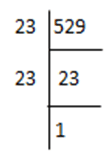
529 = 23 × 23
529 = (23)2
√529 = 23
(x)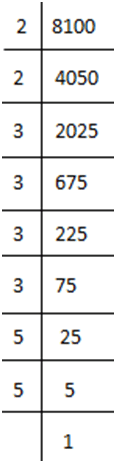
8100 = 2 × 2 × 3 × 3 × 3 × 3 × 5 × 5 × 1
⇒ 8100 = (2 × 2) × (3 × 3) × (3 × 3) × (5 × 5)
⇒ 8100 = (2 × 3 × 3 × 5) × (2 × 3 × 3 × 5)
⇒ 8100 = 90 × 90
⇒ 8100 = (90)2
⇒ √8100 = 90
Q5. For each of the following numbers, find the smallest whole number by which it should be multiplied so as to get a perfect square number. Also find the square root of the square number so obtained.
(i) 252
(ii) 180
(iii) 1008
(iv) 2028
(v) 1458
(vi) 768
Ans:
(i)
252 = 2 × 2 × 3 × 3 × 7
= (2 × 2) × (3 × 3) × 7
Here, 7 cannot be paired.
∴ We will multiply 252 by 7 to get perfect square.
New number = 252 × 7 = 1764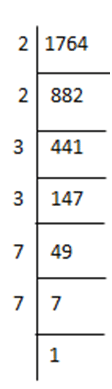
1764 = 2 × 2 × 3 × 3 × 7 × 7
⇒ 1764 = (2 × 2) × (3 × 3) × (7 × 7)
⇒ 1764 = 22 × 32 × 72
⇒ 1764 = (2 × 3 × 7)2
⇒ √1764 = 2 × 3 × 7 = 42
(ii)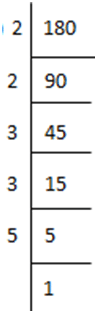
180 = 2 × 2 × 3 × 3 × 5
= (2 × 2) × (3 × 3) × 5
Here, 5 cannot be paired.
∴ We will multiply 180 by 5 to get perfect square.
New number = 180 × 5 = 900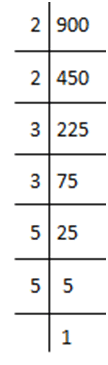
900 = 2 × 2 × 3 × 3 × 5 × 5 × 1
⇒ 900 = (2 × 2) × (3 × 3) × (5 × 5)
⇒ 900 = 22 × 32 × 52
⇒ 900 = (2 × 3 × 5)2
⇒ √900 = 2 × 3 × 5 = 30
(iii)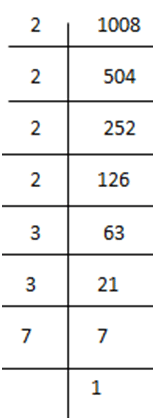
1008 = 2 × 2 × 2 × 2 × 3 × 3 × 7
= (2 × 2) × (2 × 2) × (3 × 3) × 7
Here, 7 cannot be paired.
∴ We will multiply 1008 by 7 to get perfect square.
New number = 1008 × 7 = 7056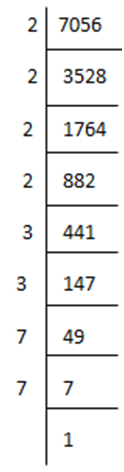
7056 = 2 × 2 × 2 × 2 × 3 ×3×7×7
⇒ 7056 = (2×2)×(2×2)×(3×3)×(7×7)
⇒ 7056 = 22×22×32×72
⇒ 7056 = (2×2×3×7)2
⇒ √7056 = 2×2×3×7 = 84
(iv)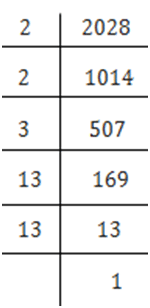
2028 = 2 × 2 × 3 × 13 × 13
= (2 × 2) × (13 × 13) × 3
Here, 3 cannot be paired.
∴ We will multiply 2028 by 3 to get perfect square. New number = 2028 × 3 = 6084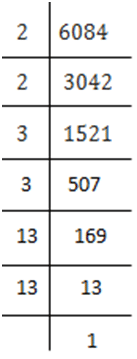
6084 = 2 × 2 × 3 × 3 × 13 × 13
⇒ 6084 = (2 × 2) × (3 × 3) × (13 × 13)
⇒ 6084 = 22 × 32 × 132
⇒ 6084 = (2 × 3 × 13)2
⇒ √6084 = 2 × 3 × 13 = 78
(v)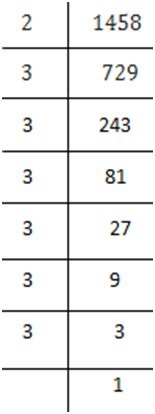
1458 = 2 × 3 × 3 × 3 × 3 × 3 × 3
= (3 × 3) × (3 × 3) × (3 × 3) × 2
Here, 2 cannot be paired.
∴ We will multiply 1458 by 2 to get perfect square. New number = 1458 × 2 = 2916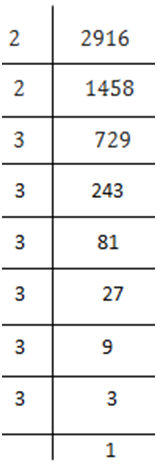
2916 = 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3
⇒ 2916 = (3 × 3) × (3 × 3) × (3 × 3) × (2 × 2)
⇒ 2916 = 32 × 32 × 32 × 22
⇒ 2916 = (3 × 3 × 3 × 2)2
⇒ √2916 = 3 × 3 × 3 × 2 = 54
(vi)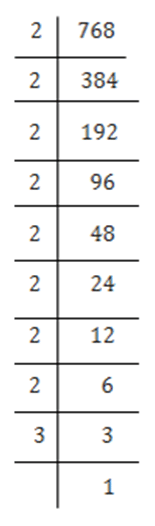
768 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3
= (2 × 2) × (2 × 2) × (2 × 2) × (2 × 2) × 3
Here, 3 cannot be paired.
∴ We will multiply 768 by 3 to get perfect square.
New number = 768 × 3 = 2304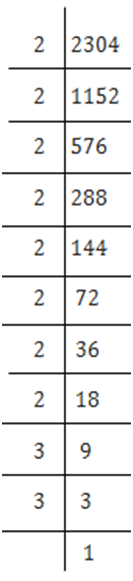
2304 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3
⇒ 2304 = (2 × 2) × (2 × 2) × (2 × 2) × (2 × 2) × (3 × 3)
⇒ 2304 = 22 × 22 × 22 × 22 × 32
⇒ 2304 = (2 × 2 × 2 × 2 × 3)2
⇒ √2304 = 2 × 2 × 2 × 2 × 3 = 48
Q6. For each of the following numbers, find the smallest whole number by which it should be divided so as to get a perfect square. Also find the square root of the square number so obtained.
(i) 252
(ii) 2925
(iii) 396
(iv) 2645
(v) 2800
(vi) 1620
Ans:
(i)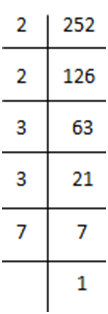
252 = 2 × 2 × 3 × 3 × 7
= (2 × 2) × (3 × 3) × 7
Here, 7 cannot be paired.
∴ We will divide 252 by 7 to get perfect square. New number = 252 ÷ 7 = 36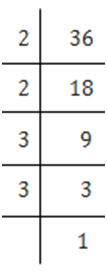
36 = 2 × 2 × 3 × 3
⇒ 36 = (2 × 2) × (3 × 3)
⇒ 36 = 22 × 32
⇒ 36 = (2 × 3)2
⇒ √36 = 2 × 3 = 6
(ii)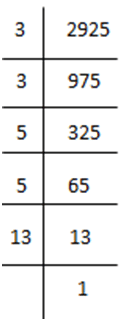
2925 = 3 × 3 × 5 × 5 × 13
= (3 × 3) × (5 × 5) × 13
Here, 13 cannot be paired.
∴ We will divide 2925 by 13 to get perfect square. New number = 2925 ÷ 13 = 225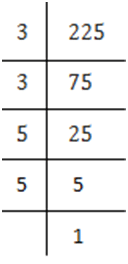
225 = 3 × 3 × 5 × 5
⇒ 225 = (3 × 3) × (5 × 5)
⇒ 225 = 32 × 52
⇒ 225 = (3 × 5)2
⇒ √36 = 3 × 5 = 15
(iii)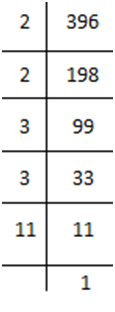
396 = 2 × 2 × 3 × 3 × 11
= (2 × 2) × (3 × 3) × 11
Here, 11 cannot be paired.
∴ We will divide 396 by 11 to get perfect square. New number = 396 ÷ 11 = 36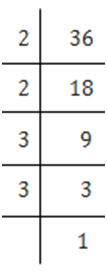
36 = 2 × 2 × 3 × 3
⇒ 36 = (2 × 2) × (3 × 3)
⇒ 36 = 22 × 32
⇒ 36 = (2 × 3)2
⇒ √36 = 2 × 3 = 6
(iv)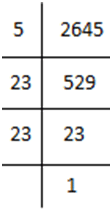
2645 = 5 × 23 × 23
⇒ 2645 = (23 × 23) × 5
Here, 5 cannot be paired.
∴ We will divide 2645 by 5 to get perfect square.
New number = 2645 ÷ 5 = 529
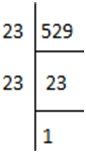
529 = 23×23
⇒ 529 = (23)2
⇒ √529 = 23
(v)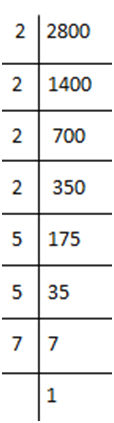
2800 = 2 × 2 × 2 × 2 × 5 × 5 × 7
= (2 × 2) × (2 × 2) × (5 × 5) × 7
Here, 7 cannot be paired.
∴ We will divide 2800 by 7 to get perfect square. New number = 2800 ÷ 7 = 400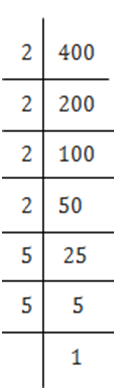
400 = 2 × 2 × 2 × 2 × 5 × 5
⇒ 400 = (2 × 2) × (2 × 2) × (5 × 5)
⇒ 400 = (2 × 2 × 5)2
⇒ √400 = 20
(vi)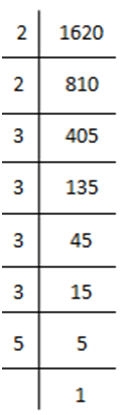
1620 = 2 × 2 × 3 × 3 × 3 × 3 × 5
= (2 × 2) × (3 × 3) ×(3 × 3) × 5
Here, 5 cannot be paired.
∴ We will divide 1620 by 5 to get perfect square. New number = 1620 ÷ 5 = 324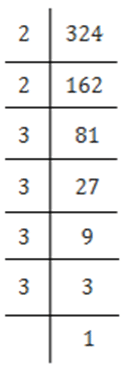
324 = 2 × 2 × 3 × 3 × 3 × 3
⇒ 324 = (2 × 2) × (3 × 3) × (3 × 3)
⇒ 324 = (2 × 3 × 3)2
⇒ √324 = 18
Q7. The students of Class VIII of a school donated ₹ 2401 in all, for Prime Minister’s National Relief Fund. Each student donated as many rupees as the number of students in the class. Find the number of students in the class.
Ans: Let the number of students in the school be, x.
∴ Each student donate Rs.x .
Total amount contributed by all the students = x × x = x2 Given, x2 = Rs.2401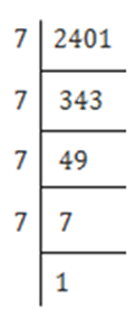
x2 = 7 × 7 × 7 × 7
⇒ x2 = (7 × 7) × (7 × 7)
⇒ x2 = 49 × 49
⇒ x = √(49 × 49)
⇒ x = 49
∴ The number of students = 49
Q8. 2025 plants are to be planted in a garden in such a way that each row contains as many plants as the number of rows. Find the number of rows and the number of plants in each row.
Ans: Let the number of rows be, x.
∴ the number of plants in each rows = x.
Total plants to be planted in the garden = x × x =x2
Given,
x2 = Rs.2025
x2 = 3×3×3×3×5×5
⇒ x2 = (3×3)×(3×3)×(5×5)
⇒ x2 = (3×3×5)×(3×3×5)
⇒ x2 = 45×45
⇒ x = √45×45
⇒ x = 45
∴ The number of rows = 45 and the number of plants in each rows = 45.
Q9. Find the smallest square number that is divisible by each of the numbers 4, 9 and 10.
Ans: We know that LCM is the smallest number divisible by all its factors.
Since, LCM of 4, 9 and 10 = 2 * 2 * 9 * 5 = 180
But 180 is not a perfect square.
Again,
180 = 2 × 2 × 3 × 3 × 5 [∵ 9 = 3 × 3]
∵ It has 5 as unpaired.
∴ [180] × 5 = [2 × 2 × 3 × 3 × 5] × 5
or 900 = 2 × 2 × 3 × 3 × 5 × 5
∵ All the prime factors of 900 are paired.
∴ 900 is a perfect square.
Thus, the required number = 900.
Q10. Find the smallest square number that is divisible by each of the numbers 8, 15 and 20.
Ans: The smallest number divisible by 8, 15 and 20 is their LCM.
We have LCM = 2 * 2 * 5 * 2 * 3 = 120
But 120 is not a square number.
Now, to make it a perfect square, we have
120 = 2 × 2 × 2 × 3 × 5
or [120] × 2 × 3 × 5 = [2 × 2 × 2 × 3 × 5] × 2 × 3 × 5
or 3600 = 2 × 2 × 2 × 2 × 3 × 3 × 5 × 5
All factors of 3600 are paired. Therefore, 3600 is a perfect squared.
∴ The required number = 3600.
Exercise 5.4
Q1. Find the square root of each of the following numbers by the Division method.
(i) 2304
(ii) 4489
(iii) 3481
(iv) 529
(v) 3249
(vi) 1369
(vii) 5776
(viii) 7921
(ix) 576
(x) 1024
(xi) 3136
(xii) 900
Ans:
(i)
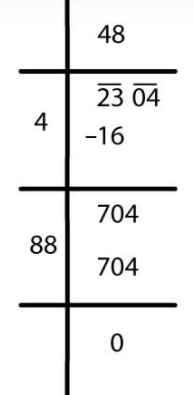
√2304 = 48
(ii)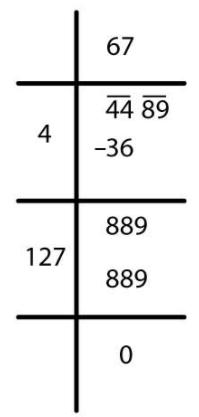
√4489 = 67
(iii)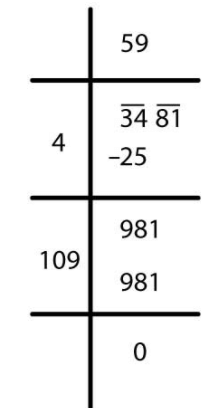
√3481 = 59
(iv)
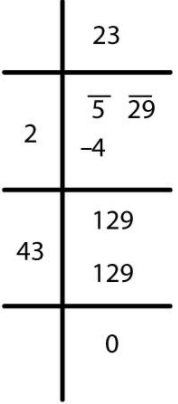
√529 = 23
(v)
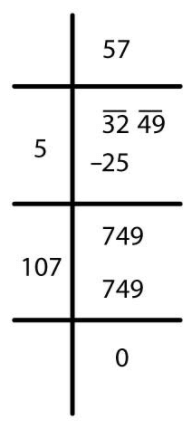
√3249 = 57
(vi)
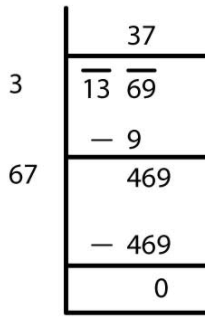
√1369 = 37
(vii)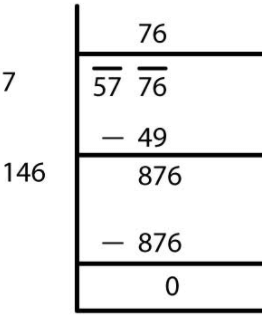
√5776 = 76
(viii)
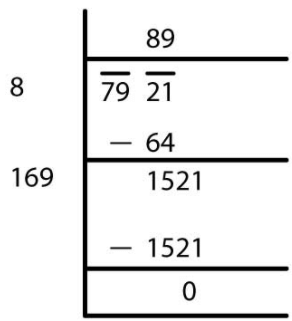
√7291 = 89
(ix)

√576 = 24
(x)

√1024 = 32
(xi)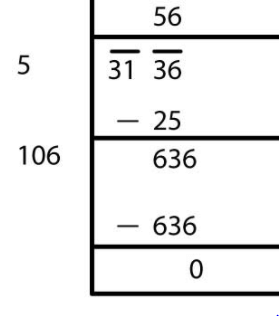
√3136 = 56
(xii)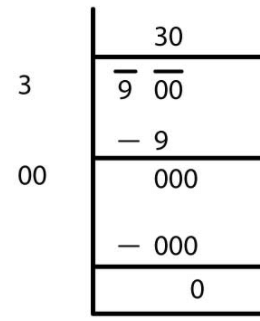
√900 = 30
Q2. Find the number of digits in the square root of each of the following numbers (without any calculation).
(i) 64
(ii) 144
(iii) 4489
(iv) 27225
(v) 390625
Ans: If ‘n’ stands for the number of digits in the given number, then
(i) For 64, n = 2 [even number]
∴ Number of digit is its square root = n/2 = 2/2 = 1
(ii) For 144, n = 3 [odd number]
∴ Number of digits in its square root = n+1/2 = 3+1/2 = 4/2 = 2
(iii) For 4489, n = 4 [even number]
∴ Number of digits in its square root = n/2 = 4/2 = 2
(iv) For 27225, n = 5 [odd number]
∴ Number of digits in its square root = n+1/2 = 5+1/2 = 6/2 = 3
(v) For 390625, n = 6 [even number]
∴ Number of digits in its square root = n/2 =6/2 =3
Q3. Find the square root of the following decimal numbers.
(i) 2.56
(ii) 7.29
(iii) 51.84
(iv) 42.25
(v) 31.36
Ans: (i) √2.56
Here, number of decimal places, are already even.
∴ We mark off the periods and find the square root.
∴ √2.56 = 1.6
(ii) √7.29
Here, number of decimal places are already even.
Therefore, we mark off the periods and find the square root.
∴ √7.29 = 2.7
(iii) √51.84
Here, the decimal places are already even.
∴ We mark off the periods and find the square root.
∴ √51.84 = 7.2
(iv) √42.25
Here, the decimal places are already even.
∴ We mark off periods and find the square root.
∴ √42.25 = 6.5
(v) √31.36
Here, the decimal places are already even.
∴ We mark off the periods and find the square root.
∴ √31.36 = 5.6
Q4. Find the least number which must be subtracted from each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.
(i) 402
(ii) 1989
(iii) 3250
(iv) 825
(v) 4000
Ans:
(i) On proceeding to find the square root of 402, we have since we get a remainder 2
∴ The required least number to be subtracted from 402 is 2.
∴ 402 – 2 = 400, and √400 = 20
(ii) Since, we get a remainder of 53
∴ The least number to be subtracted from the given number = 53
1989 – 53 = 1936, and √1936 = 44
(iii) Since, we get a remainder 1.
∴ The smallest number to be subtracted from the given number = 1
Now, 3250 – 1 = 3249, and 

(iv) Since, we get a remainder 41.
∴ The required smallest number to be subtracted from the given number = 41
Now, 825 – 41 = 784, and 

(v) Since, we get a remainder 31,
∴ The required smallest number to be subtracted from the given number = 31
Now, 4000 – 31 = 3969, and 

Q5. Find the least number which must be added to each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.
(i) 525
(ii) 1750
(iii) 252
(iv) 1825
(v) 6412
Ans:
(i) Since, we get a remainder 41.
i.e. 525 > 222.
and next square number is 23.
∴ The required number to be added = 232 – 525
= 529 – 525 = 4
Now, 525 + 4 = 529, and 

(ii) Since, we get a remainder 69.
i.e. 1750 > (41)2
and next square number is 422.
∴ The required number to be added = 422 – 1750
= 1764 – 1750 = 14
Now, 1750 + 14 = 1764, and 

(iii) Since, we get a remainder 27.
Since, 252 > (15)2 and next square number = 16
∴ The required number to be added = 162 – 252
= 256 – 252 = 4
Now, 252 + 4 = 256, and 

(iv) Since, we get a remainder, 61.
∴ 1825 > (42)2
∵ Next square number = 43
∴ The required number to be added = (43)2 – 1825
= 1849 – 1825 = 24
Now, 1825 + 24 = 1849, and 

(v) Since, we get a remainder 12.
∴ 6412 > (80)2
∵ Next square number = 81
∴ Required number to be added = (81)2 – 6412
= 6561 – 6412 = 149
Now, 6412 + 149 = 6561 and 

Q6. Find the length of the side of a square whose area is 441 m2.
Ans: Let the side of the square = x metre
∴ Area = side * side
= x * x = x2 metre2
Thus, the required side is 21 m.
Q7. In a right triangle ABC, ∠B = 90°.
(a) If AB = 6 cm, BC = 8 cm, find AC.
(b) If AC = 13 cm, BC = 5 cm, find AB.
Ans:

Remember
I. In a right triangle, the longest side is called the hypotenuse.
II. (Hypotenuse)2 = [Sum of the squares of other two sides]
(a) ∵ ∠B = 90°
∴ Hypotenuse = AC
∴ AC2 = AB2 + BC2
= 82 + 62
= 64 + 36 = 100
AC = 10
Thus, AC = 10 cm
(b) Here ∠B = 90°
∴ Hypotenuse = AC
∵ AC2 = AB2 + BC2
or 132 = AB2 + 52
or AB2 = 132 – 52
= 169 – 25 = 144
Now 
or AB = 12
Thus, AB = 12 cm

Q8. A gardener has 1000 plants. He wants to plant these so that the number of rows and the number of columns remain the same. Find the minimum number of plants he needs more for this.
Ans: Since the number of plants in a row and the number of columns are the same.
∴ Their product must be a square number.
∵ Th gardener has 1000 plants.
∴ 1000 is not a perfect square, and (31)2 < 1000
(∵ There is a remainder of 39).
Obviously the next square number = 32
∴ Number of plants required to be added = (32)2 – 1000
= 1024 – 1000 = 24
Q9. There are 500 children in a school. For a P.T. drill they have to stand in such a manner that the number of rows is equal to number of columns. How many children would be left out in this arrangement.
Ans: Since the number of rows and the number of columns are same.
∴ The total number (i.e. their product) must be a square number, we have Since, we get a remainder of 16
∴ 500 > (22)2 or 500 – 16 = (22)2
Thus, the required number of children to be left out = 16
Deleted Questions from NCERT
Finding square root by division method- For larger numbers, we use the division method for finding their square roots. This requires the pre estimation of a number of digits in the square root.
- We know that the smallest 3-digit perfect square number is 100, which is the square of 10 (2-digit number).
- The greatest 3-digit perfect number is 961, which is the square of 31 (2-digit number).
- The greatest 4-digit perfect number is 9801, which is the square of 99 (2-digit number).
- We can say that “if a perfect square is a 3-digit or 4-digit number, then its square root will have 2-digits.”
 Division Method
Division Method
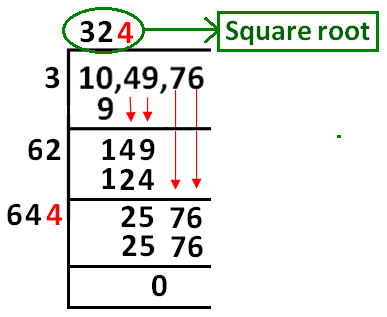
Example: Find the square root of 1369.
Solution.
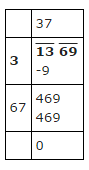
√1369 = 37
Think, Discuss, Write
Q.1. Can we say that if a perfect square is of n-digits, then its square root will have n/2 digits if n is even or (n+1)/2 if n is odd?
Solution. Yes, it is true.
Examples:
(i) 529 (a perfect number), n = 3 (odd number)
∴ Number of digits in square root = n+1/2
= 3+1/2 = 2
Also, square root of 529 = 23 (2-digits).
(ii) 1296 (is perfect square) and n = 4 (even number)
∴ Number of digits of its square root = n/2 = 4/2 =2
Now √1296 = 36 (2-digits).
Q.2. Without calculating square roots, find the number of digits in the square root of the following numbers.
(i) 25600
(ii) 100000000
(iii) 36864
Solution.
(i) 25600
∵ n = 5 [an odd number]
∴ Its square root will have (n+1)/2 digits,
i.e, 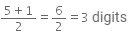
(ii) 100000000
∵ n = 9 → odd number
∴ Number of digits of its square root = n+1/2
= 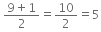
(iii) 36864
∵ n = 5 → odd number
∴ Number of digits in its square root = n+1/2
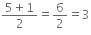
Q.3. Estimate the value of the following to the nearest whole number.
(i) √80
(ii) √1000
(iii) √350
(iv) √500
Solution.
(i) √80
∵ 102 = 100, 92 = 81, 82 = 64
and 80 is between 64 and 81.
i.e. 64 < 80 < 81
or 82 < 80 < 92
or 8 < √80 < 9
Thus, √80 lies between 8 and 9.
(ii) √1000
We know that
302 = 900, 312 = 961, 322 = 1024
∴ 1000 lies between 961 and 1024.
i.e. 916 < 1000 < 1024
or 312 < 1000 < 322
or 31 < √1000 < 32
Thus,√1000 lies between 31 and 32.
(iii) √350
We have 182 = 324, 192 = 361
Since, 350 lies between 324 and 316.
or 324 < 350 < 361
or 182 < 350 < 192
or 18 < √350 < 19
Thus, √350 lies between 18 and 19.
(iv) √500
∵ 222 = 484 and 232 = 529
Since, 500 lies between 484 and 529.
or 484 < 500 < 529
or 222 < 500 < 232
or 22 < √500 < 23
∴ √500 lies between 22 and 23.
|
79 videos|408 docs|31 tests
|
FAQs on NCERT Solutions for Class 8 Maths Chapter 5 - Squares and Square Roots (Exercise 5.3)
| 1. How to find the square root of a given number? |  |
| 2. What is the difference between a perfect square and a square root? |  |
| 3. Can a negative number have a square root? |  |
| 4. How to check if a number is a perfect square or not? |  |
| 5. Can a decimal number have a square root? |  |

|
Explore Courses for Class 8 exam
|

|