Mass Moment of Inertia | Additional Study Material for Mechanical Engineering PDF Download
Mass moment of inertia
Mass moment of inertia for a particle: The mass moment of inertia is one measure of the distribution of the mass of an object relative to a given axis. The mass moment of inertia is denoted by I and is given for a single particle of mass m as


where O-O is the axis around which one is evaluating the mass moment of inertia, and r is the perpendicular distance between the mass and the axis O-O. As can be seen from the above equation, the mass moment of inertia has the units of mass times length squared. The mass moment of inertial should not be confused with the area moment of inertia which has units of length to the power four. Mass moments of inertia naturally appear in the equations of motion, and provide information on how difficult (how much inertia there is) it is rotate the particle around given axis.
Mass moment of inertia for a rigid body: When calculating the mass moment of inertia for a rigid body, one thinks of the body as a sum of particles, each having a mass of dm. Integration is used to sum the moment of inertia of each dm to get the mass moment of inertia of body. The equation for the mass moment of inertia of the rigid body is


The integration over mass can be replaced by integration over volume, area, or length. For a fully three dimensional body using the density ρ one can relate the element of mass to the element of volume. In this case the density has units of mass per length cubed and the relation is given as
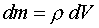
and the equation for the mass moment of inertia becomes

The integral is actually a triple integral. If the coordinate system used is rectangular then dV=dxdydz . If the coordinates uses are cylindrical coordinates then 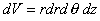 .
.
For a two dimensional body like a plate or a shell one can use density ρ per unit area (units of mass per length squared) to change the integration using the relation
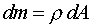
where A is the surface are and dA differential element of area. For example, for rectangular coordinates dA=dxdy and for polar coordinates 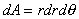 . After this substitution one gets the equation to calculate the mass moment of inertia as
. After this substitution one gets the equation to calculate the mass moment of inertia as

If the body is a rod like object then one can use the relation
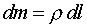
to get

where l is a coordinate along the length of the rod and the density ρ is in units of mass per unit length.
Radius of gyration: Sometime in place of the mass moment of inertia the radius of gyration k is provided. The mass moment of inertia can be calculated from k using the relation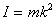
where m is the total mass of the body. One can interpret the radius of gyration as the distance from the axis that one could put a single particle of mass m equal to the mass of the rigid body and have this particle have the same mass moment of inertia as the original body.
Parallel-axis theorem: The moment of inertia around any axis can be calculated from the moment of inertia around parallel axis which passes through the center of mass. The equation to calculate this is called the parallel axis theorem and is given as
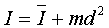

where d is the distance between the original axis and the axis passing through the center of mass, m is the total mass of the body, and  is the moment of inertia around the axis passing through the center of mass.
is the moment of inertia around the axis passing through the center of mass.
Composite bodies: If a body is composed of several bodies, to calculate the moment of inertia about a given axis one can simply calculate the moment of inertia of each part around the given axis and then add them to get the mass moment of inertia of the total body.
|
1 videos|30 docs|57 tests
|
FAQs on Mass Moment of Inertia - Additional Study Material for Mechanical Engineering
| 1. What is the mass moment of inertia in mechanical engineering? |  |
| 2. How is the mass moment of inertia calculated for simple shapes? |  |
| 3. Can objects with the same mass have different mass moments of inertia? |  |
| 4. How does the mass moment of inertia affect the rotational motion of an object? |  |
| 5. What are some practical applications of the mass moment of inertia in mechanical engineering? |  |
|
1 videos|30 docs|57 tests
|

|
Explore Courses for Mechanical Engineering exam
|

|












