NCERT Solutions for Class 10 Maths - Polynomials Exercise 2.4
Exercise 2.4
Ques 1: Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also verify the relationship between the zeroes and the coefficients in each case:
(i) 2x3 + x2 - 5x + 2; 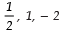
(ii) x3 - 4x2 + 5x - 2; 2, 1, 1
Sol: (i) ∵ p (x) = 2x3 + x2 - 5x + 2
∴ 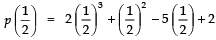
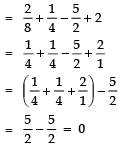
⇒ 1/2 is a zero of p(x).
Again,
p (1) = 2 (1)3 + (1)2 - 5 (1) + 2
= 2 + 1 - 5 + 2
= (2 + 2 + 1) - 5
= 5 - 5 = 0
⇒ 1 is a zero of p (x).
Also p (- 2) = 2 (- 2)3 + (- 2)2 - 5 (- 2) + 2
= 2(- 8) + (4) + 10 + 2
= - 16 + 4 + 10 + 2
= - 16 + 16 = 0
⇒ -2 is a zero of p (x).
Relationship
∵ p(x) = 2x3 + x2 - 5x + 2
∴ Comparing it with ax3 + bx2 + cx + d, we have :
a = 2, b = 1, c = - 5 and d = 2
Also 1/2 , 1 and - 2 are the zeroes of p(x)
Let α = 1/2 , β = 1 and γ = - 2
∴ α + β +γ =
Sum of the zeroes =
⇒ α + β + γ = -b/a
Sum of product of zeroes taken in pair:
Product of zeroes 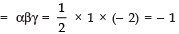
Also 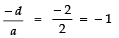
⇒ 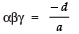
Thus, the relationship between the co-efficients and the zeroes of p (x) is verified.
(ii) Here, p(x) = x3 - 4x2 + 5x - 2
∴ p (2) = (2)3 - 4 (2)2 + 5 (2) - 2
= 8 - 16 + 10 - 2
= 18 - 18 = 0
⇒ 2 is a zero of p(x)
Again p (1) = (1)3 - 4 (1)2 + 5 (1) - 2
= 1 - 4 + 5 - 2
= 6 - 6 = 0
⇒ 1 is a zero of p(x).
∴ 2, 1, 1 are zeroes of p(x).
Now,
Comparing p (x) = x3 - 4 (x2) + 5x - 2 with ax3 + bx2 + cx + d = 0, we have
a = 1, b = - 4, c = 5 and d = - 2
∵ 2, 1, and 1 are the zeroes of p (x)
∴ Let α = 2
β = 1
γ = 1
Relationship, α + β + γ = 2 + 1 + 1 = 4
Sum of the zeroes =
⇒ α + β + γ = (-b/a)
Sum of product of zeroes taken in pair:
αβ + βγ + γα = 2 (1) + 1 (1) + 1 (2)
= 2 + 1 + 2 = 5
and = c/a = 5/1 = 5
⇒ αβ + βγ + γα = c/a
Product of zeroes = αβγ = (2) (1) (1) = 2
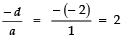
⇒ 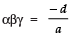
Thus, the relationship between the zeroes and the co-efficients of p(x) is verified.
Ques 2: Find the cubic polynomial with the sum, sum of the products of its zeroes taken two at a time and the product of its zeroes as 2, - 7, - 14 respectively.
Sol: Let the required cubic polynomial be ax3 + bx2 + cx + d and its zeroes be a, b and g.
∴ 

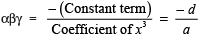
Since,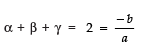
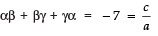
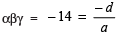
If a = 1, then -b/a = 2 ⇒ b = - 2
c/a = - 7 ⇒ c = - 7
-d/a = - 14 ⇒ d = 14
∴ The required cubic polynomial
= 1x3 + (-2) x2 + (-7) x + 14
= x3 - 2x2 - 7x + 14
Ques 3: If the zeroes of the polynomial x3 - 3x2 + x + 1 are a - b, a and a + b then find ‘a’ and ‘b’.
Sol: We have
p (x) = x3 - 3x2 + x + 1
comparing it with Ax3 + Bx2 + Cx + D.
We have
A = 1, B = - 3, C = 1 and D = 1
∵ It is given that (a - b), a and (a + b) are the zeroes of the polynomial.
∴ Let, α = (a - b)
β = a
and γ = (a + b)
∴ α + β + γ =
⇒ (a - b) + a + (a + b) = 3
⇒ 3a = 3
⇒ a = 3/3 = 1
Again, αβγ = -D/A = -1
⇒ (a - b) × a × (a + b) = - 1
⇒ (1 - b) × 1 × (1 + b) = - 1 [∵ a = 1, proved above]
⇒ 1 - b2 = - 1
⇒ b2 = 1 + 1 = 2
⇒ b = √2
Thus, a = 1 and b = .√2
Ques 4: If two zeroes of the polynomial x4 - 6x3 - 26x2 + 138x - 35 are 2 √3, find other zeroes.
Sol: Here, p(x) = x4 - 6x3 - 26x2 + 138x - 35.
∵ Two of the zeroes of p (x) are : 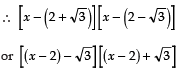
or (x - 2)2 - (√3)2
or (x2 + 4 - 4x) - 3
or x2 - 4x + 1 is a factor of p(x).
Now, dividing p (x) by x2 - 4x + 1, we have :
∴ (x2 - 4x + 1) (x2 - 2x - 35) = p (x)
⇒ (x2 - 4x + 1) (x - 7) (x + 5) = p (x)
i.e., (x - 7) and (x + 5) are other factors of p(x).
∴ 7 and - 5 are other zeroes of the given polynomial.
Ques 5: If the polynomial x4 - 6x3 + 16x2 - 25x + 10 is divided by another polynomial x2 - 2x + k, the remainder comes out to be (x + α), find k and α.
Sol: Applying the division algorithm to the polynomials x4 - 6x3 + 16x2 - 25x + 10 and
x2 - 2x + k, we have:
∴ Remainder = (2k - 9) x - k (8 - k) + 10
But the remainder = x + α
Therefore, comparing them, we have :
2k - 9 = 1
⇒ 2k = 1 + 9 = 10
⇒ k = 10/2 = 5
and
α = - k (8 - k) + 10
= - 5 (8 - 5) + 10
= - 5 (3) + 10
= - 15 + 10
= - 5
Thus, k = 5 and α = - 5
|
70 videos|242 docs|187 tests
|
FAQs on NCERT Solutions for Class 10 Maths - Polynomials Exercise 2.4
| 1. What are polynomials? |  |
| 2. How can we classify polynomials based on their degrees? |  |
| 3. What is the Remainder Theorem? |  |
| 4. How can we find the zeroes of a polynomial? |  |
| 5. What is the Fundamental Theorem of Algebra? |  |

















