NCERT Solutions for Class 10 Maths Chapter 4 - Quadratic Equations (Exercise 4.2)
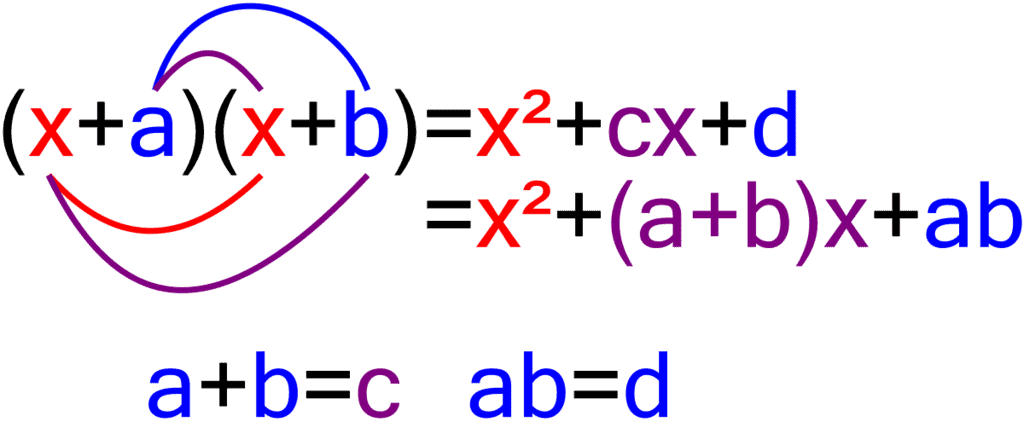 Factorisation Q1. Find the roots of the following quadratic equations by factorisation:
Factorisation Q1. Find the roots of the following quadratic equations by factorisation:
Solutions:
(i) x2 – 3x – 10 = 0
Taking LHS,
⇒x2 – 5x + 2x – 10
⇒x(x – 5) + 2(x – 5)
⇒(x – 5)(x + 2)
The roots of this equation, x2 – 3x – 10 = 0 are the values of x for which (x – 5)(x + 2) = 0
Therefore, x – 5 = 0 or x + 2 = 0
⇒ x = 5 or x = -2
(ii) 2x2 + x – 6 = 0
Taking LHS,
⇒ 2x2 + 4x – 3x – 6
=> 2x(x + 2) – 3(x + 2)
=> (x + 2)(2x – 3)
The roots of this equation, 2x2 + x – 6=0 are the values of x for which (x – 5)(x + 2) = 0
Therefore, x + 2 = 0 or 2x – 3 = 0
⇒ x = -2 or x = 3/2
(iii) √2 x2 + 7x + 5√2 = 0
Taking LHS,
⇒ √2 x2 + 5x + 2x + 5√2
⇒ x (√2x + 5) + √2(√2x + 5)= (√2x + 5)(x + √2)
The roots of this equation, √2 x2 + 7x + 5√2=0 are the values of x for which (x – 5)(x + 2) = 0
Therefore, √2x + 5 = 0 or x + √2 = 0
⇒ x = -5/√2 or x = -√2
(iv) 2x2 – x +1/8 = 0
Taking LHS,
⇒1/8 (16x2 – 8x + 1)
⇒ 1/8 (16x2 – 4x -4x + 1)
⇒1/8 (4x(4x – 1) -1(4x – 1))
⇒1/8 (4x – 1)2
The roots of this equation, 2x2 – x + 1/8 = 0, are the values of x for which (4x – 1)2= 0
Therefore, (4x – 1) = 0 or (4x – 1) = 0
⇒ x = 1/4
(v) Given, 100x2 – 20x + 1=0
Taking LHS,
⇒100x2 – 10x – 10x + 1
⇒10x(10x – 1) -1(10x – 1)
⇒(10x – 1)2
The roots of this equation, 100x2 – 20x + 1=0, are the values of x for which (10x – 1)2= 0
∴ (10x – 1) = 0 or (10x – 1) = 0
⇒x = 1/10
Q2. Solve the problems given in Example 1.
Represent the following situations mathematically:
(i) John and Jivanti together have 45 marbles. Both of them lost 5 marbles each, and the product of the number of marbles they now have is 124. We would like to find out how many marbles they had to start with.
(ii) A cottage industry produces a certain number of toys in a day. The cost of production of each toy (in rupees) was found to be 55 minus the number of toys produced in a day. On a particular day, the total cost of production was Rs 750. We would like to find out the number of toys produced on that day.
Solutions:
(i) Let us say, the number of marbles John has = x.
Therefore, the number of marble Jivanti have = 45 – x
After losing 5 marbles each,
Number of marbles John have = x – 5
Number of marble Jivanti have = 45 – x – 5 = 40 – x
Given that the product of their marbles is 124.
∴ (x – 5)(40 – x) = 124
⇒ x2 – 45x + 324 = 0
⇒ x2 – 36x – 9x + 324 = 0
⇒ x(x – 36) -9(x – 36) = 0
⇒ (x – 36)(x – 9) = 0
Thus, we can say,
x – 36 = 0 or x – 9 = 0
⇒ x = 36 or x = 9
Therefore,
If, John’s marbles = 36,
Then, Jivanti’s marbles = 45 – 36 = 9
And if John’s marbles = 9,
Then, Jivanti’s marbles = 45 – 9 = 36
(ii) Let us say, the number of toys produced in a day is x.
Therefore, cost of production of each toy = Rs(55 – x)
Given, the total cost of production of the toys = Rs 750
∴ x(55 – x) = 750
⇒ x2 – 55x + 750 = 0
⇒ x2 – 25x – 30x + 750 = 0
⇒ x(x – 25) -30(x – 25) = 0
⇒ (x – 25)(x – 30) = 0
Thus, either x -25 = 0 or x – 30 = 0
⇒ x = 25 or x = 30
Hence, the number of toys produced in a day, will be either 25 or 30.
Q3. Find two numbers whose sum is 27 and product is 182.
Solution:
Let us say, first number be x and the second number is 27 – x.
Therefore, the product of two numbers
x(27 – x) = 182
⇒ x2 – 27x – 182 = 0
⇒ x2 – 13x – 14x + 182 = 0
⇒ x(x – 13) -14(x – 13) = 0
⇒ (x – 13)(x -14) = 0
Thus, either, x = -13 = 0 or x – 14 = 0
⇒ x = 13 or x = 14
Therefore, if first number = 13, then second number = 27 – 13 = 14
And if first number = 14, then second number = 27 – 14 = 13
Hence, the numbers are 13 and 14.
Q4. Find two consecutive positive integers, sum of whose squares is 365.
Solution:
Let us say, the two consecutive positive integers be x and x + 1.
Therefore, as per the given questions,
x2 + (x + 1)2 = 365
⇒ x2 + x2 + 1 + 2x = 365
⇒ 2x2 + 2x – 364 = 0
⇒ x2 + x – 182 = 0
⇒ x2 + 14x – 13x – 182 = 0
⇒ x(x + 14) -13(x + 14) = 0
⇒ (x + 14)(x – 13) = 0
Thus, either, x + 14 = 0 or x – 13 = 0,
⇒ x = – 14 or x = 13
since, the integers are positive, x can be 13, only.
∴ x + 1 = 13 + 1 = 14
Therefore, two consecutive positive integers will be 13 and 14.
Q5. The altitude of a right triangle is 7 cm less than its base. If the hypotenuse is 13 cm, find the other two sides.
Solution:
Let us say, the base of the right triangle be x cm.
Given, the altitude of right triangle = (x – 7) cm
From Pythagoras theorem, we know,
Base2 + Altitude2 = Hypotenuse2
∴ x2 + (x – 7)2 = 132
⇒ x2 + x2 + 49 – 14x = 169
⇒ 2x2 – 14x – 120 = 0
⇒ x2 – 7x – 60 = 0
⇒ x2 – 12x + 5x – 60 = 0
⇒ x(x – 12) + 5(x – 12) = 0
⇒ (x – 12)(x + 5) = 0
Thus, either x – 12 = 0 or x + 5 = 0,
⇒ x = 12 or x = – 5
Since sides cannot be negative, x can only be 12.
Hence, the base of the given triangle is 12 cm and the altitude of this triangle will be (12 – 7) cm = 5 cm.
Q6. A cottage industry produces a certain number of pottery articles in a day. It was observed on a particular day that the cost of production of each article (in rupees) was 3 more than twice the number of articles produced on that day. If the total cost of production on that day was Rs.90, find the number of articles produced and the cost of each article.
Solution:
Let us say, the number of articles produced is x.
Therefore, cost of production of each article = Rs (2x + 3)
Given, the total cost of production is Rs.90
∴ x(2x + 3) = 90
⇒ 2x2 + 3x – 90 = 0
⇒ 2x2 + 15x -12x – 90 = 0
⇒ x(2x + 15) -6(2x + 15) = 0
⇒ (2x + 15)(x – 6) = 0
Thus, either 2x + 15 = 0 or x – 6 = 0
⇒ x = -15/2 or x = 6
As the number of articles produced can only be a positive integer, therefore, x can only be 6.
Hence, the number of articles produced = 6
Cost of each article = 2 × 6 + 3 = Rs 15.
|
70 videos|242 docs|187 tests
|

















