Class 9 Maths Chapter 2 HOTS Questions - Polynomials

Q.1. If  p(x) = x² – 2√2 x + 1, then find  p (2√2) .

Sol. Since, p(x) = x² – 2 √2 x + 1 , replacing value of x=2√2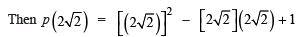

= 4 (2) – 4 (2) + 1

= 8 – 8 + 1 

= 1

Q.2. Factorize 3x²-14x - 5$\mathrm{ }2x^2\; +\; 7x\; +\; 3$using the middle term splitting method.

Sol. $a=3,b=-14,c=-\mathrm{5, }$We need two numbers that multiply to $ac=3\times (-5)=-15ac\; =\; 3\; \backslash times\; (-5)\; =\; -15$ and add up to $b=-14.=\; -14$

The numbers are -15 and 1 because $-15\times 1=-15-15\; \backslash times\; 1\; =\; -15$ and $-15+1=-14.-15\; +\; 1\; =\; -14$

Rewrite the middle term: $3x\mathrm{<sup\; style="word-break:\; break-word;\; line-height:\; 1.8;\; font-family:\; Lato,\; sans-serif;\; font-size:\; 20px;\; letter-spacing:\; inherit;\; color:\; black;">2</sup>}-15x+x-5$

$-\; 15x\; +\; x\; -\; 5$Factor by grouping: $(3x\mathrm{<sup\; style="word-break:\; break-word;\; line-height:\; 1.8;\; font-family:\; Lato,\; sans-serif;\; font-size:\; 20px;\; letter-spacing:\; inherit;\; color:\; black;">2</sup>}-15x)+(x-5)(3x^2\; -\; 15x)\; +\; (x\; -\; 5)$$\mathrm{ }$  ⇒ $3x(x-5)+1(x-5)3x(x\; -\; 5)\; +\; 1(x\; -\; 5)$  ⇒ $(3x+1)(x-5)(3x\; +\; 1)(x\; -\; 5)$(3x+1)(x−5)

So, the factorized form of $3x\mathrm{<sup\; style="word-break:\; break-word;\; line-height:\; 1.8;\; font-family:\; Lato,\; sans-serif;\; font-size:\; 20px;\; letter-spacing:\; inherit;\; color:\; black;">2</sup>}-14x-53x^2\; -\; 14x\; -\; 5$  is $(3x+1)(x-5)(3x\; +\; 1)(x\; -\; 5)$.

Q.3. If  a + b + c = 9, and ab + bc + ca = 26, find a² + b² + c²

Sol.nGiven: a + b + c = 9, ab + bc + ca = 26

Squaring, we get

(a+b+c)²=(9)²

a²+b²+c²+2(ab+bc+ca)=81

⇒ a²+b²+c²+2×26=81

⇒ a²+b²+c²=81−52  =29

Q.4. Factorise 4x² – 12x + 9 

Sol: To factorize this expression, we need to find two numbers α and β such that α + β = –12 and αβ = 36

4x² – 6x – 6x + 9

2x(x – 3) – 3(x – 3)

(2x – 3)(x – 3)

Q.5. If a, b, c are all non-zero and a + b + c = 0, prove that 

Sol. ∵ a + b + c = 0  ⇒  a³ + b³ + c³ – 3abc = 0
or a³ + b³ + c³ = 3abc 

dividing the whole equation by abc, we get 

⇒

Q.6. If then find the value of

Sol: 

Q.7. Factorize 12x²-29x +15$\mathrm{ }$using the middle term splitting method.

Sol. $a=12,b=-29,c=+15,$We need two numbers that multiply to $ac=12\times (15)=\; 180$ and add up to $b=-29.$

The numbers are -20 and -9 because $-20\times -9=180-20\; \backslash times\; -9\; =\; 180$ and $-20+-9=-29-20\; +\; -9\; =\; -29$.

Rewrite the middle term: 12x²−20x−9x+15

Factor by grouping: (12x²−20x)+(−9x+15) $\mathrm{\Rightarrow \; 4}x(3x-5)-3(3x-5)4x(3x\; -\; 5)\; -\; 3(3x\; -\; 5)$ ⇒ (4x−3)(3x−5)
So, the factorized form of 12x²−29x+15 is $(4x-3)(3x-5)(4x\; -\; 3)(3x\; -\; 5)$.

Q.8. Factorise: (a – b)³ + (b – c)³ + (c – a)³

Q.10.  Factorise 10y² – 28y + 14

Sol: Consider the equation 3x + 2y = 12
Now, square both sides:
(3x + 2y)² = 12²
=> 9x² + 12xy + 4y² = 144
=>9x² + 4y² = 144 – 12xy
From the questions, xy = 6
So,
9x² + 4y² = 144 – 72
Thus, the value of 9x² + 4y² = 72

Q.12.Give possible expressions for the length and breadth of each of the following rectangles, in which their areas are given: 

(i) Area: 25a²–35a+12

(ii) Area: 35y²+13y–12

Sol:(i) Area: 25a²–35a+12

Using the splitting the middle term method,

We have to find a number whose sum = -35 and product =25×12 = 300

We get -15 and -20 as the numbers [-15+-20=-35 and -15×-20 = 300]

25a²–35a+12 = 25a²–15a−20a+12

= 5a(5a–3)–4(5a–3)

= (5a–4)(5a–3)

Possible expression for length  = 5a–4

Possible expression for breadth  = 5a –3

(ii) Area: 35y²+13y–12

Using the splitting the middle term method,

We have to find a number whose sum = 13 and product = 35×-12 = 420

We get -15 and 28 as the numbers [-15+28 = 13 and -15×28=420]

35y²+13y–12 = 35y²–15y+28y–12

= 5y(7y–3)+4(7y–3)

= (5y+4)(7y–3)

Possible expression for length  = (5y+4)

Possible expression for breadth  = (7y–3)