Class 10 Maths Chapter 6 Question Answers - Triangles
Very Short Answer Type Questions
Q1.In the given figure, DE || BC such that AC = 9 cm, AB = 7.2 cm and AD = 1.8 cm. Find AE.
Sol. In Δ ABC DE || BC
∴ Using the Basic Proportionality Theorem, we have:
Thus, the required value of AE = 2.25 cm.
Q2. In the figure, DE || BC such that AD = 4.8 cm, AE = 6.4 cm and EC = 9.6 cm, find DB.
Sol. Since DE || BC
∴In Δ ABC, we have
=
[using the Basic Prop. Theorem]
⇒ DB = = 7.2 cm
Thus, the required value of DB = 7.2 cm.
Q3. In the given figure, DE || BC such that
AD = (7x - 4) cm, AE = (5x - 2) cm. If EC = 3x and DB = (3x + 4) cm, then find the value of x.
Sol. Since, in Δ ABC, DE || BC.
∴ Using Basic Proportionality Theorem, we have
=
⇒ 3x (7x - 4) = (5x - 2) (3x + 4)
⇒ 21x2 - 12x = 15x2 + 20x - 6x - 8
⇒ 21x2 - 15x2 - 12x - 20x + 6x = - 8
⇒ 6x2 - 26x + 8 = 0
⇒ 3x2 - 13x + 4 = 0
⇒ 3x2 - 12x - x + 4 = 0
⇒ 3x (x - 4) - 1 (x - 4) = 0
⇒ (3x - 1) (x - 4) = 0
⇒ x = or 4.
Q4. In the given figure, DE || BC such that . If AB = 4.8 cm then find AD.
Sol. Since DE || BC
∴ Using Basic Proportionality Theorem in Δ ABC, we have
=
Q5.In the given figure, if DE || BC and, then find AC such that AE = 2.1 cm.
Sol. DE || BC
∴In Δ ABC, using the Basic Proportionality Theorem, we have:
Now, AC = AE + EC = (2.1 + 3.5) cm
= 5.6 cm.
Q6. In the figure, Δ ABC ~ Δ DEF and AB/DE=3. If BC = 4 cm then find EF.
Sol. Δ ABC ~ Δ DEF .
Q7. The areas of two similar triangles ABC and DEF are 81 cm2 and 100 cm2 respectively.
If EF = 5 cm, then find BC.
Sol. Δ ABC ~ Δ DEF
∴
Q8. The area of Δ PQR = 64 cm2. Find the area of Δ LMN, if and Δ PQR ~ Δ LMN.
Sol. Δ PQR ~ Δ LMN
Q9. In the figure, <B = 90. Find AC.
Sol. < B = 90°
∴Δ ABC is a right angled triangle.
∴Using Pythagoras theorem, we have:
AC2 = AB2 + BC2
= (4.5)2 + 42
= 20.25 + 16
= 36.25
⇒ AC = = 6.02 cm (approx.)
Q10. In the figure, if <A = 30° then find the measure of <C.
Sol. In Δ ABC, we have:
52 = 32 + 42
∴Using the converse of Pythagoras Theorem, we have
Δ ABC is right-angled at B; i.e., <B = 90°
Now using the angle-sum-property of a triangle, we get
90° + 30° + <C = 180°
⇒ <C = 180° - 90° - 30° = 60°.
Q11. In the figure, ST || QR, PS = 2 cm and QS = 3 cm. What is the ratio of the area of Δ PQR to the area of Δ PST.
Sol. In Δ PQR and Δ PST
<P = <P [Common]
And <PQR = <PST [ΠST y QR]
∴ ΔPQR ~ Δ PST
[By AA similarity rule]
Q12. In the figure, DE || AB, find the length of AC.
Sol. In Δ ABC, we have DE || AB
∴Using the Basic Proportionality Theorem, we have:
=
Now AC = AD + DC
= 4.5 + 3 = 7.5 cm
Thus, AC = 7.5 cm.
Q13. In < LMN, <L = 50° and <N = 60°. If Δ LMN ~ Δ PQR, then find <Q.
Sol. By the angle-sum property,
<L + <M + <N = 180°
⇒ 50° + <M + 60° = 180°
⇒ <M = 180° - 60° - 50°
= 70°.
Since, Δ LMN ~ Δ PQR
∴<L = <P
<M = <Q
<N = <R
Now, <Q = <M = 70°
⇒ <Q = 70°.
Q14. In the figure, <M = <N = 46°. Express x in terms of a, b and c where a, b, and c are lengths of LM, MN and NK respectively.
Sol. In Δ KML and Δ KNP
<M = <N = 46° [Given]
<MKL = <NKP [Common]
⇒ Δ KML ~ Δ KNP
[AA similarity rule]
∴Their corresponding sides are proportional.
⇒
Q15. If the areas of two similar triangles are in the ratio 25 : 64, write the ratio of their corresponding sides.
Sol. Here, Δ ABC ~ Δ DEF
Since the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides,
∴
Q16. In a Δ ABC, DE || BC. If DE = BC and area of Δ ABC = 81 cm2, find the area of Δ ADE.
Sol. Since DE || BC
∴<1 = <2 [Corresponding angle]
Also <3 = <4 [Corresponding angles]
⇒ Δ DAE ~ Δ BAC [By AA criterion]
∴
Thus, area of Δ ADE = 36 cm2.
Q17. In the figure, P and Q are points on the sides AB and AC respectively of Δ ABC such that AP = 3.5 cm, PB = 7 cm, AQ = 3 cm and
QC = 6 cm. If PQ = 4.5 cm, find BC.
Sol. We have:
In Δ AQP and Δ ACD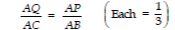
⇒ Δ AQP ~ Δ ACB
[SAS similarity rule] [In similar Δ s, the ratio of corresponding sides are equal]
⇒ BC = 4.5 × 3 = 13.5 cm.
Q18. In the figure, PQ = 24 cm, QR = 26 cm, <PQR = 90°, PA = 6 cm and AR = 8 cm. Find <QPR.
Sol. In right Δ PAR,
By the Pythagoras theorem,
PR2 = PA2 + AR2
= 62 + 82 = 36 + 64 = 100
But length cannot be negative
∴ PR = 10 cm
Now,
PR2 + PQ2 = 102 + 242
= 100 + 576 = 676
= 262 = QR2
⇒ PR2 + PQ2 = QR2
⇒ <QPR = 90°
Q19. D, E and F are the mid-points of the sides BC, AC and AB respectively of Δ ABC. Find .
Sol. E and F are the midpoints of CA
and AB [Given]
∴ By midpoint theorem, ...
Similarly, ...(2)
...(3)
From (1), (2) and (3), we get
=
Q20. In the figure, PQ || BC and AP : BP = 1 : 2. Find
Sol. It is given that
AP : PB = 1 : 2
Let AP = k ⇒ PB = 2k
∴ AB = AP + PB = k + 2k = 3k
PQ || BC
∴ <APQ = <ABC and
<AQP = <ACB [Corresponding Angles]
Q21. In the figure, DE || BC and if AC = 4.8 cm, find the length of AE.
Sol. Let AE = x
∴EC = AC - AE
= (4.8 - x) cm
DE || BC [Given]
∴
[By the Basic Proportionality Theorem]
⇒
Q22. In Δ ABC (shown in the figure), DE || BC. If BC = 8 cm, DE = 6 cm and area of Δ ADE = 45 cm2, then what is the area of Δ ABC?
Sol. DE || BC
∴In Δ ADE and Δ ABC,
<D = <B and <E = <C
[Corresponding angles]
∴ Δ ADE ~ Δ ABC
Q23. In the figure, DE || BC and AD = 1 cm, BD = 2 cm. What is the ratio of the areas of Δ ABC to the area of Δ ADE?
Sol. In Δ ABC and Δ ADE
<A = <A [Common]
<B = <ADE
[Corresponding angles]
∴Using AA similarity, we have:
Δ ADE ~ Δ ABC
⇒
⇒ The required ratio = 9: 1.
Q24. In the figure, AC || BD. Is ?
Sol. In Δ ACE and Δ DBE,
<A = <D
[Alternate Interior Angles]
<C = <B
∴Using AA similarity
Δ ACE ~ Δ DBE
∴Their corresponding sides are proportional.
⇒
|
127 videos|584 docs|79 tests
|
FAQs on Class 10 Maths Chapter 6 Question Answers - Triangles
| 1. What are the properties of an equilateral triangle? |  |
| 2. How do you find the area of a triangle? |  |
| 3. What is the Pythagorean Theorem? |  |
| 4. How do you determine if three given sides can form a triangle? |  |
| 5. What is the difference between an acute triangle and an obtuse triangle? |  |
















