RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths | RD Sharma Solutions for Class 9 Mathematics PDF Download
Q1. x3+6x2+11x+6
Sol:
Given polynomial, f(x) = x3+6x2+11x+6
The constant term in f(x) is 6
The factors of 6 are ±1, ±2, ±3, ±6
Let, x + 1 = 0
⇒ x = -1
Substitute the value of x in f(x)
f(-1) = (−1)3+6(−1)2+11(−1)+6
= -1 + 6 -11 + 6
= 12 – 12
= 0
So, (x + 1) is the factor of f(x)
Similarly, (x + 2) and (x + 3) are also the factors of f(x)
Since, f(x) is a polynomial having a degree 3, it cannot have more than three linear factors.
∴ f(x) = k(x + 1)(x + 2)(x + 3)
⇒ x3+6x2+11x+6 = k(x + 1)(x + 2)(x + 3)
Substitute x = 0 on both the sides
⇒ 0 + 0 + 0 + 6 = k(0 +1)(0 + 2)(0 + 3)
⇒ 6 = k(1*2*3)
⇒ 6 = 6k
⇒ k = 1
Substitute k value in f(x) = k(x + 1)(x + 2)(x + 3)
⇒ f(x) = (1)(x + 1)(x + 2)(x + 3)
⇒ f(x) = (x + 1)(x + 2)(x + 3)
∴ x3+6x2+11x+6 = (x + 1)(x + 2)(x + 3)
Q2. x3+2x2–x–2
Sol:
Given, f(x) = x3+2x2–x–2
The constant term in f(x) is -2
The factors of (-2) are ±1, ±2
Let , x – 1 = 0
⇒ x = 1
Substitute the value of x in f(x)
f(1) = (1)3+2(1)2–1–2
= 1 + 2 – 1 – 2
= 0
Similarly , the other factors (x + 1) and (x + 2) of f(x)
Since, f(x) is a polynomial having a degree 3, it cannot have more than three linear factors.
∴ f(x) = k(x – 1)(x + 2)(x + 1)
x3+2x2–x–2 = k(x – 1)(x + 2)(x + 1)
Substitute x = 0 on both the sides
0 + 0 – 0 – 2 = k(-1)(1)(2)
⇒ – 2 = -2k
⇒ k = 1
Substitute k value in f(x) = k(x – 1)(x + 2)(x + 1)
f(x) = (1)(x – 1)(x + 2)(x + 1)
⇒ f(x) = (x – 1)(x + 2)(x + 1)
So, x3+2x2–x–2 = (x – 1)(x + 2)(x + 1)
Q3. x3–6x2+3x+10
Sol:
Let, f(x) = x3–6x2+3x+10
The constant term in f(x) is 10
The factors of 10 are ±1, ±2, ±5, ±10
Let , x + 1 = 0
⇒ x = -1
Substitute the value of x in f(x)
f(-1) = (−1)3–6(−1)2+3(−1)+10
= -1 – 6 – 3 + 10
= 0
Similarly , the other factors (x – 2) and (x – 5) of f(x)
Since, f(x) is a polynomial having a degree 3, it cannot have more than three linear factors.
∴ f(x) = k(x + 1)(x – 2)(x – 5 )
Substitute x = 0 on both sides
⇒ x3–6x2+3x+10 = k(x + 1)(x – 2)(x – 5)
⇒ 0 – 0 + 0 + 10 = k(1)(-2)(-5)
⇒ 10 = k(10)
⇒ k = 1
Substitute k = 1 in f(x) = k(x + 1)(x – 2)(x – 5)
f(x) = (1)(x + 1)(x – 2)(x – 5)
so, x3–6x2+3x+10 = (x + 1)(x – 2)(x – 5)
Q4. x4–7x3+9x2+7x–10
Sol:
Given, f(x) = x4–7x3+9x2+7x–10
The constant term in f(x) is 10
The factors of 10 are ±1, ±2, ±5, ±10
Let , x – 1 = 0
⇒ x = 1
Substitute the value of x in f(x)
f(x) = 14–7(1)3+9(1)2+7(1)–10
= 1 – 7 + 9 + 7 – 10
= 10 – 10
= 0
(x – 1) is the factor of f(x)
Simarly, the other factors are (x + 1) ,(x – 2) , (x – 5)
Since, f(x) is a polynomial of degree 4. So, it cannot have more than four linear factor.
So, f(x) = k(x – 1)(x + 1)(x – 2)(x – 5)
⇒ x4–7x3+9x2+7x–10 = k(x – 1)(x + 1)(x – 2)(x – 5)
Put x = 0 on both sides
0 – 0 + 0 – 10 = k(-1)(1)(-2)(-5)
– 10 = k(-10)
⇒ k = 1
Substitute k = 1 in f(x) = k(x – 1)(x + 1)(x – 2)(x – 5)
f(x) = (1)(x – 1)(x + 1)(x – 2)(x – 5)
= (x – 1)(x + 1)(x – 2)(x – 5)
So, x4–7x3+9x2+7x–10 = (x – 1)(x + 1)(x – 2)(x – 5)
Q5. x4–2x3–7x2+8x+12
Sol:
Given , f(x) = x4–2x3–7x2+8x+12
The constant term f(x) is equal is 12
Tha factors of 12 are ±1, ±2, ±3, ±4, ±6, ±12
Let, x + 1 = 0
⇒ x = -1
Substitute the value of x in f(x)
f(-1) = (−1)4–2(−1)3–7(−1)2+8(−1)+12
= 1 + 2 – 7 – 8 + 12
= 0
So, x + 1 is factor of f(x)
Similarly, (x + 2), (x – 2), (x – 3) are also the factors of f(x)
Since, f(x) is a polynomial of degree 4 , it cannot have more than four linear factors.
⇒ f(x) = k(x + 1)(x + 2)(x – 3)(x – 2)
⇒ x4–2x3–7x2+8x+12 = k(x + 1)(x + 2)(x – 3)(x – 2)
Substitute x = 0 on both sides,
⇒ 0 – 0 – 0 + 12 = k(1)(2)(-2)(-3)
⇒ 12 = k12
⇒ k = 1
Substitute k = 1 in f(x) = k(x – 2)(x + 1)(x + 2)(x – 3)
f(x) = (x – 2)(x + 1)(x + 2)(x – 3)
so, x4–2x3–7x2+8x+12 = (x – 2)(x + 1)(x + 2)(x – 3)
Q6. x4+10x3+35x2+50x+24
Sol:
Given, f(x) = x4+10x3+35x2+50x+24
The constant term in f(x) is equal to 24
The factors of 24 are ±1, ±2, ±3, ±4, ±6, ±8, ±12, ±24
Let, x + 1 = 0
⇒ x = -1
Substitute the value of x in f(x)
f(-1) = (−1)4+10(−1)3+35(−1)2+50(−1)+24
= 1 – 10 + 35 – 50 + 24
= 0
⇒ (x + 1) is the factor of f(x)
Similarly, (x + 2),(x + 3),(x + 4) are also the factors of f(x)
Since, f(x) is a polynomial of degree 4, it cannot have more than four linear factors.
⇒ f(x) = k(x + 1)(x + 2)(x + 3)(x + 4)
⇒ x4+10x3+35x2+50x+24 = k(x + 1)(x + 2)(x + 3)(x + 4)
Substitute x = 0 on both sides
⇒ 0 + 0 + 0 + 0 + 24 = k(1)(2)(3)(4)
⇒ 24 = k(24)
⇒ k = 1
Substitute k = 1 in f(x) = k(x + 1)(x + 2)(x + 3)(x + 4)
f(x) = (1)(x + 1)(x + 2)(x + 3)(x + 4)
f(x) = (x + 1)(x + 2)(x + 3)(x + 4)
hence, x4+10x3+35x2+50x+24 = (x + 1)(x + 2)(x + 3)(x + 4)
Q7. 2x4–7x3–13x2+63x–45
Sol :
Given, f(x) = 2x4–7x3–13x2+63x–45
The factors of constant term -45 are ±1, ±3, ±5, ±9, ±15, ±45
The factors of the coefficient of x4 is 2. Hence possible rational roots of f(x) are
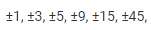
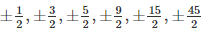
Let, x – 1 = 0
⇒ x= 1
f(1) = 2(1)4–7(1)3–13(1)2+63(1)–45
= 2 – 7 – 13 + 63 – 45
= 0
Let, x – 3 = 0
⇒ x = 3
f(3) = 2(3)4–7(3)3–13(3)2+63(3)–45
= 162 – 189 – 117 + 189 – 45
= 0
So, (x – 1) and (x – 3) are the roots of f(x)
⇒ x2 – 4x + 3 is the factor of f(x)
Divide f(x) with x2 – 4x + 3 to get other three factors
By long division,
2x2 + x – 15
x2 – 4x + 3 2x4 – 7x3 – 13x2 + 63x – 45
2x4 – 8x3 + 6x2
(-) (+) (-)
x3 – 19x2 + 63x
x3 – 4x2 + 3x
(-) (+) (-)
– 15x2 + 60x – 45
(+) (-) (+)
0
⇒ 2x4–7x3–13x2+63x–45 = (x2 – 4x + 3)(2x2 + x – 15)
⇒ 2x4–7x3–13x2+63x–45 = (x – 1) (x – 3)(2x2 + x – 15)
Now,
2x2 + x – 15 = 2x2+ 6x – 5x – 15
= 2x(x + 3) – 5 (x + 3)
= (2x – 5) (x + 3)
So, 2x4–7x3–13x2+63x–45 = (x – 1)(x – 3)(x + 3)(2x – 5)
Q8. 3x3−x2–3x+1
Sol :
Given , f(x) = 3x3−x2–3x+1
The factors of constant term 1 is ±1
The factors of the coefficient of x2 = 3
The possible rational roots are ±1 ,1/3
Let, x – 1 = 0
⇒ x = 1
f(1) = 3(1)3−(1)2–3(1)+1
= 3 – 1 – 3 + 1
= 0
So, x – 1 is tha factor of f(x)
Now, divide f(x) with (x – 1) to get other factors
By long division method,
3x2 + 2x – 1
x – 1 3x3 – x2 – 3x + 1
3x3 – x2
(-) (+)
2x2 – 3x
2x2 – 2x
(-) (+)
-x + 1
-x + 1
(+) (-)
0
⇒ 3x3−x2–3x+1 = (x – 1)( 3x2 + 2x – 1)
Now,
3x2 + 2x – 1 = 3x2 + 3x – x – 1
= 3x(x + 1) -1(x + 1)
= (3x – 1)(x + 1)
Hence , 3x3−x2–3x+1 = (x – 1) (3x – 1)(x + 1)
Q9. x3−23x2+142x–120
Sol :
Let, f(x) = x3−23x2+142x–120
The constant term in f(x) is -120
The factors of -120 are ±1, ±2, ±3, ±4, ±5, ±6, ±8, ±10, ±12, ±15, ±20, ±24, ±30, ±40, ±60, ±120
Let, x – 1 = 0
⇒ x = 1
f(1) = (1)3−23(1)2+142(1)–120
= 1 – 23 + 142 – 120
= 0
So, (x – 1) is the factor of f(x)
Now, divide f(x) with (x – 1) to get other factors
By long division,
x2 – 22x + 120
x – 1 x3 – 23x2 + 142x – 120
x3 – x2
(-) (+)
-22x2 + 142x
-22x2 + 22x
(+) (-)
120x – 120
120x – 120
(-) (+)
0
⇒ x3 – 23x2 + 142x – 120 = (x – 1) (x2 – 22x + 120)
Now,
x2 – 22x + 120 = x2 – 10x – 12x + 120
= x( x – 10) – 12( x – 10)
= (x – 10) (x – 12)
Hence, x3 – 23x2 + 142x – 120 = (x – 1) (x – 10) (x – 12
FAQs on RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths - RD Sharma Solutions for Class 9 Mathematics
| 1. What is the importance of factorizing polynomials? |  |
| 2. How can we determine if a polynomial is factorizable? | |
| 3. What is the process of factorizing a polynomial? | |
| 4. Can all polynomials be factorized? | |
| 5. How does factorization help in solving polynomial equations? | |
|
4.66/5 Rating |
|
Oct 31, 2024 Last updated |
|
Explore Courses for Class 9 exam
|
|
shortcuts and tricks
,MCQs
,(Part -1)
,RD Sharma Solutions Ex-6.5
,Semester Notes
,(Part -1)
,Summary
,Sample Paper
,RD Sharma Solutions Ex-6.5
,(Part -1)
,Maths | RD Sharma Solutions for Class 9 Mathematics
,video lectures
,Free
,Objective type Questions
,study material
,Extra Questions
,mock tests for examination
,Exam
,Factorization Of Polynomials
,Class 9
,practice quizzes
,ppt
,Class 9
,Important questions
,past year papers
,Maths | RD Sharma Solutions for Class 9 Mathematics
,RD Sharma Solutions Ex-6.5
,Viva Questions
,Previous Year Questions with Solutions
,Class 9
,Factorization Of Polynomials
,Factorization Of Polynomials
,Maths | RD Sharma Solutions for Class 9 Mathematics
;
RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths Free PDF Download
Importance of RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths
RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths Notes
RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths Class 9 Questions
Study RD Sharma Solutions Ex-6.5, (Part -1), Factorization Of Polynomials, Class 9, Maths on the App
|
© EduRev
|
Education Revolution
|
Follow Us
|
