Ex-23.3, Data Handling II Central Values, Class 7, Math RD Sharma Solutions | RD Sharma Solutions for Class 7 Mathematics PDF Download
Question 1:
Find the median of the following data (1-8)
83, 37, 70, 29, 45, 63, 41, 70, 34, 54
Answer 1:
Arranging the data in ascending order, we have:
29, 34, 37, 41, 45, 54, 63, 70, 70, 83
Here, the number of observations, n = 10 (Even).


Hence, the median of the given data is 49.5.
Question 2:
Find the median of the following data (1-8)
133, 73, 89, 108, 94, 104, 94, 85, 100, 120
Answer 2:
Arranging the data in ascending order, we have:
73, 85, 89, 94, 94, 100, 104, 108, 120, 133.
Here, the number of observations n = 10 (Even).

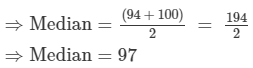
Hence, the median of the given data is 97.
Question 3:
Find the median of the following data (1-8)
31, 38, 27, 28, 36, 25, 35, 40
Answer 3:
Arranging the data in ascending order, we have:
25,27, 28, 31, 35, 36, 38, 40
Here, the number of observations n = 8 (Even).

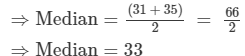
Hence, the median of the given data is 33.
Question 4:
Find the median of the following data (1-8)
15, 6, 16, 8, 22, 21, 9, 18, 25
Answer 4:
Arranging the data in ascending order, we have:
6, 8, 9, 15,16,18, 21, 22, 25
Here, the number of observations n = 9 (Odd).
⇒Median=Value of 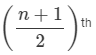 observati5on i.e , value of the 5th observation = 16
observati5on i.e , value of the 5th observation = 16
Hence, the median of the given data is 16
Question 5:
Find the median of the following data (1-8)
41, 43, 127, 99, 71, 92, 71, 58, 57
Answer 5:
Arranging the given data in ascending order, we have:
41, 43, 57, 58, 71,71, 92, 99, 127
Here, n = 9, which is odd.
∴ Median = Value of 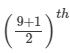 observation, i.e., the 5th observation = 71.
observation, i.e., the 5th observation = 71.
Question 6:
Find the median of the following data (1-8)
25, 34, 31, 23, 22, 26, 35, 29, 20, 32
Answer 6:
Arranging the given data in ascending order, we have:
20, 22, 23, 25, 26, 29, 31, 32, 34, 35
Here, n = 10, which is even.

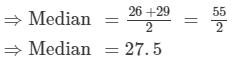
Hence, the median is 27.5 for the given data.
Question 7:
Find the median of the following data (1-8)
12, 17, 3, 14, 5, 8, 7, 15
Answer 7:
Arranging the given data in ascending order, we have:
3,5,7,8,12,14,15,17
Here, n = 8, which is even.

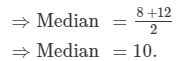
Hence, the median of the given data is 10.
Question 8:
Find the median of the following data (1-8)
92, 35, 67, 85, 72, 81, 56, 51, 42, 69
Answer 8:
Arranging the given data in ascending order, we have:
35, 42, 51, 56, 67, 69, 72, 81, 85, 92
Here, n = 10, which is even.

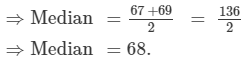
Hence, the median of the given data is 68.
Question 9:
Numbers 50, 42, 35, 2x + 10, 2x − 8, 12, 11, 8, 6 are written in descending order and their median is 25, find x.
Answer 9:
Here, the number of observations n is 9. Since n is odd , the median is the 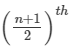 observation, i.e. the 5th observation.
observation, i.e. the 5th observation.
As the numbers are arranged in the descending order, we therefore observe from the last.
Median = 5th observation.
⇒ 25 = 2x -8
⇒ 2x = 25 +8
⇒ 2x = 33
⇒x = 33/2
⇒x = 16.5
Hence, x = 16.5.
Question 10:
Find the median of the following observations : 46, 64, 87, 41, 58, 77, 35, 90, 55, 92, 33. If 92 is replaced by 99 and 41 by 43 in the above data, find the new median?
Answer 10:
Arranging the given data in ascending order, we have:
33, 35, 41, 46, 55, 58, 64, 77, 87, 90, 92
Here, the number of observations n is 11 (odd).
Since the number of observations is odd, therefore,
Median = Value of 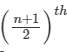 observation = Value of the 6th observation = 58.
observation = Value of the 6th observation = 58.
Hence, median = 58.
If 92 is replaced by 99 and 41 by 43, then the new observations arranged in ascending order are:
33, 35, 43, 46, 55, 58, 64, 77, 87, 90, 99.
∴ New median = Value of the 6th observation = 58.
Question 11:
Find the median of the following data : 41, 43, 127, 99, 61, 92, 71, 58, 57, If 58 is replaced by 85, what will be the new median?
Answer 11:
Arranging the given data in ascending order, we have:
41, 43, 57, 58, 61, 71, 92, 99,127
Here, the number of observations, n, is 9(odd).
∴ Median = Value of 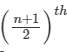 observation = Value of the 5th observation = 61.
observation = Value of the 5th observation = 61.
Hence, the median = 61.
If 58 is replaced by 85 , then the new observations arranged in ascending order are:
41, 43, 57, 61, 71, 85, 92, 99,12 .
∴ New median = Value of the 5th observation = 71.
Question 12:
The weights (in kg) of 15 students are : 31, 35, 27, 29, 32, 43, 37, 41, 34, 28, 36, 44, 45, 42, 30. Find the median. If the weight 44 kg is replaced by 46 kg and 27 kg by 25 kg, find the new median.
Answer 12:
Arranging the given data in ascending order, we have:
27, 28, 29, 30, 31, 32, 34, 35, 36, 37, 41, 42, 43, 44, 45.
Here, the number of observations n is 15(odd).
Since the number of observations is odd, therefore,
Median = Value of 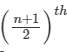 observation = Value of the 8th observation = 35.
observation = Value of the 8th observation = 35.
Hence, median = 35 kg.
If 44 kg is replaced by 46 kg and 27 kg by 25 kg , then the new observations arranged in ascending order are:
25, 28, 29, 30, 31, 32, 34, 35, 36, 37, 41, 42, 43, 45, 46.
∴ New median = Value of the 8th observation = 35 kg.
Question 13:
The following observations have been arranged in ascending order. If the median of the data is 63, find the value of x :
29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
Answer 13:
Here, the number of observations n is 10. Since n is even,

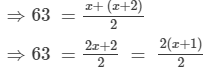
⇒63 = x+1
⇒x = 63−1 = 62.
Hence, x = 62.
FAQs on Ex-23.3, Data Handling II Central Values, Class 7, Math RD Sharma Solutions - RD Sharma Solutions for Class 7 Mathematics
| 1. What are the central values in data handling? |  |
| 2. How is the mean calculated in data handling? |  |
| 3. What does the median represent in data handling? |  |
| 4. How is the mode determined in data handling? |  |
| 5. What role do central values play in data analysis? |  |

|
Explore Courses for Class 7 exam
|

|

















