Symmetry Class 7 Worksheet Maths Chapter 12
Q.1. Fill in the blanks :
(i) Rotation turns an object above a fixed point. This fixed point is called .............
(ii) The order of rotational symmetry of a circle is ..................
(iii) Rhombus is a figure that has ............... lines of symmetry and has a rotational symmetry of order .....................
(iv) ....................... is a figure that has neither a line of symmetry non a rotational symmetry.
 View Answer
View Answer 
Ans.
(i) Centre of rotation
(ii) Infinite or uncountable
(iii) 2, 2
(iv) Quadrilateral
Q.2. State whether the given statements are True or False :
(i) A regular pentagon has no lines of symmetry.
(ii) Mirror reflection leads to symmetry always.
(iii) The number of lines of symmetry of a regular polygon is equal to the vertices of the polygon.
(iv) An angle has two line symmetry.
 View Answer
View Answer 
Ans. (i) False, A regular pentagon actually has five lines of symmetry.
(ii) False, Mirror reflection does not always result in symmetry; it depends on the shape being reflected.
(iii) True
(iv) False, An angle typically has one line of symmetry.
Q.3. Draw all the lines of symmetry for the following letters of they exist.


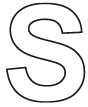

 View Answer
View Answer 
Ans. 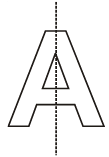
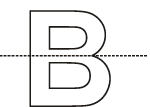

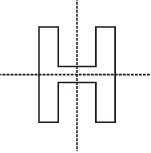
Q.4. In each of the following figures write the number of lines of symmetry and order of rotational symmetry.
(i)  (ii)
(ii) 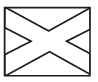 (iii)
(iii)  (iv)
(iv) 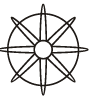
 View Answer
View Answer 
Ans. (i) 1
(ii) 2, 2
(iii) 2,1
(iv) 4, 4
Q.5. The Angle of rotation of a figure is 40°. What is the number of lines of symmetry?
 View Answer
View Answer 
Ans. 9
Q.6. What is the center of rotation of an equilateral triangle?
 View Answer
View Answer 
Ans. Centroid
Q.7. In the given square

(i) Find the number of lines of symmetry. (ii) What is the order of rotational symmetry?
 View Answer
View Answer 
Ans.
(i) Number of lines of symmetry:
A square has 4 lines of symmetry:
Two lines that cut through the midpoints of opposite sides (vertical and horizontal).
Two lines cut through the diagonals of the square.
(ii) Order of rotational symmetry:
The order of rotational symmetry is the number of times the square looks exactly the same during a 360-degree rotation. A square has an order of rotational symmetry of 4, as it looks the same after a rotation of 90 degrees, 180 degrees, 270 degrees, and 360 degrees.
So, the answers are:
(i) The number of lines of symmetry: 4
(ii) The order of rotational symmetry: 4
Q.8. In the given figure :
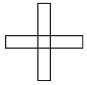
(i) Find the order of rotational symmetry. (ii) Find the degree measure of the anlge of rotation.
 View Answer
View Answer 
Ans.
(i) Order of Rotational Symmetry:
The order of rotational symmetry tells us how many times we can rotate the figure and have it look the same.
In this case, the figure has 4 arms, so it can be rotated by 90 degrees, 180 degrees, or 270 degrees to match its original position.
Therefore, the order of rotational symmetry is 4.
(ii) Degree Measure of the Angle of Rotation:
To find the angle of rotation, we divide a full circle (360 degrees) by the order of rotational symmetry.
Angle of rotation = 360° / Order of symmetry
Angle of rotation = 360° / 4 = 90°
So, the angle of rotation is 90 degrees.
Q.9. Draw all lines of symmetry for each of the following figures given below :
(i) (ii)
(ii) 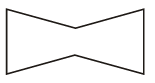 (iii)
(iii) 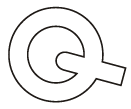 (iv)
(iv) 
 View Answer
View Answer 
Ans.
(i)

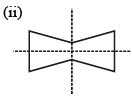
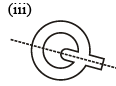
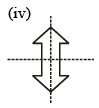
Q.10. Tell whether each figure has rotational symmetry or not.
(i)  (ii)
(ii) 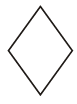 (iii)
(iii)  (iv)
(iv) 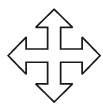
 View Answer
View Answer 
Ans. (i) No
(ii) Yes
(iii) No
(iv) Yes
|
76 videos|386 docs|39 tests
|
FAQs on Symmetry Class 7 Worksheet Maths Chapter 12
| 1. What is symmetry in mathematics? |  |
| 2. How is symmetry used in geometry? |  |
| 3. What are the different types of symmetry? |  |
| 4. How can symmetry be helpful in problem-solving? |  |
| 5. Can symmetry exist in real-life objects and structures? |  |

















