Open App

Class 10 Exam > Class 10 Notes > Extra Documents, Videos & Tests for Class 10 > RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths

Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 PDF Download

1. The following table gives the distribution of total household expenditure (in rupees) of manual workers in a city.

Expenditure (in rupees) (x)	Frequency (f_i)	Expenditure (in rupees) (x_i)	Frequency (f_i)
100 – 150	24	300 – 350	30
150 – 200	40	350 – 400	22
200 – 250	33	400 – 450	16
250 – 300	28	450 – 500	7

Find the average expenditure (in rupees) per household

Sol: Let the assumed mean (A) = 275

Class interval	Mid value (x_i)	d_i= x_i – 275	u_i= (x_i-275)/50	Frequency f_i	f_iu_i
100 – 150	125	-150	-3	24	-12
150 – 200	175	-100	-2	40	-80
200 – 250	225	-50	-1	33	-33
250 – 300	275	0	0	28	0
300 – 350	325	50	1	30	30
350 – 400	375	100	2	22	44
400 – 450	425	150	3	16	48
450 – 500	475	200	4	7	28
				N = 200	Sum = -35

We have

A = 275, h = 50

Mean = A + h * sum/N = 275 + 50 * -35/200 = 275 – 8.75 = 266.25

2. A survey was conducted by a group of students as a part of their environmental awareness program, in which they collected the following data regarding the number of plants in 200 houses in a locality. Find the mean number of plants per house.

Number of plants:	0-2 2-4 4-6 6-B 8-10 10-12 12-14
Number of houses:	1 2 1 5 6 2 3

Which method did you use for finding the mean, and why?

Sol:

Let us find class marks (x_i) = (upper class limit + lower class limit)/2

Now we may compute x_i and f_ix_i as following.

Number of plants	Number of house (f_i)	x_i	F_ix_i
0 – 2	1	1	1
2 – 4	2	3	6
4 – 6	1	5	5
6 – 8	5	7	35
8 – 10	6	9	54
10 – 12	2	11	22
12 – 14	3	13	39
Total	N = 20		Sum = 162

From the table we may observe that

N = 20

Sum = 162

Mean 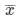Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 = Sum/N

162/20 = 8.1

So mean number of plants per house is 8.1

We have used for the direct method values X_iand f_i are very small

3. Consider the following distribution of daily wages of workers of a factory

Daily wages (in Rs)	100-120 120-140 140-160 160-180 180-200
Number of workers:	12 16 8 6 10

Find the mean daily wages of the workers of the factory by using an appropriate method.

Sol: Let the assume mean (A) = 150

Class interval	Mid value x_i	d_i= x_i – 150	u_i = (x_i-150)/20	Frequency f_i	F_iu_i
100 – 120	110	-40	-2	12	-24
120 – 140	130	-20	-1	14	-14
140 – 160	150	0	0	8	0
160 – 180	170	20	1	6	6
180 – 200	190	40	2	10	20
			N = 50	Sum = -12

We have

N = 50, h = 20

Mean = A + h x sum/N

= 150 + 2 x (-15)/5

= 150 – 4.8

= 145.2

4.Thirty women were examined in a hospital by a doctor and the number of heart beats per minute recorded and summarized as follows. Find the mean heart beats per minute for these women, choosing a suitable method.

Number of heart

Beats Per minute:	65 – 68 68 – 71 71 – 74 74 – 77 77 – 80 80 – 83 83 – 86
Number of women:	2 4 3 8 7 4 2

Sol: we may find marks of each interval (x_i) by using the relation

(x_i) = (upper class limit + lower class limit)/2

Class size of this data = 3

Now talking 75.5 as assumed mean (a)

We may calculate d_i, u_i, f_iu_i as following

Number of heart beats per minute	Number of women (x_i)	x_i	d_i = x_i – 75.5	u_i = (x_i– 755)/h	f_iu_i
65-68	2	66.5	-9	-3	-6
68-71	9	69.5	-6	-2	-8
71-74	3	72.5	-3	-1	-3
74-77	8	75.5	0	0	0
77-80	7	78.5	3	1	7
80-83	4	81.5	6	2	8
83-86	2	84.5	9	3	6
	N = 30				Sum = 4

Now we may observe from table that N = 30, sum = 4

Mean 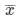Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 = 75.5 + (4/3)x3

= 75.5 + 0.4

= 75.9

So mean heart beats per minute for those women are 75.9 beats per minute

5.Find the mean of each of the following frequency distributions: (5 – 14)

Class interval:	0-6 6-12 12-18 18-24 24-30
Frequency:	6 8 10 9 7

Sol:

Let us assume mean be 15

Class interval	Mid – value	d_i= x_i– 15	u_i= (x_i– 15)/6	f_i	f_iu_i
0 – 6	3	-12	-2	6	-12
6 – 12	9	-6	-1	8	-8
12 – 18	15	0	0	10	0
18 – 24	21	6	1	9	9
24 – 30	27	18	2	7	14
				N = 40	Sum = 3

A = 15, h = 6

Mean = A + h(sum/A)

= 15 + 6(3/40)

= 15 + 0.45

= 15.45

Class interval:	50-70 70-90 90-110 110-130 130-150 150-170
Frequency:	18 12 13 27 8 22

Sol: Let us assumed mean be 100

Class interval	Mid-value x_i	d_i= x_i – 100	u_i= (x_i – 100)/20	f_i	f_iu_i
50 – 70	60	-40	-2	18	-36
70 – 90	80	-20	-1	12	-12
90 – 110	100	0	0	13	0
110 – 130	120	20	1	27	27
130 – 150	140	40	2	8	16
150 – 170	160	60	3	22	66
					61

A = 100, h = 20

Mean = 100 + 20 (61/100)

= 100 + 12.2

= 112.2

Class interval:	0-8 8-16 16-24 24-32 32-40
Frequency:	6 7 10 8 9

Sol: Let the assumed mean (A) = 20

Class interval	Mid- value	d_i= x_i– 20	u_i = (x_i– 20)/8	f_i	f_iu_i
0-8	4	-16	-2	6	-12
8-16	12	-8	-1	7	-7
16-24	20	0	0	10	0
24-32	28	8	1	8	8
32-40	36	16	2	9	18
				N = 40	Sum = 7

We have A = 20, h = 8

Mean= A + h (sum/N)

= 20 + 8 (7/40)

= 20 + 1.4

= 21.4

Class interval:	0 – 6 6 – 12 12 – 18 18 – 24 24 – 30
Frequency:	7 5 10 12 6

Sol: Let the assumed mean be (A) = 15

Class interval	Mid – value	d_i= x_i-15	u_i= (x_i-15)/6	Frequency f_i	f_iu_i
0 – 6	3	-12	-2	-1	-14
6 – 12	9	-6	-1	5	-5
12 – 18	15	0	0	10	0
18 – 24	21	6	1	12	12
24 – 30	27	12	2	6	12
				N = 40	Sum = 5

We have

A = 15, h = 6

Mean = A + h(sum/N)

= 15 + 6 (5/40)

= 15 + 0.75

= 15.75

Class interval:	0 – 10 10 – 20 20 – 30 30 – 40 40 – 50
Frequency:	9 12 15 10 14

Sol: Let the assumed mean (A) = 25

Class interval	Mid – value	d_i= x_i-25	u_i= (x_i-25)/10	Frequency f_i	f_iu_i
0 – 10	5	-20	-2	9	-18
10 – 20	15	-10	-1	10	-12
20 – 30	25	0	0	15	0
30 – 40	35	10	1	10	10
40 – 50	45	20	2	14	28
				N = 60	Sum = 8

We have A = 25, h = 10

Mean = A + h(sum/N)

= 25 + 19 (8/60)

= 25 + (4/3)

= 26.333

10.

Class interval:	0-8 8-16 16-24 24-32 32-40
Frequency:	5 9 10 8 8

Sol: Let the assumed mean (A) = 20

Class interval	Mid value x_i	d_i= x_i – 20	u_i= (x_i-20)/8	Frequency f_i	f_iu_i
0-8	4	-16	-2	5	-10
8-16	12	-8	-1	9	-9
16-24	20	0	0	10	0
24-32	28	8	1	8	8
32-40	36	16	2	8	16
				N = 40	Sum = 5

We have,

A = 20, h = 8

Mean = A + h (sum/N)

= 20 + 8 (5/ 40)

= 20 + 1

= 21

11.

Class interval:	0-8 8-16 16-24 24-32 32-40
Frequency:	5 6 4 3 2

Sol: Let the assumed mean (A) = 20

Class interval	Mid value x_i	d_i= x_i – 20	u_i= (x_i-20)/8	Frequency f_i	f_iu_i
0-8	4	-16	-2	-2	-10
8-16	12	-8	-1	-1	-6
16-24	20	0	0	0	0
24-32	28	8	1	1	3
32-40	36	16	2	2	4
				N = 20	Sum = -9

We have,

A = 20, h = 8

Mean = A + h (sum/N)

= 20 + 8 (-9/ 20)

= 20 – (72/20)

= 20 – 3.6

= 16.4

12.

Class interval:	10-30 30-50 50-70 70-90 90-110 110-130
Frequency:	5 8 12 20 3 2

Sol: Let the assumed mean (A) = 60

Class interval	Mid value x_i	d_i= x_i – 60	u_i= (x_i-60)/20	Frequency f_i	f_iu_i
10 – 30	20	-40	-2	5	-10
30 – 50	40	-20	-1	8	-8
50 – 70	60	0	0	12	0
70 – 90	80	20	1	20	20
90 – 110	100	40	2	3	6
110 – 130	120	60	3	2	6
				N = 50	Sum = 14

We have

A = 60, h = 20

Mean = A + h (sum/N)

= 60 + 20 (14/ 5)

= 60 + 5.6

= 65.6

13.

Class interval:	25-35 35-45 45-55 55-65 65-75
Frequency:	6 10 8 10 4

Sol: Let the assumed mean (A) = 50

Class interval	Mid value x_i	d_i= x_i – 50	u_i= (x_i– 50)/ 10	Frequency f_i	f_iu_i
25 – 35	30	-20	-2	6	-12
35 – 45	40	-10	-1	10	-10
45 – 55	50	0	0	8	0
55 – 65	60	10	1	12	12
65 – 75	70	20	2	4	8
				N = 40	Sum = -2

We have

A = 50, h = 10

Mean = A + h (sum/N)

= 50 + 10 (-2/ 40)

= 50 – 0.5

= 49.5

14.

Class interval:	25-29 30-34 35-39 40-44 45-49 50-54 55-59
Frequency:	14 22 16 6 5 3 4

Sol: Let the assumed mean (A) = 42

Class interval	Mid value x_i	d_i= x_i – 42	u_i= (x_i– 42)/ 5	Frequency f_i	f_iu_i
25 – 29	27	-15	-3	14	-42
30 – 34	32	-10	-2	22	-44
35 – 39	37	-5	-1	16	-16
40 – 44	42	0	0	6	0
45 – 49	47	5	1	5	5
50 – 54	52	10	2	3	6
55 – 59	57	15	3	4	12
				N = 70	Sum = -79

We have

A = 42, h = 5

Mean = A + h (sum/N)

= 42 + 5 (-79/70)

= 42 – 79/14

= 36.357

15.For the following distribution, calculate mean using all suitable methods:

Size of item:	1 – 4 4 – 9 9 – 16 16 – 20
Frequency:	6 12 26 20

Sol: By direct method

Class interval	Mid value x_i	Frequency f_i	f_ix_i
1 – 4	2.5	6	15
4 – 9	6.5	12	18
9 – 16	12.5	26	325
16 – 27	21.5	20	430
		N = 64	Sum = 848

Mean = (sum/N) + A

= 848/ 64

= 13.25

By assuming mean method

Let the assumed mean (A) = 65

Class interval	Mid value x_i	u_i= (x_i– A) =x_i– 65	Frequency f_i	f_iu_i
1 – 4	2.5	-4	6	-25
4 – 9	6.5	0	12	0
9 – 16	12.5	6	26	196
16 – 27	21.5	15	20	300
			N = 64	Sum = 432

Mean = A + sum/N

= 6.5 + 6.75

= 13.25

16.The weekly observation on cost of living index in a certain city for the year 2004 – 2005 are given below. Compute the weekly cost of living index.

Cost of living index	Number of students	Cost of living index	Number of students
1400 – 1500	5	1700 – 1800	9
1500 – 1600	10	1800 – 1900	6
1600 – 1700	20	1900 – 2000	2

Sol: Let the assumed mean (A) = 1650

Class interval	Mid value x_i	d_i= x_i – A = x_i– 1650	u_i= (x_i– 1650) 100	Frequency f_i	f_iu_i
1400 – 1500	1450	-200	-2	5	-10
1500 – 1600	1550	-100	-1	10	-10
1600 – 1700	1650	0	0	20	0
1700 – 1800	1750	100	1	9	9
1800 – 1900	1850	200	2	6	12
1900 – 2000	1950	300	3	2	6
				N = 52	Sum = 7

We have

A = 16, h = 100

Mean = A + h (sum/N)

= 1650 + 100 (7/52)

= 1650 + (175/13)

= 21625/13

= 1663.46

17.The following table shows the marks scored by 140 students in an examination of a certain paper:

Marks:	0-10 10-20 20-30 30-40 40-50
Number of students:	20 24 40 36 20

Calculate the average marks by using all the three methods: direct method, assumed mean deviation and shortcut method.

Sol: (i) Direct method:

Class interval	Mid value x_i	Frequency f_i	f_ix_i
0 – 10	5	20	100
10 – 20	15	24	360
20 – 30	25	40	1000
30 – 40	35	36	1260
40 – 50	45	20	900
		N = 140	Sum = 3620

Mean = sum/ N

= 3620/ 140

= 25.857

(ii)Assumed mean method:

Let the assumed mean = 25

Mean = A + (sum/ N)

Class interval	Mid value x_i	u_i= (x_i– A)	Frequency f_i	f_iu_i
0 – 10	5	-20	20	-400
10 – 20	15	-10	24	-240
20 – 30	25	0	40	0
30 – 40	35	10	36	360
40 – 50	45	20	20	400
			N = 140	Sum = 120

Mean = A + (sum/ N)

= 25 + (120/ 140)

= 25 + 0.857

= 25.857

(iii)Step deviation method:

Let the assumed mean (A) = 25

Class interval	Mid value x_i	d_i= x_i – A = x_i– 25	u_i= (x_i– 25) 10	Frequency f_i	f_iu_i
0 – 10	5	-20	-2	20	-40
10 – 20	15	-10	-1	24	-24
20 – 30	25	0	0	40	0
30 – 40	35	10	1	36	36
40 – 50	45	20	2	20	40
				N = 140	Sum = 12

Mean = A + h(sum/ N)

= 25 + 10(12/ 140)

= 25 + 0.857

= 25.857

18.The mean of the following frequency distribution is 62.8 and the sum of all the frequencies is 50. Compute the miss frequency f₁and f₂.

Class:	0-20 20-40 40-60 60-80 80-100 100-120
Frequency:	5 f₁10 f₂7 8

Sol:

Class interval	Mid value x_i	Frequency f_i	f_ix_i
0 – 20	10	5	50
20 – 40	30	f₁	30f₁
40 – 60	50	10	500
60 – 80	70	f₂	70f₂
80 – 100	90	7	630
100 – 120	110	8	880
		N = 50	Sum = 30f1+70f₂+ 2060

Given,

sum of frequency = 50

5 + f₁+ 10 + f₂+ 7 + 8 = 50

f₁+ f₂= 20

3f₁+ 3f₂= 60 —- (1) [multiply both side by 3]

And mean = 62.8

Sum/ N = 62.8

(30f1+70f₂+ 2060)/ 50 = 62.8

30f1+70f₂= 3140 – 2060

30f1+70f₂= 1080

3f1 + 7f₂= 108 —- (2) [divide it by 10]

subtract equation (1) from equation (2)

3f1 + 7f₂– 3f₁– 3f₂= 108 – 60

4f₂ = 48

f₂= 12

Put value of f₂ in equation (1)

3f₁+ 3(12) = 60

f₁= 24/3 = 8

f₁= 8, f₂= 12

19.The following distribution shows the daily pocket allowance given to the children of a multistory building. The average pocket allowance is Rs 18.00. Find out the missing frequency.

Class interval:	11-13 13-15 15-17 17-19 19-21 21-23 23-25
Frequency:	7 6 9 13 – 5 4

Sol: Given mean = 18,

Let the missing frequency be v

Class interval	Mid value x_i	Frequency f_i	f_ix_i
11 – 13	12	7	84
13 – 15	14	6	88
15 – 17	16	9	144
17 – 19	18	13	234
19 – 21	20	x	20x
21 – 23	22	5	110
23 – 25	14	4	56
		N =44 + x	Sum = 752 + 20x

Mean = sum/ N

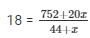
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

792 + 18x = 752 + 20x

2x = 40

x = 20

20.If the mean of the following distribution is 27. Find the value of p.

Class:	0-10 10-20 20-30 30-40 40-50
Frequency:	8 p 12 13 10

Sol:

Class interval	Mid value x_i	Frequency f_i	f_ix_i
0 – 10	5	8	40
10 – 20	15	P	152
20 – 30	25	12	300
30 – 40	35	13	455
40 – 50	45	16	450
		N = 43 + P	Sum = 1245 + 15p

Given mean =27

Mean = sum/ N

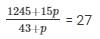
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

1245 + 15p = 1161 + 27p

12p = 84

P = 7

21.In a retail market, fruit vendors were selling mangoes kept in packing boxes. These boxes contain varying number of mangoes. The following was the distribution of mangoes according to the number of boxes.

Number of mangoes:	50-52 53-55 56-58 59-61 62-64
Number of boxes:	15 110 135 115 25

Find the mean number of mangoes kept in packing box. Which method of finding the mean did you choose?

Sol:

Number of mangoes	Number of boxes (f_i)
50 – 52	15
53 – 55	110
56 – 58	135
59 – 61	115
62 – 64	25

We may observe that class internals are not continuous

There is a gap between two class intervals. So we have to add ½ from lower class limit of each interval and class mark (x_i) may be obtained by using the relation

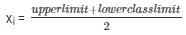
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

Class size (h) of this data = 3

Now taking 57 as assumed mean (a) we may calculated d_i,u_i, f_iu_i as follows

Class interval	Frequency f_i	Mid value x_i	d_i= x_i – A = x_i– 25	u_i= (x_i– 25) 10	f_iu_i
49.5 – 52.5	15	51	-6	-2	-30
52.5 – 55.5	110	54	-3	-1	-110
55.5 – 58.5	135	57	0	0	0
58.5 – 61.5	115	60	3	1	115
61.5 – 64.5	25	63	6	2	50
Total	N = 400				Sum = 25

Now we have N = 400

Sum = 25

Mean = A + h (sum/ N)

= 57 + 3 (45/400)

= 57 + 3/ 16

= 57+ 0.1875

= 57.19

Clearly mean number of mangoes kept in packing box is 57.19

22.The table below shows the daily expenditure on food of 25 households in a locality

Daily expenditure (in Rs):	100-150 150-200 200-250 250-300 300-350
Number of households:	4 5 12 2 2

Find the mean daily expenditure on food by a suitable method.

Sol: we may calculate class mark (x_i) for each interval by using the relation

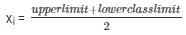
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

Class size = 50

Now, talking 225 as assumed mean (x_i) we may calculate d_i,u_i, f_iu_i as follows

Daily expenditure	Frequency f_i	Mid value x_i	d_i= x_i– 225	u_i= (x_i– 225) 50	f_iu_i
100 – 150	4	125	-100	-2	-8
150 – 200	5	175	-50	-1	-5
200 – 250	12	225	0	0	0
250 – 300	2	275	50	1	2
300 – 350	2	325	100	2	4
	N = 25				Sum = -7

Now we may observe that

N = 25

Sum = -7

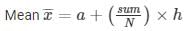
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

225 + 50 (-7/ 25)

225 – 14 = 211

So, mean daily expenditure on food is Rs 211

23.To find out the concentration of SO₂in the air (in parts per million i.e ppm) the data was collected for localities for 30 localities in a certain city and is presented below:

Concentration of SO₂(in ppm)	Frequency
0.00 – 0.04	4
0.04 – 0.08	9
0.08 – 0.12	9
0.12 – 0.16	2
0.16 – 0.20	4
0.20 – 0.24	2

Find the mean concentration of SO₂in the air

Sol: we may find class marks for each interval by using the relation

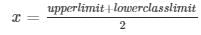
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

Class size of this data = 0.04

Now taking 0.04 assumed mean (x_i) we may calculate d_i,u_i, f_iu_i as follows

Concentration of SO₂	Frequency f_i	Class interval x_i	d_i = x_i– 0.14	u_i	f_iu_i
0.00 – 0.04	4	0.02	-0.12	-3	-12
0.04 – 0.08	9	0.06	-0.08	-2	-18
0.08 – 0.12	9	0.10	-0.04	-1	-9
0.12 – 0.16	2	0.14	0	0	0
0.16 – 0.20	4	0.18	0.04	1	4
0.20 – 0.24	2	0.22	0.08	2	4
Total	N = 30				Sum = -31

From the table we may observe that

N = 30

Sum = -31

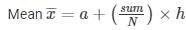
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

= 0.14 +(0.04)(-31/30)

= 0.099 ppm

So mean concentration of SO₂in the air is 0.099 ppm

24.A class teacher has the following absentee record of 40 students of a class for the whole term. Find the mean number of days a student was absent.

Number of days:	0-6 6-10 10-14 14-20 20-28 28-38 38-40
Number of students:	11 10 7 4 4 3 1

Sol: We may find class mark of each interval by using the relation

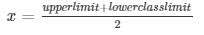
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

Now, taking 16 as assumed mean (a) we may

Calculate d_i and f_id_ias follows

Number of days	Number of students f_i	X_i	d = x_i+ 10	f_id_i
0 – 6	11	3	-13	-143
6 – 10	10	8	-8	-280
10 – 14	7	12	-4	-28
14 – 20	7	16	0	0
20 – 28	8	24	8	32
28 – 36	3	33	17	51
30 – 40	1	39	23	23
Total	N = 40			Sum = -145

Now we may observe that

N = 40

Sum= -145

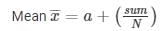
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

= 16 + (-145/ 40)

= 16 – 3.625

= 12.38

So mean number of days is 12.38 days, for which student was absent

25.The following table gives the literacy rate (in percentage) of 35 cities. Find the mean literacy rate.

Literacy rate (in %):	45-55 55-65 65-75 75-85 85-95
Number of cites:	3 10 11 8 3

Sol: We may find class marks by using the relation

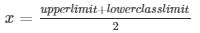
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

Class size (h) for this data = 10

Now taking 70 as assumed mean (a) wrong

Calculate d_i,u_i, f_iu_i as follows

Litracy rate (in %)	Number of cities (f_i)	Mid value x_i	d_i= x_i– 70	u_i= d_i 50	f_iu_i
45 – 55	3	50	-20	-2	-6
55 – 65	10	60	-10	-1	-10
65 – 75	11	70	0	0	0
75 – 85	8	80	10	1	8
85 – 95	3	90	20	2	6
Total	N = 35				Sum = -2

Now we may observe that

N = 35

Sum = -2

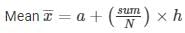
Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

= 70 + (-2/35)

= 70 – 4/7

= 70 – 0.57

= 69.43

So, mean literacy rate is 69.43 %

The document Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 is a part of the Class 10 Course Extra Documents, Videos & Tests for Class 10.

All you need of Class 10 at this link: Class 10

Extra Documents, Videos & Tests for Class 10

5 videos|292 docs|59 tests

Join Course for Free

Top Courses for Class 10

View all

FAQs on Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions - Extra Documents, Videos & Tests for Class 10

1. What are RD Sharma Solutions?

Ans. RD Sharma Solutions are a set of comprehensive solutions provided for the RD Sharma textbook. These solutions are designed to help students understand and solve the different mathematical problems and exercises given in the book.

2. What is Ex-7.3 Statistics in RD Sharma Class 10 Maths?

Ans. Ex-7.3 Statistics is a chapter in the RD Sharma Class 10 Maths textbook that covers the topic of statistics. It includes concepts such as mean, median, mode, and graphical representation of data. This exercise provides various problems and exercises for students to practice and develop their understanding of statistics.

3. How can RD Sharma Solutions for Ex-7.3 Statistics help in exam preparation?

Ans. RD Sharma Solutions for Ex-7.3 Statistics can be extremely helpful in exam preparation. These solutions provide step-by-step explanations for solving the problems in the textbook. By referring to these solutions, students can understand the concepts and techniques required to solve statistical problems effectively. Practicing these solutions can also help students improve their problem-solving skills and increase their chances of scoring well in the exams.

4. Are the RD Sharma Solutions for Ex-7.3 Statistics available online?

Ans. Yes, RD Sharma Solutions for Ex-7.3 Statistics are available online. There are several websites and educational platforms that provide these solutions for free. Students can easily access these solutions and use them for reference and practice. They can also download these solutions in PDF format for offline use.

5. Can RD Sharma Solutions for Ex-7.3 Statistics be used for self-study?

Ans. Yes, RD Sharma Solutions for Ex-7.3 Statistics can be used for self-study. These solutions are designed to be easily understandable and comprehensive. By referring to these solutions, students can learn and practice the concepts of statistics on their own. They can solve the problems given in the textbook and then cross-check their solutions with the provided RD Sharma Solutions to assess their understanding and identify any mistakes. Self-study using these solutions can be a great way to reinforce concepts and prepare for exams.

Related Exams

Class 10

About this Document

Nov 19, 2024 Last updated

Document Description: RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths for Class 10 2024 is part of Extra Documents, Videos & Tests for Class 10 preparation. The notes and questions for RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths have been prepared according to the Class 10 exam syllabus. Information about RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths covers topics like and RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Example, for Class 10 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths.

Introduction of RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths in English is available as part of our Extra Documents, Videos & Tests for Class 10 for Class 10 & RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths in Hindi for Extra Documents, Videos & Tests for Class 10 course. Download more important topics related with notes, lectures and mock test series for Class 10 Exam by signing up for free. Class 10: Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10

Description

Full syllabus notes, lecture & questions for Ex-7.3 Statistics, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 - Class 10 | Plus excerises question with solution to help you revise complete syllabus for Extra Documents, Videos & Tests for Class 10 | Best notes, free PDF download

Information about RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths

In this doc you can find the meaning of RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths defined & explained in the simplest way possible. Besides explaining types of RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths theory, EduRev gives you an ample number of questions to practice RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths tests, examples and also practice Class 10 tests

Extra Documents, Videos & Tests for Class 10

5 videos|292 docs|59 tests

Join Course for Free

Download as PDF

Explore Courses for Class 10 exam

Top Courses for Class 10

Explore Courses

Signup for Free!

Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

Start learning for Free

10M+ students study on EduRev

Semester Notes

Videos & Tests for Class 10

practice quizzes

Viva Questions

MCQs

shortcuts and tricks

Maths RD Sharma Solutions | Extra Documents

Previous Year Questions with Solutions

Class 10

mock tests for examination

Ex-7.3 Statistics

Videos & Tests for Class 10

pdf

video lectures

Extra Questions

Important questions

Free

Class 10

Sample Paper

ppt

Ex-7.3 Statistics

Videos & Tests for Class 10

study material

Summary

Exam

Class 10

Maths RD Sharma Solutions | Extra Documents

Objective type Questions

past year papers

Maths RD Sharma Solutions | Extra Documents

;

Additional Information about RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths for Class 10 Preparation

RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Free PDF Download

The RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths is an invaluable resource that delves deep into the core of the Class 10 exam. These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective. With the help of these notes, you can grasp complex subjects quickly, revise important points easily, and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner, allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material, or toppers' notes, this PDF has got you covered. Download the RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths now and kickstart your journey towards success in the Class 10 exam.

Importance of RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths

The importance of RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths cannot be overstated, especially for Class 10 aspirants. This document holds the key to success in the Class 10 exam. It offers a detailed understanding of the concept, providing invaluable insights into the topic. By knowing the concepts well in advance, students can plan their preparation effectively. Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.

RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Notes

RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Notes offer in-depth insights into the specific topic to help you master it with ease. This comprehensive document covers all aspects related to RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths. It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation. Practice papers and question papers enable you to assess your progress effectively. Additionally, the paper analysis provides valuable tips for tackling the exam strategically. Access to Toppers' notes gives you an edge in understanding complex concepts. Whether you're a beginner or aiming for advanced proficiency, RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Notes on EduRev are your ultimate resource for success.

RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Class 10 Questions

The "RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths Class 10 Questions" guide is a valuable resource for all aspiring students preparing for the Class 10 exam. It focuses on providing a wide range of practice questions to help students gauge their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation. The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level. Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.

Study RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths on the App

Students of Class 10 can study RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths alongwith tests & analysis from the EduRev app, which will help them while preparing for their exam. Apart from the RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths, students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc. The EduRev App will make your learning easier as you can access it from anywhere you want. The content of RD Sharma Solutions - Ex-7.3 Statistics, Class 10, Maths is prepared as per the latest Class 10 syllabus.

Education Revolution

Signup to see your scores go up within 7 days!

Access 1000+ FREE Docs, Videos and Tests

Continue with Google

Takes less than 10 seconds to signup