Ex-8.10 Quadratic Equations, Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 PDF Download

Q.1)  The hypotenuse of a right triangle is 25 cm. The difference between the lengths of the other two sides of the triangle is 5 cm. Find the lengths of these sides.

Sol:  let the length of one side of the right triangle be x cm then,

the other side be = (x + 5) cm

and given that hypotenuse = 25 cm

By using Pythagoras Theorem,

x² + (x + 5)²  = 25²

x² + x² + 10x + 25 = 625

2x² + 10x + 25 – 625 = 0

2x² + 10x – 600 = 0

x² + 5x – 300 = 0

x² – 15x + 20x – 300 = 0

x(x – 15) + 20(x -15) = 0

(x – 15)(x + 20) = 0

x = 15  or x = – 20

Since, the side of triangle can never be negative

Therefore, when, x = 15

And, x + 5 = 15 + 5 = 20

Therefore, length of side of right triangle is = 15 cm and other side is = 20 cm

Q.2:  The diagonal of a rectangular field is 60 meters more than the shorter side. If the longer side is 30 meters more than the shorter side, find the sides of the field.

Sol:

let the length of smaller side of rectangle be x metres then, the larger side be (x + 30) metres and

diagonal be = (x + 60) metres

By using Pythagoras theorem,

x² + (x + 30)²  = (x + 60)²

x² + x² + 60x + 900 = x² + 120x + 3600

2x² + 60x + 900 – x² – 120x – 3600 = 0

x² – 60x – 2700 = 0

x² – 90x + 30x – 2700 = 0

x(x – 90) + 30(x – 90) = 0

(x – 90)(x + 30) = 0

x = 90   or x = -30

Since, the side of rectangle can never be negative

Therefore, x = 90

x + 30 = 90 + 30 = 120

Therefore, the length of smaller side of rectangle is = 90 metres and larger side is = 120 metres.

Q.3:  The hypotenuse of a right triangle is 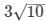 cm. If the smaller leg is tripled and the longer leg doubled, new hypotenuse will be 9√5cm. How long are the legs of the triangle?

Sol:

let the length of smaller side of right triangle be = x cm then large side be = y cm

By using Pythagoras theorem,

x² + y² =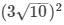

x² + y²  = 90  ….eqn.(1)

If the smaller side is triple and the larger side is doubled, the new hypotenuse is 9√5cm

Therefore,

(3x)² + (2y)²  =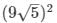  

9x² + 4y²  = 405  ….eqn.(2)

From equation (1) we get,

y²  = 90 – x²

Now putting the value of y² in eqn. (2)

9x² + 4(90 – x²) = 405

9x² + 360 – 4X² – 405 = 0

5x² – 45 = 0

5(x² – 9) = 0

x² – 9 = 0

x²  = 9

x = √9 

x = ± 3

Since, the side of triangle can never be negative

Therefore, when x = 3

Then,  y²  = 90 – x²  = 90 – (3)²  = 90 – 9 = 81

y =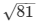

y = ± 9

Hence, the length of smaller side of right triangle is = 3cm and larger side is = 9cm

Q.4)  A pole has to be erected at a point on the boundary of a circular park of diameter meters in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 meters. Is it possible to do so? If yes, at what distances from the two gates should the pole be erected?

Sol:

let P be the required location on the boundary of circular park such that its distance from the gate B is x metres that is BP = x metres

Then, AP = x + 7

In right triangle ABP, by using Pythagoras theorem,

AP² + BP²  = AB²

(x + 7)² + x²   = 13²

x² + 14x + 49 + x²  = 169

2x²  + 14x + 49 – 169 = 0

2x² + 14x – 120 = 0

2(x² + 7x – 60) = 0

x² + 12x – 5x – 60 = 0

x(x + 12) – 5(x + 12) = 0

(x + 12)(x – 5) = 0

x = – 12  or x = 5

Since, the side of triangle can never be negative

Therefore, P is at a distance of 5 metres from the gate B