Coordinate Geometry Exercise 14.1 (Part-8) | Extra Documents, Videos & Tests for Class 10 PDF Download
Question 30: Find the coordinates of the points which divide the line segment joining the points (−4, 0) and (0, 6) in four equal parts.
Answer :
The co-ordinates of the midpoint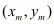 
between two points 
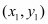and 
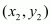is given by,
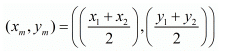
Here we are supposed to find the points which divide the line joining A(−4,0) and B(0,6) into 4 equal parts.
We shall first find the midpoint M(x, y) of these two points since this point will divide the line into two equal parts
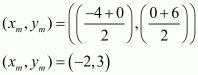
So the point M(−2,3) splits this line into two equal parts.
Now, we need to find the midpoint of A(−4,0) and M(−2,3) separately and the midpoint of B(0,6) and M(−2,3). These two points along with M(−2,3) split the line joining the original two points into four equal parts.
Let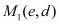 
be the midpoint of A(−4,0) and M(−2,3).
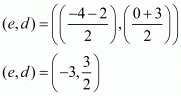
Now let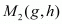 
bet the midpoint of B(0,6) and M(−2,3).
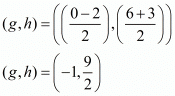
Hence the co-ordinates of the points which divide the line joining the two given points are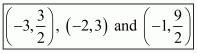
.
Question 31: Show that the mid-point of the line segment joining the points (5, 7) and (3, 9) is also the mid-point of the line segment joining the points (8, 6) and (0, 10).
Answer :
We have two points A (5, 7) and B (3, 9) which form a line segment and similarly
C (8, 6) and D (0, 10) form another line segment.
We have to prove that mid-point of AB is also the mid-point of CD.
In general to find the mid-point
of two points
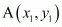and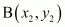
we use section formula as,
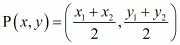
Therefore mid-point P of line segment AB can be written as,
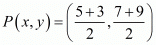
Now equate the individual terms to get,
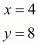
So co-ordinates of P is (4, 8)
Similarly mid-point Q of side CD can be written as,
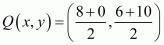
Now equate the individual terms to get,
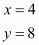
So co-ordinates of Q is (4, 8)
Hence the point P and Q coincides.
Thus mid-point of AB is also the mid-point of CD.
Question 32: Find the distance of the point (1, 2) from the mid-point of the line segment joining the points (6, 8) and (2, 4).
Answer :
We have to find the distance of a point A (1, 2) from the mid-point of the line segment joining P (6, 8) and Q (2, 4).
In general to find the mid-point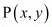
of any two points
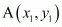and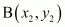
we use section formula as,
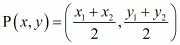
Therefore mid-point B of line segment PQ can be written as,
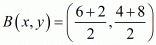
Now equate the individual terms to get,
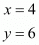
So co-ordinates of B is (4, 6)
Therefore distance between A and B,
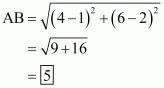
Question 33: If A and B are (1, 4) and (5, 2) respectively, find the coordinates of P when AP/BP = 3/4.
Answer :
The co-ordinates of the point dividing two points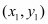 
and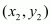 
in the ratio 
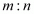is given as,
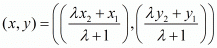where,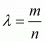 
Here the two given points are A(1,4) and B(5,2). Let point P(x, y) divide the line joining ‘AB’ in the ratio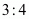 
Substituting these values in the earlier mentioned formula we have,
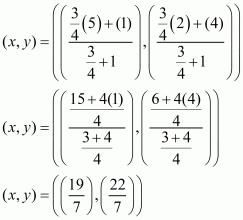
Thus the co-ordinates of the point which divides the given points in the required ratio are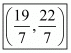
.
Question 34: Show that the points A (1, 0), B (5, 3), C (2, 7) and D (−2, 4) are the vertices of a parallelogram.
Answer :
Let A (1, 0); B (5, 3); C (2, 7) and D (−2, 4) be the vertices of a quadrilateral. We have to prove that the quadrilateral ABCD is a parallelogram.
We should proceed with the fact that if the diagonals of a quadrilateral bisect each other than the quadrilateral is a parallelogram.
Now to find the mid-point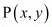
of two points
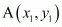and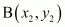
we use section formula as,
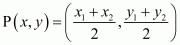
So the mid-point of the diagonal AC is,
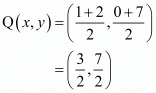
Similarly mid-point of diagonal BD is,
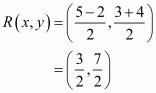
Therefore the mid-points of the diagonals are coinciding and thus diagonal bisects each other.
Hence ABCD is a parallelogram.
Question 35: Determine the ratio in which the point P (m, 6) divides the join of A(−4, 3) and B(2, 8). Also, find the value of m.
Answer :
The co-ordinates of a point which divided two points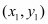 
and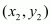 
internally in the ratio 
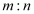is given by the formula,
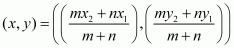
Here we are given that the point P(m,6) divides the line joining the points A(−4,3) and B(2,8) in some ratio.
Let us substitute these values in the earlier mentioned formula.
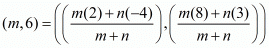
Equating the individual components we have
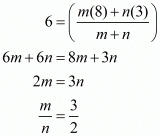
We see that the ratio in which the given point divides the line segment is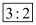
.
Let us now use this ratio to find out the value of ‘m’.
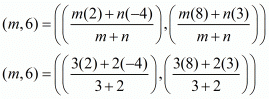
Equating the individual components we have
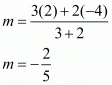
Thus the value of ‘m’ is
.
Question 36:Determine the ratio in which the point (−6, a) divides the join of A (−3, 1) and B (−8, 9). Also find the value of a.
Answer :
The co-ordinates of a point which divided two points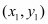 
and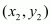 
internally in the ratio 
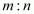is given by the formula,
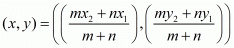
Here we are given that the point P(−6,a) divides the line joining the points A(−3,1) and B(−8,9) in some ratio.
Let us substitute these values in the earlier mentioned formula.
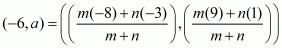
Equating the individual components we have
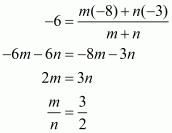
We see that the ratio in which the given point divides the line segment is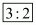
.
Let us now use this ratio to find out the value of ‘a’.
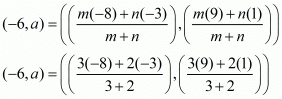
Equating the individual components we have
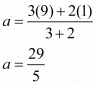
Thus the value of ‘a’ is
.
Question 37:
ABCD is a rectangle formed by joining the points A (−1, −1), B(−1 4) C (5 4) and D (5, −1). P, Q, R and S are the mid-points of sides AB, BC, CD and DA respectively. Is the quadrilateral PQRS a square? a rectangle? or a rhombus? Justify your answer.
Answer :
We have a rectangle ABCD formed by joining the points A (−1,−1); B (−1, 4); C (5, 4) and D (5,−1). The mid-points of the sides AB, BC, CD and DA are P, Q, R, S respectively.
We have to find that whether PQRS is a square, rectangle or rhombus.
In general to find the mid-point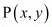
of two points
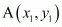and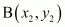
we use section formula as,
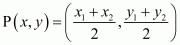
Therefore mid-point P of side AB can be written as,
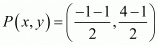
Now equate the individual terms to get,
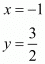
So co-ordinates of P is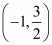 
Similarly mid-point Q of side BC can be written as,
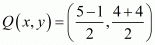
Now equate the individual terms to get,
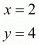
So co-ordinates of Q is (2, 4)
Similarly mid-point R of side CD can be written as,
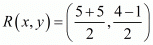
Now equate the individual terms to get,
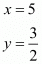
So co-ordinates of R is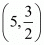 
Similarly mid-point S of side DA can be written as,
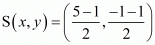
Now equate the individual terms to get,
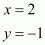
So co-ordinates of S is (2,−1)
So we should find the lengths of sides of quadrilateral PQRS.
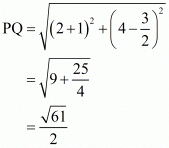
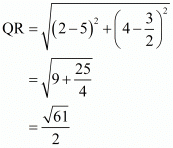
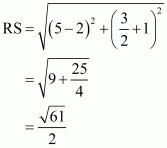
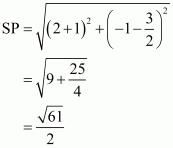
All the sides of quadrilateral are equal.
So now we will check the lengths of the diagonals.
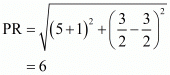
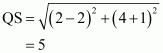
All the sides are equal but the diagonals are unequal. Hence ABCD is a rhombus.
Question 38: Points P, Q, R and S divides the line segment joining A(1, 2) and B(6, 7) in 5 equal parts. Find the coordinates of the points P, Q and R.
Answer :
It is given that P, Q, R and S divides the line segment joining A(1, 2) and B(6, 7) in 5 equal parts.
∴ AP = PQ = QR = RS = SB .....(1)
Now,
AP + PQ + QR + RS + SB = AB
⇒ AP + AP + AP + AP + AP = AB [From (1)]
⇒ 5AP = AB
⇒ AP = 1/5 AB .....(2)
Now,
PB = PQ + QR + RS + SB =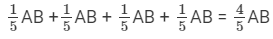
.....(3)
From (2) and (3), we get
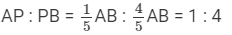
Similarly,
AQ : QB = 2 : 3 and AR : RB = 3 : 2
Using section formula, we get
Coordinates of P =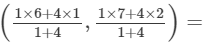 
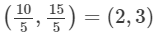
Coordinates of Q =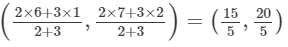 
= (3 , 4)
Coordinates of R = 
= (4 , 5)
Question 39: If A and B are two points having coordinates (−2, −2) and (2, −4) respectively, find the coordinates of P such that AP = 3/7 AB.
Answer :
We have two points A (−2,−2) and B (2,−4). Let P be any point which divide AB as,
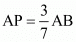
Since,
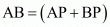
So,
Now according to the section formula if any point P divides a line segment joining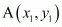 
and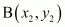
in the ratio m: n internally than,
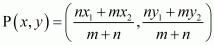
Therefore P divides AB in the ratio 3: 4. So,
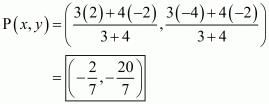
Question 40: Find the coordinates of the points which divide the line segment joining A(−2, 2) and B (2, 8) into four equal parts.
Answer :
The co-ordinates of the midpoint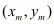 
between two points 
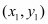and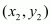 
is given by,
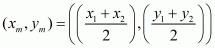
Here we are supposed to find the points which divide the line joining A(−2,2) and B(2,8) into 4 equal parts.
We shall first find the midpoint M(x, y) of these two points since this point will divide the line into two equal parts.
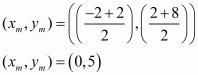
So the point M(0,5) splits this line into two equal parts.
Now, we need to find the midpoint of A(−2,2) and M(0,5) separately and the midpoint of B(2,8) and M(0,5). These two points along with M(0,5) split the line joining the original two points into four equal parts.
Let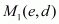 
be the midpoint of A(−2,2) and M(0,5).
Now let 
bet the midpoint of B(2,8) and M(0,5).
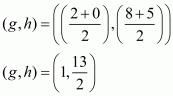
Hence the co-ordinates of the points which divide the line joining the two given points are
.
Question 41: Three consecutive vertices of a parallelogram are (−2,−1), (1, 0) and (4, 3). Find the fourth vertex.
Answer :
Let ABCD be a parallelogram in which the co-ordinates of the vertices are A (−2,−1); B (1, 0) and C (4, 3). We have to find the co-ordinates of the forth vertex.
Let the forth vertex be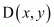
Since ABCD is a parallelogram, the diagonals bisect each other. Therefore the mid-point of the diagonals of the parallelogram will coincide.
Now to find the mid-point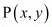
of two points
and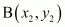
we use section formula as,
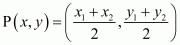
The mid-point of the diagonals of the parallelogram will coincide.
So,
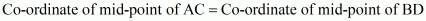
Therefore,
Now equate the individual terms to get the unknown value. So,
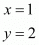
So the forth vertex is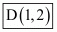 
Question 42:
The points (3, −4) and (−6, 2) are the extremities of a diagonal of a parallelogram. If the third vertex is (−1,−3). Find the coordinates of the fourth vertex.
Answer :
Let ABCD be a parallelogram in which the co-ordinates of the vertices are A (3,−4); B (−1,−3) and C (−6, 2). We have to find the co-ordinates of the forth vertex.
Let the forth vertex be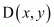
Since ABCD is a parallelogram, the diagonals bisect each other. Therefore the mid-point of the diagonals of the parallelogram will coincide.
Now to find the mid-point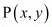
of two points
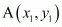and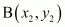
we use section formula as,
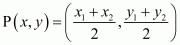
The mid-point of the diagonals of the parallelogram will coincide.
So,
Therefore,
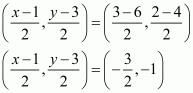
Now equate the individual terms to get the unknown value. So,
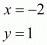
So the forth vertex is 
Question 43: If the coordinates of the mid-points of the sides of a triangle are (1, 1) (2, −3) and (3, 4), find the vertices of the triangle.
Answer :
The co-ordinates of the midpoint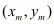 
between two points 
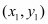and 
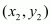is given by,
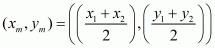
Let the three vertices of the triangle be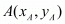
, 
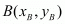and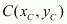
.
The three midpoints are given. Let these points be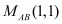
, 
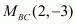and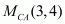
.
Let us now equate these points using the earlier mentioned formula,
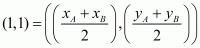
Equating the individual components we get,
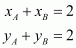
Using the midpoint of another side we have,
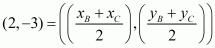
Equating the individual components we get,
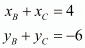
Using the midpoint of the last side we have,
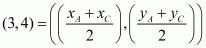
Equating the individual components we get,
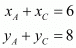
Adding up all the three equations which have variable ‘x’ alone we have,
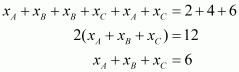
Substituting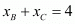 
in the above equation we have,
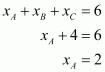
Therefore,
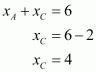
And
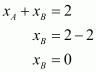
Adding up all the three equations which have variable ‘y’ alone we have,
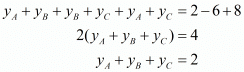
Substituting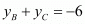 
in the above equation we have,
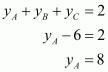
Therefore,
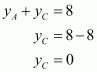
And
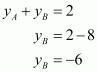
Therefore the co-ordinates of the three vertices of the triangle are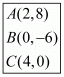
.
Question 44: Determine the ratio in which the straight line x − y − 2 = 0 divides the line segment joining (3, −1) and (8, 9).
Answer :
Let the line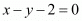
divide the line segment joining the points A (3,−1) and B (8, 9) in the ratio 
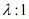at any point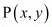 
Now according to the section formula if point a point P divides a line segment joining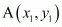 
and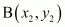
in the ratio m: n internally than,
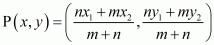
So,
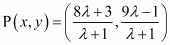
Since, P lies on the given line. So,
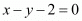
Put the values of co-ordinates of point P in the equation of line to get,
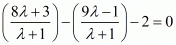
On further simplification we get,
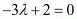
So,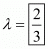 
So the line divides the line segment joining A and B in the ratio 2: 3 internally.
Question 45: Three vertices of a parallelogram are (a+b, a−b), (2a+b, 2a−b), (a−b, a+b). Find the fourth vertex.
Answer :
Let ABCD be a parallelogram in which the co-ordinates of the vertices are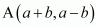
;
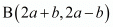and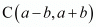
. We have to find the co-ordinates of the forth vertex.
Let the forth vertex be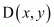
Since ABCD is a parallelogram, the diagonals bisect each other. Therefore the mid-point of the diagonals of the parallelogram will coincide.
In general to find the mid-point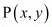
of two points
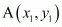and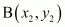
we use section formula as,
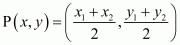
The mid-point of the diagonals of the parallelogram will coincide.
So,
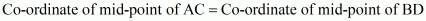
Therefore,
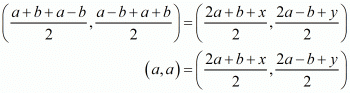
Now equate the individual terms to get the unknown value. So,
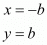
So the forth vertex is 
Question 46: If two vertices of a parallelogram are (3, 2) (−1, 0) and the diagonals cut at (2, −5), find the other vertices of the parallelogram.
Answer :
We have a parallelogram ABCD in which A (3, 2) and B (−1, 0) and the co-ordinate of the intersection of diagonals is M (2,−5).
We have to find the co-ordinates of vertices C and D.
So let the co-ordinates be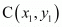
and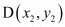
In general to find the mid-point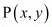
of two points
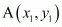and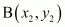
we use section formula as,
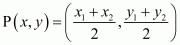
The mid-point of the diagonals of the parallelogram will coincide.
So,
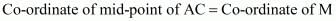
Therefore,
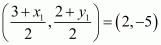
Now equate the individual terms to get the unknown value. So,
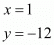
So the co-ordinate of vertex C is (1,−12) 
Similarly,
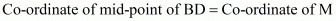
Therefore,
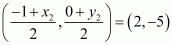
Now equate the individual terms to get the unknown value. So,
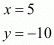
So the co-ordinate of vertex C is (5,−10)
Question 47: If the coordinates of the mid-points of the sides of a triangle are (3, 4) (4, 6) and (5, 7), find its vertices.
Answer :
The co-ordinates of the midpoint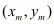 
between two points 
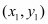and 
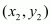is given by,
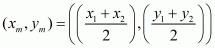
Let the three vertices of the triangle be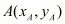
, 
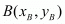and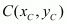
.
The three midpoints are given. Let these points be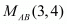
, 
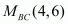and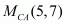
.
Let us now equate these points using the earlier mentioned formula,
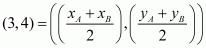
Equating the individual components we get,
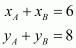
Using the midpoint of another side we have,
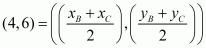
Equating the individual components we get,
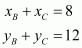
Using the midpoint of the last side we have,
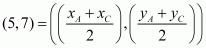
Equating the individual components we get,
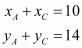
Adding up all the three equations which have variable ‘x’ alone we have,
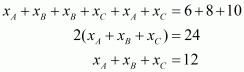
Substituting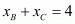 
in the above equation we have,
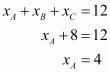
Therefore,
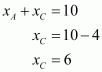
And
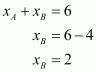
Adding up all the three equations which have variable ‘y’ alone we have,
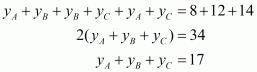
Substituting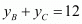 
in the above equation we have,
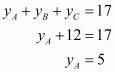
Therefore,
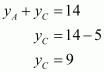
And
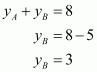
Therefore the co-ordinates of the three vertices of the triangle are
.
Question 48: The line segment joining the points P(3, 3) and Q(6, −6) is trisected at the points A and B such that A is nearer to P. If A also lies on the line given by 2x + y + k = 0, find the value of k.
Answer :
We have two points P (3, 3) and Q (6,−6). There are two points A and B which trisect the line segment joining P and Q.
Let the co-ordinate of A be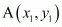
Now according to the section formula if any point P divides a line segment joining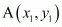 
and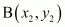
in the ratio m: n internally than,
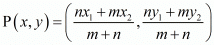
The point A is the point of trisection of the line segment PQ. So, A divides PQ in the ratio 1: 2
Now we will use section formula to find the co-ordinates of unknown point A as,
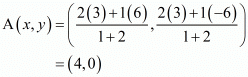
Therefore, co-ordinates of point A is(4, 0)
It is given that point A lies on the line whose equation is
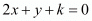
So point A will satisfy this equation.
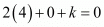
So,
Question 49: If three consecutive vertices of a parallelogram are (1, −2), (3, 6) and (5, 10), find its fourth vertex.
Answer :
Let ABCD be a parallelogram in which the co-ordinates of the vertices are A (1,−2);
B (3, 6) and C(5, 10). We have to find the co-ordinates of the forth vertex.
Let the forth vertex be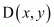
Since ABCD is a parallelogram, the diagonals bisect each other. Therefore the mid-point of the diagonals of the parallelogram will coincide.
Now to find the mid-point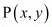
of two points
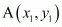and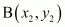
we use section formula as,
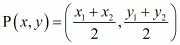
The mid-point of the diagonals of the parallelogram will coincide.
So,
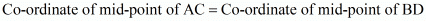
Therefore,
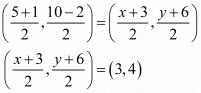
Now equate the individual terms to get the unknown value. So,
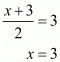
Similarly,
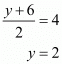
So the forth vertex is 
Question 50: If the points A (a, −11), B (5, b), C(2, 15) and D (1, 1) are the vertices of a parallelogram ABCD, find the values of a and b.
Answer :
Let ABCD be a parallelogram in which the co-ordinates of the vertices are A (a,−11); B (5, b); C (2, 15) and D (1, 1).
Since ABCD is a parallelogram, the diagonals bisect each other. Therefore the mid-point of the diagonals of the parallelogram will coincide.
In general to find the mid-point
of two points
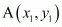and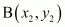
we use section formula as,
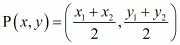
The mid-point of the diagonals of the parallelogram will coincide.
So,
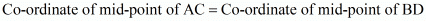
Therefore,
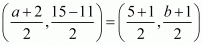
Now equate the individual terms to get the unknown value. So,
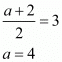
Similarly,
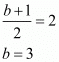
Therefore,
|
5 videos|292 docs|59 tests
|
|
Nov 19, 2024 Last updated |
|
5 videos|292 docs|59 tests
|
|
Explore Courses for Class 10 exam
|
|
past year papers
,MCQs
,Videos & Tests for Class 10
,Videos & Tests for Class 10
,Important questions
,Sample Paper
,practice quizzes
,Coordinate Geometry Exercise 14.1 (Part-8) | Extra Documents
,Extra Questions
,Videos & Tests for Class 10
,Objective type Questions
,study material
,ppt
,Coordinate Geometry Exercise 14.1 (Part-8) | Extra Documents
,Free
,Coordinate Geometry Exercise 14.1 (Part-8) | Extra Documents
,mock tests for examination
,Summary
,Previous Year Questions with Solutions
,Semester Notes
,shortcuts and tricks
,video lectures
,Exam
,Viva Questions
;
Coordinate Geometry Exercise 14.1 (Part-8) Free PDF Download
Importance of Coordinate Geometry Exercise 14.1 (Part-8)
Coordinate Geometry Exercise 14.1 (Part-8) Notes
Coordinate Geometry Exercise 14.1 (Part-8) Class 10 Questions
Study Coordinate Geometry Exercise 14.1 (Part-8) on the App
|
© EduRev
|
Education Revolution
|
Follow Us
|
