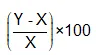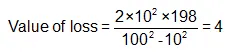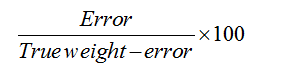Basic Concepts: Profit, Loss & Discount | General Test Preparation for CUET UG - CUET Commerce PDF Download
Introduction
- The Profit and Loss formula is used in mathematics to determine the price of a commodity in the market and to understand how profitable a business is.
- Every product has a cost price and a selling price.
- Based on these prices, we calculate the profit gained or the loss incurred on a particular product.
- Problems typically involve applying percentage concepts, successive changes, and sometimes tricky cases like dishonest dealers.
Basic Terms
1. Cost Price (CP)
The amount paid by a vendor to purchase a product or commodity is called the cost price, denoted as CP.
This cost price is further classified into two categories:
- Fixed Cost: The fixed cost remains constant and does not vary with the quantity of production or sales.
- Variable Cost: This cost varies depending on the number of units produced or sold.
2. Selling Price (SP)
The amount for which a product is sold is called the selling price, denoted as SP. It is also called the sale price.
3. Profit and Loss
Profit: When an article is sold for more than its cost price, the difference is called profit or gain.
- Profit or Gain = S.P - C.P
Loss: When an article is sold for less than its cost price, the difference is called loss.
- Loss = C.P - S.P
The formula for the profit and loss percentage is:
- Profit percentage = (Profit / Cost Price) x 100
- Loss percentage = (Loss / Cost price) x 100
Important: If the selling price of X articles is equal to the cost price of Y articles, then the net profit percentage is given by
Example 1: Brijesh purchased a book for Rs.1260 and sold it to Rakesh for Rs.1320. Rakesh sold it to Kishore for Rs.1400. Who gained more and by how much?
Sol: For Brijesh:
Cost Price = Rs. 1260;
Selling Price = Rs. 1320.
Profit = Rs. 1320 − Rs. 1260 = Rs. 60.
For Rakesh:
Cost Price = Rs. 1320;
Selling Price = Rs. 1400.
Profit = Rs. 1400 − Rs. 1320 = Rs. 80.
Rakesh gained more by Rs. 80 − Rs. 60 = Rs. 20.
Therefore, Rakesh gained Rs. 20 more than Brijesh.
Example 2: If the selling price of 10 articles is the same as the cost price of 11 articles, find the profit or loss percent.
Sol:
Let the cost price of 1 article be Re. 1.
Cost Price of 10 articles = Rs. 10.
Cost Price of 11 articles = Rs. 11.
Hence, Selling Price of 10 articles = Rs. 11.
Profit = Selling Price − Cost Price = Rs. 11 − Rs. 10 = Rs. 1.
Profit percentage = (Profit / Cost Price) × 100 = (1/10) × 100 = 10%.Shortcut:
Here X = 10 and Y = 11, hence profit percentage = ((Y − X) / X) × 100 = 10%.Important: Profit and loss percentage are always calculated with cost price as the base.
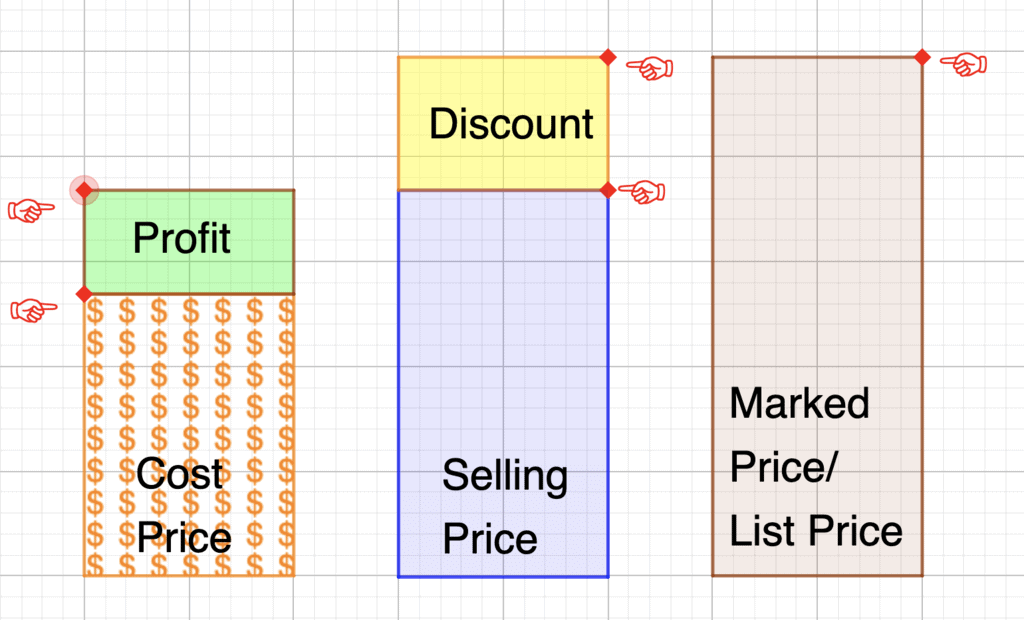
4. Marked Price (MP)
The price at which an item is marked for sale is called the marked price, denoted as MP.
Markup: The difference between the cost price of a product and its selling price, typically expressed as a percentage or a fixed amount. It is the extra amount added to cost price to ensure a profit.
- Marked Price = Cost Price + Markup
- Marked Price = Cost Price + % Markup on Cost Price
Example: If a shop buys a product for ₹50 and sells it for ₹70, the markup is ₹70 − ₹50 = ₹20.
Markup percentage = (20 / 50) × 100 = 40%.
5. Discount
Discounts are reductions in the marked price made by the seller. In case of no discount, SP=MP. However, if a discount is offered, then SP<MP.
- Discount = Marked Price – Selling Price
- Discount Percentage = (Discount/Marked price) x 100
Types of Costs
Business costs are broadly classified into three types:
- Direct / Variable Costs: Costs that vary directly with the number of units produced or sold (e.g., raw materials, piece-rate wages).
- Indirect / Fixed Costs: Costs that remain constant regardless of the level of production or sales (e.g., rent, salaries).
- Semi-Variable Costs: Costs that remain fixed up to a certain level of activity but increase beyond that level (e.g., extra rent on larger premises).
Margin and Break-Even Point
- Margin (Contribution): This is the difference between the selling price of a product and its variable cost (the cost directly linked to making or buying that item). This margin first goes towards covering the fixed costs of the business (like rent or salaries).
Break-Even Point (BEP): This is the level of sales where the business neither makes a profit nor suffers a loss — total revenue equals total costs. Once sales cross this point, every extra unit sold adds to profit.
Formula = Break-even sales (in units)=Margin per unitFixed Costs
Example: Suppose a shop has fixed costs of ₹5000 per month. It sells pens at ₹5 each, while the variable cost per pen is ₹2.5. So, the margin per pen = ₹2.5. The shop needs to sell 5000÷2.5=2000 pens to break even. Selling more than 2000 pens gives a profit; selling fewer means a loss.
Formulae to Remember
- Profit = (Actual Sales - Break Even Sales) x Contribution per unit
- Loss = (Break Even Sales -- Actual Sales) x Contribution per unit
Profit and Loss Tricks
Here are some useful formulas and tricks for solving profit and loss problems:
- SP = {(100 + P%) / 100} × CP
- SP = {(100 − L%) / 100} × CP
- CP = {100 / (100 + P%)} × SP
- CP = {100 / (100 − L%)} × SP
- SP = MP − Discount
- For false weight, profit percentage, P% = ((True weight − False weight) / False weight) × 100.
- When there are two successive profits m% and n%, the net profit percentage = (m + n + mn/100).
- When the profit is m% and loss is n%, net profit or loss percentage = (m − n − mn/100).
- If a product is sold first at m% profit and then at n% profit, the actual cost price is CP = [100 × 100 × P / (100 + m)(100 + n)].
In case of losses, CP = [100 × 100 × P / (100 − m)(100 − n)]. - If profit percentage and loss percentage are equal (P = L), then % loss = P² / 100.
The Concept of Same Selling Price with Profit or Loss
If two items are sold at the same price, and one gives a profit of x% while the other gives a loss of x%, the overall result is always a loss.
Example:
- Item A cost ₹100, sold at 20% profit → Selling Price = ₹120
- Item B cost ₹150, sold at 20% loss → Selling Price = ₹120
Total Cost Price = ₹250, Total Selling Price = ₹240 → Net Loss = ₹10
Hence, in such cases, the selling price is immaterial. There is always a loss.
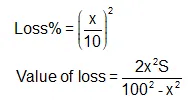
Example: Two articles are sold at Rs. 198 each, such that a profit of 10% is made on the first while a loss of 10% is incurred on the other. What would be the net profit/loss on the two transactions combined?
Sol:
Article 1: Profit = 10%, Selling Price = Rs. 198.
Cost Price = 198 / 1.1 = Rs. 180.
Article 2: Loss = 10%, Selling Price = Rs. 198.
Cost Price = 198 / 0.9 = Rs. 220.
Total Cost Price = 180 + 220 = Rs. 400.
Total Selling Price = 2 × 198 = Rs. 396.
Net loss = Rs. 400 − Rs. 396 = Rs. 4.
Shortcut:
Specific Cases and Rules
- False Weight Calculation
If a product is sold at a false weight, the profit percentage is calculated based on the difference between the true weight and the false weight:
- Net Percentage Profit when there are two successive profits
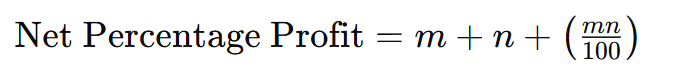
Where and are the percentages of the two successive profits. - Net Percentage Profit or Loss when there's a profit and a loss
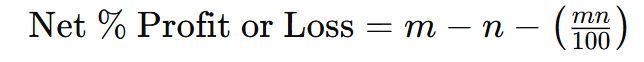
Where is the profit percentage and is the loss percentage. Effective Cost Price Calculation after successive profits
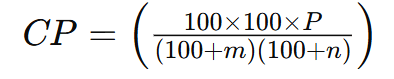
Where is the final selling price after two successive profits.Question for Basic Concepts: Profit, Loss & DiscountTry yourself:A shopkeeper bought a bag for Rs. 800 and sold it for Rs 1000. What is the profit percentage earned by the shopkeeper?View Solution
Dishonest Dealer
- A dishonest dealer sells his product, claiming to benefit the customer, but either alters the weight or marks up the price excessively and offers discounts to attract buyers.
- Let us assume that the shopkeeper gains a G % when selling his product.
Therefore,
(100+G)/(100+x) = True weight/ False weight
Here,
G = Overall gain percentage
X = %loss or % gain - Also, another formula that you can remember, in case the shopkeeper sells at the cost price, is:
Gain Percentage =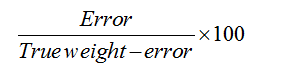
Example: Let a dishonest shopkeeper sell sugar at Rs 18/kg, which he has bought at Rs 15/kg and he is giving 800gm instead of 1000gm. Find his actual profit percentage.
Sol:
Cost price of sugar = Rs. 15/kg
Selling price = Rs. 18/kg
Profit per kg = Rs. 18 − Rs. 15 = Rs. 3
Profit percentage without weighing false weight = (3/15) × 100 = 20%.
However, the shopkeeper actually sells 800 gm instead of 1 kg.
Profit due to false weight == (200/800)x100 = 25%
The overall profit percentage = {P + Q + (PQ/100)} = [20+25+{(20 x 25)/100}] = 50%Alternate method:
Cost price of 1 kg sugar = Rs. 15.
Since shopkeeper sells only 800 gm, cost price of 800 gm = Rs. 12.
He sells 800 gm sugar for Rs. 18, so profit = 18 − 12 = Rs. 6.
Profit percentage = (6 / 12) × 100 = 50%.Both methods give the same answer, but the first is simpler using the formula P + Q + (PQ/100).
Note: This formula is also valid if the shopkeeper incurs losses; then, loss percentages are input as negative values.
Profit Calculation based on Amount Spent and Amount Earned
Usually, profit or loss is calculated by comparing the money spent (cost price) and the money earned (selling price) when the number of items bought and sold is the same.
Sometimes, the seller recovers all money spent before selling all items; in such cases, the unsold items are considered profit.
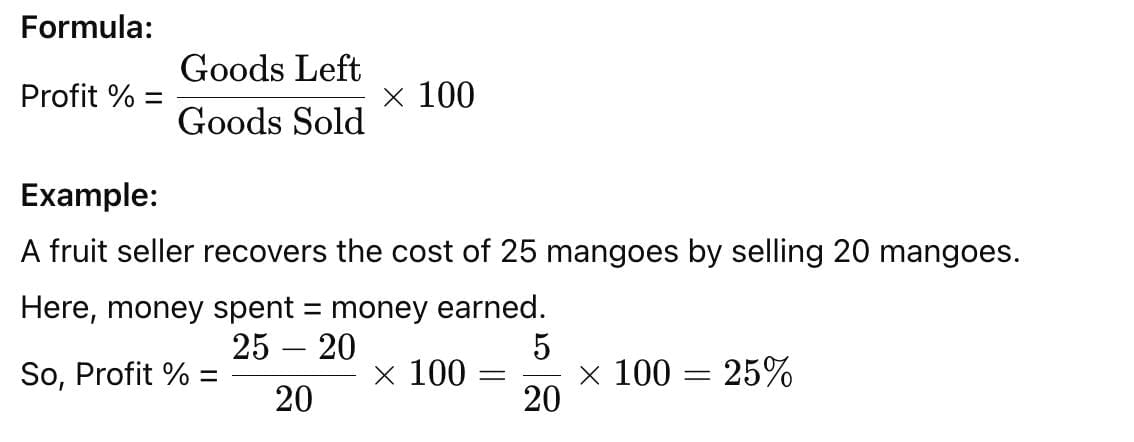
Solved Problems
To understand the concept, let's first open a shop, say a local retail stationery shop.
Example 1: Now, suppose a student shows up and wants to buy 5 gel pens worth ₹ 5 each. The cost price of one pen is ₹ 4. So, what is the profit earned by the shopkeeper in net terms and its profit percentage?
Sol:
Selling Price (SP) = ₹5 per pen.
Cost Price (CP) = ₹4 per pen.
Profit per pen = SP − CP = ₹5 − ₹4 = ₹1.
Therefore, profit percentage = (Profit / CP) × 100Total profit on 5 pens = 5 × ₹1 = ₹5.
Example 2: Suppose another customer comes to the shop and buys 2 registers worth ₹ 50 each and a pencil box from him. And this time the shopkeeper has earned 40% profit on the registers. He earned a profit of ₹10 on the pencil box, and the profit % on the pencil box is 20%. Then what is the cost price and profit on the register, and the selling price and cost price of the pencil box?
Sol: In this case, we are given S.P. = ₹ 50 for each register and profit% = 40%. Let C.P. be x.
⇒ Profit % = {(50 – x)/x} * 100
⇒ 40 = {(50 – x)/x} * 100
⇒ 4x = 500 – 10x
⇒ 14x = 500
⇒ x = 35.71
⇒ Profit = 50 – 35.71 = 14.29If profit is 40% on selling price:
⇒ Profit = profit% * S.P = 0.4* 50 = ₹20
C.P. = S.P. – Profit = ₹50 – ₹20 = ₹30Let's now move on to calculate S.P. and C.P. of pencil box. Let C.P. of pencil box be y
∵ Profit % = (profit/ Cost price) *100
⇒ 20 = (10/y) *100
⇒ 2y = 100
⇒ y = ₹ 50
Therefore, S.P. = ₹50 + ₹10 = ₹60
Example 3: Since not many customers showed up on the first day of the shop. Therefore, to popularise the shop, the shopkeeper puts up a discount of 20% on all the products. The first customer showed up and bought a packet of pencils and 3 erasers, still making up a profit of 30% on both items. Then what is the actual cost price of both the items when the pencil is marked as ₹30 and the eraser ₹ 5 each?
What does the underlined marked mean?
Here marked means Marked Price is the price that is offered to customer before discount basically, discount is just difference between marked price and Selling price i.e. Discount = M.P. – S.P.
Sol: In this case, Discount = 0.2 * Price of packet of pencil = 0.2 *30 = ₹ 6
Therefore, S.P = 30 – 6 = ₹ 24
∵ Profit is 30% on C.P., assume C.P. be x
⇒ 0.3 = (24 – x)/x
⇒ 0.3x = 24 – x
⇒ 1.3x = 24
⇒ x = 18.46
Similarly,
Discount = 0.2 * Price of 3 erasers = 0.2* 3*5 = ₹ 3
Hence, S.P. = (3*5) - 3 = ₹ 12
∵ Profit is 30% on C.P., assume C.P. be y
⇒ 0.3 = (12 – y)/y
⇒ 1.3y = 12
⇒ y (C.P. of 3 eraser) = 9.23
Example 4: Consider this situation: The stationery sold a Parker pen at a loss of 20% for ₹ 100 and a pack of colored sketch pens at a loss of 15% on S.P. What are the cost price and selling price of both the articles?
Sol: First, let's find out the C.P. and S.P. parker pen,
∵ Loss is 20% on C.P.
Let C.P. be x and S.P. be 100
⇒ S.P. = 0.8 of C.P.
⇒ 100 = 0.8x
⇒ 100/0.8 = x
⇒ 125 = x
For sketch pens,
∵ Loss is 15% on S.P.
Let S.P. be y and C.P. be 100
⇒ Loss = 0.15y
⇒ C.P. = 1.15 y
⇒ 100 = 1.15y
⇒ 100/1.15 = y
⇒ y = ₹ 87
Example 5: A watch dealer incurs an expense of Rs. 150 for producing every watch. He also incurs an additional expenditure of Rs. 30,000, which is independent of the number of watches produced. If he is able to sell a watch during the season, he sells it for Rs. 250. If he fails to do so, he has to sell each watch for Rs. 100. If he is able to sell only 1,200 out of 1,500 watches he has made in the season, then he has made a profit of:
(a) ₹ 90000
(b) ₹ 75000
(c) ₹ 45000
(d) ₹ 60000
[CAT 2016]
Sol: Here, first find out cost he has incurred to produce the watches.
∵ He made 1500 watches costing ₹ 150 each and an additional ₹ 30000 expense on them.
⇒ Total Cost = (1500*150) + 30000 = ₹ 255000
∵ He's able to sell 1200 watches in the season = ₹250 each
So, the revenue earned by him during the season = ₹250 * 1200 = ₹ 300000
Also, the leftover 300 pieces of clocks would have been sold by the watchmaker in off-season = 100 each.
Revenue earned through these 300 watches = 300*100 = ₹ 30000
Total Revenue = ₹ 300000 + ₹30000 = ₹330000
Profit = Revenue - Cost = 330000 - 255000 = ₹ 75000
Example 6: Instead of a meter scale, a cloth merchant uses a 120cm scale while buying, but uses an 80cm scale while selling the same cloth. If he offers a discount of 20% on cash payment, what is his overall profit percentage?
Sol: This question above is a special one with the faulty dealer. Here, the dealer is earning profit by using a false scale.
Assume price of cloth = ₹1/cm.
Cost Price per cm when buying with 120 cm scale:
CP = (100 / 120) × ₹1 = ₹0.8333/cm.
∵ This merchant again uses faulty scale to sell the cloth to his customers. He uses a scale that measures 80cm as 100cm i.e. he sells 80cm for ₹100
Now he also gives a discount of 20% on the cloth.
⇒ His mark up price is ₹100/80cm
∵ S.P. = M.P. - M.P. * Discount% = M.P. (1 - Discount%) = (100/80)*(80/100) = ₹1/cm
Therefore, his profit % = (1 – 0.8333)/0.8333 *100 = 20%
|
164 videos|798 docs|1153 tests
|
FAQs on Basic Concepts: Profit, Loss & Discount - General Test Preparation for CUET UG - CUET Commerce
| 1. What are the basic terms related to profit, loss, and discount in financial calculations? |  |
| 2. How do you calculate the margin and break-even point in a business? |  |
| 3. What is the significance of the same selling price with profit or loss in business transactions? |  |
| 4. How can one identify a dishonest dealer in terms of profit calculations? |  |
| 5. How do you calculate profit based on the amount spent and amount earned? |  |