Mensuration: Volume, Surface Area & Solid Figures | CSAT Preparation - UPSC PDF Download
What is Mensuration?
Mensuration is a branch of mathematics that deals with the measurement of length, area, and volume of geometric figures. These shapes exist in 2 dimensions or 3 dimensions. Mensuration deals with the measurement of 3D solids in terms of total surface area, lateral/curved surface area, and volume.
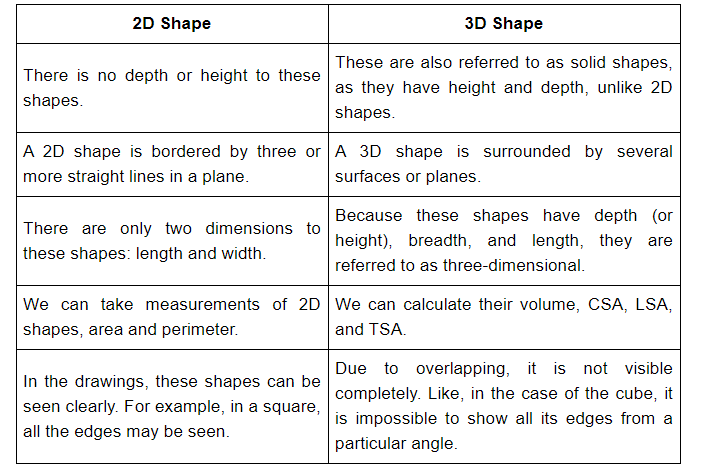
2D and 3D Shapes
Important Terms in Mensuration
- Area (A): The surface covered by a closed shape, measured in square units.
- Perimeter (P): The total length of the boundary of a 2D shape, measured in linear units.
- Volume (V): The space occupied by a 3D object, measured in cubic units.
- Curved Surface Area (CSA): The area of the curved part of a shape, excluding its base and top.
- Lateral Surface Area (LSA): The total area of all the sides of a shape, excluding the base and top.
- Total Surface Area (TSA): The sum of all surface areas of a closed shape.
- Square Unit: The area of a square with sides of one unit length, used for measuring surface area.
- Cubic Unit: The volume of a cube with sides of one unit length, used for measuring volume.
Tools Used for Mensuration
- Caliper: Measures diameters.
- Try Square: Checks squareness and flatness of surfaces.
- Meter Stick: A one-metre long measuring tool.
- Compass: Draws arcs and circles.
Some Important Formulas of Mensuration
| SOLID | Total Surface Area | Lateral/ Curved Surface area | Volume | Length of Leading Diagonal/ Slant Height |
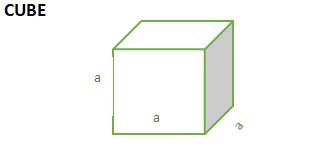 | 6a2 | 4a2 | a3 | √3a |
 | 2(LB+ BH+ HL) | 2H (L + B) | LBH | √(L2 + H2 + B2) |
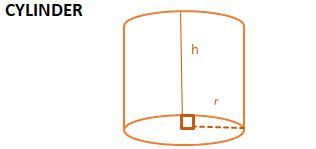 | 2Πr (r + h) | 2Πrh | Πr2h | No Slant height or diagonal |
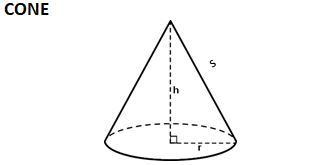 | Πr (r + l) | Πrl | ⅓Πr2h | √(h2 + r2) |
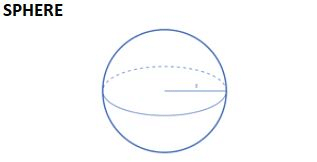 | 4Πr2 | 4Πr2 | 4/3Πr3 | No Slant height or diagonal |
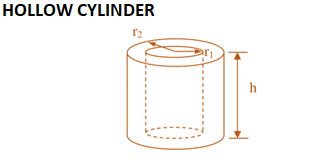 | 2Π(r1 + r2) (r2 - r1 + h) | 2Πh(r1 + r2) | Πh(r22-r12) | No Slant height or diagonal |
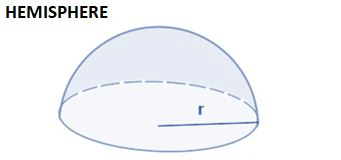 | 3Πr2 | 2Πr2 | 2/3Πr3 | No Slant height or diagonal |
Prism
A prism is a 3D shape with a polygon for a base, and both ends of the prism have the same shape. The sides of the prism are parallelograms, and the cross-section remains the same along its length.
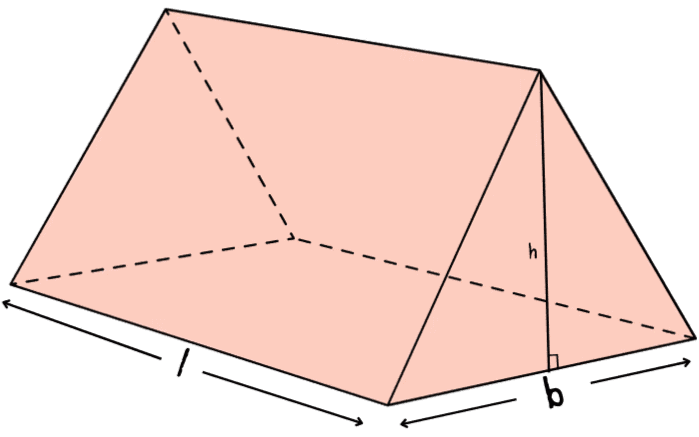
- Overview: A prism is a three-dimensional geometric shape with a polygonal base. The base is identical at both ends, and the sides are parallelograms, ensuring a consistent cross-section along the length of the prism.
- Base and Side Faces: The number of side faces on a prism corresponds to the number of sides in the polygonal base. For instance, if the base is triangular, the prism will have three side faces. Similarly, if the base is a quadrilateral, the prism will have four side faces, and so on.
- Right Prism: A prism is termed a right prism when its side edges are perpendicular to the bases. Examples of right prisms include rectangular prisms, commonly known as cuboids, and cubes.
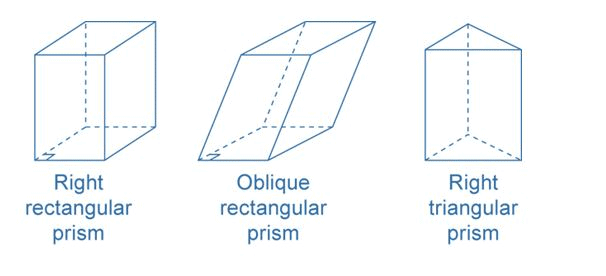
Mensuration of Prism
- Lateral Surface Area of a Prism: This is calculated by multiplying the perimeter of the base by the height of the prism.
- Total Surface Area of a Prism: This includes the lateral surface area plus twice the area of the base.
- Volume of a Prism: The volume is found by multiplying the area of the base by the height of the prism.
Pyramids
A pyramid is a three-dimensional shape formed by connecting a flat polygonal base to a single point called the apex. The sides of the pyramid are triangular in shape.
- When we think of "pyramid," we often picture the famous pyramids in Egypt, which are known for their square bases.
- A pyramid with an n-sided base has n + 1 vertices, n + 1 faces, and 2n edges.
- In a right pyramid, the apex is positioned directly above the centre of the base, creating a symmetrical shape.
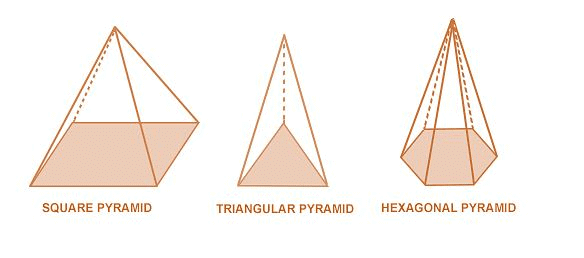
Mensuration of Pyramid
- Lateral surface area of Pyramid: (perimeter / 2) × slant height
- Total surface area of Pyramid: curved surface area + area of the base
- Volume of the Pyramid: Base area × (⅓) × height
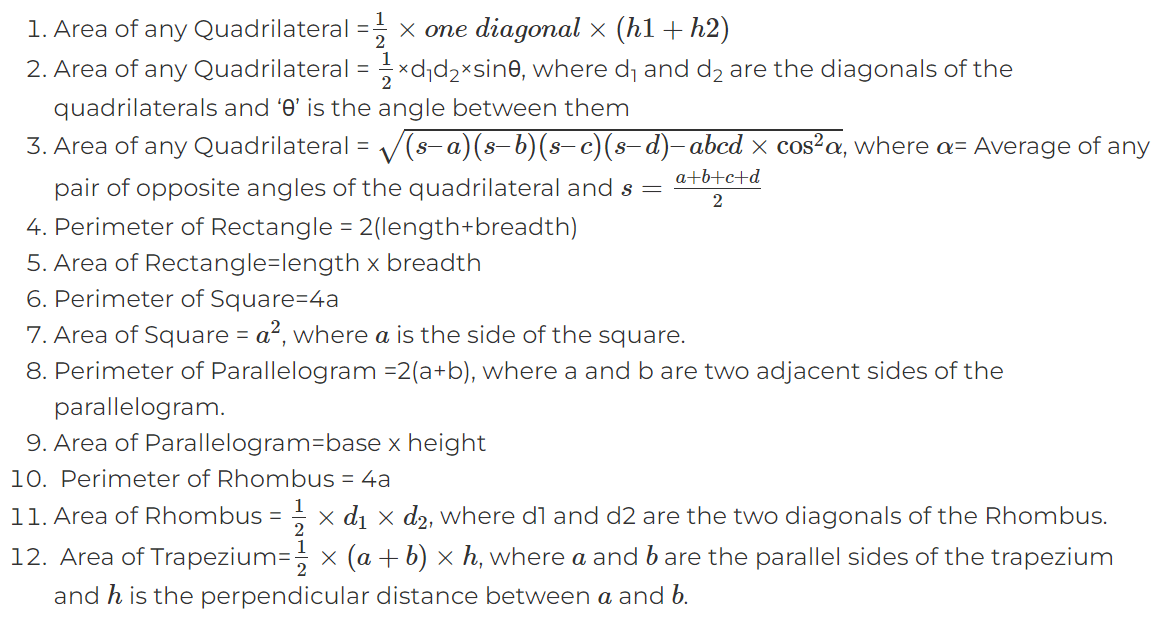
Quadrilateral
To find the area of any quadrilateral, we can divide it into two triangles and then calculate the area of both triangles separately.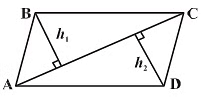
- Area of ABCD = Area of ∆ABC + Area of ∆ACD
= (1/2) × AC × h1 + (1/2) × AC × h2
Area of Quadrilateral
Area of Quadrilateral = (1/2) (h1 + h2) d
Where h1 and h2 are the heights of both triangles, and d is the length of the common diagonal, i.e., AC.
Rhombus
A rhombus is a quadrilateral with all sides equal and parallel but not necessarily right angled. Its two diagonals are perpendicular bisectors of each other.
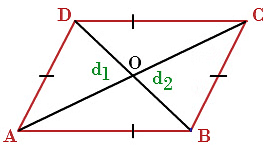
Here, we can also split the rhombus into two triangles and find the area of the rhombus easily.
Area of Rhombus
Area of a rhombus is half the product of its two diagonals.
Mensuration of Quadrilaterals
Circles
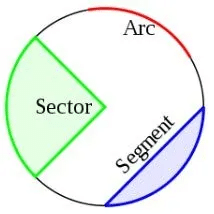
- Circumference of the circle = 2πr
- Area of the circle = πr2
- Length of arc =
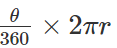
- Area of Sector =
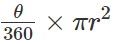
- Area of Segment =
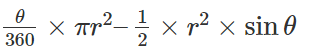
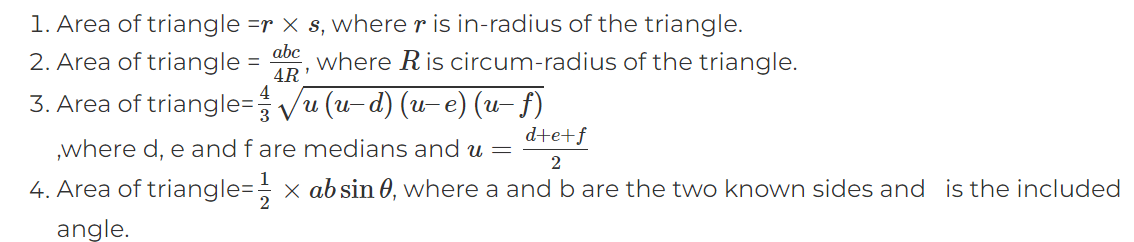
Triangles
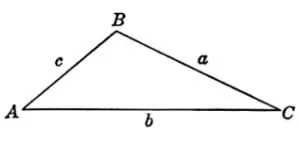
- Formula of Perimeter of triangle (P) = a + b + c
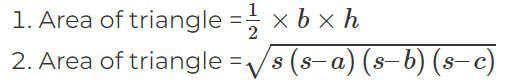
- Some advanced mensuration formulas for area of triangle are:
Polygons

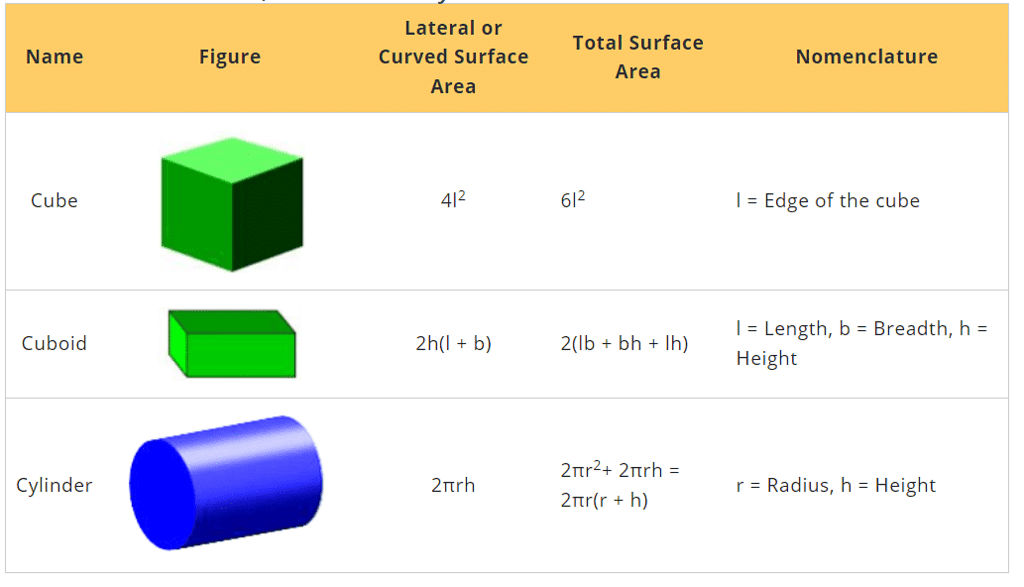
Cuboid, Cube and Cylinder
- Surface Area
If we draw the net of the solid shape, then we can see all the faces clearly. Adding the areas of all the faces gives the total surface area of that solid shape. The unit of surface area is a square unit. - Lateral or Curved Surface Area
If we exclude the top and bottom faces of the solid shape, the area of the remaining part is the lateral surface area of the shape. The unit of lateral surface area is a square unit.
- Volume
Volume is the space occupied by any solid figure, i.e., the amount of capacity to contain something. The unit of volume is a cubic unit.
Cone
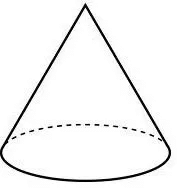
- Curved Surface Area of Cone = πrl, where l = slant height
- Total Surface Area of Cone = πr(r + l)
- Volume of Cone = (1/3)πr2h
Sphere 
- Total Surface Area of Sphere = 4πr2
- Volume of Sphere = (4/3) × πr3
Hemisphere
- Curved Surface Area of Hemisphere = 2πr2
- Total Surface Area of Hemisphere = 3πr2
- Volume of Hemisphere = (2/3) × πr3
Practice Questions
Problem 1: The length of a garden is thrice its breadth. A playground measuring 180 sq. m occupies 1/15th of the total area of the garden. The length of the garden is
- 60 m
- 30 m
- 90 m
- 50 m
Sol: Correct Answer is Option 3
Let L be the length and B be the breadth of the garden. We have L = 3B.
Total area of the garden = 180 × 15 = 2700 sq m. ⇒ LB = 2700
⇒ 3B² = 2700 ⇒ B² = 900 ⇒ B = 30 m
Hence, the length of the garden = 30 × 3 = 90 m.
Problem 2: The perimeter of an equilateral triangle is 96√3 cm. Find its height.
- 32 cm
- 48 cm
- 16 cm
- 64 cm
- 24 cm
Sol: Correct Answer is Option 2
Perimeter of the equilateral triangle is 96√3 cm.
Each side of the equilateral triangle is 96√3 / 3 = 32√3 cm.
The height of the equilateral triangle will be = (√3 / 2) × 32√3 = 48 cm.
Problem 3: If the ratio of radius of two spheres is 4:7, the ratio of their volume is
- 4 : 7
- 64 : 343
- 49 : 16
- 16 : 49
- None of these
Sol: Correct Answer is Option 2
The ratio of radii of 2 spheres is 4:7.
Therefore, the ratio of their volumes = (4³) : (7³) = 64 : 343.
Problem 4: The diameter (in metre) of a sphere whose volume is 268 (4/21) m³ is
- 6
- 12
- 8
- 24
- 16
Sol: Correct Answer is Option 3
Volume of the sphere = 268 (4/21) = 5632 / 21 m³.
Now, using the formula for the volume of a sphere:
4/3 × π × r³ = 5632 / 21
⇒ r³ = (5632 / 21) × (3/4) × (22/7) = 64 ⇒ r = 4 m
Hence, the diameter = 8 m.
Problem 5: The slant height of a right circular cone is 13 m and its height is 5 m. Find the area of the curved surface.
- 490.28 m²
- 288.28 m²
- 450 m²
- 200 m²
- None of these
Sol: Correct Answer is Option 1
The formula for the area of the curved surface of a cone is:
Area = π × r × l
Now, using the Pythagorean theorem to find the radius:
r = √(l² - h²) = √(13² - 5²) = √169 - 25 = √144 = 12 m
Hence, the required area = (22/7) × 13 × 12 = 490.28 m².
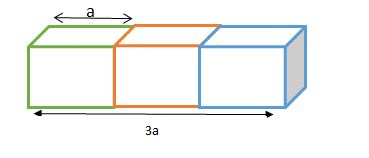
Problem 6: If three cubes are placed adjacently in a row, then the ratio of the total surface area of the new cuboid to the sum of the surface area of the three cubes will be
(1) 1:3
(2) 2:3
(3) 5:9
(4) 7:9
Correct Answer is Option (4)
We are given 3 cubes in the above question each with side a cm. When the cubes are placed adjacent in a row, then the cuboid formed has length 3a cm (a + a + a) and height and breadth as a cm. Thus,
T.S.A of the cuboid = 2(3a × a + a × a + 3a × a)
= 14a2 cm2
T.S.A. of three cubes = 3 × 6a2
= 18a2 cm2
Thus, the ratio of T.S.A of cuboid to that of cubes will be
14a2 cm2 : 18a2 cm2
7 : 9
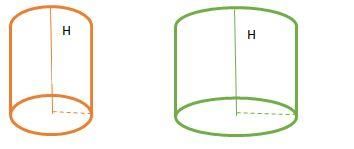
Problem 7: X and Y are two cylinders of the same height. The base of X has diameter that is half the diameter of the base of Y. If the height of X is doubled, the volume of X becomes
(1) Equal to the volume of Y
(2) Double the volume of Y
(3) Half the volume of Y
(4) Greater than the volume of Y
Correct Answer is Option (3)
Original Solids
There are two cylinders X and Y each with height H and diameter of X is 1/2 of the diameter of Y. Thus, volume of each solid is
Volume of X = Πr²H
Volume of Y = Π(2r)² H = 4Πr²H
Now, if the height of X is doubled then,
Volume of X = Πr² × 2H = 2Πr²H = ½ × 4Πr²H
Thus, the resultant cylinder has volume ½ of Y.
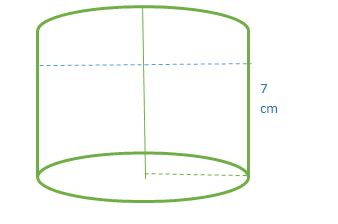
Problem 8: Water is poured into an empty cylindrical tank at a constant rate for 5 minutes. After the water has been poured into the tank, the depth of the water is 7 feet. The radius of the tank is 100 feet. Which of the following is the best approximation for the rate at which the water was poured into the tank
(1) 140 cubic feet/sec
(2) 440 cubic feet/sec
(3) 733 cubic feet/sec
(4) 2200 cubic feet/sec
Correct Answer is Option (3)
We need to find out the rate at which water is flowing into the tank. The volume of water flowed into the tank in 5 minutes = Πr²h = (22/7 × 100² × 7)
= 220000 ft³
The rate at which water is flowing into the tank is = (220000 / (5 × 60)) = 733.33 ft³/sec
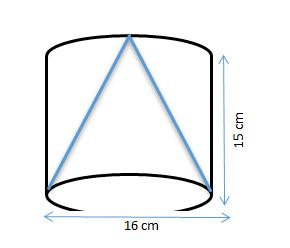
Problem 9: A conical cavity is drilled in a circular cylinder of 15 cm height and 16 cm base diameter. The height and base diameter of the cone is the same as those of the cylinder. Determine the total surface area of the remaining solid?
(1) 215 Π cm2
(2) 376 Π cm2
(3) 440 Π cm2
(4) 542 Π cm2
Correct Answer is Option (3)
In this question, we are given a cylinder out of which a cone is removed and we need to find out the total surface area of the remaining solid given in the above figure.
Therefore, T.S.A of the remaining solid = C.S.A of cylinder + C.S.A of the cone + area of the base of cylinder
= 2Πrh + Πrl + Πr²
= Π(2 × 8 × 15 + 8 × 17 + 8²)
= Π(240 + 136 + 64)
= 440Π cm2
Problem 10: Base of a right prism is a rectangle; the ratio of whole length and breadth is 3:2. If the height of the prism is 12 cm and total surface area is 288 cm², then the volume of the prism is
(1) 291 cm3
(2) 288 cm3
(3) 290 cm3
(4) 286 cm3
Correct Answer is Option (2)
Since the prism has a rectangular base, the prism formed is a cuboid with the ratio of length and breadth as 3:2 and height is 12 cm. Therefore T.S.A of cuboid = 288 cm²
288 = 2(lb + bh + hl)
288 = 2(3x × 2x + 2x × 12 + 12 × 3x)
288 = 2(6x² + 24x + 36x)
144 = 6x² + 60x
x² + 10x – 24 = 0
x² + 12x – 2x – 24 = 0
(x + 12)(x – 2) = 0
x = 2
Therefore, length is 6 cm and breadth is 4 cm. Thus, volume of cuboid = l × b × h = 6 × 4 × 12 = 288 cm³
Problem 11: Five marbles of various sizes are placed in a conical funnel. Each marble is in contact with the adjacent marble(s). Also, each marble is in contact all around the funnel wall. The smallest marble has a radius of 8 mm. The largest marble has a radius of 18 mm. What is the radius (in mm) of the middle marble?
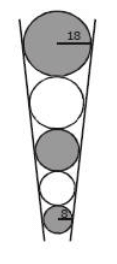 (1) 10
(1) 10
(2) 11
(3) 12
(4) 15
Correct Answer is Option (3)
The marbles are arranged in a conical funnel, so the radii form a geometric progression.
General Formula for a Geometric Progression: The formula for the n-th term of a geometric progression is given by:
rn = r1 × qn−1
Problem 12: Three identical cones with base radius r are placed on their base so that each is touching the other two. The radius of the circle drawn through their vertices is
(1) Smaller than r
(2) Equal to r
(3) Larger than r
(4) Depends on the height of the cones
Correct Answer is Option (3)
When three identical cones with base radius r are placed on their base such that each is touching the other two, the circle formed by the vertices of the cones (which connects the points at the top of the cones) will have a radius larger than r.
This is because the three cones form an equilateral triangle at their bases, and the radius of the circle passing through their vertices (at the top of the cones) will be determined by the distance from the centre of this equilateral triangle to the vertices, which is larger than the base radius r.
Hence, the radius of the circle drawn through their vertices is larger than r.
OR
The centres of the bases of the cones form a triangle of side 2r. The circumcircle of this triangle will be identical to a circle drawn through the vertices of the cones and thus, it will have a radius of (2/√3) times r, which is greater than r.
|
205 videos|264 docs|136 tests
|
FAQs on Mensuration: Volume, Surface Area & Solid Figures - CSAT Preparation - UPSC
| 1. What is the formula to calculate the volume of a cylinder? |  |
| 2. How do you find the surface area of a cube? |  |
| 3. What is the difference between a prism and a pyramid? |  |
| 4. How do you calculate the area of a circle? |  |
| 5. How do you find the perimeter of a quadrilateral? |  |