Class 10 Maths Chapter 3 HOTS Questions - Pair of Linear Equations in Two Variables
Q1. If two of the roots of f(x) = x3 – 5x2 – 16x + 80 are equal in magnitude but opposite in sign, then find all of its zeroes.
Sol: Let α and β are the two zeroes which are equal in magnitude but opposite in sign.
∴ α + β = 0
(Let the third zero is γ)
Sum of zeroes of
f(x)= α + β + γ = -(-5)1 = 5
∴ γ = 5 [α + β = 0]
Product of zeroes
= αβγ = -801 ⇒ 5 × αβ = -80 [∵ γ = 5]
∴ αβ = -805 = -16
⇒ – α2 = –16 [α + β = 0 ⇒ β = – α]
⇒ α2 = 16 ⇒ α = ± 4
α = ± 4 ⇒ β = ∓ 4
[∴ β = –α]
Thus, the zeroes are : [± 4, ∓ 4, and 5]
Q2. Solve :
3x + 2y = 13
5x - 3y = 9
Sol : Given equations:
3x + 2y = 13
5x - 3y = 9
Let 1x = p and 1y = q
The equations become:
3p + 2q = 13 ...(i)
5p - 3q = 9 ...(ii)
From equation (i):
2q = 13 - 3p
q = 13 - 3p2
Substitute q into equation (ii):
5p - 3( 13 - 3p2 ) = 9
Multiply through by 2 to eliminate the fraction:
10p - (39 - 9p) = 18
19p = 57
p = 3
Solve for q:
q = 13 - 92 = 42 = 2
Back-substitute for x and y:
x = 1p = 13
y = 1q = 12
Final Answer:
x = 13 , y = 12
Q3. Solve :
8x + 2y + 32x − y = 3
12x + 2y − 62x − y = 1
Hint: Put x + 2y = p and 2x – y = q
Sol: Given equations:
8x + 2y + 32x - y = 3
12x + 2y - 62x - y = 1
Let:
x + 2y = p, 2x - y = q
Substituting:
8p + 3q = 3 ...(1)
12p - 6q = 1 ...(2)
Eliminating fractions:
8q + 3p = 3pq ...(3)
12q - 6p = pq ...(4)
From (4):
pq = 12q - 6p
Substitute pq in (3):
8q + 3p = 3(12q - 6p)
8q + 3p = 36q - 18p
21p = 28q
p = 4q3
Substitute p into (1):
8p + 3q = 3
6q + 3q = 3
q = 3
Substitute q = 3 into p:
p = 4 × 33 = 4
Back-substitute:
x + 2y = 4, 2x - y = 3
Solving gives: x = 2, y = 1
Final Answer: x = 2, y = 1
Q4. Solve : x + y = 18 ; y + z = 12 ; z + x = 16.
Sol: Adding the three equations, we get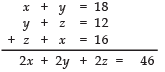
⇒ x + y + z = 23
Now, (x + y + z = 23) – (x + y = 18)
⇒ z = 5 (x + y + z = 23) – (y + z = 12)
⇒ x = 11 (x + y + z = 23) – (z + x = 16)
⇒ y = 7
Thus, x = 11, y = 7 and z = 5
Q5. Solve : xyx + y = 43 , yzy + z = 125 , zxz + x = 32
Sol: Inverting the equations:
x + yxy = 34 ⇒ 1x + 1y = 34 ...(1)
y + zyz = 512 ⇒ 1y + 1z = 512 ...(2)
z + xzx = 23 ⇒ 1z + 1x = 23 ...(3)
Adding (1), (2) and (3), we get
2x + 2y + 2z = 34 + 512 + 23 = 2212 = 116
⇒ 1x + 1y + 1z = 1112 ...(4)
Now, subtracting (1), (2) and (3) turn by turn from (4), we get x = 2, y = 4 and z = 6
Q6. Solve: x + y − 32 = x + 2y − 43 = 3x + y11
Sol:
From x + y − 32 = 3x + y11
⇒ 11(x + y – 3) = 2(3x + y) ⇒ 5x + 9y = 33 ...(1)
From x + 2y − 43 = 3x + y11
⇒ 11(x + 2y – 4) = 3(3x + y)
⇒ 2x + 9y = 44 ...(2)
Solving (1) and (2), we get x = 3 and y = 2
|
127 videos|683 docs|84 tests
|
FAQs on Class 10 Maths Chapter 3 HOTS Questions - Pair of Linear Equations in Two Variables
| 1. What are the methods to solve a pair of linear equations in two variables? |  |
| 2. How do you determine if a pair of linear equations has no solution, one solution, or infinitely many solutions? |  |
| 3. Can you explain the graphical representation of a pair of linear equations? |  |
| 4. What are the real-life applications of solving pairs of linear equations? |  |
| 5. How can you solve a pair of linear equations using the elimination method step by step? |  |

















