Basic Geometrical Concepts - RD Sharma 2019 | RD Sharma Solutions for Class 6 Mathematics PDF Download
Page No 10.10:
Question 27: Give the correct matching of the statements of Column A and Column B.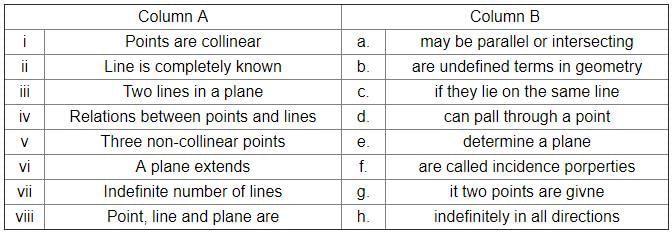
ANSWER: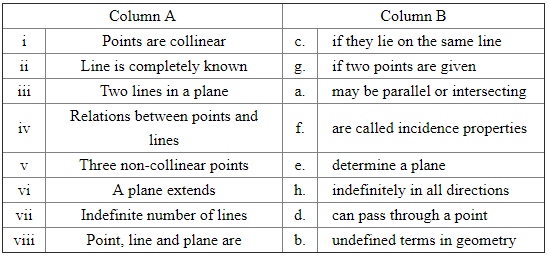
Page No 10.13:
Question 1: In fig. 10.32, points are given in two rows. Join the points AM, HE, TO, RUN, IF. How many liens segments are formed?
ANSWER:
If we join the points AM, HE, TO, RUN, IF, 6 line segments can be formed.
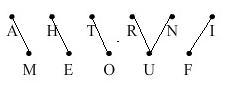
These six line segments are AM, HE, TO, RU, UN and IF.
Page No 10.13:
Question 2:
In Fig. 10.33, name:
(i) Five line segments.
(ii) Five rays.
(iii) Non-intersecting line segments.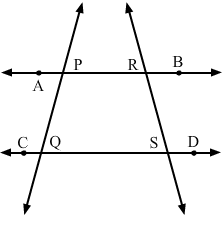
ANSWER:
(i) Five line segments are PQ, RS, PR, QS and AP.
(ii) Five rays are 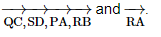
(iii) Non-intersecting line segments are PR and QS.
Page No 10.13:
Question 3: In each of the following cases, state whether you can draw line segments on the given surfaces:
(i) The face of a cuboid.
(ii) The surface of an egg or apple.
(iii) The curved surface of a cylinder.
(iv) The curved surface of a cone.
(v) The base of a cone.
ANSWER:
(i) Yes, we can draw line segments on the face of a cuboid.
(ii) No, we cannot draw a line segment on the surface of an egg or apple.
(iii) Yes, we can draw line segments on the curved surface of a cylinder.
Every line segment parallel to the axis of a cylinder on the curved surface will be a line segment.
(iv) Yes, we can draw line segments on the curved surface of a cone.
Every line segment joining the vertex of a cone and any point on the circumference of the cone will be a line segment.
(v) Yes, we can draw line segments on the base of a cone.
Page No 10.13:
Question 4: Mark the following points on a sheet of a paper. Tell how many line segments can be obtained in each case:
(i) Two points A, B.
(ii) Three non-collinear points A, B, C.
(iii) Four points such that no three of them boelong to the same line.
(iv) Any five points so that no three of them are collinear.
ANSWER:
If there are n points in a plane and no three of them are collinear, the number of line segments obtained by joining these points is equal to n(n−1)2n(n-1)2.
On applying the above formula, we get:
(i) For two points A and B:
Number of line segments = 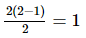
(ii) For three non-collinear points A, B and C:
Number of line segments = 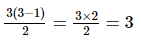
(iii) For four points such that no three of them belong to the same line:
Number of line segments = 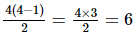
(iv) For any five points so that no three of them are collinear:
Number of line segments = 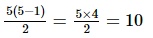
Page No 10.14:
Question 5: Count the number of line segments in Fig. 10.34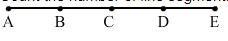
ANSWER:
Line segments in the given figure are AB, AC, AD, AE, BC, BD, BE, CD, CE and DE.
Thus, there are 10 line segments.
Page No 10.14:
Question 6:
In Fig. 10.35, name all rays with initial points as A, B and C respectively.
(i) Is ray AB−→−AB→ different from ray AC−→−AC→?
(ii) Is ray BA−→−BA→ different from ray CA−→−CA→ ?
(iii) Is ray CP−→−CP→ different from ray CQ−→−CQ→ ?
ANSWER:
Name of all rays with initial point as A : −→AP→AP and −→AB or −→AC or −→AQ→AB or →AC or →AQ
Name of all rays with initial point as B : −→BP, or −→BA, →BP, or →BA, and −→BC or −→BQ→BC or →BQ
Name of all rays with initial point as C : −→CP, or −→CA or −→CB→CP, or →CA or →CB and −→CQ→CQ
(i) No, because the origin point of both the rays −→AB→AB and −→AC→AC is same.
(ii) Yes, because the origin point of both the rays −→BA→BA and −→CA→CA is different.
(iii) Yes, because both the rays −→CP→CP and −→CQ→CQ are in opposite directions.
Page No 10.14:
Question 7:
Give three examples of line segments from your environment.
ANSWER:
Examples of line segments in our home is:
(i) grout lines in the tile floors
(ii) groves where wooden flooring connects
(iii) metal outline of a sliding glass door
Page No 10.16:
Question 1:
Draw rough diagram to illustrate the following:
(i) Open curve
(ii) Closed curve
ANSWER:
(i) Open curve
(ii) Closed curve
Page No 10.16:
Question 2:
Classify the following curves as open or closed:
ANSWER:
(i) Open
(ii) Closed
(iii) Closed
(iv) Open
(v) Open
(vi) Closed
Page No 10.16:
Question 3:
Draw a polygon and shade its interior. Also draw its diagonals, if any.
ANSWER:
ABCD is a polygon and AC and BD are its two diagonals.
Page No 10.17:
Question 4:
Illustrate, if possible, each one of the following with a rough diagram:
(i) A closed curve that is not a polygon.
(ii) An open curve made up entirely of line segments.
(iii) A polygon with two sides.
ANSWER:
(i) A circle is a simple closed curve but not a polygon. A polygon has line-segments, but a circle only has a curve.
(ii) Rough diagram of an open curve made up entirely of line segments:
(iii) A polygon with two sides is not possible.
Page No 10.7:
Question 1:
Mark three points in your notebook and name them.
ANSWER:
Three points, namely A, P and H can be marked as follows:
Page No 10.7:
Question 2:
Draw a line in your notebook and name it using a small letter of the alphabet.
ANSWER:
Let us draw a line and name it l.
Page No 10.7:
Question 3:
Draw a line in your notebook and name it by taking any two points on it.
ANSWER:
Let us first draw a line. Two points on it are P and Q. Now, the line can be written as line PQ.
Page No 10.8:
Question 4:
Give three examples from your environment of:
(i) Points
(ii) Portion of a line
(iii) Plane surface
(iv) Portion of a plane
(v) Curved surfaces
ANSWER:
(i) Three examples of points are:
The period at the end of a sentence, a pinhole on a map and the point at which two walls and the floor meet at the corner of the room
(ii) Three examples of portion of a line are:
Tightly stretched power cables, laser beams and thin curtain rods
(iii) Three examples of plane of a surface are:
The surface of a smooth wall, the surface of the top of a table and the surface of a smooth white board
(iv) Three examples of portion of a plane are:
The surface of a sheet of a paper, the surface of a calm water in a swimming pool and the surface of a mirror
(v) Three examples of curved surfaces are:
The surface of a gas cylinder, the surface of a tea pot and the surface of an ink pot
Page No 10.8:
Question 5:
There are a number of ways by which we can visualise a portion of a line. State whether the following represent a portion of a line of not:
(i) A piece of elastic stretched to the breaking point.
(ii) Wire between two electric poles.
(iii) The line thread by which a spider lowers itself.
ANSWER:
(i) Yes
(ii) No
(iii) Yes
Page No 10.8:
Question 6:
Can you draw a line on the surface of a sphere which lies wholly on it?
ANSWER:
No, we cannot draw a line on the surface of a sphere, which lies wholly on it.
Page No 10.8:
Question 7:
Mark a point on a sheet of paper and draw a line passing through it. How many lines can you raw through this point?
ANSWER:
Unlimited number of lines can be drawn passing through a point L.
Page No 10.8:
Question 8:
Mark any two points P and Q in your note book and draw a line passing through the points. How many liens can you draw passing through both the points?
ANSWER:
We have two points P and Q and we draw a line passing through these two points.
Only one line can be drawn passing through these two points.
Page No 10.8:
Question 9:
Give an example of a horizontal plane and a vertical plane form your environment.
ANSWER:
Ceiling of a room is an example of a horizontal plane in our environment.
Wall of a room is an example of a vertical plane in our environment.
Page No 10.8:
Question 10:
How many lines may pass through one given point, two given points, any three collinear points?
ANSWER:
Lines passing through one point: Unlimited
Lines passing through two points: One
Lines passing through any three collinear points : One
Page No 10.8:
Question 11:
Is it ever possible for aline to have a mid-point.
ANSWER:
Yes, it is possible if the three points lie on a straight line.
Page No 10.8:
Question 12:
Explain why it is not possible for aline to have a mid-point.
ANSWER:
The length of a line is infinite. Thus, it is not possible to find its mid-point.
On the other hand, we can find the mid-point of a line segment.
Page No 10.8:
Question 13:
Mark three non-collinear points A, B and C in your note book. Draw lines through these points taking two at a time. Name these lines. How many such different lines can be draw?
ANSWER:
There are three non-collinear points A, B and C.
Three lines can be drawn through these three points. These three lines are AB, BC and AC.
Page No 10.8:
Question 14:
Coplanar points are the points that are in the same plane. Thus,
(i) Can 150 points be coplanar?
(ii) Can 3 points be non-coplanar?
ANSWER:
(i) Yes
A group of points that lie in the same plane are called coplanar points.
Thus, it is possible that 150 points can be coplanar.
(ii) No
3 points will be coplanar because we can have a plane that can contain all 3 points on it.
Thus, it is not possible that 3 points will be non-coplanar.
Page No 10.8:
Question 15:
Using a ruler, check whether the following points given in Fig. 10.20 are collinear or not:
(i) D, A and C
(ii) A, B and C
(iii) A, B and E
(iv) B, C and E
ANSWER:
From the given figure, we can observe that:
(i) D, A and C are collinear points.
(ii) A, B and C are non-collinear points.
(iii) A, B and E are collinear points.
(iv) B, C and E are non-collinear points.
Page No 10.8:
Question 16:
Lines p, q are coplanar. So are the lines p, r. Can we conclude that the lines p, q, r are coplanar?
ANSWER:
No p, q and r are not necessarily coplanar .
e.g.:-If we take p as intersecting line of two consecutive walls of a room , q as a line on the first wall and r on the second wall whose(both walls) intersection is line p .
Then we can see that p , q and r are not coplanar.
Page No 10.8:
Question 17:
Give three examples each of:
(i) intersecting lines
(ii) parallel lines from your environment.
ANSWER:
(i) Three examples of intersecting lines in our environment:
(ii) Three examples of parallel lines in our environment:
Page No 10.8:
Question 18:
From Fig. 10.21, write
(i) all paris of parallel lines.
(ii) all pairs ofintersecting lines.
(iii) lines whose point of intersection is I.
(iv) lines whose point of intersection is D.
(v) lines whose point of intersection is E.
(vi) lines whose point of intersection is A.
(vii) Collinear points
ANSWER:
We have:
(i) All pairs of parallel lines: (l, m), (m, n) and (l, n)
(ii) All pairs of intersecting lines: (l, p), (m, p), (n, p), (l, r), (m, r), (n, r), (l, q), (m, q), (n, q), (q, p) and (q, r)
(iii) Lines whose point of intersection is I: (m, p)
(iv) Lines whose point of intersection is D: (l, r)
(v) Lines whose point of intersection is E: (m, r)
(vi) Lines whose point of intersection is A: (l, q)
(vii) Collinear points: (G, A, B and C), (D, E, J and F), (G, H, I , J and K), (A, H and D), (B, I and E), and (C, F and K)
Page No 10.9:
Question 19:
From Fig. 10.22, write concurrent lines and their points of concurrence.
ANSWER:
From the given figure, we have:
Concurrent lines can be defined as three or more lines which share the same meeting point.
Clearly lines n, q and l are concurrent with A as the point of concurrence.
Lines m, q and p are concurrent with B as the point of concurrence.
Page No 10.9:
Question 20:
Mark four points A, B, C and D in your notebook such that no three of them are collinear. Draw all the lines which join them in pairs as shown in Fig. 10.23.
(i) How many such lines can be drawn?
(ii) Write the names of these lines.
(iii) Name the lines which are concurrent at A.
ANSWER:
From the given figure, we have:
(i) Six lines can be drawn through these four points as given in the figure.
(ii) These lines are AB, BC, CD, AD, BD and AC.
(iii) Lines which are concurrent at A are AC, AB and AD.
Page No 10.9:
Question 21:
What is the maximum number of points of intersection of three lines in a plane? What is the minimum number?
ANSWER:
Maximum number of points of intersection of three lines in a plane will be three.
Minimum number of points of intersection of three lines in a plane will be zero.
Page No 10.9:
Question 22:
With the help of a figure, find the maximum and minimum number of points of intersection of four lines in a plane.
ANSWER:
Maximum number of points of intersection of four lines in a plane will be six.
Minimum number of points of intersection of four lines in a plane will be zero.
Page No 10.9:
Question 23:
Lines p, q and r are concurrent. Also, lines p, r and s are concurrent. Draw a figure and state whether lines p, q, r and s are concurrent or not.
ANSWER:
We have:
Thus, lines p, q and r intersect at a common point O.
Also, lines p, r and s are concurrent.
Therefore, lines p, r and s intersect at a common point. But q and r intersect each other at O.
So, s, q and r intersect at O.
Hence, p, q, r and s are concurrent. Lines p, q, r and s intersect at O.
Page No 10.9:
Question 24:
Lines p, q and r are concurrent. Also lines p, s and t are concurrent. Is it always true that the lines q, r and s will be concurrent? Is it always true for lines q, r and t?
ANSWER:
Lines p, q, and r are concurrent. So, lines p, q and r intersect at a common point O.
Given lines p, s and t are concurrent. So, lines p, s and t also intersect at a common point.
However, it is not always true that q, r and s or q, r and t are concurrent.
Page No 10.9:
Question 25:
Fill in the blank in the following statements using suitable words:
(i) A page of a book is a physical example of a .....
(ii) An inkpot has both .... surfaces
(iii) Two lines in a plane are either .....or are
ANSWER:
(i) A page of a book is a physical example of a plane.
(ii) An ink pot has both curved and plane surfaces.
(iii) Two lines in a plane are either parallel or are intersecting.
Page No 10.9:
Question 26:
State which of the following statements are true (T) and which are false (F).
(i) Point has a size because we can see it as a thick dot on paper.
(ii) By liens in geometry, we mean only straight lines.
(iii) Two lines in a plane always intersect in a point.
(iv) Any plane through a vertical line is vertical.
(v) Any plane through a horizontal line is horizontal.
(vi) There cannot be a horizontal line a vertical plane.
(vii) All lines in a horizontal plane are horizontal.
(viii) Two lines in a plane always intersect in a point.
(ix) If two lines intersect at a point P, then P is called the point of concurrence of the two lines.
(x) If two lines intersect at a point P, then p is called the point of intersection of the two lines.
(xi) If A,B,C and D are collinear points D, P and Q are collinear, then points A, B, C, D, P and Q are always collinear.
(xii) Two different lines can be drawn passing through two given points.
(xiii) Through a given point only one line can be drawn.
(xiv) Four points are collinear if any three of them lie on the same line.
(xv) The maximum number of points of intersection of three lines is three.
(xvi) The minimum number of points of intersection of three lines is one.
ANSWER:
(i) False
(ii) True
(iii) False
They may be parallel.
(iv) True
(v) False
In every vertical plane there must be horizontal line.
e.g.:-Intersecting line of a wall and floor of a room is horizontal line and it is also a line on the wall that is on the vertical plane.
(vi) False
Counter example:-Intersecting line of a wall and floor of a room is horizontal line and it is also a line on the wall that is on the vertical plane.
(vii) True
(viii) False
They can be parallel line also.
(ix) False
Through a point of concurrence, at least three lines should pass.
(x) True
(xi) False
Here ABCD is in one plane and DPQ may be in different plane and they may be intersecting through the point D.
(xii) False
Two lines are either intersecting at one point or they may be parallel lines or they may be coincident lines.
(xiii) False
Through a given point infinite line can be drawn.
(xiv) False
Points are collinear only if all points are in the same plane.
(xv) True
(xvi) False
Minimum number of points of intersection of three lines be zero.
FAQs on Basic Geometrical Concepts - RD Sharma 2019 - RD Sharma Solutions for Class 6 Mathematics
| 1. What are the basic geometrical concepts covered in RD Sharma 2019 Class 6? |  |
| 2. How can I understand basic geometrical concepts better for my Class 6 exams? |  |
| 3. What are some real-life examples of geometrical concepts covered in RD Sharma 2019 Class 6? |  |
| 4. How can I improve my problem-solving skills in geometry for Class 6 exams? |  |
| 5. Are there any online resources or websites that can help me with understanding basic geometrical concepts for Class 6 exams? |  |
















