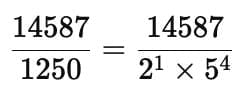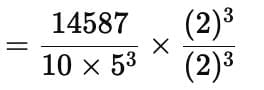NCERT Exemplar: Real Numbers | Mathematics (Maths) Class 10 PDF Download
| Table of contents |

|
| Exercise 1.1 |

|
| Exercise 1.2 |

|
| Exercise 1.3 |

|
| Exercise 1.4 |

|
Exercise 1.1
Choose the correct answer from the given four options in the following questions:
Q.1. For some integer m, every even integer is of the form:
(a) m
(b) m + 1
(c) 2m
(d) 2m + 1
Correct Answer is option (c)
Even integers are those integers which are divisible by 2.
Hence, we can say that every integer which is a multiple of 2 must be an even integer.
Therefore, let us conclude that,
for an integer ‘m’, every even integer must be of the form
2 × m = 2m.
Hence, option (c) is the correct answer.
Q.2. For some integer q, every odd integer is of the form
(a) q
(b) q +1
(c) 2q
(d) 2q +1
Correct Answer is option (d)
Odd integers are those integers which are not divisible by 2.
Hence, we can say that every integer which is a multiple of 2 must be an even integer, while 1 added to every integer which is multiplied by 2 is an odd integer.
Therefore, let us conclude that,
for an integer ‘q’, every odd integer must be of the form
(2 × q) + 1 = 2q + 1.
Hence, option (d) is the correct answer.
Q.3. n2 – 1 is divisible by 8, if n is
(a) an integer
(b) a natural number
(c) an odd integer
(d) an even integer
Correct Answer is option (c)
Let x = n2 – 1
In the above equation, n can be either even or odd.
Let us assume that n= even.
So, when n = even i.e., n = 2k, where k is an integer,
We get,
⇒ x = (2k)2-1
⇒ x = 4k2 – 1
At k = -1, x = 4(-1)2 – 1 = 4 – 1 = 3, is not divisible by 8.
At k = 0, x = 4(0)2 – 1 = 0 – 1 = -1, is not divisible by 8
Let us assume that n= odd:
So, when n = odd i.e., n = 2k + 1, where k is an integer,
We get,
⇒ x = 2k + 1
⇒ x = (2k + 1)2 – 1
⇒ x = 4k2 + 4k + 1 – 1
⇒ x = 4k2 + 4k
⇒ x = 4k(k + 1)
At k = -1, x = 4(-1)(-1 + 1) = 0 which is divisible by 8.
At k = 0, x = 4(0)(0 + 1) = 0 which is divisible by 8.
At k = 1, x = 4(1)(1 + 1) = 8 which is divisible by 8.
From the above two observation, we can conclude that, if n is odd, n2-1 is divisible by 8.
Hence, option (c) is the correct answer.
Q.4. If the HCF of 65 and 117 is expressible in the form 65m – 117, then the value of m is
(a) 4
(b) 2
(c) 1
(d) 3
Correct Answer is option (b)
Let us find the HCF of 65 and 117,
117 = 1 × 65 + 52
65 = 1 × 52 + 13
52 = 4 × 13 + 0
Hence, we get the HCF of 65 and 117 = 13.
According to the question,
65m – 117 = 13
65m = 117 + 13 = 130
∴ m = 130/65 = 2
Hence, option (b) is the correct answer.
Q.5. The largest number which divides 70 and 125, leaving remainders 5 and 8, respectively, is
(a) 13
(b) 65
(c) 875
(d) 1750
Correct Answer is option (a)
According to the question,
We have to find the largest number which divides 70 and 125, leaving remainders 5 and 8.
This can be also written as,
To find the largest number which exactly divides (70 – 5), and (125 – 8)
The largest number that divides 65 and 117 is also the Highest Common Factor of 65 and 117
Therefore, the required number is the HCF of 65 and 117
Factors of 65 = 1, 5, 13, 65
Factors of 117 = 1, 3, 9, 13, 39, 117
Common Factors = 1, 13
Highest Common factor (HCF) = 13
i.e., the largest number which divides 70 and 125, leaving remainders 5 and 8, respectively = 13
Hence, option (a) is the correct answer.
Q.6. If two positive integers a and b are written as a = x3y2 and b = xy3; x, y are prime numbers, then HCF (a, b) is
(a) xy
(b) xy2
(c) x3y3
(d) x3y2
Correct Answer is option (b)
It is given that,
a = x3y2 = x × x × x × y × y
b = xy3 = x × y × y × y
The HCF (Highest Common Factor) of two or more numbers is the highest number among all the common factors of the given numbers.
As HCF is the product of the smallest power of each common prime factor involved in the numbers.
HCF of a and b = HCF (x3y2, xy3)
= x × y × y
= xy2
Therefore, HCF (a, b) is xy2
Hence, option (b) is the correct answer.
Q.7. If two positive integers p and q can be expressed as p = ab2 and q = a3b; a, b being prime numbers, then LCM (p, q) is
(a) ab
(b) a2b2
(c) a3b2
(d) a3b3
Correct Answer is option (c)
It is given that,
p = ab2 = a × b × b
q = a3b = a × a × a × b
Least Common Multiple(LCM) is a method to find the smallest common multiple between any two or more numbers
LCM is the product of the greatest power of each prime factor involved in the numbers.
LCM of p and q = LCM (ab2, a3b) = a × b × b × a × a = a3b2
Therefore, LCM (p, q) is a3b2
Hence, option (c) is the correct answer.
Q.8. The product of a non-zero rational and an irrational number is
(a) always irrational
(b) always rational
(c) rational or irrational
(d) one
Correct Answer is option (a)
It is given that,
Product of a non-zero rational and an irrational number is always irrational.
Therefore, the answer is a. always irrational
If a is a rational number and b is an irrational number
Then ab is irrational.
Example: a = 2 and b = √3
ab = 2 √3
2 √3 is an irrational number.
Therefore, the product of a rational and irrational number is always irrational
Hence, option (a) is the correct answer.
Q.9. The least number that is divisible by all the numbers from 1 to 10 (both inclusive) is
(a) 10
(b) 100
(c) 504
(d) 2520
Correct Answer is option (d)
Given,
Factors of numbers from 1 to 10
1 = 1
2 = 1 × 2
3 = 1 × 3
4 = 1 × 2 × 2
5 = 1 × 5
6 = 1 × 2 × 3
7 = 1 × 7
8 = 1 × 2 × 2 × 2
9 = 1 × 3 × 3
10 = 1 × 2 × 5
LCM of numbers from 1 to 10 = LCM(1, 2, 3, 4, 5, 6, 7, 8, 9, 10)
=1 × 2 × 2 × 2 × 3 × 3 × 5 × 7
= 2520
Therefore, the least number is 2520.
Hence, option (d) is the correct answer.
Q.10. The decimal expansion of the rational number 14587/1250 will terminate after:
(a) one decimal place
(b) two decimal places
(c) three decimal places
(d) four decimal places
Correct Answer is option (d)
Given, 14587/ 1250
The denominator can be written asTherefore, it will terminate after four decimal placesHence, option (d) is the correct answer.
Exercise 1.2
Q.1. Write whether every positive integer can be of the form 4q + 2, where q is an integer. Justify your answer.
No, every positive integer cannot be of the form 4q + 2, where q is an integer.
Justification:
All the numbers of the form 4q + 2, where ‘q’ is an integer, are even numbers which are not divisible by ‘4’.
For example,
When q = 1,
4q + 2 = 4(1) + 2 = 6.
When q = 2,
4q + 2 = 4(2) + 2 = 10
When q = 0,
4q + 2 = 4(0) + 2 = 2 and so on.
So, any number which is of the form 4q + 2 will give only even numbers which are not multiples of 4.
Hence, every positive integer cannot be written in the form 4q + 2
Q.2. “The product of two consecutive positive integers is divisible by 2”. Is this statement true or false? Give reasons.
Yes, the statement “the product of two consecutive positive integers is divisible by 2” is true.
Justification:
Let the two consecutive positive integers = a, a + 1
According to Euclid’s division lemma,
We have,
a = bq + r, where 0 ≤ r < b
For b = 2, we have a = 2q + r, where 0 ≤ r < 2 … (i)
Substituting r = 0 in equation (i),
We get,
a = 2q, is divisible by 2.
a + 1 = 2q + 1, is not divisible by 2.
Substituting r = 1 in equation (i),
We get,
a = 2q + 1, is not divisible by 2.
a + 1 = 2q + 1 + 1 = 2q + 2, is divisible by 2.
Thus, we can conclude that, for 0 ≤ r < 2, one out of every two consecutive integers is divisible by 2. So, the product of the two consecutive positive numbers will also be even.
Hence, the statement “product of two consecutive positive integers is divisible by 2” is true.
Q.3. “The product of three consecutive positive integers is divisible by 6”. Is this statement true or false? Justify your answer.
Yes, the statement “the product of three consecutive positive integers is divisible by 6” is true.
Justification:
Consider the 3 consecutive numbers 2, 3, 4
(2 × 3 × 4)/6 = 24/6 = 4
Now, consider another 3 consecutive numbers 4, 5, 6
(4 × 5 × 6)/6 = 120/6 = 20
Now, consider another 3 consecutive numbers 7, 8, 9
(7 × 8 × 9)/6 = 504/6 = 84
Hence, the statement “product of three consecutive positive integers is divisible by 6” is true.
Q.4. Write whether the square of any positive integer can be of the form 3m + 2, where m is a natural number. Justify your answer.
No, the square of any positive integer cannot be written in the form 3m + 2 where m is a natural number
Justification:
According to Euclid’s division lemma,
A positive integer ‘a’ can be written in the form of bq + r
a = bq + r, where b, q and r are any integers,
For b = 3
a = 3(q) + r, where, r can be an integers,
For r = 0, 1, 2, 3……….
3q + 0, 3q + 1, 3q + 2, 3q + 3……. are positive integers,
(3q)2 = 9q² = 3(3q²) = 3m (where 3q² = m)
(3q+1)2 = (3q+1)² = 9q²+1+6q = 3(3q²+2q) +1 = 3m + 1 (Where, m = 3q²+2q)
(3q+2)2 = (3q+2)² = 9q²+4+12q = 3(3q²+4q) +4 = 3m + 4 (Where, m = 3q²+2q)
(3q+3)2 = (3q+3)² = 9q²+9+18q = 3(3q²+6q) +9 = 3m + 9 (Where, m = 3q²+2q)
Hence, there is no positive integer whose square can be written in the form 3m + 2 where m is a natural number.
Q.5. A positive integer is of the form 3q + 1, q being a natural number. Can you write its square in any form other than 3m + 1, i.e., 3m or 3m + 2 for some integer m? Justify your answer.
No.
Justification:
Consider the positive integer 3q + 1, where q is a natural number.
(3q + 1)2 = 9q2 + 6q + 1
= 3(3q2 + 2q) + 1
= 3m + 1, (where m is an integer which is equal to 3q2 + 2q.
Thus (3q + 1)2 cannot be expressed in any other form apart from 3m + 1.
Q.6. The numbers 525 and 3000 are both divisible only by 3, 5, 15, 25 and 75. What is HCF (525, 3000)? Justify your answer.
Using the Euclid’s lemma,
3000 = 525 × 5 + 375
We know that
dividend = divisor × quotient + remainder
525 = 375 × 1 + 150
375 = 150 × 2 + 75
150 = 75 × 2 + 0
HCF (525, 3000) = 75.
As 3, 5, 15, 25 and 75 divides 525 and 3000, then all are common factors of 525 and 3000.
Highest common factor is 75
Therefore, the highest common factor among these numbers is 75
Q.7. Explain why 3 × 5 × 7 + 7 is a composite number.
Yes.
Justification:
A composite number is a number that is divisible by another number other than by itself and one. The number 2 is the only even prime number. All other even numbers are composite numbers.
As per BODMAS rule:
3 × 5 × 7 + 7
= 105 + 7
= 112.
112 is an even number.
Therefore,112 is a composite number
Q.8. Can two numbers have 18 as their HCF and 380 as their LCM? Give reasons.
No.
Justification:
HCF is always a factor of LCM but 18 is not a factor of 380
Therefore, 18 is not a factor of 380.
Q.9. Without actually performing the long division, find if 987/10500 will have terminating or non-terminating (repeating) decimal expansion. Give reasons for your answer.
Termination Decimal
Justification:
The given number is 987/10500
Let us determine the HCF of 987 and 10500.
987 = 3 × 7 × 47
10500 = 2 × 2 × 3 × 5 × 5 × 5 × 7
HCF of (987, 10500) = 3 × 7
HCF of (987, 10500) = 21.
By dividing the numerator and denominator with HCF,
= (987 / 21) / (10500 / 21)
= 47 / 500
987 / 10500 = 47 / 500.
The denominator 500 can be written as
500 = 22 × 53
2 and 5 are the factors.
If a fraction contains no other prime factors other than 2 and 5, it can be written in the lowest terms as the terminating decimal.
Therefore, 987/10500 is terminating decimal.
Q.10. A rational number in its decimal expansion is 327.7081. What can you say about the prime factors of q, when this number is expressed in the form p/q? Give reasons.
The prime factors of q are 2 and 5
Justification:
327.7081 is the terminating decimal number.
It represents a rational number and also its denominator must have the form 2m x 5n.
327.7081 = 3277081/10000 = p/q
q = 104 = 2 × 2 × 2 × 2 × 5 × 5 × 5 × 5 = 24 × 54 = (2 × 5)4
The prime factors of q are 2 and 5
Therefore, the prime factors of q are 2 and 5.
Exercise 1.3
Q.1. Show that the square of any positive integer is either of the form 4q or 4q + 1 for some integer q.
According to Euclid’s division lemma,
a=bq + r
According to the question,
When b = 4.
a = 4k + r, 0 < r < 4
When r = 0, we get, a = 4k
a2 = 16k2 = 4(4k2) = 4q, where q = 4k2
When r = 1, we get, a = 4k + 1
a2 = (4k + 1)2 = 16k2 + 1 + 8k = 4(4k + 2) + 1 = 4q + 1, where q = k(4k + 2)
When r = 2, we get, a = 4k + 2
a2 = (4k + 2)2 = 16k2 + 4 + 16k = 4(4k2 + 4k + 1) = 4q, where q = 4k2 + 4k + 1
When r = 3, we get, a = 4k + 3
a2 = (4k + 3)2 = 16k2 + 9 + 24k = 4(4k2 + 6k + 2) + 1
= 4q + 1, where q = 4k2 + 6k + 2
Therefore, the square of any positive integer is either of the form 4q or 4q + 1 for some integer q.
Hence Proved.
Q.2. Show that cube of any positive integer is of the form 4m, 4m + 1 or 4m + 3, for some integer m.
Let a be any positive integer and b = 4.
According to Euclid Division Lemma,
a = bq + r [0 ≤ r < b]
a = 3q + r [0 ≤ r < 4]
According to the question, the possible values of r are,
r = 0, r = 1, r = 2, r = 3
When r = 0,
a = 4q + 0
a = 4q
Taking cubes on LHS and RHS,
We have,
a³ = (4q)³
a³ = 4 (16q³)
a³ = 4m [where m is an integer = 16q³]
When r = 1,
a = 4q + 1
Taking cubes on LHS and RHS,
We have,
a³ = (4q + 1)³
a³ = 64q³ + 1³ + 3 × 4q × 1 (4q + 1)
a³ = 64q³ + 1 + 48q² + 12q
a³ = 4 (16q³ + 12q² + 3q) + 1
a³ = 4m + 1 [where m is an integer = 16q³ + 12q² + 3q]
When r = 2,
a = 4q + 2
Taking cubes on LHS and RHS,
We have,
a³ = (4q + 2)³a³ = 64q³ + 2³ + 3 × 4q × 2 (4q + 2)
a³ = 64q³ + 8 + 96q² + 48q
a³ = 4 (16q³ + 2 + 24q² + 12q)
a³ = 4m [where m is an integer =16q³ + 2 + 24q² + 12q]
When r = 3,
a = 4q + 3
Taking cubes on LHS and RHS,
We have,
a³ = (4q + 3)³
a³ = 64q³ + 27 + 3 × 4q × 3 (4q + 3)
a³ = 64q³ + 24 + 3 + 144q² + 108q
a³ = 4 (16q³ + 36q² + 27q + 6) + 3
a³ = 4m + 3 [where m is an integer =16q³ + 36q² + 27q + 6]
Hence, the cube of any positive integer is in the form of 4m, 4m+1 or 4m+3.
Q.3. Show that the square of any positive integer cannot be of the form 5q + 2 or 5q + 3 for any integer q.
Let the positive integer = a
According to Euclid’s division lemma,
a = bm + r
According to the question, b = 5
a = 5m + r
So, r= 0, 1, 2, 3, 4
When r = 0, a = 5m.
When r = 1, a = 5m + 1.
When r = 2, a = 5m + 2.
When r = 3, a = 5m + 3.
When r = 4, a = 5m + 4.
Now,
When a = 5m
a2 = (5m)2 = 25m2
a2 = 5(5m2) = 5q, where q = 5m2
When a = 5m + 1
a2 = (5m + 1)2 = 25m2 + 10 m + 1
a2 = 5 (5m2 + 2m) + 1 = 5q + 1, where q = 5m2 + 2m
When a = 5m + 2
a2 = (5m + 2)2
a2 = 25m2 + 20m + 4
a2 = 5 (5m2 + 4m) + 4
a2 = 5q + 4 where q = 5m2 + 4m
When a = 5m + 3
a2 = (5m + 3)2 = 25m2 + 30m + 9
a2 = 5 (5m2 + 6m + 1) + 4
a2 = 5q + 4 where q = 5m2 + 6m + 1
When a = 5m + 4
a2 = (5m + 4)2 = 25m2 + 40m + 16
a2 = 5 (5m2 + 8m + 3) + 1
a2 = 5q + 1 where q = 5m2 + 8m + 3
Therefore, square of any positive integer cannot be of the form 5q + 2 or 5q + 3.
Hence Proved.
Q.4. Show that the square of any positive integer cannot be of the form 6m + 2 or 6m + 5 for any integer m.
Let the positive integer = a
According to Euclid’s division algorithm,
a = 6q + r, where 0 ≤ r < 6
a2 = (6q + r)2 = 36q2 + r2 + 12qr [∵(a+b)2 = a2 + 2ab + b2]
a2 = 6(6q2 + 2qr) + r2 …(i), where,0 ≤ r < 6
When r = 0, substituting r = 0 in Eq.(i), we get
a2 = 6 (6q2) = 6m, where, m = 6q2 is an integer.
When r = 1, substituting r = 1 in Eq.(i), we get
a2 = 6 (6q2 + 2q) + 1 = 6m + 1, where, m = (6q2 + 2q) is an integer.
When r = 2, substituting r = 2 in Eq(i), we get
a2 = 6(6q2 + 4q) + 4 = 6m + 4, where, m = (6q2 + 4q) is an integer.
When r = 3, substituting r = 3 in Eq.(i), we get
a2 = 6(6q2 + 6q) + 9 = 6(6q2 + 6a) + 6 + 3
a2 = 6(6q2 + 6q + 1) + 3 = 6m + 3, where, m = (6q + 6q + 1) is integer.
When r = 4, substituting r = 4 in Eq.(i) we get
a2 = 6(6q2 + 8q) + 16
= 6(6q2 + 8q) + 12 + 4
⇒ a2 = 6(6q2 + 8q + 2) + 4 = 6m + 4, where, m = (6q2 + 8q + 2) is integer.
When r = 5, substituting r = 5 in Eq.(i), we get
a2 = 6 (6q2 + 10q) + 25 = 6(6q2 + 10q) + 24 + 1
a2 = 6(6q2 + 10q + 4) + 1 = 6m + 1, where, m = (6q2 + 10q + 4) is integer.
Hence, the square of any positive integer cannot be of the form 6m + 2 or 6m + 5 for any integer m.
Hence Proved
Q.5. Show that the square of any odd integer is of the form 4q + 1, for some integer q.
Let a be any odd integer and b = 4.
According to Euclid’s algorithm,
a = 4m + r for some integer m ≥ 0
And r = 0,1,2,3 because 0 ≤ r < 4.
So, we have that,
a = 4m or 4m + 1 or 4m + 2 or 4m + 3 So, a = 4m + 1 or 4m + 3
We know that, a cannot be 4m or 4m + 2, as they are divisible by 2.
(4m + 1)2 = 16m2 + 8m + 1
= 4(4m2 + 2m) + 1
= 4q + 1, where q is some integer and q = 4m2 + 2m.
(4m + 3)2 = 16m2 + 24m + 9
= 4(4m2 + 6m + 2) + 1
= 4q + 1, where q is some integer and q = 4m2 + 6m + 2
Therefore, Square of any odd integer is of the form 4q + 1, for some integer q.
Hence Proved.
Q.6. If n is an odd integer, then show that n2 – 1 is divisible by 8.
We know that any odd positive integer n can be written in form 4q + 1 or 4q + 3.
So, according to the question,
When n = 4q + 1,
Then n2 – 1 = (4q + 1)2 – 1 = 16q2 + 8q + 1 – 1 = 8q(2q + 1), is divisible by 8.
When n = 4q + 3,
Then n2 – 1 = (4q + 3)2 – 1 = 16q2 + 24q + 9 – 1 = 8(2q2 + 3q + 1), is divisible by 8.
So, from the above equations, it is clear that, if n is an odd positive integer
n2 – 1 is divisible by 8.
Hence Proved.
Q.7. Prove that if x and y are both odd positive integers, then x2 + y2 is even but not divisible by 4.
Let the two odd positive numbers x and y be 2k + 1 and 2p + 1, respectively
i.e., x2 + y2 = (2k + 1)2 +(2p + 1)2
= 4k2 + 4k + 1 + 4p2 + 4p + 1
= 4k2 + 4p2 + 4k + 4p + 2
= 4 (k2 + p2 + k + p) + 2
Thus, the sum of square is even the number is not divisible by 4
Therefore, if x and y are odd positive integer, then x2 + y2 is even but not divisible by four.
Hence Proved
Q.8. Use Euclid’s division algorithm to find the HCF of 441, 567, 693.
The Euclidean Algorithm to determine the HCF (A,B) is:
If A = 0 then HCF (A, B) = B,
As HCF (0, B) = B we can stop.
If B = 0 then HCF (A, B) = A,
As HCF (A, 0) = A we can stop.
Now let us write A in quotient remainder form i.e A = BQ + R
By using the Euclidean Algorithm as HCF (A, B) = HCF(B, R) we can determine the HCF (B, R)
We know that, HCF of 441 and 567 is
567 = 441 × 1 + 126
441 = 126 × 3 + 63
126 = 63 × 2 + 0
Remainder is 0,
Therefore, H.C.F of (441, 567) is = 63.
H.C.F of 63 and 693 is 693 = 63 × 11 + 0
Therefore, H.C.F of (441, 567, 693) = 63
Q.9. Using Euclid’s division algorithm, find the largest number that divides 1251, 9377 and 15628 leaving remainders 1, 2 and 3, respectively.
The remainders of 1251, 9377 and 15628 are 1, 2 and 3
By subtracting these remainders from the numbers, we get
1251 - 1 = 1250,
9377 - 2 = 9375
15628 - 3 = 15625,which are divisible by the required number.
Required number = HCF (1250, 9375, 15625).
Using Euclid’s division algorithm,
a = bq + r --- (i)
We know that dividend = divisor x quotient + remainder
Consider a = 15625 and b = 9375
15625 = 9375 × 1 + 6250 [from eq. (i)]
9375 = 6250 × 1 +3125
6250 = 3125 × 2 + 0
HCF (15625, 9375) = 3125.
Taking c = 1250 and d = 3125,
Again by using Euclid’s division algorithm, d = cq + r
3125 = 1250 × 2 + 625
1250 = 625 × 2 + 0
HCF (1250, 9375,15625) = 625
Therefore, 625 is the largest number which divides 1251, 9377 and 15628 leaving remainders, 1, 2 and 3, respectively.
Q.10. Prove that √3 + √5 is irrational
Considering √3 + √5 is rational.
Here √3 + √5 = a , where a is rational.
We can write it as
√3 = a - √5
By squaring both sides,
(√3)2 = (a - √5)2
Using the algebraic identity (a - b)2 = a2 + b2 - 2ab
3 = a2 + 5 - 2a√5
2a√5 = a2 + 2
Therefore, √5 = a2 + 2/ 2a, which is a contradiction as the right hand side is a rational number while √5 is irrational
Therefore, √3 + √5 is irrational
Hence Proved.
Q.11. Show that 12n cannot end with the digit 0 or 5 for any natural number n.
If any number ends with the digit 0 or 5, it is always divisible by 5.
If 12n ends with the digit zero it must be divisible by 5.
This is possible only if prime factorisation of 12n contains the prime number 5.
Now, 12 = 2 × 2 × 3 = 22 × 3
⇒ 12n = (22 × 3)n = 22n × 3n [since, there is no term contains 5]
Hence, there is no value of n for which 12n ends with digit zero or five.
Hence Proved.
Q.12. On a morning walk, three persons step off together and their steps measure 40 cm, 42 cm and 45 cm, respectively. What is the minimum distance each should walk so that each can cover the same distance in complete steps?
The LCM of 40 cm, 42 cm and 45 cm has to be found to get the required minimum distance.
Now,
40 = 2 × 2 × 2 × 5
42 = 2 × 3 × 7
45 = 3 × 3 × 5
LCM(40, 42, 45) = 2 × 3 × 5 × 2 × 2 × 3 × 7
= 2520.
Therefore, the minimum distance each should walk is 2520 cm so that each can cover the same distance in complete steps.
Q,13. Write the denominator of the rational number 257/5000 n the form 2m × 5n, where m, n are non-negative integers. Hence, write its decimal expansion, without actual division.
We know that
Denominator of the rational number 257/5000 is 5000.
Factors of 5000 = 2 × 2 × 2 × 5 × 5 × 5 × 5
= (2)3 × (5)4
which is of the type 2m × 5n.
m, n are non-negative integers.
257/5000 = 257/ (2)3 × (5)4 × 2/2.
= 514/(2)4 × (5)4
= 514/(10)4
= 514/10000
= 0.0514
Therefore, 0.0514 is the required decimal expansion
Q14. Prove that √p + √q is irrational, where p, q are primes.
Consider that √p + √q is rational.
Now √p + √q = a , where a is rational.
Therefore, √q = a - √p
By squaring on both sides, we get q = a2 + p - 2 a√p
Using the algebraic identity
(a - b)2 = a2 + b2 - 2ab
√p = a2 + p - q/2a, which is a contradiction as the right hand side is a rational number while √p is irrational, since p is a prime number
Therefore, √p + √q is irrational
Hence Proved.
Exercise 1.4
Q.1. Show that the cube of a positive integer of the form 6q + r, q is an integer and r = 0, 1, 2, 3, 4, 5 is also of the form 6m + r.
6q + r is a positive integer, where q is an integer and r = 0, 1, 2, 3, 4, 5
Then, the positive integers are of form 6q, 6q+1, 6q+2, 6q+3, 6q+4 and 6q+5.
Taking cube on L.H.S and R.H.S,
For 6q,
(6q)³ = 216 q³ = 6(36q)³ + 0
= 6m + 0, (where m is an integer = (36q)³)
For 6q + 1,
(6q + 1)³ = 216q³ + 108q2 + 18q + 1
= 6(36q³ + 18q2 + 3q) + 1
= 6m + 1, (where m is an integer = 36q³ + 18q2 + 3q)
For 6q + 2,
(6q + 2)³ = 216q³ + 216q2 + 72q + 8
= 6(36q³ + 36q2 + 12q + 1) +2
= 6m + 2, (where m is an integer = 36q³ + 36q2 + 12q + 1)
For 6q + 3,
(6q + 3)³ = 216q³ + 324q2 + 162q + 27
= 6(36q³ + 54q2 + 27q + 4) + 3
= 6m + 3, (where m is an integer = 36q³ + 54q2 + 27q + 4)
For 6q + 4,
(6q + 4)³ = 216q³ + 432q2 + 288q + 64
= 6(36q³ + 72q2 + 48q + 10) + 4
= 6m + 4, (where m is an integer = 36q³ + 72q2 + 48q + 10)
For 6q + 5,
(6q + 5)³ = 216q³ + 540q2 + 450q + 125
= 6(36q³ + 90q2 + 75q + 20) + 5
= 6m + 5, (where m is an integer = 36q³ + 90q2 + 75q + 20)
Hence, the cube of a positive integer of the form 6q + r, q is an integer and r = 0, 1, 2, 3, 4, 5 is also of the form 6m + r.
Q.2. Prove that one and only one out of n, n + 2 and n + 4 is divisible by 3, where n is any positive integer.
According to Euclid’s division Lemma,
Let the positive integer = n
And b = 3
n = 3q+r, where q is the quotient and r is the remainder
0 < r < 3 implies remainders may be 0, 1 and 2
Therefore, n may be in the form of 3q, 3q+1, 3q+2
When n=3q
n + 2 = 3q + 2
n + 4 = 3q + 4
Here n is only divisible by 3
When n = 3q + 1
n + 2 = 3q = 3
n + 4 = 3q + 5
Here only n + 2 is divisible by 3
When n = 3q + 2
n + 2 = 3q + 4
n + 4 = 3q + 2 + 4 = 3q + 6
Here only n + 4 is divisible by 3
So, we can conclude that one and only one out of n, n + 2 and n + 4 is divisible by 3.
Hence Proved
Q.3. Prove that one of any three consecutive positive integers must be divisible by 3.
Consider three consecutive positive integers as n, n +1 and n + 2
Now dividing n by 3, consider q as the quotient and r as the remainder.
Using Euclid’s division algorithm,
n = 3q + r, where 0 ≤ r < 3
n = 3q or n = 3q + 1 or n = 3q + 2.
Case I:
When n = 3q, divisible by 3
(n + 1) and (n + 2) are not divisible by 3.
Only n is divisible by 3.
Case II:
When n = 3q + 1, then n + 2 = 3q + 3 = 3(q + 1) is divisible by 3
n and (n + 1) are not divisible by 3.
Only (n + 2) is divisible by 3.
Case III:
When n - 3q + 2, then n + 1 = 3q + 3 = 3(q + 1) is divisible by 3
n and (n + 2) are not divisible by 3.
Only (n + 1) is divisible by 3.
Therefore, one of any three consecutive positive integers is divisible by 3.
Hence Proved.
Q.4. For any positive integer n, prove that n3 – n is divisible by 6.
Consider a = n3 - n.
a = n - (n2 - 1)
a = n - (n - 1)(n + 1)
Using the algebraic identity (a2 - b2) = (a - b)(a + b)
a = (n - 1) n (n + 1).
If a number is divisible by both 2 and 3, then it is divisible by 6.
Three consecutive integers are n - 1, n and n + 1.
a = (n - 1)n (n + 1) is a product of three consecutive integers.
One among these should be divisible by 2 and the other one should be divisible by 3.
a is divisible by both 2 and 3.
Therefore, a = n3 - n is divisible by 6
Hence Proved.
Q.5. Show that one and only one out of n, n + 4, n + 8, n + 12 and n + 16 is divisible by 5, where n is any positive integer.
By dividing n by 5,
Consider q as the quotient and r as the remainder.
Now n = 5q + r, where 0 ≤ r < 5
n = 5q + r, where r = 0, 1, 2, 3, 4
n = 5q or 5q + 1 or 5q + 2 or 5q + 3 or 5q + 4.
Case I:
When n = 5q,
then only n is divisible by 5.
Case II:
When n = 5q + 1, then n + 4 = 5q + 1 + 4 = 5q + 5 = 5(q + 1) which is divisible by 5
Only (n + 4) is divisible by 5.
Case III:
When n = 5q + 2, then
n + 8 = 5q + 10 = 5 (q + 2) which is divisible by 5.
Only (n + 8) is divisible by 5.
Case IV :When n = 5q + 3,
n + 12 = 5q + 3 + 12 = + 15 = 5(q + 3) is divisible by 5
Only (n + 12) is divisible by 5.
Case V :When n = 5q + 4,
n + 16 = 5q + 4 + 16
= 5q + 20
= 5(q + 4) is divisible by 5.
Only (n + 16) is divisible by 5.
Therefore, one and only one out of n, n + 4, n + 8, n + 12 and n + 16 is divisible by 5, where n is any positive integer.
Hence Proved.
|
129 videos|736 docs|84 tests
|
FAQs on NCERT Exemplar: Real Numbers - Mathematics (Maths) Class 10
| 1. What are real numbers and how are they classified? |  |
| 2. How do you find the HCF (Highest Common Factor) of two numbers? |  |
| 3. What is the difference between rational and irrational numbers? |  |
| 4. What are some properties of real numbers that are often used in calculations? |  |
| 5. How can we express a decimal number as a fraction? |  |