Class 10 Maths Chapter 11 Previous Year Questions - Areas Related to Circles
Previous Year Questions 2024
Q1: Perimeter of a sector of a circle whose central angle is 90º and radius 7 cm is: (CBSE 2024)(a) 35 cm
(b) 11 cm
(c) 22 cm
(d) 25 cm
 View Answer
View AnswerAns: (d)
Perimeter of sector 

Q2: A stable owner has four horses. He usually ties these horses with 7 m long rope to pegs at each corner of a square-shaped grass field of 20 m length, to graze in his farm. But tying with rope sometimes results in injuries to his horses, so he decided to build a fence around the area so that each horse can graze. (CBSE 2024)

Based on the above, answer the following questions:
(A) Find the area of the square-shaped grass field.
(B) Find the area of the total field in which these horses can graze.
OR
If the length of the rope of each horse is increased from 7 m to 10 m, find the area grazed by one horse.
(Use π = 3.14)
(C) What is area of the field that is left ungrazed, if the length of the rope of each horse is 7 m?
 View Answer
View AnswerAns:
(A) Area of square shaped field
= 20 × 20
= 400 sq. m.
(B) Area of 4 quadrant = area of a. circle of radius 7m = πr2
OR
New radius = 10 m
So, area grazed by one horse = 

(C) Area of ungrazed portion = Area of square field – Area of circle with radius 7 m
Previous Year Questions 2023
Q3: What is the area of a semi-circle of diameter 'd'? (CBSE 2023)(a) 1/16πd2
(b) 1/4πd2
(c) 1/8πd2
(d) 1/2πd2
 View Answer
View AnswerAns: (c)
Given diameter of semi circle = d

∴ Radius, r = d/2
Area of semi circle
= 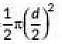
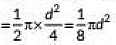
Q4: Case Study: The Governing council of a local public development authority of Dehradun decided to build an adventurous playground on the top of a bill, which will have adequate space for parking.

After the survey, it was decided to build a rectangular playground, with a semi-circular area allotted for parking at one end of the playground. The length and breadth of the rectangular playground are 14 units and 7 units, respectively. There are two quadrants of radius 2 units on one side for special seats. Based on the above information, answer the following questions:
(i) What is the total perimeter of the parking area?
(ii) (a) What is the total area of parking and the two quadrants?
(b) What is the ratio of area of playground to the area of parking area ?
(iii) Find the cost of fencing the playground and parking area at the rate of ? 2 per unit. (CBSE 2023)
 View Answer
View AnswerAns: (i) Length of play ground . AB = 14 units, Breadth of play ground. AD = 7 units
Radius of semi - circular part is 7/2 units
Total perimeter of parking area = πr + 2r

= 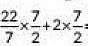
= 11 + 7 = 18 Units
(ii) (a): Area of parking = πr2 / 2
= 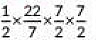
= 19.25 sq. units
Area of two quadrants (I) a n d [II) =1/2 x 1/4 x πr2
= 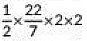
= 6.29 sq. units
Total area of parking and two quadrant
= 19.25 + 6.29
= 25.54 sq. units
Q5: A chord of a circle of radius 14 cm subtends an angle of 60° at the centre. Find the area of the corresponding minor segment of the circle. Also, find the area of the major segment of the circle. (2023)
 View Answer
View AnswerAns: Here, radius t(r) = 14 cm and Sector angle (θ) = 60°
∴ Area of the sector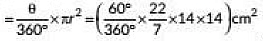
= 102.67 cm2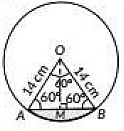
Since ∠O = 60° and OA = OB = 14 cm
∴ AOB is an equilateral triangle.
⇒ AS = 14 cm and ∠A = 60°
Draw OM ⊥ AB.
In ΔAMO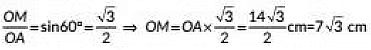
Now,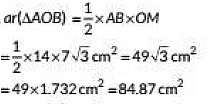
Now, area of the minor segment= (Area of minor sector) - (ar ΔAOB)
= 102.67 - 84.87 cm2
= 17.8 cm2
Area of the major segment
= Area of the circle - Area of the minor segment 
= (616 - 17.8) cm2 = 598.2 cm2
Previous Year Questions 2022
Q6: The area swept by 7 cm long minute band of a clock in 10 minutes is (CBSE 2022)
(a) 77 cm2
(b)

(c)
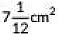
(d)
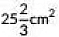
 View Answer
View AnswerAns: (d)
Angle formed by minute hand of a clock in 60 minutes = 360°
∴ Angle formed by minute hand of a clock in 10 minutes = 10/60 x 360° = 60°
Length of minute hand of a dock = radius = 7 cm
∴ Required area
= 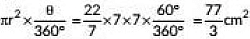
= 
Q7: Given below is the picture of the Olympic rings made by taking five congruent circles of radius 1 cm each, intersecting in such a way that the chord formed by joining the point of intersection of two circles is also of length 1 cm. The total area of all the dotted regions assuming the thickness of the rings to be negligible is (2022)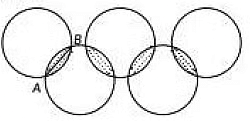 (a)
(a) 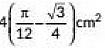
(b) 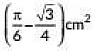
(c) 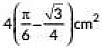
(d) 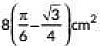
 View Answer
View AnswerAns: (d)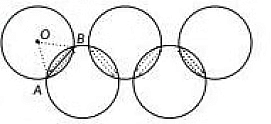
Let O be the centre of the circle. So. OA = OB = AB = 1 cm
So ΔOAB is an equilateral triangle.
∴ ∠AOB = 60°
∴ Required area = 8 x area of one segment with r = 1 cm,θ = 60°
= 8 x (area of sector - area of ΔAOB)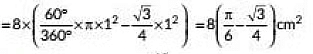
Previous Year Questions 2020
Q8: A piece of wire 22 cm long is bent into the form of an arc of a circle subtending an angle of 60° at its centre. Find the radius of the circle. [Use π = 22/7] (2020) View Answer
View AnswerAns: Let AB be the wire of length 22 cm in the form of an art of a circle so blending an ∠AOB - 60° at centre O.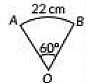
∵ Length of arc = 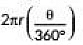
⇒ 
= 21 cm
Hence, radius of the circle is 21cm.
Q9: A circular park is surrounded by a road 21 m wide. If the radius of the park is 105 m, find the area of the road. (CBSE 2020)
 View Answer
View AnswerAns:

Given: Width of road = 21 m
Radius of park, r1 = 105 m
⇒ Radius of the whole circular portion (park + road)
r2 = 105 + 21 = 126 m
So, Area of road = Area of park and road – Area of park 
Hence, the area of the road is 15246 m2.
Previous Year Questions 2019
Q10: A car has two wipers which do not overlap. Each wiper has a blade of length 21 cm sweeping through an angle 120°. Find the total area cleaned at each sweep of the blades. (Take π = 22/7) (2019)
 View Answer
View AnswerAns: Here radius (r) = 21 cm
5ector angle (θ) = 120°
∴ Area cleaned by each sweep of the blades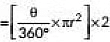 [∵ there are 2 blades]
[∵ there are 2 blades]
= 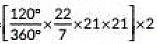
= 22 x 7 x 3 x 2 cm2
= 924 cm2
Q11: Find the area of the segment shown in the given figure, if radius of the circle is 21 cm and ∠AOB = 120°. (Take π = 22/7) (2019)
 View Answer
View AnswerAns: Given. O is the centre of the circle of radius 21cm and AB is the chord that subtends an angle of 120° at the centre.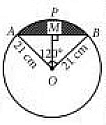
Draw OM ⊥ AB,
Area of the minor segment AMBP = Area of sector OAPB - Area of ΔAOB
Now, area of sector OAPB
= 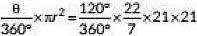
= 462 cm2
Since, OM ⊥ AB.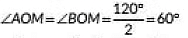 [∵ Perpendicular from the centre to the chord bisects the angle subtended by the chord at the centre.]
[∵ Perpendicular from the centre to the chord bisects the angle subtended by the chord at the centre.]
In ΔAOM, sin60° = AM/AO, cos60° = OM/OA
⇒ 
⇒ 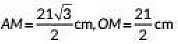
∵ 
Area of ΔAOB =
Hence, Required Area = 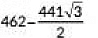
= 462 - 381.92= 80.08 cm2
Q12: In the given figure, three sectors of a circle of radius 7 cm, making angles of 60°, 80° and 40° at the centre are shaded. Find the area of the shaded region. (2019)
 View Answer
View AnswerAns: Radius (r) of circle = 7 cm
Area of shaded region =

= 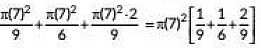
= 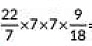
= 77 cm2
Previous Year Questions 2017
Q13: A chord PQ of a circle of radius 10 cm subtends an angle of 60° at the centre of the circle. Find the area of major and minor segments of the circle. (Delhi 2017) View Answer
View AnswerAns: Radius of the circle = 10 cm
Central angle subtended by chord AB = 60°
Area of minor sector OACB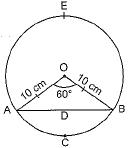
Area of equilateral triangle OAB formed by radii and chord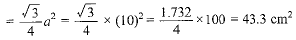
∴ Area of minor segment ACBD
= Area of sector OACB - Area of triangle OAB
= (52.38 - 43.30) cm2 = 9.08 cm2
Area of circle = πr2 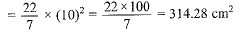
∴ Area of major segment ADBE
= Area circle - Area of minor segment
= (314.28 - 9.08) cm2 = 305.20 cm2
Q14: In the given figure, ΔABC is a right-angled triangle in which ∠A is 90°. Semi circles are drawn on AB, AC and BC as diameters. Find the area of the shaded region. (Al 2017)
 View Answer
View AnswerAns: In right triangle ABC.
AB2 + AC2 = BC2
⇒ (3)2 + (4)2 = BC2 ⇒ 9 + 16 = BC2 ⇒ 25 = BC2
∴ BC = 5 cm
Now, Area of shaded region = Area of semicircle on side AB + area of semicircle on side AC - area of semicircle on side BC + area of ΔABC
Now, Area o f semicircle on side AB 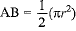
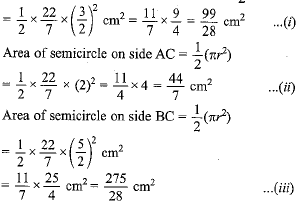
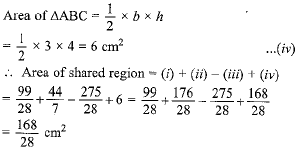
Hence area of the shaded region = 6 cm2
Q15: In Fig. 12.51, O is the centre of the circle with AC = 24 cm , AB = 7 cm and ∠BOD = 90°. Find the area of the shaded region. (CBSE (AI) 2017)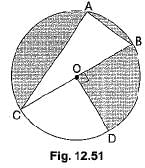
 View Answer
View AnswerAns: In right angle triangle ABC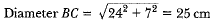
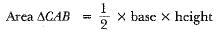
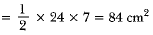
Area of shaded region = area of semicircle - area of ΔCAB + area of quadrant BOD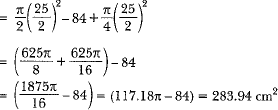
Q16: Two circles touch internally. The sum of their areas is 116π cm2 and the distance between their centres is 6 cm. Find the radii of the circles. (CBSE 2017)
 View Answer
View AnswerAns: Let ‘r’ and ‘R’ be the radii of the smaller and bigger circles, respectively.
Then, OO’ = R – r = 6 cm [Given] ...(i)
Also, sum of their areas = 116π cm2
i.e., πR2 + πr2 = 116π
 ⇒ R2 + r2 = 116 ...(ii)
⇒ R2 + r2 = 116 ...(ii)
We know, (R – r)2 = R2 + r2 – 2Rr
⇒ (6)2 = 116 – 2Rr
⇒ 2Rr = 116 – 36 = 80
⇒ Rr = 40 ...(iii)
Also, (R + r)2 = R2 + r2 + 2Rr = 116 + 2 × 40 [Using (ii) and (iii)]
⇒ (R + r)2 = 196
⇒ R + r = 14 ...(iv)
Now, adding equations (i) and (iv), we get
2R = 20
⇒ R = 10 cm
Putting the value of R in equation (i), we get
r = 4 cm
Hence, the radii of the bigger and smaller circles are 10 cm and 4 cm, respectively.
Previous Year Questions 2016
Q17: In Fig. 12.34, O is the centre of a circle such that diameter AB =13 cm and AC = 12 cm. BC is joined. Find the area of the shaded region. (Take k = 3.14) (CBSE (AI) 2016)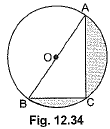
 View Answer
View AnswerAns: In ΔABC, ∠ACB = 90° (Angle in the semicircle)
∴ BC2 + AC2 = AB2
∴ BC2 = AB2 -AC2
= 169 - 144 = 25
∴ BC = 5 cm
Area of the shaded region = area of semicircle - area of right AABC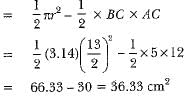
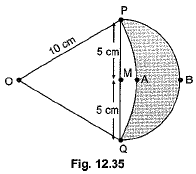
Q18: In Fig. 12.35, are shown two arcs PAQ and PBQ. Arc PAQ is a part of the circle with centre 0 and radius OP while arc PBQ is a semi-circle drawn on PQ as diameter with centre M.
If OP = PQ = 10 cm show that the area of the shaded region is 25 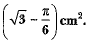 (CBSE (Delhi) 2016)
(CBSE (Delhi) 2016)
 View Answer
View AnswerAns: Since OP = PQ = QO
⇒ APOQ is an equilateral triangle
∴ ∠POQ = 60°
Area of segment PAQM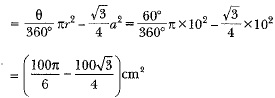
Area of semicircle with M as centre 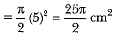
Area of shaded region 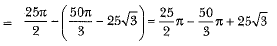
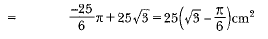
Q19: In the figure, the boundary of the shaded region consists of four semicircular arcs, two smallest being equal. If the diameter of the largest is 14 cm and that of the smallest is 3.5 cm, calculate the area of the shaded region. 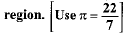 (Foreign 2016)
(Foreign 2016)
 View Answer
View AnswerAns: Given AD = 14 cm, AB = CD = 3.5 cm
∴ BC = 7 cm Area of shaded region
Area of shaded region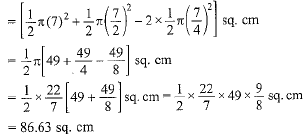
Q20: Find the area of the shaded region in Fig. 12.23, where a circular arc of radius 6 cm has been drawn with vertex 0 of an equilateral triangle ΔOAB of side 12 cm as the centre. (NCERT, CBSE (F) 2016)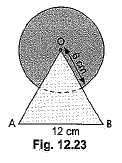
 View Answer
View AnswerAns: We have, radius of circular region = 6 cm and each side of ΔOAB = 12 cm.
∴ Area of the circular portion
= area of circle - area of the sector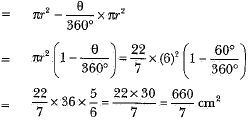
Now, area of the equilateral triangle OAB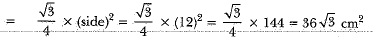
∴ Area of shaded region = area of circular portion + area of equilateral triangle OAB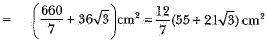
Q21: An elastic belt is placed around the rim of a pulley of radius 5 cm. (Fig. 12.46). From one point C on the belt, the elastic belt is pulled directly away from the centre O of the pulley until it is at P, 10 cm from the point O. Find the length of the belt that is still in contact with the pulley. Also, find the shaded area. (Use π = 3.14 and √3 = 1.73) (CBSE Delhi 2016)
 View Answer
View AnswerAns: 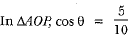
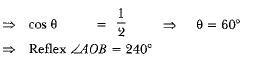

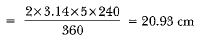
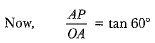
PA = 5√S cm = BP (Tangents from an external point are equal)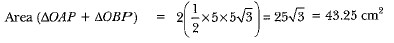
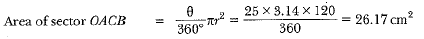
Shaded area = 43 .25 - 26 .17 = 17.08 cm2
Q22: In Fig. 12.47, a sector OAP of a circle with centre O, containing angle 0. AB is perpendicular to the radius OA and meets OP produced at B. Prove that the perimeter of the shaded region is 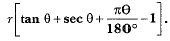 (CBSE (AI) 2016)
(CBSE (AI) 2016)
 View Answer
View AnswerAns: 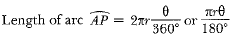
In right ΔAOB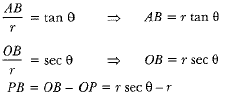
Perimeter of shaded region 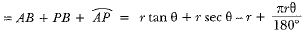
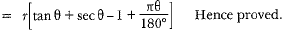
Q23: In the figure, AB is a chord of a circle with a centre O and radius 10 cm, that subtends a right angle at the centre of the circle. Find the area of the minor segment AQBP. Also, find the area of the major segment ALBQA. (Use π = 3.14) (CBSE 2016)

 View Answer
View AnswerAns: Given, A circle of radius (r) = 10 cm in which ∠AOB = 90º.
Area of the minor segment AQBP = Area of sector OAPB – Area of ΔAOB
= θ / 360° × πr2 – 1 /2 × OA × OB
= 90 / 360 ° × 3.14 × 10 × 10 – 1/ 2 × 10 × 10
= 3.14 × 5 × 5 – 5 × 10
= 78.5 – 50
= 28.5 cm2
So, Area of the minor segment AQBP = 28.5 cm2
Area of the major segment ALBQA = Area of circle – Area of minor segment AQBP
= 3.14 × (10)2 – 28.5
= 314 – 28.5
= 285.5 cm2
Area of major segment ALBQA = 285.5 cm2. Hence, the area of the minor segment AQBP is 28.5 cm2 and the area of the major segment ALBQA is 285.5 cm2.
Previous Year Questions 2015
Q24: In figure, ABCD is a trapezium with AB || DC, AB = 18 cm, DC = 32 cm and distance between AB and DC = 14 cm. If arcs of equal radii 7 cm with centres A, B, C and D have been drawn, then find the area of the shaded region. (Foreign 2015) View Answer
View AnswerAns: Area of shaded region = area of trapezium - (area of 4 sectors)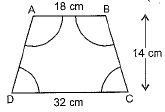
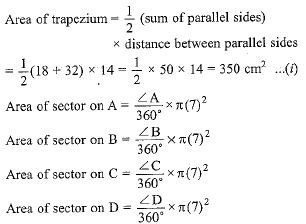
Total area of all sector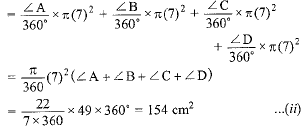
[Sum of angles of a quadrilateral is 360°]
From (i) and (ii),
Area of shaded region = 350 - 154 = 196 cm2
|
126 videos|457 docs|75 tests
|
FAQs on Class 10 Maths Chapter 11 Previous Year Questions - Areas Related to Circles
| 1. What is the formula for the area of a circle? |  |
| 2. How do you find the circumference of a circle? |  |
| 3. What is the difference between radius and diameter in circles? |  |
| 4. How do you calculate the area of a sector of a circle? |  |
| 5. What is the relationship between the radius, diameter, and circumference of a circle? |  |

|
Explore Courses for Class 10 exam
|

|


















