JEE Main Previous Year Questions (2016- 2024): Conic Sections | Mathematics for Airmen Group X - Airforce X Y / Indian Navy SSR PDF Download

Q.1. If the distance between the foci of an ellipse is 6 and the distance between its directrices is 12, then the length of its latus rectum is    (2020)
(1) √3
(2) 3√2
(3) 3/√2
(4) 2√3
Ans. (2)
Solution. The distance between the foci of an ellipse is
2ae = 6 ⇒ ae = 3 ...(1)
The distance between ellipse directrices is
2a/e = 12 ⇒ a = 6e ...(2)
On solving Eqs. (1) and (2), we get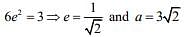

Now,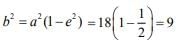
Hence, the length of latus rectum is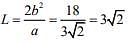


Q.2. If y = mx + 4 is a tangent to both the parabolas y² = 4x and x² = 2by, then b is equal to   (2020)
(1) −32
(2) −64
(3) −128
(4) 128
Ans. (3)
Solution. The equation of tangent of parabola y² = 4ax is given by
y = mx + a/m ...(1)
Now, y = mx + 4 is a tangent to the parabola y² = 4x, then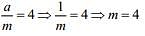

Therefore, the equation of tangent is y = 4x + 1/4. It is also the tangent of parabola x2 = 2by, then

The value of b cannot be zero, hence b = −128.

Q.3. If 3x + 4y = 12√2 is a tangent to the ellipse 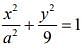 for some 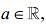then the distance between the foci of the ellipse is    (2020)
(1) 2√7
(2) 4
(3) 2√5
(4) 2√2
Ans. (1)
Solution. The given tangent of ellipse 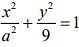is

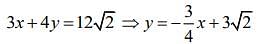
The line y = mx + c will be a tangent of ellipse 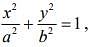 if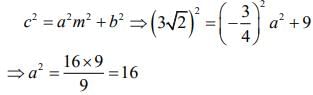

Now, the foci of ellipse is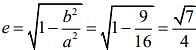
Hence, the distance between the foci of the ellipse is
2ae = 2 x 4 x √7/4 = 2√7

Q.4. Let the line y = mx and the ellipse 2x² + y² = 1 intersect at a point P in the first quadrant. If the normal to this ellipse at P meets the co-ordinate axes at 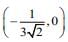 and (0, β), then β is equal to    (2020)
(1) (2√2)/3
(2) 2/(√3)
(3) 2/3
(4) (√2)/3
Ans. (2)
Solution. Let the coordinates of point P be (x₁, y₁). So, the equation of normal at point P is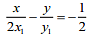
...(1)
It passes through the point 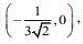 then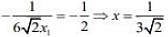

Now,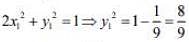
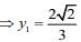 (since P lies in first quadrant)
The normal also passes through the point(0, β), then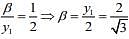


Q.5. The locus of a point which divides the line segment joining the point (0, −1) and a point on the parabola x² = 4y, internally in the ratio 1 : 2 is    (2020)
(1) 9x² - 12y = 8
(2) 9x² - 3y = 2
(3) x² - 3y = 2
(4) 4x² - 3y = 2
Ans. (1)
Solution. Let the point on parabola x² = 4y be (2t, t²). Therefore,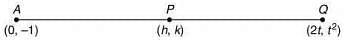

Now,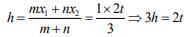
 ...(1)
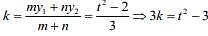 ...(2)
From Eqs. (1) and (2), we get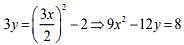


Q.6. If a hyperbola passes through the point P (10, 16) and it has vertices at (±6, 0), then the equation of the normal to it at P is    (2020)
(1) 3x + 4y = 94
(2) 2x + 5y = 100
(3) x + 2y = 42
(4) x + 3y = 58
Ans. (2)
Solution. Let the equation of hyperbola is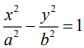

The coordinates of vertices are (±a, 0) = (±6, 0) ⇒ a = 6.
The equation of hyperbola is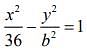

Now, hyperbola passes through the point P (10, 16), then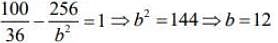

Therefore, the equation of hyperbola is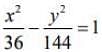

Now, the equation of normal at point P is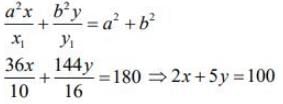


Q.7. If a line y = mx + c is a tangent to the circle (x - 3)² + y² = 1 and it is perpendicular to a line L₁, where L₁ is the tangent to the circle x² + y² = 1 at the point 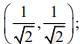 then    (2020)
(1) c² - 7c + 6 = 0
(2) c² + 7c + 6 = 0
(3) c² + 6c + 7 = 0
(d) c² - 6c + 7 = 0
Ans. (3)
Solution.

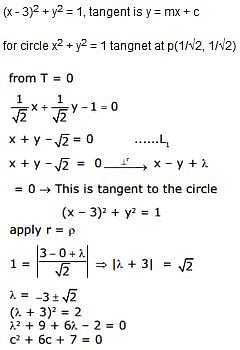
Q.8. If e₁ and e₂ are the eccentricities of the ellipse 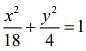 and the hyperbola  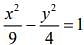respectively and (e₁, e₂) is a point on the ellipse 15x² + 3y² = k, then k is equal to    (2020)
(1) 16
(2) 17
(3) 15
(4) 14
Ans. (1)
Solution. The eccentricity of ellipse 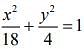is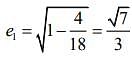
 ...(1)
The eccentricity of hyperbola 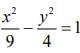 is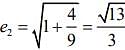
 ...(2)
Point (e₁, e₂) lies on the ellipse 15x² + 3y² = k, then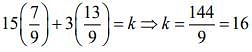


Q.9. The length of the minor axis (along y-axis) of an ellipse in the standard form is 4/(√3). If this ellipse touches the line x + 6y = 8; then its eccentricity is    (2020)
(1)
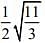
(2)
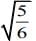
(3)
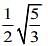
(4)
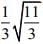
Ans. (1)
Solution. The length of the minor axis (along y-axis) of ellipse is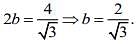

The line x + 6y = 8 touches the ellipse and the equation of tangent of ellipse is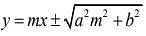
 ...(1)
and 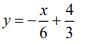...(2)
Comparing Eqs. (1) and (2), we get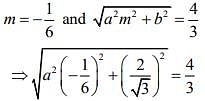

The eccentricity of ellipse is


Q.10. If one end of a focal chord AB of the parabola y² = 8x is at 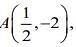 then the equation of the tangent to it at B is    (2020)
(1) 2x + y - 24 = 0
(2) x - 2y + 8 = 0
(3) x + 2y + 8 = 0
(4) 2x - y - 24 = 0
Ans. (2)
Solution. Let the coordinates of point A be (at², 2at) and y² = 8x ⇒ a = 2.
Therefore,
Now, t₁.t₂ = -1 ⇒ t₂ = 2
Therefore, coordinates of other point of focal chord is (8,8). Hence, the equation of tangent at point B is
8y = 4(x + 8) ⇒ 2y = x + 8 ⇒ x - 2y + 8 = 0

Q.11. If tangents are drawn to the ellipse x² + 2y² - 2 at all points on the ellipse other than its four vertices then the mid points of the tangents intercepted between the coordinate axes lie on the curve:    (2019)
(1)
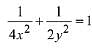
(2)
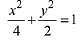
(3)
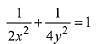
(4)
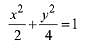
Ans. (3)
Solution. Given the equation of ellipse,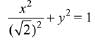




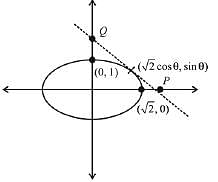
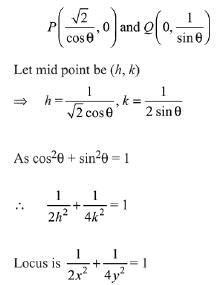
Q.12. Let the length of the latus rectum of an ellipse with its major axis along x-axis and centre at the origin, be 8. If the distance between the foci of this ellipse is equal to the length of its minor axis, then which one of the following points lie on it?    (2019)
(1)
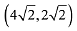
(2)
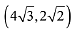
(3)
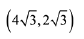
(4)
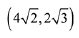
Ans. (2)
Solution.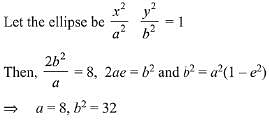


Q.13. Let S and S' be the foci of an ellipse and B be any one of the extremities of its minor axis. If ΔS'BS is a right angled triangle with right angle at B and area (ΔS'BS) = 8 sq. units, then the length of a latus rectum of the ellipse is:    (2019)
(1) 4    
(2) 2√2
(3) 4√2    
(4) 2
Ans. (1)
Solution. 
∵ ΔS'BS is right angled triangle, then
(Slope of 55) x (Slope of BS') = - 1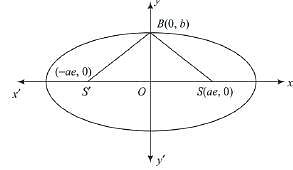


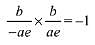
b² = a²e²   ...(1)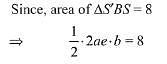

b² = 8   ...(2)
From eqⁿ (1)
a²e² = 8
Also,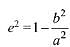

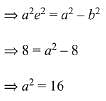
Hence, required length of latus rectum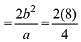
= 4 units

Q.14. If the tangents on the ellipse 4x² +y² = 8 at the points (1,2) and (a, b) are perpendicular to each other, then a² is equal to:    (2019)
(1) 128/17
(2) 64/17
(3) 4/17
(4) 2/17
Ans. (4)
Solution.
Since (a,b) touches the given ellipse 4x² + y² = 8
∴ 4a² + b² = 8   ...(1)
Equation of tangent on the ellipse at the point A (1,2) is: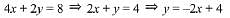

But, also equation of tangent at P(a, b) is: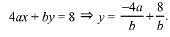

Since, tangents are perpendicular to each other.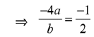

⇒ b = 8a   ...(2)
From (1) & (2) we get: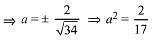


Q.15. In an ellipse, with centre at the origin, if the difference of the lengths of major axis and minor axis is 10 and one of the foci is at 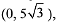 then the length of its latus rectum is:    (2019)
(1) 10    
(2) 5    
(3) 8    
(4) 6
Ans. (2)
Solution.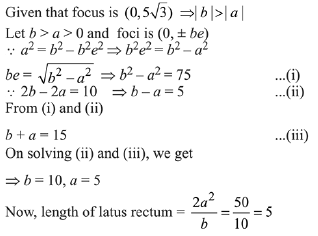


Q.16. If the line x - 2y = 12 is tangent to the ellipse 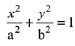 at the point  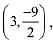, then the length of the latus rectum of the ellipse is:    (2019)
(1) 9    
(2)  
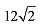
(3) 5    
(4)
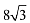
Ans. (1)
Solution.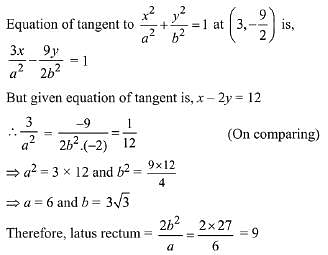


Q.17. The tangent and normal to the ellipse 3x² + 5y² = 32 at the point P(2,2) meet the x-axis at Q and R, respectively. Then the area (in sq. units) of the triangle PQR is:    (2019)
(1) 34/15
(2) 14/3
(3) 16/3
(4) 68/15
Ans. (4)
Solution.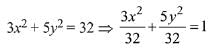


Tangent on the ellinse at P is


Q.18. If the normal to the ellipse 3x² + 4y² = 12 at a point P on it is parallel to the line, 2x + y = 4 and the tangent to the ellipse at P passes through Q (4,4), then PQ is equal to:    (2019)
(1)
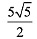
(2)
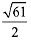
(3)
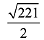
(4)
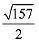
Ans. (1)
Solution. Slope of tangent on the line 2x +y = 4 at point P is 1/2
Given ellipse is,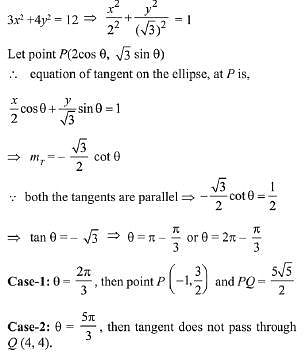


Q.19. An ellipse, with foci at (0, 2) and (0, -2) and minor axis of length 4, passes through which of the following points ?    (2019)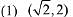




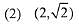
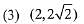
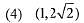
Ans. (1)
Solution. Let the equation of ellipse: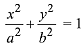

Given that length of minor axis is 4 i.e. a = 4. Also given be = 2


Q.20. Axis of a parabola lies along x-axis. If its vertex and focus are at distance 2 and 4 respectively from the origin, on the positive x-axis then which of the following points does not lie on it?    (2019)




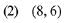
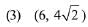
Ans. (2)
Solution. Since, vertex and focus of given parabola is (2, 0) and (4, 0) respectively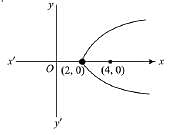

Then, equation of parabola is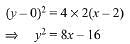

Hence, the point (8, 6) does not lie on given parabola.

Q.21. If θ denotes the acute angle between the curves, y = 10 - x² and y = 2 + x² at a point of their intersection, then |tan θ| is equal to:    (2019)
(1) 4/9
(2) 8/15
(3) 7/17
(4) 8/17
Ans. (2)
Solution. Since, the equation of curves are


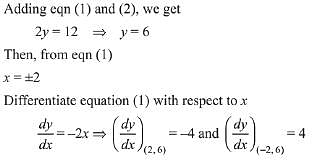
Differentiate equation (2) with respect to x


Q.22. Two cards are drawn successively with replacement from a well-shuffled deck of 52 cards. Let X denote the random variable of number of aces obtained in the two drawn cards. Then P(X = 1) + P(X = 2) equals:    (2019)
(1) 49/169   
(2) 52/169
(3) 24/169    
(4) 25/169
Ans. (4)
Solution. X = number of aces drawn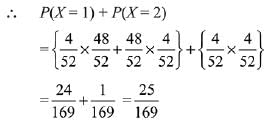


Q.23. Let A (4, -4) and B (9, 6) be points on the parabola, y² = 4x. Let C be chosen on the arc AOB of the parabola, where O is the origin, such that the area of ΔACB is maximum. Then, the area (in sq. units) of ΔACB, is:    (2019)


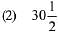
(3) 32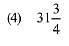

Ans. (1)
Solution.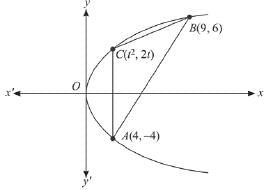

Let the coordinates of C is (t², 2t).
Since, area of ΔACB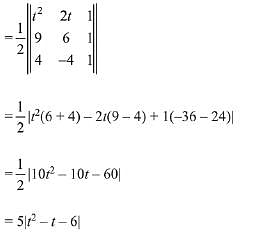




Q.24. If the parabolas y² = 4b(x - c) and y² = 8ax have a common normal, then which one of the following is a valid choice for the ordered triad (a, b, c)?    (2019)
(1) (1/2,2,3)    
(2) (1,1,3)
(3) (1/2,2,0)
(4) (1,1,0)
Ans. (1,2,3,4)
Solution.

and normal to y² = 4b(x- c) with slope m is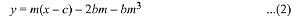

Since, both parabolas have a common normal.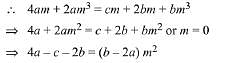

or (X-axis is common normal always)

Since, x-axis is a common normal. Hence all the options are correct for m = 0.

Q.25. The length of the chord of the parabola x² = 4y having equation 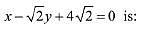    (2019)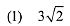




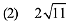
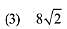
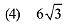
Ans. (4)
Solution. Let intersection points be P(x₁,y₁) and Q(x₂,y₂)
The given equations
x² =4y   ...(1)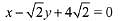
   ...(2)
Use eqn (1) in eqn (2)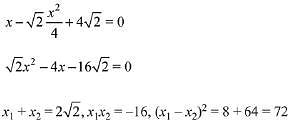

Since, points P and Q both satisfy the equations (2), then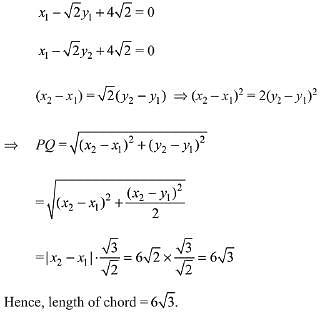


Q.26. If the area of the triangle whose one vertex is at the vertex of the parabola, y² + 4(x - a²) = 0 and the other two vertices are the points of intersection of the parabola and y-axis, is 250 sq. units, then a value of ‘a’ is:    (2019)
(1)     
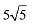
(2) 5(2^1/3)
(3) (10)^2/3    
(4) 5
Ans. (4)
Solution. y² = -4(x - a²)



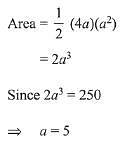
Q.27. The equation of a tangent to the parabola, x² = 8y, which makes an angle θ with the positive direction of x-axis, is:    (2019)
(1) y = x tanθ + 2 cotθ
(2) y = x tanθ - 2 cotθ
(3) x = y cotθ + 2 tanθ
(4) x = y cotθ - 2 tanθ
Ans. (3)
Solution. x² = 8y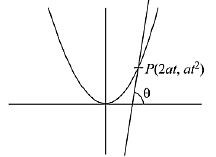

Then, equation of tangent at P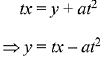



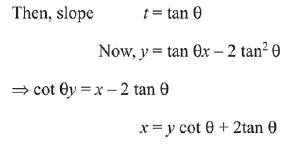
Q.28. The tangent to the parabola y² = 4x at the point where it intersects the circle x² + y²  = 5 in the first quadrant, passes through the point:    (2019)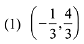




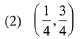
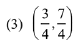
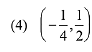
Ans. (3)
Solution. To find intersection point of x² + y² = 5 and y² = 4x,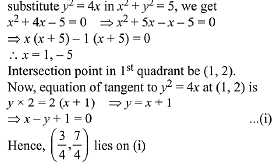


Q.29.If one end of a focal chord of the parabola, y² = 16x is at (1,4), then the length of this focal chord is:    (2019)
(1) 25    
(2) 22    
(3) 24    
(4) 20
Ans. (1)
Solution.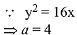

One end of focal of the parabola is at (1,4)
∵ y - coordinate of focal chord is 2at
∴ 2 at = 4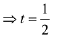

Hence, the required length of focal chord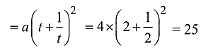


Q.30. The area (in sq. units) of the smaller of the two circles that touch the parabola, y² = 4x at the point (1,2) and the x - axis is:    (2019)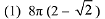




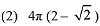
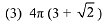
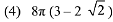
Ans. (4)
Solution. The circle and parabola will have common tangent at P (1, 2).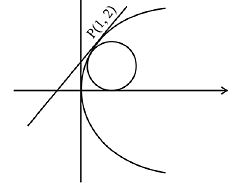

So, equation of tangent to parabola is,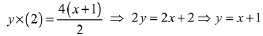

Let equation of circle (by family of circles) is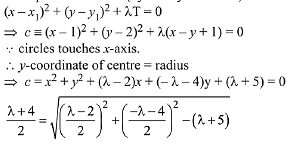



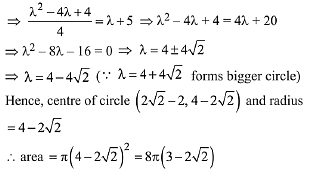
Q.31. If the tangent to the parabola y² = x at a point (α, β), (β > 0) is also a tangent to the ellipse, x² + 2y² = 1, then a is equal to:    (2019)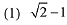




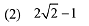
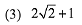
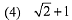
Ans. (4)
Solution. Let tangent to parabola at point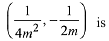  


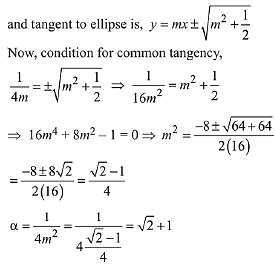
Q.32. If the line ax + y = c, touches both the curves x² + y² = 1 and 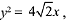, then |c| is equal to:    (2019)
(1) 2
(2)
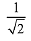
(3) 1/2
(4)
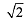
Ans. (4)
Solution.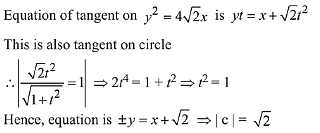


Q.33. Let P be the point of intersection of the common tangents to the parabola y² = 12x and hyperbola 8x² - y² = 8. If S and S' denote the foci of the hyperbola where S lies on the positive x-axis, then P divides SS' in a ratio:    (2019)
(1) 13 : 11    
(2) 14 : 13
(3) 5 : 4   
(4) 2 : 1
Ans. (3)
Solution. Equation of tangent to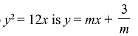
Equation of tangent to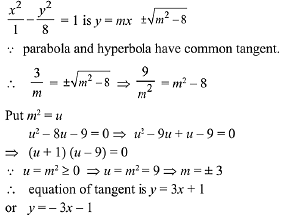


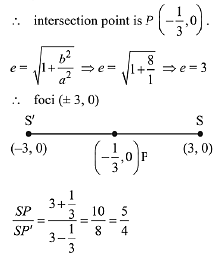
Q.34. If the eccentricity of the hyperbola 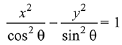is greater than 2, then the length of its latus rectum lies in the interval:    (2019)
(1) (3,∞)    
(2) (3/2,2]
(3) (2,3]    
(4) (1,3/2]
Ans. (1)
Solution.
∵ a² = cos²θ, b² = sin²θ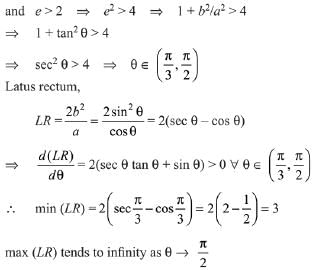

Hence, length of latus rectum lies in the interval (3, ∞)

Q.35. A hyperbola has its centre at the origin, passes through the point (4, 2) and has transverse axis of length 4 along the x - axis. Then the eccentricity of the hyperbola is:    (2019)
(1) 3/2
(2)
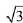
(3) 2
(4)
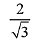
Ans. (4)
Solution.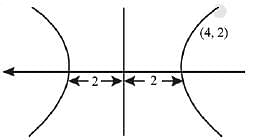

Consider equation of hyperbola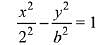

∵ (4, 2) lies on hyperbola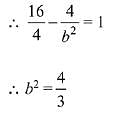



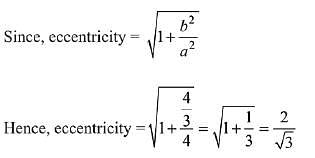
Q.36. The equation of a tangent to the hyperbola 4x² - 5y² = 20 parallel to the line x - y = 2 is:    (2019)
(1) x - y + 1 = 0    
(2) x - y + 7 = 0
(3) x - y + 9 = 0    
(4) x - y - 3 = 0
Ans. (1)
Solution. Given, the equation of line,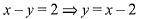




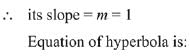
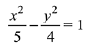
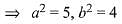
The equation of tangent to the hyperbola is,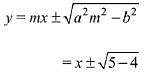



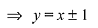
Q.37. Let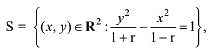
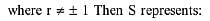    (2019)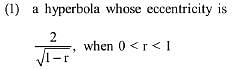




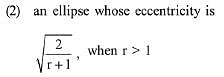
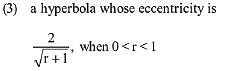
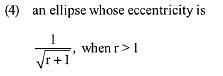
Ans. (2)
Solution. Since, 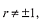 then there are two cases, when r > 1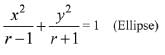

Then,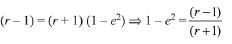


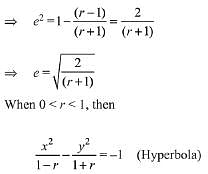
Then,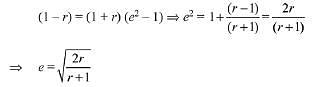


Q.38. Equation of a common tangent to the parabola y² = 4x and the hyperbola xy = 2 is:    (2019)
(1) x + y + 1 =0    
(2) x - 2y + 4 = 0
(3) x + 2y + 4 =0    
(4) 4x + 2y + 1=0
Ans. (3)
Solution. Equation of a tangent to parabola y² = 4x is: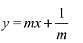

This line is a tangent to xy = 2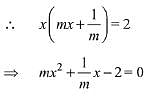

∵ Tangent is common for parabola and hyperbola.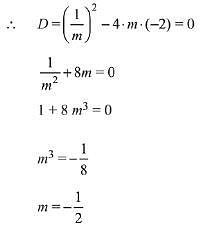



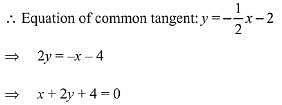
Q.39. If a hyperbola has length of its conjugate axis equal to 5 and the distance between its foci is 13, then the eccentricity of the hyperbola is:    (2019)
(1) 13/12
(2) 2
(3) 13/6
(4) 13/8
Ans. (1)
Solution.
∴ Conjugate axis = 5
∴ 2b = 5
Distance between foci =13
2ae = 13
Then, b² = a² (e² - 1)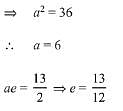


Q.40. If the vertices of a hyperbola be at (-2, 0) and (2, 0) and one of its foci be at (-3, 0), then which one of the following points does not lie on this hyperbola?    (2019)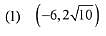




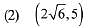
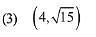
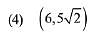
Ans. (4)
Solution. Let the points are,
 
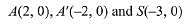
⇒ Centre of hyperbola is 0(0, 0)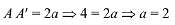

∵ Distance between the centre and foci is ae.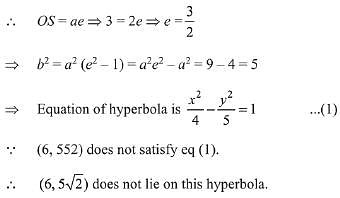


Q.41. If the eccentricity of the standard hyperbola passing through the point (4, 6) is 2, then the equation of the tangent to the hyperbola at (4, 6) is:    (2019)
(1) x - 2y + 8 = 0    
(2) 2x - 3y + 10 = 0
(3) 2x - y - 2 = 0    
(4) 3x - 2y = 0
Ans. (3)
Solution. Let equation of hyperbola be
    ...(i)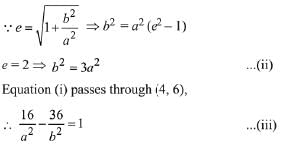

On solving (i) and (ii), we get
a² = 4, b² = 12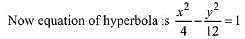

Now equation of tangent to the hyperbola at (4, 6) is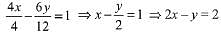


Q.42. If the line y = mx + 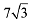 is normal to the hyperbola  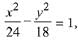then a value of m is:    (2019)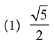




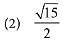
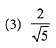
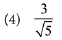
Ans. (3)
Solution. Since, 1x + my + n = 0 is a normal to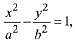  

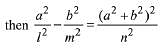
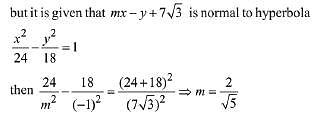
Q.43. If a directrix of a hyperbola centered at the origin and passing through the point  is 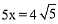 and its eccentricity is e, then:    (2019)
(1) 4e⁴ - 24e² + 27 = 0    
(2) 4e⁴ - 12e² - 27 = 0
(3) 4e⁴ - 24e² + 35 = 0    
(4) 4e⁴ + 8e² - 35 = 0
Ans. (3)
Solution.
∵ directrix of a hyperbola is,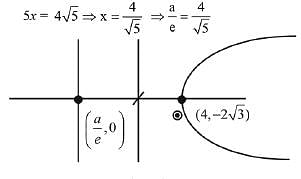



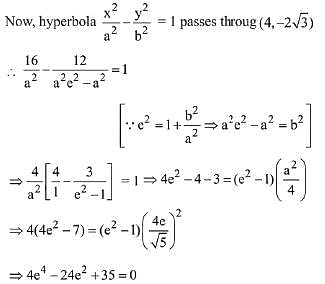
Q.44. If 5x + 9 = 0 is the directrix of the hyperbola 16x² - 9y² = 144, then its corresponding focus is:    (2019)
(1) (5,0)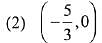


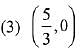
(4) (-5, 0)
Ans. (4)
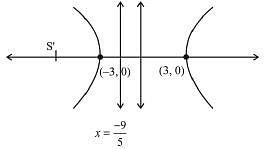
Solution.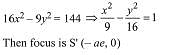 




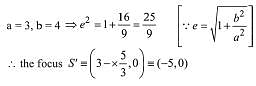
Q.45. If the curve y² = 6x, 9x² + by² = 16 intersect each other at right angles, then the value of b is:    (2018)
(1) 6
(2) 7/2
(3) 4
(4) 9/2
Ans. (4)
Solution. 
Let the point of intersection be (x₁, y₁) finding slope of both the curves at point of intersection
for y² = 6x, 9x² + by² = 16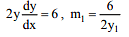



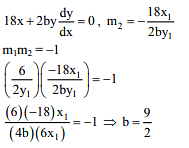
Q.46. Tangent and normal are drawn at P(16, 16) on the parabola y² = 16x, which intersect the axis of the parabola at A and B, respectively. If C is the centre of the circle through the points P, A and B and ∠CPB = θ, then a value of tanq is:    (2018)
(1) 1/2
(2) 2
(3) 3
(4) 4/3
Ans. (2)
Solution. The equation of tangent at P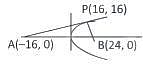


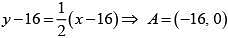
The normal is y = y – 16 = -2(x – 16)
B = (24, 0)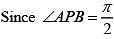

AB is the diameter
Centre of the circle C = (4, 0)
lope of PB = -2 = m₁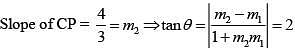 


Q.47. Tangents are drawn to the hyperbola 4x² -y²= 36 at the points P and Q. If these tangents intersect at the point T(0, 3) then the area (in sq. units) of ΔPTQ is:    (2018)
(1) 45√5
(2) 54√3
(3) 60√3
(4) 36√5
Ans. (1)
Solution. Equation of PQ,
4x (0) - 3y = 36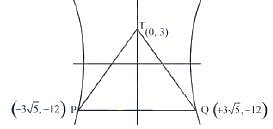



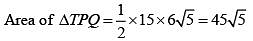
Q.48. If β is one of the angles between the normals to the ellipse, x² + 3y² = 9 at the points (3 cosθ, √3 sinθ) and (−3 sin θ√3 cosθ); θ ∈ (0, π/2); then 2cotβ/sin2θ is equal to:    (2018)
(1)
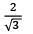
(2)
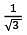
(3)
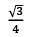
(4)
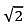
Ans. (1)
Solution.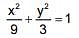


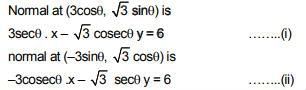
Angle between normal is β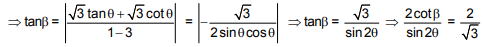


Q.49. If the tangents drawn to the hyperbola 4y² = x² + 1 intersect the co-ordinate axes at the distinct points A  and B, then the locus of the mid-point of AB is:    (2018)
(1) 4x² – y² + 16x²y² = 0
(2) x² – 4y² – 16x²y² = 0
(3) 4x² – y² + 16x²y² = 0
(4) 4x² – y² – 16x²y² = 0
Ans. (2)
Solution. 
Let tangent drawn at point (x, y) to the hyperbola 4y² = x² + 1 is : 4yy, = xx₁ + 1
This tangent intersect co-ordinate axes at A and B respectively then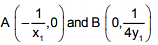
Let mid point is M (h,k) then of AB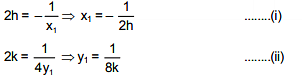

Since point P(x₁, y₁) lies on the hyperbola so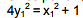

from (i) & (ii)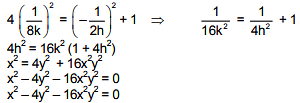

locus of M

Q.50. Two parabolas with a common vertex and with axes along x-axis and y-axis, respectively, intersect each other in the first quadrant. If the length of the latus rectum of each parabola is 3, then the equation of  the common tangent to the two parabolas is:    (2018)
(1) 8(2x + y) + 3 = 0
(2) 3(x + y) + 4 = 0
(3) 4(x + y) + 3 = 0
(4) x + 2y + 3 = 0
Ans. (3)
Solution. Equation two parabola are y² = 3x and x² = 3y
Let equation of tangent to y² = 3x is y = mx + 3/4m
is also tangent to x² = 3y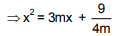

⇒ 4mx² – 12m² x – 9 = 0 have equal roots
⇒ D = 0
⇒ 144 m⁴ = 4(4m) (–9)
⇒ m⁴ + m = 0 ⇒ m = – 1
Hence common tangent is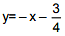
4(x + y) + 3 = 0

Q.51. If the length of the latus rectum of an ellipse is 4 units and the distance between a focus and its nearest vertex on the major axis is 3/2 units, then its eccentricity is:    (2018)
(1) 2/3
(2) 1/2
(3) 1/9
(4) 1/3
Ans. (4)
Solution.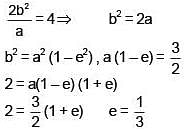


Q.52. The locus of the point of intersection of the lines, √2 x – y + 4√2 k = 0 and √2 kx + ky – 4 √2 = 0 (k is any non-zero real parameter), is    (2018)
(1) an ellipse whose eccentricity is 1/√3
(2) a hyperbola whose eccentricity is √3
(3) a hyperbola with length of its transverse axis 8√2
(4) an ellipse with length of its major axis 8√2
Ans. (3)
Solution.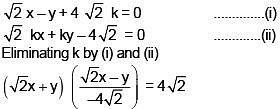



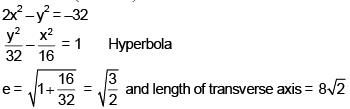
Q.53. Let P be a point on the parabola, x² = 4y. If the distance of P from the centre of the circle, x² + y² + 6x + 8 = 0 is minimum, then the equation of the tangent to the parabola at P, is:    (2018)
(1) x + y + 1 = 0
(2) x + 4y – 2 = 0
(3) x + 2y = 0
(4) x – y + 3 = 0
Ans. (1)
Solution. Let P(2t, t²) be any point on the parabola.
Centre of the given circle C = (-g,-f) = (-3,0)
For PC to be minimum, it must be the normal to the parabola at P.
Slope of line PC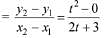
Also, slope of tangent to parabola at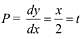
∴ Slope of normal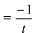

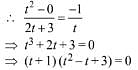
∴ Real roots of above equation is t= -1
Coordinate of P = (2t, t²) = (-2,1)
Slope of tangent to parabola at P = t = -1
Therefore, equation of tangent is:
(y-1) = (-1)(x+2)
⇒ x + y + 1 = 0

Q.54. The normal to the curve y (x - 2)(x - 3) = x + 6 at the point where the curve intersects the y-axis passes through the point    (2017)
(1)
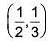
(2)
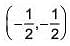
(3)
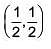
(4)
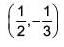
Ans. (3)
Solution. y (x - 2)( x - 3) = x + 6
At y-axis, x = 0, y = 1
Now, on differentiation.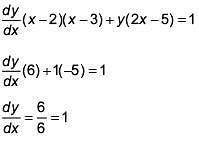

Now slope of normal = –1
Equation of normal  y – 1 = –1(x – 0)
y + x – 1 = 0 ... (i)
Line (i) passes through (1/2,1/2)

Q.55. A hyperbola passes through the point P(√2,√3) and has foci at (±2, 0). Then the tangent to this hyperbola at P also passes through the point    (2017)
(1) (-√2,-√3)
(2) (3√2, 2√3)
(3) (2√2, 3√3)
(4) (√3,-√2)
Ans. (3)
Solution.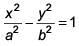

a² +b²= 4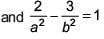


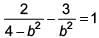
⇒ b² = 3

∴ a²= 1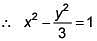

∴ Tangent at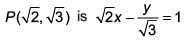
Clearly it passes through (2√2, 3√3)

Q.56. The radius of a circle, having minimum area, which touches the curve y = 4 – x² and the lines, y = |x| is    (2017)
(1) 4 (√2 + 1)
(2) 2 (√2 + 1)
(3) 2 (√2 - 1)
(4) 4 (√2 - 1)
Ans. (4)
Solution.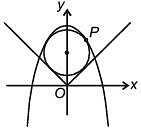

x² =-(y - 4)
Let a point on the parabola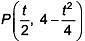
Equation of normal at P is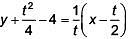


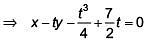
It passes through centre of circle, say (0, k)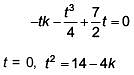


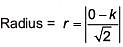
(Length of perpendicular from (0, k) to y = x)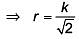

Equation of circle is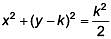

It passes through point P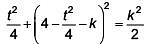

t⁴ +t² (8k- 28) + 8k² - 128k + 256 = 0
For t = ⇒  k² - 16k+ 32 = 0
k = 8±4√2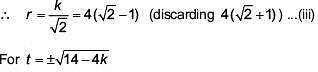

(14 - 4k)²+ (14 - 4k) (8k - 28) + 8k² -128k + 256 = 0
2k² +4k-15 = 0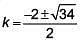


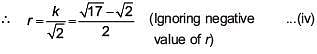
From (iii) & (iv),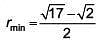

But from options, r = 4(√2-1)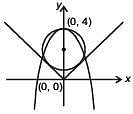


Q.57. The eccentricity of an ellipse whose centre is at the origin is 1/2. If one of its directrices is x = – 4, then the equation of the normal to it at 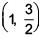 is    (2017)
(1) x + 2y = 4
(2) 2y – x = 2
(3) 4x – 2y = 1
(4) 4x + 2y = 7
Ans. (3)
Solution.

x = –4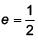


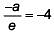
-a= - 4 x e
a = 2
Now,  b² =a² (1- e²) = 3
Equation to ellipse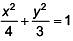

Equation of normal is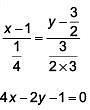


Q.58. The tangent at the point (2,–2) to the curve x²y²– 2x = 4(1 – y) does not pass through the point:    (2017)
(1) (–2,–7)
(2) (8,5)
(3) (–4,–9)
(4)
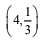
Ans. (1)
Solution. x²y²–2x = 4 – 4y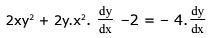



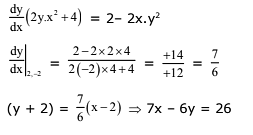
Q.59. The locus of the point of intersection of the straight lines,
tx – 2y – 3t = 0
x – 2ty + 3 = 0 (t ∈ R) , is:    (2017)
(1) a hyperbola with the length of conjugate axis 3
(2) a hyperbola with eccentricity √5
(3) an ellipse with the length of major axis 6
(4) an ellipse with eccentricity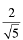
Ans. (1)
Solution.  tx – 2y – 3t = 0
x – 2ty + 3 = 0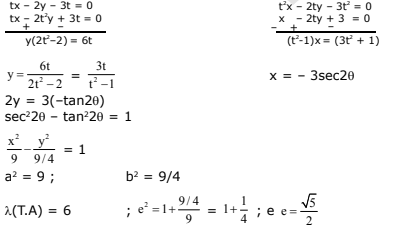


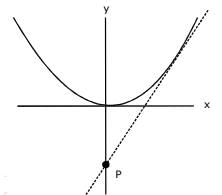
Q.60. If the common tangents to the parabola, x² = 4y and the circle, x² + y² = 4 intersect at the point P, then the distance of P from the origin, is:    (2017)




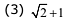
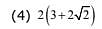
Ans. (4)
Solution. 
tangent to x² + y² = 4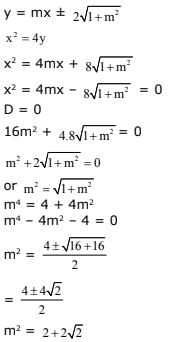



Q.61. Consider an ellipse, whose centre is at the origin and its major axis is along the x - axis. if its eccentricity is 3/5 and the distance between its foci is 6, then the area (in sq. units) of the quadrilateral inscribed in the ellipse, with the vertices as the vertices of the ellipse, is:    (2017)
(1) 32
(2) 80
(3) 40
(4) 8
Ans. (3)
Solution.
e = 3/5, 2ae = 6, a(5) a = 5
b² = a² (1–e²)
b2 = 25 (1–9/25)
b = 4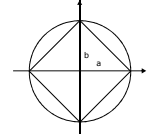

area = 4 (1/2 ab)
= 2ab = 40

Q.62. If y = mx+c is the normal at a point on the parabola y²=8x whose focal distance is 8 units, then |c| is equal to:    (2017)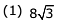




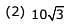
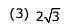
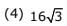
Ans. (2)
Solution. c = – 29m – 9m³ 
a = 2
Given (at² – a)² + 4a²t  = 64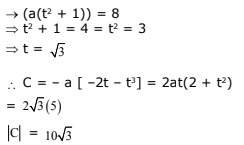


Q.63. The eccentricity of an ellipse having centre at the origin, axes along the co-ordinate axes and passing through the points (4, –1) and (–2,2) is:    (2017)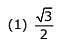




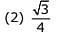
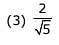
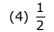
Ans. (1)
Solution. e = ?, centre at (0,0)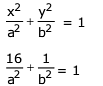

16b² + a² = a²b²    ...(1)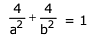

4b² + 4a² = a²b²   ...(2)
From (1) & (2)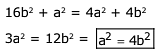


Q.64. Let P be the point on the parabola, y² = 8x which is at a minimum distance from the centre C of the circle, x² + (y + 6)² = 1. Then the equation of the circle, passing through C and having its centre at P is:    (2016) 
(1) x² + y² - 4x + 8y + 12 = 0 
(2) x² + y² - x + 4y - 12 = 0 
(3) x² + y² - 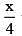+ 2y - 24 = 0 
(4) x² + y² - 4x + 9y + 18 = 0 
Ans. (1)
Normal at P(at², 2at) is y + tx = 2at + at³ Given it passes (0, -6) 
⇒ -6 = 2at + at³ (a = 2) 
-6 = 4t + 2t³ 
t³ + 2t + 3 = 0 
t = -1 
so, P (a, -2a) = (2, -4) . [a = 1) 
radius of circle = CP =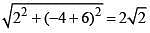 
Circle is (x - 2)² + (y + 4)² =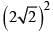 
x² + y² - 4x + 8y + 12 = 0 

Q.65. The eccentricity of the hyperbola whose length of the latus rectum is equal to 8 and the length of its conjugate axis is equal to half the distance between its foci, is:    (2016)
(1) 4/3
(2) 4/√3
(3) 2/√3
(4) √3
Ans. (3)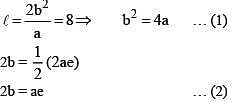

Squaring eqn. (2), we get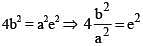
and we know that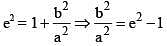 


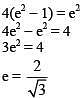
Q.66. If the tangent at a point on the ellipse 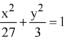 meets the coordinate axes at A and B, and O is the origin, then the minimum area (in sq. units) of the triangle OAB is:    (2016)
(1) 9
(2) 9/2
(3) 9√3
(4) 3√3
Ans. (1)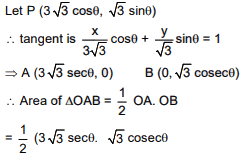



Q.67. The minimum distance of a point on the curve y = x² - 4 from the origin is:    (2016)
(1) √15/2
(2) √19/2
(3)
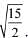
(4)
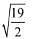
Ans. (1)
Let point at minimum distance from O is


Q.68.  Let a and b respectively be the semi-transverse and semi-conjugate axes of a hyperbola whose eccentricity satisfies the equation 9e² - 18e + 5 = 0. If S(5, 0) is a focus and 5x = 9 is the corresponding directrix of hyperbola, then a² - b² is equal to    (2016)
(1) -7
(2) -5
(3) 5
(4) 7
Ans. (1)
9e² - 18e + 5 = 0
⇒ e = 5/3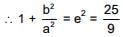
   (i)
Also distance between foci and directrix is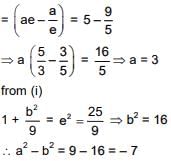


Q.69. If the tangent at a point P, with parameter t, on the curve x = 4t² + 3, y = 8t³ - 1, t ∈ R, meets the curve again at a point Q, then the coordinates of Q are:    (2016)
(1) (t² + 3, - t³ - 1)
(2) (t² + 3, t³ - 1)
(3) (16t² + 3, - 64t³ - 1)
(4) (4t² + 3, - 8t³ - 1)
Ans. (1)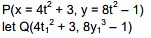



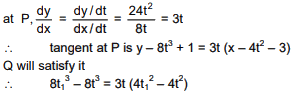
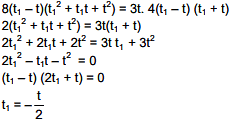
∴ Q(t² + 3, - t³ - 1)

Q.70. P and Q are two distinct points on the parabola, y²  = 4x, with parameters t and t₁ respectively. If the normal at p passes through Q, then the minimum value of t₁² is    (2016)
(1) 4
(2) 6
(3) 8
(4) 2
Ans. (3)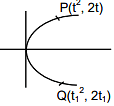



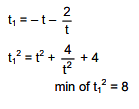
Q.71. A hyperbola whose transverse axis is along the major axis of the conic, 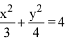 and has vertices at the foci of this conic. If the eccentricity of the hyperbola is 3/2, then which of the following points does NOT lie on it?    (2016)
(1) √5, 2√2
(2) 5, 2√3
(3) 0, 2
(4) √10, 2√3
Ans. (2)



Q.72. Let C be a curve given by y(x) = 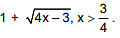If P is a point on C, such that the tangent at P has slope 2/3,  then a point through which the normal at P passes, is    (2016)
(1) 3, -4
(2) 1, 7
(3) 4, -3
(4) 2, 3
Ans. (2)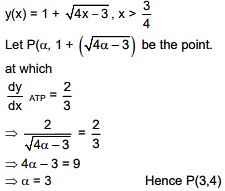


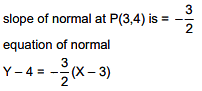
2y - 8 = - 3x + 9
3x + 2y = 17
clearly it is passes through (1, 7)