Scientific Notation & Significant Figures | Chemistry Class 11 - NEET PDF Download
Many times in the study of chemistry, one has to deal with experimental data as well as theoretical calculations.
There are meaningful ways to handle the numbers conveniently and present the data realistically with certainty to the extent possible like:
- Scientific Notation
- Significant Figures
Scientific Notation
- Scientific Notation is a way of expressing numbers that are too big or too small to be conveniently written in decimal form.
- In scientific notation, any number can be represented in the form N × 10n (where n is an exponent having positive or negative values and N can vary between 1 to 10).
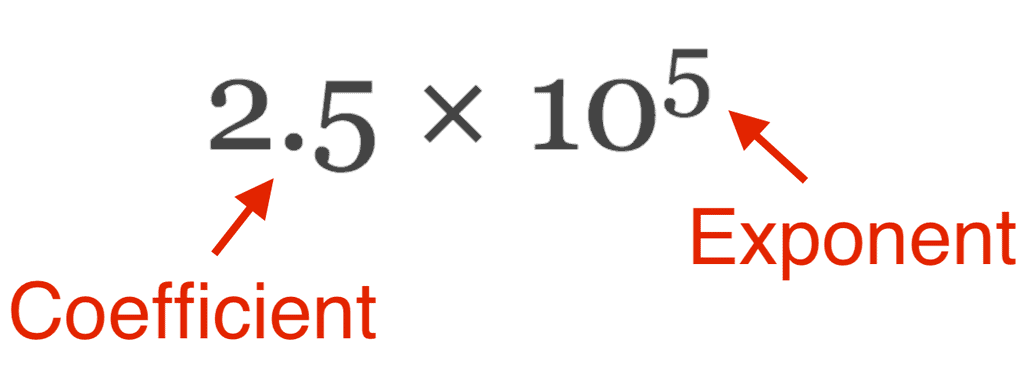 Scientific Notation
Scientific Notation
Example: We can write 232.508 as 2.32508 × 102 in scientific notation. Similarly, 0.00016 can be written as 1.6 × 10–4.
Note that while writing it, the decimal had to be moved to the left by two places and the same is the exponent (2) of 10 in the scientific notation.
Similarly, 0.00016 can be written as 1.6 × 10–4. Here the decimal has to be moved four places to the right and (– 4) is the exponent in the scientific notation.
Multiplication and Division for Exponential Numbers
- These two operations follow the same rules which are there for exponential numbers, i.e.
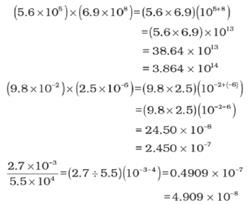 Multiplication and Division
Multiplication and Division
Addition and Subtraction for Exponential Numbers
- For these two operations, first, the numbers are written in such a way that they have the same exponent. After that, the coefficients (digit terms) are added or subtracted as the case may be.
- Thus, for adding 6.65 × 104 and 8.95 × 103, the exponent is made the same for both numbers. Thus, we get (6.65 × 104) + (0.895 × 104).
Examples:
Q.1. Which of the following options is not correct?
(a) 8008 = 8.008 x 103
(b) 208 = 0.208 x 103
(c) 5000 = 5.0 x 103
(d) 2.0034 = 4
Ans: (d)
Solution:
2.0034 = 4
Q.2. Exponential notation in which any number can be represented in the form, Nx 10n here N is termed as
(a) non–digit term
(b) digit term
(c) numeral
(d) base term
Ans: (b)
Solution:
In exponential notation N × 10n, N is a number called digit term which varies between 1.000 and 9.000….
Significant Figures
- The reliability of measurement is indicated by the number of digits used to represent it.
- To express the measurement more accurately, we express it with digits that are known with certainty. These are called significant figures.
- They contain all the certain digits plus one doubtful digit in a number.
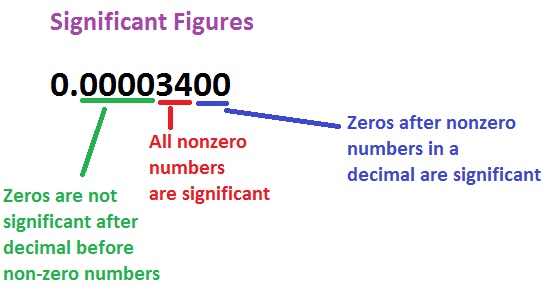 Rules for Determining the Number of Significant Figures
Rules for Determining the Number of Significant Figures
- All non-zero digits are significant.
Example:
- 6.9 has two significant figures.
- 2.16 has three significant figures. - The decimal place does not determine the number of significant figures.
- A zero becomes significant in case it comes in between non-zero numbers.
Example:
- 2.003 has four significant figures.
- 4.02 has three significant figures. - Zeros at the beginning of a number are not significant.
Example:
- 0.002 has one significant figure.
- 0.0045 has two significant figures. - All zeros placed to the right of a number are significant.
Example:
- 16.0 has three significant figures.
- 16.00 has four significant figures. - Zeros at the end of a number without a decimal point are ambiguous.
- In exponential notations, the numerical portion represents the number of significant figures.
Example: 0.00045 is expressed as 4.5 × 10-4 in terms of scientific notations. The number of significant figures in this number is 2, while in Avogadro's number (6.023 × 1023) it is four. - The decimal point does not count towards the number of significant figures.
Example: The number 345601 has six significant figures but can be written in different ways, as 345.601 or 0.345601, or 3.45601, all having the same number of significant figures.
Math with Significant Figures
(i) Addition And Subtraction of Significant Figures
The result cannot have more digits to the right of the decimal point than either of the original numbers.
12.11
18.0
1.012
31.122
Here, 18.0 has only one digit after the decimal point, and the result should be reported only up to one digit after the decimal point, which is 31.1.
12.11 + 18.0 + 1.012 = 31.122
The result reported in this addition should be
(ii) Multiplication and Division of Significant Figures
The result must be reported in these operations with no more significant figures as in the measurement with the few significant figures.
2.5 × 1.25 = 3.125
Since 2.5 has two significant figures, the result should not have more than two significant figures. Thus, it is 3.1.
Practice Questions
Q.1. How many significant figures are in each term?
(a) 34.6209 = 6
(b) 0.003048 = 4
(c) 5010.0 = 5
(d) 4032.090 = 7
Q.2. Solve the following equations using the correct number of significant figures.
(a) 34.683 + 58.930 + 68.35112 = 161.964
(b) 45001 - 56.355 - 78.44 = 44866
(c) 0.003 + 3.5198 + 0.0118 = 3.535
(d) 36.01 - 0.4 - 15 = 21
Q.3. Solve the following equations using the correct number of significant figures.
(a) 98.1 × 0.03 = 3
(b) 57 × 7.368 = 4.2 × 102
(c) 8.578/4.33821 = 1.977
(d) 6.90/2.8952 = 2.38
Q.4. How many significant figures are in each term?
(a) 1.40 × 103 = 3
(b) 6.01 = 3
(c) 02947.1 = 5
(d) 583.02 = 5
Rounding
When doing calculations using significant figures, you will find it necessary to round your answer to the nearest significant digit. There are therefore a few rules of rounding that help retain as much accuracy as possible in the final answer.
- Complete all sequential calculations BEFORE doing any rounding, since rounding early reduces the number of significant figures available for subsequent calculations.
- If the last significant digit is followed by a 6, 7, 8, or 9, round up.
Example: 5.677, rounded to three significant digits, is 5.68 - If the last significant digit is followed by a 0, 1, 2, 3, or 4, simply drop the trailing digits.
Example: 561200, rounded to three significant digits, is 561000 - If the last significant digit is EVEN, and is followed by a 5, drop the trailing digits.
Example: 45850, rounded to three significant digits, is 45800 - If the last significant digit is ODD, and is followed by a 5, round up.
Example: 3.47588, rounded to three significant digits, is 3.48
AMBIGUOUS ZEROS
So what happens if your calculation or measurement ends in a zero? For example, what if you measured a branch that was 200 cm (not 199 or 201 cm) long? The zeros in a measured value of 200 cm in this case appear ambiguous, since it could suggest that there is only one significant digit.
One way to reduce this ambiguity is to use significant figures with scientific notation.
Precision and Accuracy
- Every experimental measurement has some amount of uncertainty associated with it. However, one would always like the results to be precise and accurate. Precision and accuracy are often referred to when we talk about measurement.
- Accuracy refers to the closeness of a measured value to a standard or known value.
Example: If in the lab you obtain a weight measurement of 3.2 kg for a given substance, but the actual or known weight is 10 kg, then your measurement is not accurate. In this case, your measurement is not close to the known value.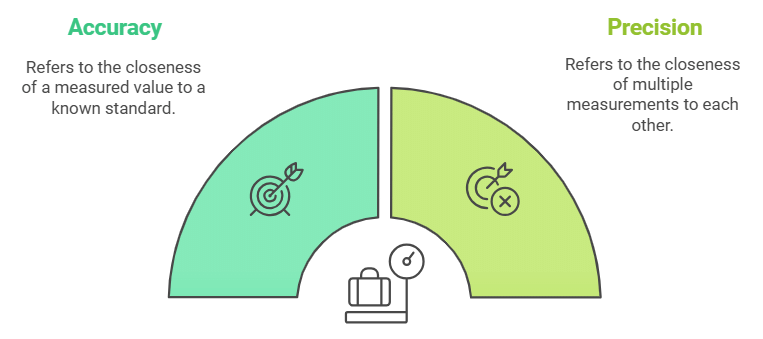
- Precision refers to the closeness of two or more measurements to each other.
Using the example above, if you weigh a given substance five times, and get 3.2 kg each time, then your measurement is very precise. Precision is independent of accuracy. - You can be very precise but inaccurate, as described above. You can also be accurate but imprecise.
Example: If on average, your measurements for a given substance are close to the known value, but the measurements are far from each other, then you have accuracy without precision.
Example: If the true value for a result is 2.00 g.
(a) Student ‘A’ takes two measurements and reports the results as 1.95 g and 1.93 g.
These values are precise as they are close to each other but are not accurate.
(b) Another student repeats the experiment and obtains 1.94 g and 2.05 g as the results for two measurements.
These observations are neither precise nor accurate.
(c) When a third student repeats these measurements and reports 2.01g and 1.99 g as the result.
These values are both precise and accurate.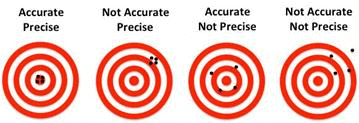 Accuracy and Precision
Accuracy and Precision
|
114 videos|263 docs|74 tests
|
FAQs on Scientific Notation & Significant Figures - Chemistry Class 11 - NEET
| 1. What is scientific notation and why is it used? |  |
| 2. How do you multiply numbers in scientific notation? |  |
| 3. How do you add or subtract numbers in scientific notation? |  |
| 4. What are significant figures and why are they important? |  |
| 5. How do you determine the number of significant figures in a calculation? |  |






















