Chapter 1 - Real Numbers, RD Sharma Solutions - (Part - 1) | RD Sharma Solutions for Class 10 Mathematics PDF Download
Page No 1.10
Q.1. If a and b are two odd positive integers such that a > b, then prove that one of the two numbers (a + b)/2 and (a - b)/2 is odd and the other is even.
Ans. Given: If a and b are two odd positive integers such that a > b.
To Prove: That one of the two numbers (a + b)/2 and (a - b)/2 is odd and the other is even
Proof: Let a and b be any odd odd positive integer such that a > b.
Since any positive integer is of the form q, 2q + 1
Let a = 2q + 1 and b = 2m + 1, where, q and m are some whole numbers
⇒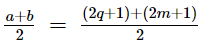
⇒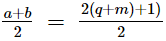
⇒ (a + b)/2 = (q + m + 1)
which is a positive integer.
Also,
⇒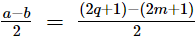
⇒ (a - b)/2 = 2 (q - m) / 2
⇒ (a - b)/2 = (q - m)
Given, a > b
∴ 2q + 1 > 2m + 1
⇒ 2q > 2m
⇒ q > m
∴ (a - b)/2 = (q - m) > 0
Thus, a - b / 2 is a positive integer.
Now, we need to prove that one of the two numbers (a + b)/2 and (a - b)/2 is odd and other is even.
Consider, 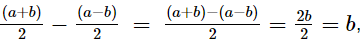 which is odd positive integer.
which is odd positive integer.
Also, we know from the proof above that (a + b)/2 and (a - b)/2 are positive integers.
We know that the difference of two positive integers is an odd number if one of them is odd and another is even. (Also, difference between two odd and two even integers is even)
Hence it is proved that If a and b are two odd positive integers such that a > b then one of the two numbers (a + b)/2 and (a - b)/2 is odd and the other is even.
Q.2. Prove that the product of two consecutive positive integers is divisible by 2.
Ans. To Prove: that the product of two consecutive integers is divisible by 2.
Proof: Let n − 1 and n be two consecutive positive integers.
Then their product is n (n − 1) = n2 − n
We know that every positive integer is of the form 2q or 2q + 1 for some integer q.
So let n = 2q
So, n2 − n = (2q)2 − (2q)
⇒ n2 − n = (2q)2 − 2q
⇒ n2 − n = 4q2 − 2q
⇒ n2 − n = 2q(2q - 1)
⇒ n2 − n = 2r(where r = q(2q - 1))
⇒ n2 − n is even and divisible by 2
Let n = 2q + 1
So, n2 − n = (2q + 1)2 − (2q + 1)
⇒ n2 − n = (2q + 1)((2q + 1) - 1)
⇒ n2 − n = (2q + 1)(2q)
⇒ n2 − n = 2r(r = q(2q + 1))
⇒ n2 − n is even and divisible by 2
Hence it is proved that that the product of two consecutive integers is divisible by 2.
Q.3. Prove that the product of three consecutive positive integer is divisible by 6.
Ans. To Prove: the product of three consecutive positive integers is divisible by 6.
Proof: Let n be any positive integer.
Since any positive integer is of the form 6q or 6q + 1 or 6q + 2 or 6q + 3 or 6q + 4, 6q + 5
If n = 6q
⇒ n(n + 1)(n + 2) = 6q(6q + 1)(6q + 2), which is divisible by 6
If n = 6q + 1
⇒ n(n + 1)(n + 2) = (6q + 1)(6q + 2)(6q + 3)
⇒ n(n + 1)(n + 2) = 6(6q + 1)(3q + 1)(2q + 1)
Which is divisible by 6
If n = 6q + 2
⇒ n(n + 1)(n + 2) = (6q + 2)(6q + 3)(6q + 4)
⇒ n(n + 1)(n + 2) = 12(3q + 1)(2q + 1)(2q + 3)
Which is divisible by 6
Similarly we can prove others.
Hence it is proved that the product of three consecutive positive integers is divisible by 6.
Q.4. For any positive integer n, prove that n3 – n divisible by 6.
Ans. To Prove: For any positive integer n, n3 − n is divisible by 6.
Proof: Let n be any positive integer.
⇒ n3 − n = (n - 1)(n)(n + 1)
Since any positive integer is of the form 6q or 6q + 1 or 6q + 2 or 6q + 3 or 6q + 4, 6q + 5
If n = 6q
Then, (n - 1)n(n + 1) = (6q - 1)6q(6q + 1)
which is divisible by 6
If n = 6q + 1
Then, (n - 1)n(n + 1) = (6q)(6q + 1)(6q + 2)
⇒ which is divisible by 6
If n = 6q + 2
Then, (n - 1)n(n + 1) = (6q + 1)(6q + 2)(6q + 3)
⇒ (n - 1)n(n + 1) = 6(6q + 1)(3q + 1)(2q + 1)
which is divisible by 6
Similarly we can prove others.
Hence it is proved that for any positive integer n, n3 − n is divisible by 6.
Q.5. Prove that if a positive integer is of the form 6q + 5, then it is of the form 3q + 2 for some integer q, but not conversely.
Ans. To Prove: that if a positive integer is of the form 6q + 5 then it is of the form 3q + 2 for some integer q, but not conversely.
Proof: Let n = 6q + 5
Since any positive integer n is of the form of 3k or 3k + 1, 3k + 2
If q = 3k
Then, n = 6q + 5
⇒ n = 18k + 5(q = 3k)
⇒ n = 3(6k + 1) + 2
⇒ n = 3m + 2(where m = (6k + 1))
If q = 3k + 1
Then, n = (6q + 5)
⇒ n = (6(3k + 1) + 5) (q = 3k + 1)
⇒ n = 18k + 6 + 5
⇒ n = 18k + 11
⇒ n = 3(6k + 3) + 2
⇒ n = 3m + 2(where m = (6k + 3))
If q = 3k + 2
Then, n = (6q + 5)
⇒ n = (6(3k + 2) + 5)(q = 3k + 2)
⇒ n = 18k + 12 + 5
⇒ n = 18k + 17
⇒ n = 3(6k + 5) + 2
⇒ n = 3m + 2(where m = (6k + 5)
Consider here 8 which is the form 3q + 2 i.e. 3 × 2 + 2 but it can’t be written in the form 6q + 5. Hence the converse is not true
Q.6. Prove that the square of any positive integer of the form 5q + 1 is of the same form.
Ans. To Prove: that the square of a positive integer of the form 5q + 1 is of the same form
Proof: Since positive integer n is of the form 5q + 1
If n = 5q + 1
Then n2 = (5q + 1)2
⇒ n2 = (5q)2 + (1)2 + 2(5q)(1)
⇒ n2 = 25q2 + 1 + 10q
⇒ n2 = 25q2 + 10q + 1
⇒ n2 = 5(5q2 + 2q) + 1
⇒ n2 = 5m + 1
(where m = (5q2 + 2q))
Hence n2 integer is of the form 5m + 1.
Q.7. Prove that the square of any positive integer is of the form 3m or, 3m + 1 but not of the form 3m + 2.
Ans. To Prove: that the square of an positive integer is of the form 3m or 3m + 1 but not of the form 3m + 2.
Proof: Since positive integer n is of the form of 3q, 3q + 1 and 3q + 2
If n = 3q
⇒ n2 = (3q)2
⇒ n2 = 9q2
⇒ n2 = 3(3q2)
⇒ n2 = 3m(m = 3q2)
If n = 3q + 1
Then, n2 = (3q + 1)2
⇒ n2 = (3q2) + 6q + 1
⇒ n2 = 9q2 + 6q + 1
⇒ n2 = 3q(3q + 1) + 1
⇒ n2 = 3m + 1(where m = (3q + 2))
If n = 3q + 2
Then, n2 = (3q + 2)2
⇒ n2 = (3q2) + 12q + 4
⇒ n2 = 9q2 + 12q + 4
⇒ n2 = 3(3q + 4q + 1) + 1
⇒ n2 = 3m + 1(where q = (3q + 4q + 1))
Hence n2 integer is of the form 3m, 3m + 1 but not of the form 3m + 2.
Q.8. Prove that the square of any positive integer is of the form 4q or 4q + 1 for some integer q.
Ans. To Prove: that the square of any positive integer is of the form 4q or 4q + 1 for some integer q.
Proof: Since positive integer n is of the form of 2q or 2q + 1
If n = 2q
Then, n2 = (2q)2
⇒ n2 = 4q2
⇒ n2 = 4m(where m = q2)
If n = 2q + 1
Then, n2 = (2q + 1)2
⇒ n2 = (2q)2 + 4q + 1
⇒ n2 = 4q2 + 4q + 1
⇒ n2 = 4q(q + 1) + 1
⇒ n2 = 4q + 1 (where m = q(q + 1))
Hence it is proved that the square of any positive integer is of the form 4q or 4q + 1, for some integer q.
Q.9. Prove that the square of any positive integer is of the form 5q, 5q + 1, 5q + 4 for some integer q.
Ans. To Prove: that the square of any positive integer is of the form 5q or 5q + 1, 5q + 4 for some integer q.
Proof: Since positive integer n is of the form of 5q or 5q + 1, 5q + 4
If n = 5q
Then n2 = (5q)2
⇒ n2 = 25q2
⇒ n2 = 5(5q)
⇒ n2 = 5m (where m = 5q)
If n = 5q + 1
Then, n2 = (5q + 1)2
⇒ n2 = (5q)2 + 10q + 1
⇒ n2 = 25q2 + 10q + 1
⇒ n2 = 5q(5q + 2) + 1
⇒ n2 = 5m + 1 (where m = q(5q + 2))
If n = 5q + 2
Then, n2 = (5q + 2)2
⇒ n2 = (5q)2 + 20q + 1
⇒ n2 = 25q2 + 20q + 1
⇒ n2 = 5q(5q + 4) + 4
⇒ n2 = 5m + 4 (where m = q(5q + 4))
If n = 5q + 4
Then, n2 = (5q + 4)2
⇒ n2 = (5q)2 + 40q + 16
⇒ n2 = 25q2 + 40q + 16
⇒ n2 = 5(5q2 + 8q + 3) + 1
⇒ n2 = 5m + 4
(where m = (5q2 + 8q + 3))
Hence it is proved that the square of a positive integer is of the form 5q or 5q + 1, 5q + 4 for some integer q.
Q.10. Show that the square of an odd positive integer is of the form 8q + 1, for some integer q.
Ans. To Prove: that the square of an odd positive integer is of the form 8q + 1, for some integer q.
Proof: Since any positive integer n is of the form 4m + 1 and 4m + 3
If n = 4m + 1
⇒ n2 = (4m + 1)2
⇒ n2 = (4m)2 + 8m + 1
⇒ n2 = 16m2 + 8m + 1
⇒ n2 = 8m(2m + 1) + 1
⇒ n2 + 8q + 1(q = m(2m + 1))
If n = 4m + 3
⇒ n2 = (4m + 3)2
⇒ n2 = (4m)2 + 24m + 9
⇒ n2 = 16m2 + 24m + 9
⇒ n2 = 8(2m2 + 3m + 1) + 1
⇒ n2 = 8q + 1 (q = (2m2 + 3m + 1))
Hence n2 integer is of the form 8q + 1, for some integer q.
FAQs on Chapter 1 - Real Numbers, RD Sharma Solutions - (Part - 1) - RD Sharma Solutions for Class 10 Mathematics
| 1. What are real numbers? |  |
| 2. How do I determine if a number is rational or irrational? |  |
| 3. How can I perform operations on real numbers? |  |
| 4. What is the importance of real numbers in mathematics? |  |
| 5. How can I apply real numbers in real-life situations? |  |

|
Explore Courses for Class 10 exam
|

|












