Chapter 2 - Polynomials, RD Sharma Solutions - (Part-5) | RD Sharma Solutions for Class 10 Mathematics PDF Download

Page No 2.57

Ques.5. Obtain all zeros of the polynomial f(x) = x⁴ − 3x³ − x² + 9x − 6, if two of its zeros are -√3 and √3
Ans. We know that if x =α is a zero of a polynomial, then (x - α) is a factor of f(x).
Since -√3 and √3 are zeros of f(x).
Therefore
(x + √3)(x - √3) = x² + √3x - √3x - 3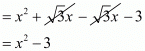

x² - 3 is a factor of f(x). Now, we divide f(x) = x⁴ - 3x³ - x² + 9x - 6 by g(x) = x² - 3 to find the other zeros of f(x).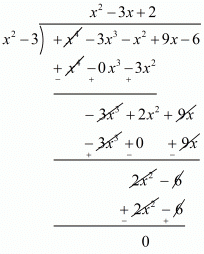
 
By using division algorithm we have, .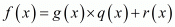 


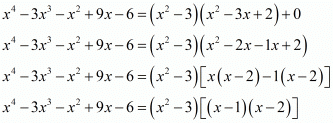
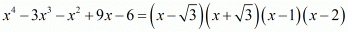
Hence, the zeros of the given polynomials are -√3, +√3, +1, +2.

Ques.6. Find all zeros of the polynomial f(x) = 2x⁴ − 2x³ − 7x² + 3x + 6, if its two zeros are 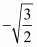  and 
An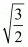s. Since 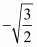  and 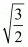 are two zeros of f(x).Therefore, 

= 1/2(2x² - 3) is a factor of f(x).
Also 2x² - 3 is a factor of f(x). 
Let us now divide f(x) by 2x² - 3. We have,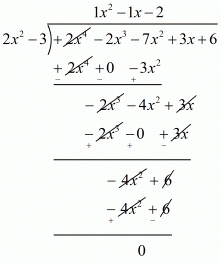 

By using division algorithm we have,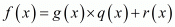 

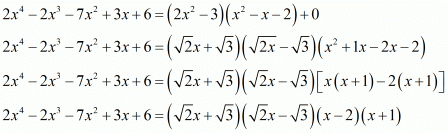
Hence, The zeros of f(x) are .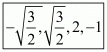 

Ques.7. Find all the zeros of the polynomial x⁴ + x³ − 34x² − 4x + 120, if two of its zeros are 2 and −2.
Ans. We know that if x =α is a zero of a polynomial, then x - α is a factor of f(x).
Since, 2 and -2 are zeros of f(x). 
Therefore
(x + 2)(x - 2) = x² - 2²
= x² - 4
x² - 4 is a factor of f(x). Now, we divide x⁴ + x³ - 34x² - 4x + 120 by g(x) = x² - 4 to find the other zeros of f(x).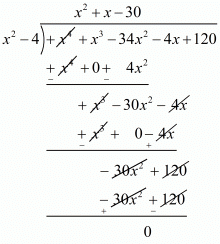 

By using division algorithm we have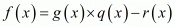 

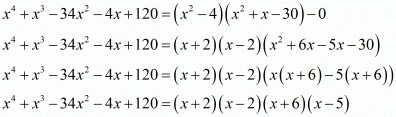
Hence, the zeros of the given polynomial are -2, +2, -6, and 5.

Ques.8. Find all zeros of the polynomial 2x⁴ + 7x³ − 19x² − 14x + 30, if two of its zeros are √2 and -√2.
Ans. We know that if x = α is a zero of a polynomial, and then x - α is a factor of f(x).
Since √2 and -√2 are zeros of f(x).
Therefore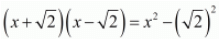

= x² - 2
x² - 2 is a factor of f(x). Now, we divide 2x⁴ + 7x³ - 19x² - 14x + 30 by g(x) = x² - 2 to find the zero of f(x).
 
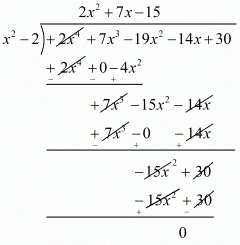
By using division algorithm we have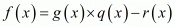 

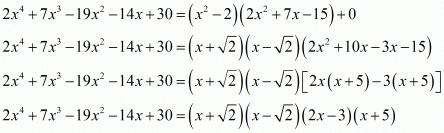
Hence, the zeros of the given polynomial are .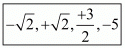 

Page No 2.58

Ques.9. Find all the zeros of the polynomial 2x³ + x² − 6x − 3, if two of its zeros are −√3 and √3.
Ans. We know that if x = α is a zero of a polynomial, and then x -α is a factor of f(x).
Since √3 and -√3 are zeros of f(x).
Therefore
(x + √3)(x - √3) = x² - 3
= x² - 3
x² - 3 is a factor of f(x).Now, we divide 2x³ + x² - 6x - 3 by g(x) = x² - 3 to find the other zeros of f(x).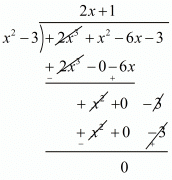 

By using division algorithm we have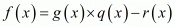 

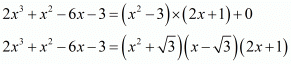
Hence, the zeros of the given polynomial are.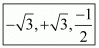 

Ques.10. Find all the zeros of the polynomial x³ + 3x² − 2x − 6, if two of its zeros are -√2 and √2.
Ans. We know that if x = α is a zero of a polynomial, and then x - α is a factor of f(x).
Since √2 and -√2 are zeros of f(x). 
Therefore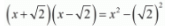

=x² - 2
x² - 2 is a factor of f(x).Now, we divide x³ + 3x² - 2x - 6 by g(x) = x² - 2 to find the other zeros of f(x).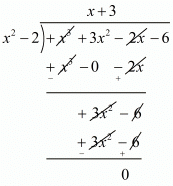 

By using division algorithm we have


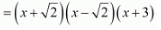
Hence, the zeros of the given polynomials are -√2, +√2, and -3.

Ques.11. Find all zeros of the polynomial 2x⁴ – 9x³ + 5x² +3x – 1, if two of its zeros are 2+√3 and 2−√3.
Ans. It is given that 2+√3 and 2−√3 are two zeroes of the polynomial f(x) = 2x⁴ – 9x³ + 5x² + 3x – 1.
∴ {x - (2 + √3)}{x - (2 - √3)}=(x - 2 -√3)(x - 2 +√3)
= (x−2)²−(√3)²
= x² − 4x + 4 − 3
= x² − 4x + 1
is a factor of f(x)
Now, divide f(x) by x² – 4x + 1.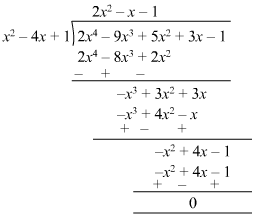

∴ f(x) = (x² – 4x + 1) (2x² – x – 1)

Hence, other two zeroes of f(x) are the zeroes of the polynomial 2x² – x – 1.

2x² – x – 1 = 2x² – 2x + x – 1 = 2x(x – 1) + 1 (x – 1) = (2x + 1) (x – 1)

Hence, the other two zeroes are −1/2 and 1.

Ques.12. For what value of k, is the polynomial f(x) = 3x⁴ – 9x³ + x² + 15x + k completely divisible by 3x² – 5?
Ans. Let f(x) = 3x⁴ – 9x³ + x² + 15x + k
It is given that f(x) is completely divisible by 3x² – 5.
Therefore, one factor of f(x) is (3x² – 5).
We get another factor of f(x) by dividing it with (3x² – 5).
On division, we get the quotient x² – 3x + 2 and the remainder k + 10.
Since, f(x) = 3x⁴ – 9x³ + x² + 15x + k completely divisible by 3x² – 5
Therefore, remainder must be zero.
⇒ k + 10 = 0
⇒ k = −10
Hence, the value of k is –10.

Ques.13. What must be added to the polynomial f(x) = x⁴ + 2x³ − 2x² + x − 1 so that the resulting polynomial is exactly divisible by x² + 2x − 3?
Ans. We know that,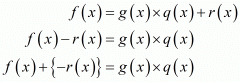

Clearly , Right hand side is divisible by g(x).
Therefore, Left hand side is also divisible by g(x). Thus, if we add -r(x) to f(x), then the resulting polynomial is divisible by g(x).
Let us now find the remainder when f(x) is divided by g(x).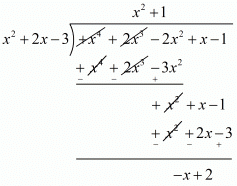

Hence, we should add -r(x) = x - 2 to f(x) so that the resulting polynomial is divisible by g(x). 

Ques.14. What must be subtracted from the polynomial f(x) = x⁴ + 2x³ − 13x² − 12x + 21 so that the resulting polynomial is exactly divisible by x² − 4x + 3?
Ans. We know that Dividend = Quotient x Divisor + Remainder.
Dividend - Remainder = Quotient x Divisor.
Clearly, Right hand side of the above result is divisible by the divisor.
Therefore, left hand side is also divisible by the divisor.
Thus, if we subtract remainder from the dividend, then it will be exactly divisible by the divisor.
Dividing x⁴ + 2x³ - 13x² - 12x + 21 by x² - 4x + 3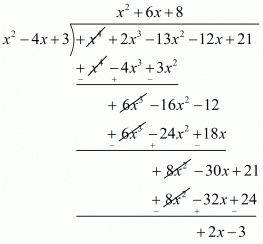

Therefore, quotient = x² + 6x + 8 and remainder = (2x - 3). 
Thus, if we subtract the remainder 2x - 3 from x⁴ + 2x³ - 13x² - 12x + 21, it will be divisible by x² - 4x + 3.

Ques.15. Given that √2 is a zero of the cubic polynomial 6x³ + √2x² − 10x − 4√2, find its other two zeroes.
Ans. We know that if x = α is a zero of a polynomial, and then x - α is a factor of f(x). 
It is given the √2 is a zero of the cubic polynomial f(x) = 6x³ + √2x² − 10x − 4√2.
Therefore, x − √2 is a factor of f(x). Now, we divide 6x³ + √2x² − 10x − 4√2 by x−√2 to find the other zeroes of f(x).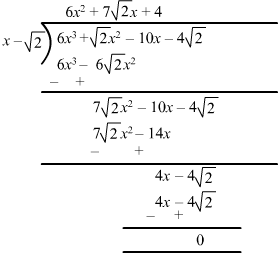

∴ Quotient = 6x² + 7√2x + 4 and remainder = 0.
By using division algorithm, we have f(x) = g(x) × q(x) + r(x).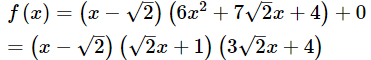

Hence, the other two zeroes of the given polynomial are -(1/√2) and -(4/3√2).

Ques.16. Given that x − √5 is a factor of the cubic polynomial x³ − 3√5x² + 13x − 3√5, find all the zeroes of the polynomial. 
Ans. We know that if x = α is a zero of a polynomial, and then x - α is a factor of f(x). 
It is given that x − √5 is a factor of f(x) = x³− 3√5x² + 13x − 3√5.
Now, we divide x³−3√5x² + 13x − 3√5 by x−√5 to find the other zeroes of f(x).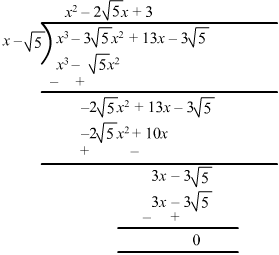

∴ Quotient = x² − 2√5x + 3 and remainder = 0.
By using division algorithm, we have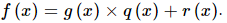

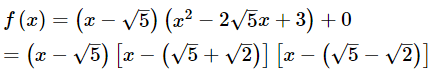
Hence, the zeroes of the given polynomial are √5, √5 + √2 and √5 − √2.

Page No 2.58

Ques.1. If α, β are the zeros of the polynomial f(x) = x² + x + 1, then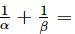
(a) 1
(b) −1
(c) 0
(d) None of these
Ans. Since α and β are the zeros of the quadratic polynomial f(x) = x² + x + 1
α + β = -(coefficient of x / coefficient of x²)
= ((-1)/1) = 1
α x β = constant term/coefficient of x²
= 1/1 = 1
We have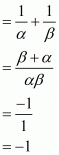

The value of 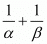 is -1
Hence, the correct choice is (b).

Ques.2. If α, β are the zeros of the polynomial p(x) = 4x² + 3x + 7, then 1/α + 1/β is equal to
(a) 7/3
(b) -(7/3)
(c) 3/7
(d) -(3/7)
Ans. Since α and β are the zeros of the quadratic polynomial p(x) = 4x² + 3x + 7
α + β = ((-coefficient of x) / coefficient of x²)
= (-3)/4
αβ = constant term/coefficient of x²
= 7/4
We have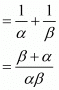


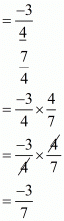
The value of 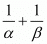 is (-3)/7. 
Hence, the correct choice is d.

Ques.3. If one zero of the polynomial f(x) = (k² + 4)x² + 13x + 4k is reciprocal of the other, then k =
(a) 2
(b) −2
(c) 1
(d) −1
Ans. We are given f(x) = (k² + 4)x² + 13x + 4k then
α + β = ((-coefficient of x) / coefficient of x²)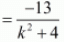

α x β = constant term/coefficient of x²

One root of the polynomial is reciprocal of the other. Then, we have
α x β = 1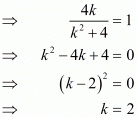

Hence the correct choice is a.

Ques.4. If the sum of the zeros of the polynomial f(x) = 2x³ − 3kx² + 4x − 5 is 6, then the value of k is
(a) 2
(b) 4
(c) −2
(d) −4
Ans. Let α, β be the zeros of the polynomial f(x) = 2x³ - 3kx² + 4x - 5 and we are given that α + β + γ = 6.
Then,
α + β + γ = −Coefficient of x/Coefficient of x²
= -((-3k)/2) = 3k/2
It is given that
α + β + γ = 6
Substituting α + β + γ = 3k/2, we get

The value of k is 4.
Hence, the correct alternative is b.

Ques.5. If α and β are the zeros of the polynomial f(x) = x² + px + q, then a polynomial having 1/α and 1/β is its zero is
(a) x² + qx + p
(b) x² − px + q
(c) qx² + px + 1
(d) px² + qx + 1
Ans. Let α and β be the zeros of the polynomial f(x) = x² + px + q. Then,
α + β = −Coefficient of x/Coefficient of x²
= -(p/1)
= -p
And
αβ = constant term/coefficient of x²
= q/1
= q
Let S and R denote respectively the sum and product of the zeros of a polynomial
Whose zeros are 1/α and 1/β then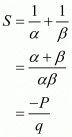


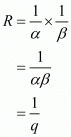
Hence, the required polynomial g(x) whose sum and product of zeros are S and R is given by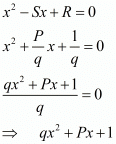

So g(x) = qx² + Px + 1
Hence, the correct choice is c.

Ques.6. If α, β are the zeros of polynomial f(x) = x² − p (x + 1) − c, then (α + 1) (β + 1) =
(a) c − 1
(b) 1 − c
(c) c
(d) 1 + c
Ans. Since α and β are the zeros of quadratic polynomial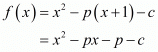

α + β = −Coefficient of x/Coefficient of x^{2
= - ((-p)/1)
= p}
α x β = constant term/coefficient of x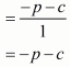<sup>2

We have</sup>
(α + 1)(β + 1)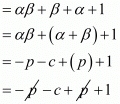

= -c + 1
= 1 - c
The value of (α + 1)(β + 1) is 1 - c.
Hence, the correct choice is b.

Ques.7. If α, β are the zeros of the polynomial f(x) = x² − p(x + 1) − c such that (α +1) (β + 1) = 0, then c =
(a) 1
(b) 0
(c) −1
(d) 2
Ans. Since α and β are the zeros of quadratic polynomial
f(x) = x² - p(x+1) - c
f(x) = x² - px - p - c
α + β = ((−Coefficient of x)/Coefficient of x²)
= -((-p)/1)
= p
αβ = constant term/coefficient of x²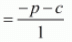

= -p-c
We have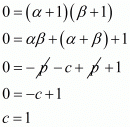

The value of c is 1.
Hence, the correct alternative is a.

Ques.8. If f(x) = ax² + bx + c has no real zeros and a + b + c = 0, then
(a) c = 0
(b) c > 0
(c) c < 0
(d) None of these
Ans. If f(x) = ax² + bx + c has no real zeros and a + b + c < 0 then c < 0
Hence, the correct choice is c.