Chapter 5 - Quadratic Equations, RD Sharma Solutions - (Part-9) | RD Sharma Solutions for Class 10 Mathematics PDF Download
Page No 5.30
Ques.11. Split 207 into three parts such that these are in A.P. and the product of the two smaller parts is 4623.
Ans. Suppose three parts of 207 are (a − d), a , (a + d) such that, (a + d) > a > (a − d).
a − d + a + a + d = 207
⇒ 3a = 207
⇒ a = 69
Now, (a − d) × a = 4623
⇒ 69(69 − d) = 4623
⇒ (69 − d) = 67
⇒ d = 2
Therefore, the three required parts are 67, 69 and 71.
Ques.12. The angles of a triangle are in A.P. The greatest angle is twice the least. Find all the angles.
Ans. Suppose the angles of a triangle are (a − d), a, (a + d) such that, (a + d) > a > (a − d).
a − d + a + a + d =180 [angle sum property]
⇒ 3a = 180
⇒ a = 60
Now, (a + d) = 2(a − d)
⇒ a + d = 2a − 2d
⇒ a = 3d
⇒ d = 60/3 = 20
Therefore, the three angles of a triangle are 40, 60, 80.
Ques.13. The sum of four consecutive numbers in A.P. is 32 and the ratio of the product of the first and last terms to the product of two middle terms is 7 : 15. Find the number.
Ans. Let the four terms of the AP be a − 3d, a − d, a + d and a + 3d.
Given:
(a − 3d) + (a − d) + (a + d) + (a + 3d) = 32
⇒ 4a = 32
⇒ a = 8
Also,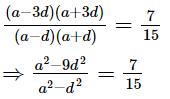
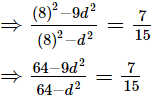
⇒ 960 − 135d2 = 448 − 7d2
⇒ 512 = 128d2
⇒ d2 = 4
⇒ d = ±2
When a = 8 and d= 2, then the terms are 2, 6, 10, 14.
When a = 8 and d= −2, then the terms are 14, 10, 6, 2.
Page No 5.50
Ques.1. Find the sum of the following arithmetic progressions:
(i) 50, 46, 42, ... to 10 terms
(ii) 1, 3, 5, 7, ... to 12 terms
(iii) 3, 9/2, 6, 15/2, ... to 25 terms
(iv) 41, 36, 31, ... to 12 terms
(v) a + b, a − b, a − 3b, ... to 22 terms
(vi) (x − y)2, (x2 + y2), (x + y)2, ..., to n terms
(vii) 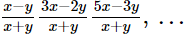 to n terms
to n terms
(viii) −26, −24, −22, ... to 36 terms.
Ans. In the given problem, we need to find the sum of terms for different arithmetic progressions. So, here we use the following formula for the sum of n terms of an A.P.,
Sn = (n/2)[2a + (n - 1)d]
Where; a = first term for the given A.P.
d = common difference of the given A.P.
n = number of terms
(i) 50, 46, 42, ... To 10 terms
Common difference of the A.P. (d)
= a2 -a1
= 46 - 50
= -4
Number of terms (n) = 10
First-term for the given A.P. (a) = 50
So, using the formula we get,
S10 = (10/2)[2(50) + (10-1)(-4)]
= (5)[100 + (9)(-4)]
= (5)[100 - 36]
= (5)[64]
= 320
Therefore, the sum of first 10 terms for the given A.P. is 320.
(ii) 1, 3, 5, 7, ... - 26 To 12 terms.
Common difference of the A.P. (d)
= a2 - a1
= 3 - 1
= 2
Number of terms (n) = 12
First-term for the given A.P. (a) = 1
So, using the formula we get,
Sn = (12/2)[2(1) + (12 - 1)(2)]
= (6)[2 + (11)(2)]
= (6)[2 + 22]
= (6([24]
= 144
Therefore, the sum of first 12 terms for the given A.P. is 144.
(iii) 3, 9/2, 6, 15/2, ... To 25 terms.
Common difference of the A.P. (d) = a2 - a1
= (9/2) - 3
= 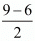
= 3/2
Number of terms (n) = 25
First term for the given A.P. (a) = 3
So, using the formula we get,
S25 = (25/2)[2(3) + (25 - 1)(3/2)]
= (25/2)[6 + (24)(3/2)]
= (25/2)[6 + (72/2)]
= (25/2)[6 + 36]
= (25/2)[42]
= (25)(21)
= 525
On further simplifying, we get,
S25 = 525
Therefore, the sum of first 25 terms for the given A.P. is 525.
(iv) 41, 36, 31, ... To 12 terms.
Common difference of the A.P. (d) = a2 - a1
36 - 41
= -5
Number of terms (n) = 12
First-term for the given A.P. (a) = 41
So, using the formula we get,
S12 = 12/2[2(41) + (12 - 1)(-5)]
= (6)[82 + (11)(-5)]
= (6)[82 - 55]
= (6)[27]
= 162
Therefore, the sum of first 12 terms for the given A.P. is 162.
(v) a + b, a - b, a - 3b, ... To 22 terms.
Common difference of the A.P. (d) = a2 - a1
= (a - b)-(a + b)
= a - b - a - b
= -2b
Number of terms (n) = 22
First-term for the given A.P. (a) = a + b
So, using the formula we get,
S22 = 22/2[2(a + b) + (22 - 1)(-2b)]
= (11)[2a + 2b + (21)(-2b)]
= (11)[2a + 2b - 42b]
= (11)[2a - 40b]
= 22a - 440b
Therefore, the sum of first 22 terms for the given A.P. is 22a - 440b.
(vi) (x − y)2, (x2 + y2), (x + y)2, ..., To n terms.
Common difference of the A.P. (d) = a2 - a1
= (x2 + y2)-(x - y)2
= x2 + y2 - (x2 + y2 - 2xy)
= x2 + y2 - x2 - y2 + 2xy
= 2xy
Number of terms (n) = n
First-term for the given A.P. (a) = (x - y)2
So, using the formula we get,
Sn = n/2[2(x - y)2 + (n - 1)2xy]
Now, taking 2 common from both the terms inside the bracket we get,
=(n/2)[(2)(x - y)2 + (2)(n - 1)xy]
=(n/2)(2)[(x - y)2 + (n - 1)xy]
= (n)[(x - y)2 + (n - 1)xy]
Therefore, the sum of first n terms for the given A.P. is (n) [(x - y)2 + (n - 1)xy]
(vii) 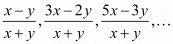 To n terms.
To n terms.
Number of terms (n) = n
First-term for the given A.P. (a) = 
Common difference of the A.P. (d) = a2 - a1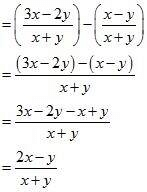
So, using the formula we get,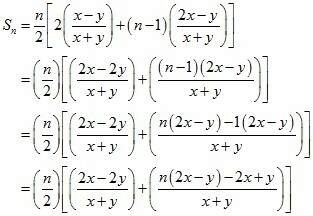
Now, on further solving the above equation we get,
Therefore, the sum of first n terms for the given A.P. is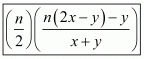 .
.
(viii) -26, -24, -22, ... To 36 terms.
Common difference of the A.P. (d) = a2 - a1
= (-24)-(-26)
= -24 + 26 = 2
Number of terms (n) = 36
First-term for the given A.P. (a) = −26
So, using the formula we get,
S36 = 36/2[2(-26) + (36 - 1)(2)]
= (18)[-52 + (35)(2)]
= (18)[-52 + 70]
= (18)[18]
= 324
Therefore, the sum of first 36 terms for the given A.P. is 324.
Page No. 5.5
Ques.1. Write the first five terms of each of the following sequences whose nth terms are:
(a) an = 3n + 2
(b) an = 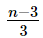
(c) an = 3n
(d) an=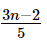
(e) an = (−1)n 2n
(f) an =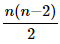
(g) an = n2 − n + 1
(h) an = 2n2 − 3n + 1
(i) an = 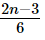
Ans. Here, we are given the nth term for various sequences. We need to find the first five terms of the sequence.
(i) an = 3n + 2
Here, the nth term is given by the above expression. So, to find the first term we use n = 1, we get,
a1 = 3(1) + 2
= 3 + 2
= 5
Similarly, we find the other four terms,
Second term (n = 2),
a2 = 3(2) + 2
= 6 + 2 = 8
Third term (n = 3),
a3 = 3(3) + 2
= 9 + 2 = 11
Fourth term (n = 4),
a4 = 3(4) + 2
= 12 + 2 = 14
Fifth term (n = 5),
a5 = 3(5) + 2
= 15 + 2 = 17
Therefore, the first five terms for the given sequence are
a1 = 5, a2 = 8, a3 = 11, a4 = 14, a5 = 17.
(ii) an = 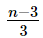
Here,the nth term is given by the above expression. So, to find the first term we use, n = 1, we get,
a1 = ((1) -(2))/3
= (-1)/3
Similarly, we find the other four terms,
Second term (n = 2),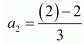
= 0/3
= 0
Third term (n = 3),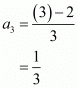
Fourth term (n = 4),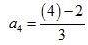
= 2/3
Fifth term (n= 5),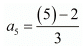
= 3/3 = 1
Therefore, the first five terms for the given sequence are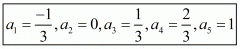 .
.
(iii) an = 3n
Here, the nth term is given by the above expression. So, to find the first term we use n= 1,we get,
a1 = 3(1)
= 3
Similarly, we find the other four terms,
Second term (n = 2)
a2 = 3(2)
= (3)(3)
= 9
Third term = (n = 3),
a3 = 3(3)
= (3)(3)(3)
= 27
Fourth term (n = 4)
a4 = 3(4)
= (3)(3)(3)(3)
= 81
Fifth term (n = 5),
a5 = 3(5)
= (3)(3)(3)(3)(3)
= 243
Therefore, the first five terms for the given sequence are a1 = 3, a2 = 9, a3 = 27, a4 = 81, a5 = 243.
(iv) an=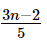
Here, the nth term is given by the above expression. So, to find the first term we use, n = 1, we get,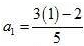
= 1/5
Similarly, we find the other four terms,
Second term (n = 2),
Third term (n = 3),
Fourth term (n = 4),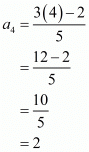
Fifth term (n = 5),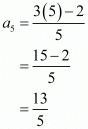
Therefore, the first five terms for the given sequence are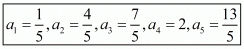 .
.
(v) an = (-1)n.2n
Here, the nth term is given by the above expression. So, to find the first term we use n = 1, we get,
a1 = (-1)1.21
= (-1).2
= -2
Similarly, we find the other four terms,
Second term (n = 2),
a2 = (-1)2.22
= 1.4 = 4
Third term (n = 3),
a3 = (-1)3.23
= (-1).8
= -8
Fourth term (n = 4),
a4 = (-1)4.24
= 1.16 = 16
Fifth term (n = 5),
a5 = (-1)5.25
=(-1).32 = -32
Therefore, the first five terms of the given A.P are a1 = -2, a2 = 4, a3 = -8, a4 = 16, a5 = -32.
(vi) 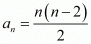
Here, the nth term is given by the above expression. So, to find the first term we use n = 1, we get,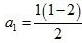
= (-1)/2
Similarly, we find the other four terms,
Second term (n = 2),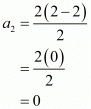
Third term(n = 3),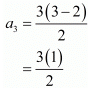
= 3/2
Fourth term (n = 4),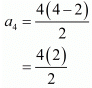
= 8/2 = 4
Fifth term (n = 5),
= 15/2
Therefore, the first five terms for the given sequence are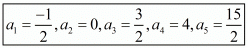 .
.
(vii) an = n2 - n + 1
Here, the nth term is given by the above expression. So, to find the first term we use n = 1, we get,
a1 = (1)2 - (1) + 1
= 1 - 1 + 1 = 1
Similarly, we find the other four terms,
Second term (n = 2),
a2 = (2)2 - (2) + 1
= 4 - 2 + 1 = 3
Third term (n = 3),
a3 = (3)2 - (3) + 1
= 9 - 3 + 1 = 7
Fourth term (n = 4),
a4 = (4)2 - (4) + 1
= 16 - 4 + 1 = 13
Fifth term (n = 5),
a5 = (5)2 - (5) + 1
= 25 - 5 + 1 = 21
Therefore, the first five terms for the given sequence are a1 = 1, a2 = 3, a3 = 7, a4 = 13, a5 = 21.
(viii) an = 2a2 - 3n + 1
Here, the nth term is given by the above expression. So, to find the first term we use n = 1, we get,
a1 = 2(1)2 - 3(1) + 1
= 2(1) - 3 + 1
= 2 - 3 + 1= 0
Similarly, we find the other four terms,
Second term (n = 2),
a2 = 2(2)2 - 3(2) + 1
= 2(4) - 6 + 1
= 8 - 6 + 1 = 3
Third term (n = 3),
a3 = 2(3)2 - 3(3) + 1
= 2(9) - 9 + 1
= 18 - 9 + 1 = 10
Fourth term (n = 4),
a4 = 2(4)2 - 3(4) + 1
= 2(16) - 12 + 1
= 32 - 12 + 1 = 21
Fifth term (n = 5),
a5 = 2(5)2 - 3(5) + 1
= 2(25) - 15 + 1
= 50 - 15 + 1 = 36
Therefore, the first five terms for the given sequence are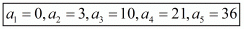 .
.
(ix) 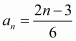
Here, the nth term is given by the above expression. So, to find the first term we use n = 1, we get,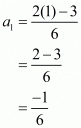
Similarly, we find the other four terms,
Second term (n = 2),
Third term (n = 3),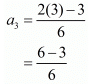
= 3/6 = 1/2
Fourth term (n = 4),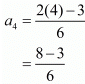
= 5/6
Fifth term (n = 5),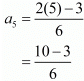
= 7/6
Therefore, the first five terms of the given A.P are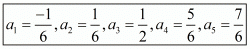 .
.
FAQs on Chapter 5 - Quadratic Equations, RD Sharma Solutions - (Part-9) - RD Sharma Solutions for Class 10 Mathematics
| 1. What is the general form of a quadratic equation? |  |
| 2. How can we solve a quadratic equation using the quadratic formula? |  |
| 3. What are the different methods to solve a quadratic equation? |  |
| 4. How can we determine the nature of the roots of a quadratic equation? |  |
| 5. How can we use the roots of a quadratic equation to find the coefficients of the equation? |  |

|
Explore Courses for Class 10 exam
|

|
















