Chapter 7 - Coordinate Geometry, RD Sharma Solutions - (Part-4) | RD Sharma Solutions for Class 10 Mathematics PDF Download
Page No 6.17
Ques.46. If the point P(x, 3) is equidistant from the point A(7, −1) and B(6, 8), then find the value of x and find the distance AP.
Ans. It is given that P(x, 3) is equidistant from the point A(7, −1) and B(6, 8).
∴ AP = BP
⇒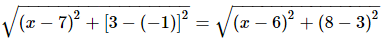 (Distance formula)
(Distance formula)
Squaring on both sides, we get
(x−7)2+16 = x−6)2 + 25
⇒x2−14x+49+16 = x2−12x+36+25
⇒−14x+12x=61−65
⇒−2x=−4
⇒x=2
Thus, the value of x is 2.
∴AP= units
units
Page No 6.17
Ques.47. If A(3, y) is equidistant from points P(8, −3) and Q(7, 6), find the value of y and find the distance AQ.
Ans. It is given that A(3, y) is equidistant from points P(8, −3) and Q(7, 6).
∴ AP = AQ
⇒ (Distance formula)
(Distance formula)
Squaring on both sides, we get
25+(y+3)2 = 16+(y−6)2
⇒25+y2+6y+9 = 16+y2−12y+36
⇒12y+6y=52−34
⇒18y=18
⇒y=1
Thus, the value of y is 1.
∴AQ= 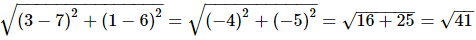 units
units
Page No 6.17
Ques.48. If (0, −3) and (0, 3) are the two vertices of an equilateral triangle, find the coordinates of its third vertex.
Ans. Let the given points be A(0, −3) and B(0, 3). Suppose the coordinates of the third vertex be C(x, y).
Now, ∆ABC is an equilateral triangle.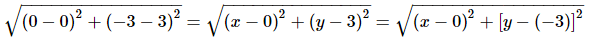
(Distance formula)
Squaring on both sides, we get
36 = x2+(y−3)2 = x2+(y+3)2
⇒ x2+(y−3)2 = x2+(y+3)2
and x2+(y−3)2 = 36
Now,
x2+(y−3)2 = x2+(y+3)2
⇒y2−6y+9 = y2+6y+9
⇒−12y=0
⇒y=0
Putting y = 0 in x2+(y−3)2=36 we get
x2+(0−3)2=36
⇒x2=36−9=27
⇒x= ± √27 = ±3√3
Thus, the coordinates of the third vertex are (3√3, 0) or (−3√3, 0).
Page No 6.17
Ques.49. If the point P(2, 2) is equidistant from the points A(−2, k) and B(−2k, −3), find k. Also, find the length of AP.
Ans. It is given that P(2, 2) is equidistant from the points A(−2, k) and B(−2k, −3).
∴ AP = BP
⇒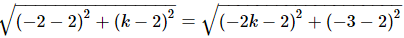 (Distance formula)
(Distance formula)
Squaring on both sides, we get
16+k2−4k+4=4k2+8k+4+2
⇒3k2+12k+9=0
⇒k2+4k+3=0
⇒(k+3)(k+1)=0
Thus, the value of k is −1 or −3.
When k = −1,
AP=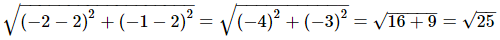 =5 units
=5 units
When k = −3,
AP=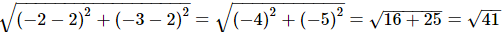 units
units
Page No 6.17
Ques.50. Show that ΔABC, where A(–2, 0), B(2, 0), C(0, 2) and ΔPQR where P(–4, 0), Q(4, 0), R(0, 2) are similar triangles.
Ans. In ΔABC, the coordinates of the vertices are A(–2, 0), B(2, 0), C(0, 2).
AB=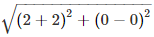 = 4
= 4
BC= = √8=2√2
= √8=2√2
CA=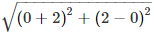 =√8=2√2
=√8=2√2
In ΔPQR, the coordinates of the vertices are P(–4, 0), Q(4, 0), R(0, 4).
PQ=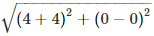 =8
=8
QR=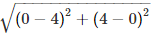 =4√2
=4√2
PR=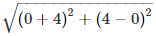 =4√2
=4√2
Now, for ΔABC and ΔPQR to be similar, the corresponding sides should be proportional.
So, AB/PQ=BC/QR=CA/PR
⇒4/8=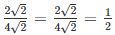
Thus, ΔABC is similar to ΔPQR.
Page No 6.17
Ques.51. An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
Ans. The distance d between two points (x1,y1) and (x2,y2) is given by the formula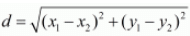
In an equilateral triangle all the sides are of equal length.
Here we are given that A (3, 4) and B (−2, 3) are two vertices of an equilateral triangle. Let C(x, y) be the third vertex of the equilateral triangle.
First let us find out the length of the side of the equilateral triangle.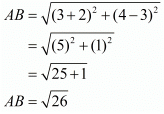
Hence the side of the equilateral triangle measures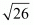 units.
units.
Now, since it is an equilateral triangle, all the sides need to measure the same length.
Hence we have BC=AC
BC=
AC=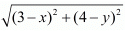
Equating both these equations we have,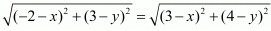
Squaring on both sides we have,
(-2-x)2 + (3-y)2 = (3-x)2 + (4-y)2
4+x2+4x+9+y2 - 6y = 9+x2-6x+16+y2-8y
10x+2y=12
5x+y=6
From the above equation we have,y=6-5x
Substituting this and the value of the side of the triangle in the equation for one of the sides we have,
BC=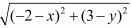
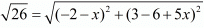
Squaring on both sides,
26=(-2-x)2+(-3+5x)2
26=4+x2+4x+9+25x2-30x
13=26x2-26x
1=2x2-2x
Now we have a quadratic equation for ‘x’. Solving for the roots of this equation,
2x2-2x-1=0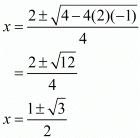
We know that y=6-5x. Substituting the value of ‘x’ we have,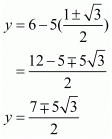
Hence the two possible values of the third vertex are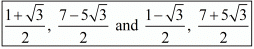
Page No 6.17
Ques.52. Find the circumcentre of the triangle whose vertices are (−2, −3), (−1, 0), (7, −6).
Ans. The distance d between two points (x1, y1) and (x2, y2) is given by the formula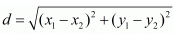
The circumcentre of a triangle is the point which is equidistant from each of the three vertices of the triangle.
Here the three vertices of the triangle are given to be A(−2.−3), B(−1,0) and C(7,−6).
Let the circumcentre of the triangle be represented by the point R(x, y).
So we have AR = BR = CR
AR = 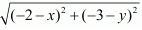
BR =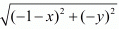
CR =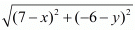
Equating the first pair of these equations we have,
AR = BR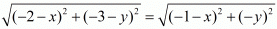
Squaring on both sides of the equation we have,
(-2-x)2+(-3-y)2 = (-1-x)2+(-y)2
4+x2+4x+9+y2+6y = 1+x2+2x+y2
2x+6y=-12
x+3y=-6
Equating another pair of the equations we have,
AR = CR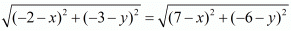
Squaring on both sides of the equation we have,
(-2-x)2+(-3-y)2 = (7-x)2+(-6-y)2
4+x2+4x+9+y2+6y=49+x2-14x+36+y2+12y
18x-6y = 72
3x-y=12
Now we have two equations for ‘x’ and ‘y’, which are
x+3y=-6
3x-y=12
From the second equation we have y=3x-12. Substituting this value of 'y' in the first equation we have,
x+3(3x-12)=-6
x+9x-36=-6
10x=30
x=3
Therefore the value of ‘y’ is,
y=3x-12
=9-12
y=-3
Hence the co−ordinates of the circumcentre of the triangle with the given vertices are (3,-3).
Page No 6.17
Ques.53. Find the angle subtended at the origin by the line segment whose end points are (0, 100) and (10, 0).
Ans. The distance d between two points (x1,y1) and (x2,y2) is given by the formula
d = 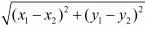
In a right angled triangle the angle opposite the hypotenuse subtends an angle of 90°.
Here let the given points be A(0,100), B(10,0). Let the origin be denoted by O(0,0).
Let us find the distance between all the pairs of points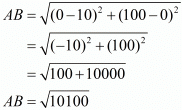

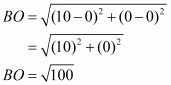
Here we can see that AB2 = AO2 + BO2
So, ΔAOB is a right angled triangle with ‘AB’ being the hypotenuse. So the angle opposite it has to be. This angle is nothing but the angle subtended by the line segment ‘AB’ at the origin.
Hence the angle subtended at the origin by the given line segment is 90°.
Page No 6.17
Question 54. Find the centre of the circle passing through (5,−8), (2,−9) and (2, 1).
Ans. The distance d between two points (x1,y1) and (x2,y2) is given by the formula
The centre of a circle is at equal distance from all the points on its circumference.
Here it is given that the circle passes through the points A(5,−8), B(2,−9) and C(2,1).
Let the centre of the circle be represented by the point O(x, y).
So we have AO = BO = CO
AO =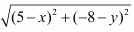
BO =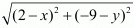
CO =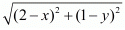
Equating the first pair of these equations we have,
AO = BO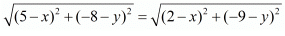
Squaring on both sides of the equation we have,
(5-x)2 + (-8-y)2 = (2-x)2 + (-9-y)2
25+x2-10x+64+y2+16y = 4+x2-4x+81+y2+18y
6x+2y = 4
3x+y = 2
Equating another pair of the equations we have,
AO = CO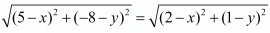
Squaring on both sides of the equation we have,
(5-x)2+(-8-y)2 = (2-x)2 + (1-y)2
25+x2-10x+64+y2+16y = 4+x2-4x+1+y2-2y
6x-18y = 84
x-3y = 14
Now we have two equations for ‘x’ and ‘y’, which are
3x+y=2
x-3y=14
From the second equation we have. Substituting this value of ‘y’ in the first equation we have,
x-3(-3x+2) = 14
x+9x-6 = 14
10x = 20
x = 2
Therefore the value of ‘y’ is,
y=-3x+2
=-3(2)+2
y=-4
Hence the co-ordinates of the centre of the circle are (2,-4).
Page No 6.17
Ques.55. If two opposite vertices of a square are (5, 4) and (1, −6), find the coordinates of its remaining two vertices.
Ans. The distance d between two points and is given by the formula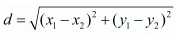
In a square all the sides are of equal length. The diagonals are also equal to √2 each other. Also in a square the diagonal is equal to times the side of the square.
Here let the two points which are said to be the opposite vertices of a diagonal of a square be A(5,4) and C(1,−6).
Let us find the distance between them which is the length of the diagonal of the square.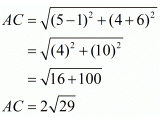
Now we know that in a square,
Side of the square = (Diagonal of the square) / √2
Substituting the value of the diagonal we found out earlier in this equation we have,
Side of the square = 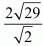
Side of the square = 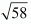
Now, a vertex of a square has to be at equal distances from each of its adjacent vertices.
Let P(x, y) represent another vertex of the same square adjacent to both ‘A’ and ‘C’.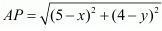

But these two are nothing but the sides of the square and need to be equal to each other.
AP = CP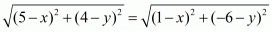
Squaring on both sides we have,
(5-x)2 + (4-y)2 = (1-x)2 + (-6-y)2
25 + x2 - 10x +16 + y2 - 8y = 1+x2-2x+36+y2+12y
8x+20y=4
2x+5y=1
From this we have,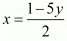
Substituting this value of ‘x’ and the length of the side in the equation for ‘AP’ we have,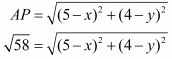
Squaring on both sides,
58 = (5-x)2 + (4-y)2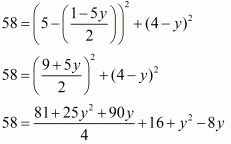
232 = 81+25y2+90y+64+4y2-32y
87 = 29y2+58y
We have a quadratic equation. Solving for the roots of the equation we have,
29y2+58y-87 = 0
29y2+87y-29y-87=0
29y(y+3)-29(y+3)=0
(y+3)(29y-29)=0
(y+3)(y-1)=0
The roots of this equation are −3 and 1.
Now we can find the respective values of ‘x’ by substituting the two values of ‘y’
When y = -3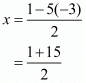
x = 8
When y=1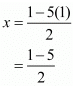
x=-2
Therefore the other two vertices of the square are (8,-3) and (-2,1).
Page No 6.17
Ques. 56. Find the centre of the circle passing through (6, −6), (3, −7) and (3, 3).
Ans. The distance d between two points (x1,y1) and (x2,y2) is given by the formula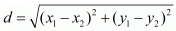
The centre of a circle is at equal distance from all the points on its circumference.
Here it is given that the circle passes through the points A(6,−6), B(3,−7) and C(3,3).
Let the centre of the circle be represented by the point O(x, y).
So we have AO = BO = CO
AO =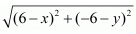
BO = 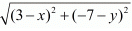
CO = 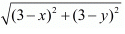
Equating the first pair of these equations we have,
AO = BO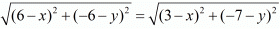
Squaring on both sides of the equation we have,
(6-x)2+(-6-y)2 = (3-x)2+(-7-y)2
36+x2-12x+36+y2+12y = 9+x2-6x+49+y2+14y
6x+2y=14
3x+y=7
Equating another pair of the equations we have,
AO = CO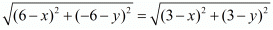
Squaring on both sides of the equation we have,
(6-x)2 + (-6-y)2 = (3-x)2 + (3-y)2
36+x2-12x+36+y2+12y = 9+x2-6x+9+y2-6y
6x-18y = 54
x-3y = 9
Now we have two equations for ‘x’ and ‘y’, which are
3x+y=7
x-3y=9
From the second equation we have. Substituting this value of ‘y’ in the first equation we have,
x-3(-3x+7) = 9
x+9x-21=9
10x=30
x=3
Therefore the value of ‘y’ is,
y=-3x+7
=-3(3)+7
y=-2
Hence the co-ordinates of the centre of the circle are (3,-2).
Page No 6.17
Ques.57. Two opposite vertices of a square are (−1, 2) and (3, 2). Find the coordinates of other two vertices.
Ans. The distance d between two points (x1,y1) and (x2,y2) is given by the formula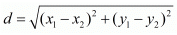
In a square all the sides are of equal length. The diagonals are also equal to each other. Also in a square the diagonal is equal to √2 times the side of the square be A(-1,2) and C(3,2). Here let the two points which are said to be the opposite vertices of a diagonal of a square be A(−1,2) and C(3,2).
Let us find the distance between them which is the length of the diagonal of the square.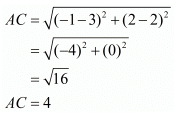
Now we know that in a square,
Side of the square =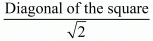
Substituting the value of the diagonal we found out earlier in this equation we have,
Side of the square = 4/√2
Side of the square = 2√2
Now, a vertex of a square has to be at equal distances from each of its adjacent vertices.
Let P(x, y) represent another vertex of the same square adjacent to both ‘A’ and ‘C’.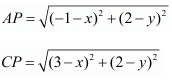
But these two are nothing but the sides of the square and need to be equal to each other.
AP = CP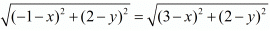
Squaring on both sides we have,
(-1-x)2+(2-y)2 = (3-x)2+(2-y)2
1+x2+2x+4+y2-4y = 9+x2-6x+4+y2-4y
8x=8
x=1
Substituting this value of ‘x’ and the length of the side in the equation for ‘AP’ we have,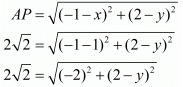
Squaring on both sides,
8 = (-2)2+(2-y)2
8 = 4+4+y2-4y1
0 = y2-4y
We have a quadratic equation. Solving for the roots of the equation we have,
y2-4y=0
y(y-4)=0
The roots of this equation are 0 and 4.
Therefore the other two vertices of the square are (1,0) and (1,4).
Page No 6.28
Ques.1. Find the coordinates of the point which divides the line segment joining (−1,3) and (4, −7) internally in the ratio 3 : 4
Ans. We have A (−1, 3) and B (4,−7) be two points. Let a point P(x,y) divide the line segment joining the points A and B in the ratio 3:4 internally.
Now according to the section formula if point a point P divides a line segment joining A(x1,y1) and B(x2,y2) in the ratio m:n internally than,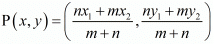
Now we will use section formula to find the co-ordinates of unknown point P as,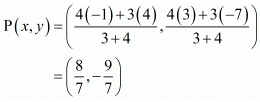
Therefore, co-ordinates of point P is (8/7,-(9/7)).
FAQs on Chapter 7 - Coordinate Geometry, RD Sharma Solutions - (Part-4) - RD Sharma Solutions for Class 10 Mathematics
| 1. How do we find the distance between two points using coordinate geometry? |  |
| 2. How can we determine the midpoint of a line segment in coordinate geometry? |  |
| 3. Can you explain how to find the slope of a line in coordinate geometry? |  |
| 4. How do we determine if two lines are parallel or perpendicular in coordinate geometry? |  |
| 5. Can you explain how to find the equation of a line in coordinate geometry given a point and slope? |  |

|
Explore Courses for Class 10 exam
|

|
















