Exercise 3.8 - Squares And Square Roots RD Sharma Solutions | Mathematics (Maths) Class 8 PDF Download
Q.1. Find the square root of each of the following correct to three places of decimal.
(i) 5
(ii) 7
(iii) 17
(iv) 20
(v) 66
(vi) 427
(vii) 1.7
(viii) 23.1
(ix) 2.5
(x) 237.615
(xi) 15.3215
(xii) 0.9
(xiii) 0.1
(xiv) 0.016
(xv) 0.00064
(xvi) 0.019
(xvii) 7/8
(xviii) 5/12
(xix)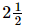
(xx)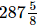
Ans:
(i) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
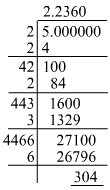
Hence, the square root of 5 up to three decimal places is 2.236.
(ii) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 7 up to three decimal places is 2.646.
(iii) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 17 up to three decimal places is 4.123.
(iv) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 20 up to three decimal places is 4.472.
(v) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
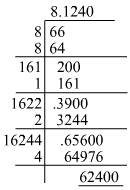
Hence, the square root of 66 up to three decimal places is 8.124.
(vi) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 427 up to three decimal places is 20.664.
(vii) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
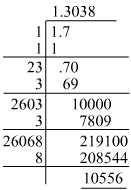
Hence, the square root of 1.7 up to three decimal places is 1.304.
(viii) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 23.1 up to three decimal places is 4.806.
(ix) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
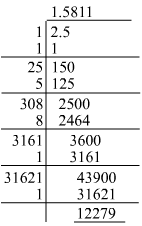
Hence, the square root of 2.5 up to three decimal places is 1.581.
(x) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 237.615 up to three decimal places is 15.415.
(xi) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
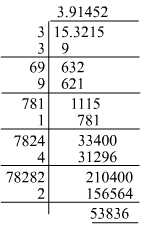
Hence, the square root of 15.3215 up to three decimal places is 3.914.
(xii) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 0.9 up to three decimal places is 0.949.
(xiii) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
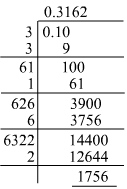
Hence, the square root of 0.1 up to three decimal places is 0.316.
(xiv) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
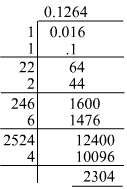
Hence, the square root of 0.016 up to three decimal places is 0.126.
(xv) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.
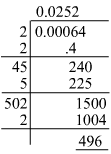
Hence, the square root of 0.00064 up to three decimal places is 0.025.
(xvi) We can find the square root up to three decimal places by using long division until we get four decimal places and then rounding it to three decimal places.

Hence, the square root of 0.019 up to three decimal places is 0.138.
(xvii) We can find the square root up to four decimal places by expanding 7/8 to decimal form up to eight digits to the right of the decimal point as shown below:
7/8 = 0.875
Hence, we have:

So, the square root of 7/8 up to three decimal places is 0.935.
(xviii) We can find the square root up to four decimal places by expanding 5/12 to decimal form up to eight digits to the right of the decimal point as shown below:
5/2=0.41666666
Hence, we have:

So, the square root of 5/12 up to three decimal places is 0.645.
(xix) We can find the square root up to four decimal places by expanding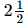 into decimal form up to eight digits to the right of the decimal point as shown below:
into decimal form up to eight digits to the right of the decimal point as shown below:
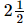 =2.50000000
=2.50000000
But, this is the same with the value 2.5 in problem (ix). Hence, the square root of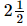 is 1.581.
is 1.581.
(xx) We can find the square root up to four decimal places by expanding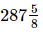 into decimal form up to eight digits to the right of the decimal point as shown below:
into decimal form up to eight digits to the right of the decimal point as shown below:
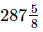 =287.62500000
=287.62500000
Hence, we have:

So, the square root of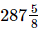 up to three decimal places is 16.960.
up to three decimal places is 16.960.
Q.2. Find the square root of 12.0068 correct to four decimal places.
Ans:
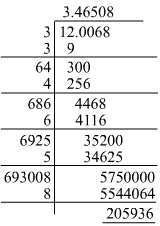
∴ √12.0068 = 3.46508
We can round it off to four decimal places, i.e. 3.4651.
Q.3. Find the square root of 11 correct to five decimal places.
Ans: Using the long division method:
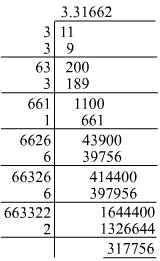
∴ √11 = 3.31662
Q.4. Given that: √2 = 1.414, √3 = 1.732, √5 = 2.236 and √7= 2.646, evaluate each of the following:
(i)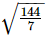
(ii)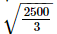
Ans: Given: √7 = 2.646
(i)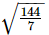 =
=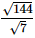 =
=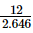 = 4.536
= 4.536
Given: √3 = 1.732
(ii) 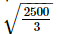 =
=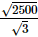 =
=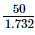 = 28.867
= 28.867
Q.5. Given that: √2 = 1.414, √3 = 1.732, √5 = 2.236 and √7= 2.646, find the square roots of the following:
(i)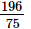
(ii)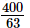
(iii)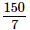
(iv)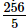
(v)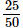
Ans: From the given values, we can simplify the expressions in the following manner:
(i)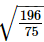 =
=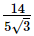 =
=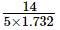 = 1.617
= 1.617
(ii)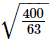 =
=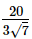 =
=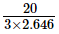 = 2.520
= 2.520
(iii)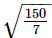 =
=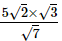 =
=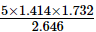 = 4.628
= 4.628
(iv)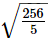 =
=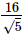 =
=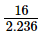 = 7.155
= 7.155
(v)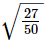 =
=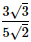 =
=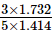 = 0.735
= 0.735
|
81 videos|452 docs|31 tests
|
















