Bending & Shear Stress in Beams - 1 | Solid Mechanics - Mechanical Engineering PDF Download
Bending and Shear Stress
- Bending Moments and Shear Stress Distribution: Bending stress and shear stress distribution are classified in the following groups
- Bending Moment in Beam:
Transverse loads or lateral loads: Forces or moments having their vectors perpendicular to the axis of the bar.
Classification of structural members
- Axially loaded bars: Loaded in perpendicular dimension along the axis of bar. Supports forces having their vectors directed along the axis of the bar.
- Bar in tension: Supports torques having their moment vectors directed along the axis to generate a force in the outward direction
- Beams: Subjected to lateral loads. Beams undergo bending (flexure) because of lateral loads.
When beam is subjected to a bending moment or bent there are induced longitudinal or bending stress in cross-section.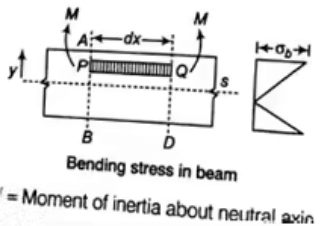
 Note that a positive bending moment M causes negative (compressive) stress above the neutral axis and positive (tensile) stress below the neutral axis
Note that a positive bending moment M causes negative (compressive) stress above the neutral axis and positive (tensile) stress below the neutral axis
Equation of Pure Bending
1. Assumptions
- The material of the beam is homogeneous and isotropic.
- The value of Young's Modulus of Elasticity is same in tension and compression.
- The transverse sections which were plane before bending, remain plane after bending also.
- The beam is initially straight and all longitudinal filaments bend into circular arcs with a common centre of curvature.
- The radius of curvature is large as compared to the dimensions of the cross-section.
- Each layer of the beam is free to expand or contract, independently of the layer, above or below it.
At the neutral axis, there is no stress of any kind. At one side of the neutral axis, there are compressive stresses and on the other side, there are tensile stresses.
2. Modulus of Section
Elastic section modulus is defined as Z(S) = I / y, where I is the second moment of area (or Izz moment of inertia) and y is the distance from the neutral axis to any given fibre.
Section Modulus- Section Modulus is a geometric property used to calculate the bending stresses in a structural member.
- Mathematically, It is defined as the ratio of the moment of inertia of a section about its centroidal axis and to the distance of the extreme layer from the neutral axis. It is denoted by Z.
- Mathematically By bending stress equation,
- M/I = σmax/ymax
- M = σmax × (I/ymax)
- I = Moment of Inertia(m⁴)
- y = distance from NA to extreme layer(m)
- The unit of section modulus is m³
- Elastic Section Modulus
- Plastic Section Modulus
- Elastic section modulus is calculated when we are to use the material behavior till the yield point.
- For general design purposes, we use elastic section modulus.
- Generally, elastic section modulus is denoted by Zₑ.
- I = Moment of inertia
- ymax = Distance from NA to extreme layer
Rectangular Section
As we know the moment of inertia of the rectangular section,
And the distance from the neutral axis,
ymax = d/2
So,
Zₑ = I/ymax
Zₑ = (bd³/12)/(d/2)
After calculating them, section modulus of rectangular section,
Zₑ = bd²/6
Hollow Rectangular Section

ymax = D/2
After putting these values,
Zₑ = I/ymax
(BD³/12) - (bd³/12)/(D/2)
Solid Circular

ymax = d/2
Then,
Zₑ = {(π/64) × d⁴}/(d/2)
Hollow Circular

And,
ymax = D/2
After putting these values,
Zₑ = (π/64) × (D⁴ - d⁴)/(D/2)
Symmetric I & C Section

ymax = D/2
So,
Zₑ = [(BD³/12) - {(B - b)d³}/12]/(D/2)
Diamond
Plastic Section Modulus
- The plastic section modulus is used for materials where the elastic yield is acceptable and the plastic behavior is considered to be an acceptable range.
- It depends on the location of the plastic neutral axis.
- The PNA is the axis that bisects the cross-section such that the compressive force from the region in compression is equal to the tension force from the region under tension.
- So, for sections with a constant yield stress, the area above and below the PNA will be equal, but for mixed sections, this is not necessarily the case.

- Ac = Area of cross-section under compression.
- Aₜ = Area of cross-section under tension.
- yc = Distance from the plastic neutral axis to the center of gravity of the areas under compression.
- yₜ = Distance from the plastic neutral axis to the center of gravity of areas under tension.
Rectangular Section

Ac = Aₜ And, yc = yₜ
Ac = Aₜ = bd/2
yc = yₜ = d/4
So,
Zₚ = (Ac × yc) + (Aₜ + yₜ)
Zₚ = (bd/2 × d/4) + (bd/2 × d/4)
Circular Section

Ac = Aₜ = πd²/8
yc = yₜ = 2d/3π
Zₚ = (πd²/8 × 2d/3π) + (πd²/8 × 2d/3π)
Zₚ = (D³ - d³)/6
3. Beams of uniform strength
- The beam is said to be in uniform strength if the maximum bending stress is constant across the varying section along its length.
- Generally, beams are having the uniform cross-section throughout their length. When a beam is loaded, there is a variation in bending moment from section to section along the length. The stress in extreme outer fibre (top and bottom) also varies from section to section along their length. The extreme fibers can be loaded to the maximum capacity of permissible stress (say pmax), but they are loaded to less capacity. Hence, in beams of the uniform cross section there is a considerable waste of materials.
- When a beam is suitably designed such that the extreme fibers are loaded to the maximum permissible stress pmax by varying the cross section it will be known as a beam of uniform strength.
Shearing Stress
Shearing stress on a layer JK of beam at distance y from neutral axis.
Where,
V = Shearing force
First moment of area
1. Shear stress in Rectangular Beam
Suppose, we have to determine the shear stress at the longitudinal layer having y distance from neutral axis.
Tmax = 3 / 2(V / A)
Tmax = 15Tav
2. Circular Beam
Centre of gravity of semi-circle lies at distance from centre or base line. As it is symmetrical above neutral axis, hence at neutral axis shear stress will be maximum.
3. Shears Stress in Hollow Circular Cross-Section
In hollow circular cross-section, if we have to calculate τ at neutral axis by the formula
4. Shear Stress in Triangular Section
In a triangular cross-section, if we have to calculate τ at neutral axis, then in formula
Shear Stress in I-section
Combined Stress
Bending stress and shear stress distribution are classified in the following groups
1. Bending Moment in Beam
Transverse loads or lateral loads: Forces or moments having their vectors perpendicular to the axis of the bar.
Classification of structural members
- Axially loaded bars: Supports forces having their vectors directed along the axis of the bar.
- Bar in tension: Supports torques having their moment vectors directed along the axis.
- Beams
(i) Subjected to lateral loads.
(ii) Beams undergo bending (flexure) because of lateral loads.
When beam is subjected to a bending moment or bent there are induced longitudinal or bending stress in cross-section.
2. Bending Stress in beam

- I is Moment of Inertia about Neutral Axis
- Note that a positive bending moment M causes negative (compressive) stress above the neutral axis and positive ( tensile) stress below the neutral axis
Equation of Pure Bending


1. Assumptions:
- The material of the beam is homogeneous and isotropic.
- The value of Young's Modulus of Elasticity is same in tension and compression.
- The transverse sections which were plane before bending, remain plane after bending also, that means strain diagram is linear.
- The beam is initially straight and all longitudinal filaments bend into circular arcs with a common centre of curvature.
- The radius of curvature is large as compared to the dimensions of the cross-section.
- Each layer of the beam is free to expand or contract, independently of the layer, above or below it.
2. Modulus of Section
- Elastic section modulus is defined as Z(S) = I / y, where I is the second moment of area (or Izz moment of inertia) and y is the distance from the neutral axis to any given fibre.
- Section modulus is a geometric property for a given cross-section used in the design of beams or flexural members.

- Rectangular section:
I = bd3 / 12
Modulus of the section : z = bd2 / 6 - Circular section:
I = (π / 64)d4
Modulus of section:
z = (π / 32)d3
2. Beams of uniform strength
- The beam is said to be in uniform strength if the maximum bending stress is constant across the varying section along its length.
- Generally, beams are having the uniform cross-section throughout their length. When a beam is loaded, there is a variation in bending moment from section to section along the length. The stress in extreme outer fibre (top and bottom) also varies from section to section along their length. The extreme fibres can be loaded to the maximum capacity of permissible stress (say pmax), but they are loaded to less capacity. Hence, in beams of uniform cross section, there is a considerable waste of materials
- When a beam is suitably designed such that the extreme fibres are loaded to the maximum permissible stress pmax by varying the cross section it will be known as a beam of uniform strength.
3. Shearing Stress
- Shearing stress on a layer JK of beam at distance y from neutral axis.


Where,
- V = Shearing force
 First moment of area
First moment of area
T = VQ / lb
4. Shear stress in Rectangular Beam
- Suppose, we have to determine the shear stress at the longitudinal layer having y distance from neutral axis.



Tmax = 3V/2A
Tmax = 1.5Tav
5. Circular Beam
- Centre of gravity of semi-circle lies at distance from centre or base line. As it is symmetrical above neutral axis, hence at neutral axis shear stress will be maximum.

- For Tmax subsitituting y = 0
l = πd4/64
Tmax = 4V/3A = 4/3 Tav
6. Shears Stress in Hollow Circular Cross-Section
In hollow circular cross-section, if we have to calculate τ at neutral axis by the formula
7. Shear Stress in Triangular Section
In a triangular cross-section, if we have to calculate τ at neutral axis, then in formula
τ = (V/3l)(hx - x2) ⇒ τmax = 3/2τav
8. Shear Stress in I-section

Combined Stresses
Definitions of Direct, Bending & Shear Stress
1. Stress due to Twisting Moment + Bending Moment
- Equivalent Torsional Moment:
The equivalent torsional moment is defined as the torsional moment, which when acting alone, will produce the same torsional shear stress in the shaft as under the combined action of bending moment (Mb) and torsional moment (Mt)
Equivalent torsional moment= Te=
- Equivalent Bending Moment:
The equivalent Bending moment is defined as the bending moment, which when acting alone, will produce the same bending stresses (tensile and compressive) in the shaft as under the combined action of bending moment (Mb) and torsional moment (Mt).
Equivalent bending moment = Me = 1/2[Mb + - ]
]
Example: A shaft of diameter 8 cm is subjected to a bending moment of 3000 Nm and a twisting moment of 4000 Nm. The maximum normal stress induced in the shaft is equal to
Solution:
2. Stress due to Axial Load + Bending Moment
Suppose a beam under direct compressive and bending load as shown in the diagram. where A = Area,
y = distance of extreme fiber from Neutral axis and I= moment of Inertia
Direct compressive stress σ = F / A
Bending stress will vary linearly from center to extreme fiber σb = My / I
so total stress at upper fiber
= Direct compressive stress + tensile stress due to a bending load
= (-σ) + σb
= -(F/A) + (My/I)
Same for lower extreme fiber
= Direct compressive stress + Compressive stress due to bending load
= (-σ) + (-σb)
= -(F/A) - (MY/I)
Example: For the component loaded with a force F as shown in the figure, the axial stress at the corner point P is
Solution:
At point P two types of stress are acting, bending & axial tensile load
So, bending stress =
Axial tensile stress =
σa = P / A = F / (2b)2 = F / 4b2
Total axial stress at P = σb + σa
|
33 videos|27 docs|29 tests
|
FAQs on Bending & Shear Stress in Beams - 1 - Solid Mechanics - Mechanical Engineering
| 1. What is bending stress in beams? |  |
| 2. How is bending stress calculated in beams? |  |
| 3. What is shear stress in beams? |  |
| 4. How is shear stress calculated in beams? |  |
| 5. What is the relationship between bending stress and shear stress in beams? |  |

|
Explore Courses for Mechanical Engineering exam
|

|



 First moment of area
First moment of area






 ]
]
















