Vertical Stress in Ground | Soil Mechanics - Civil Engineering (CE) PDF Download
Stress Distribution in The Soil
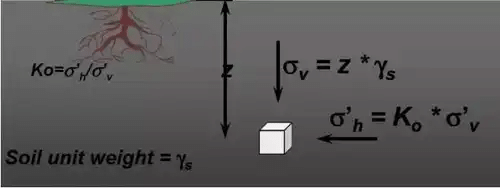
At a point within a soil mass, stresses will be developed as a result of the soil lying above the point and by any structural or other loading imposed onto that soil mass.
Stress in the soil may be caused by:
- Self weight of soil
- Applied load on soil
Finitely loaded area
If the surface loading area is finite (point, circular, strip, rectangular, square), the vertical stress increment in the subsoil decreases with increase in the depth and the distance from the surface loading area.
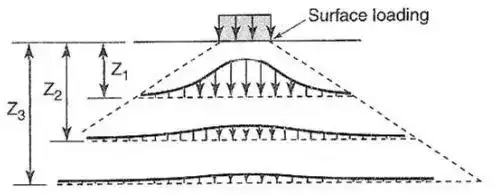
Methods have been developed to estimate the vertical stress increment in sub-soil considering the shape of the surface loading area.
Boussinesq's Theory
- Point Load
A point load or a Concentrated load is, strictly speaking, hypothetical in nature, consideration of it serves a useful purpose in arriving at the solutions for more complex loadings in practice. - Assumptions made by Boussinesq
(i) The soil medium is an elastic, homogeneous, isotropic and semi-infinite medium, which infinitely in all directions from a level surface.
(ii) The medium obeys Hookes law.
(iii) The self-weight of the soil is ignored.
(iv) The soil is initially unstressed
(v) The change in volume of the soil upon application of the loads onto it is neglected.
(vi) The top surface of the medium is free of shear stress and is subjected to only the point load at a specified location.
(vii) Continuity of stress is considered to exist in the medium.
(viii) The stresses are distributed symmetrically with respect to z-axis.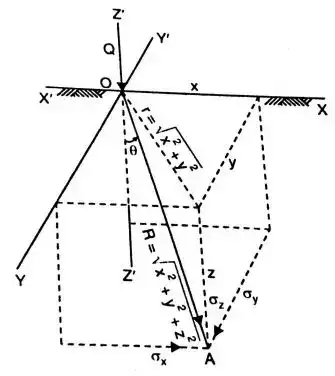 The Boussinesq equations are as follows:
The Boussinesq equations are as follows: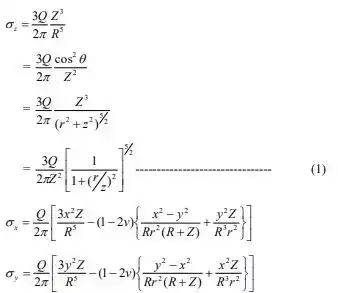
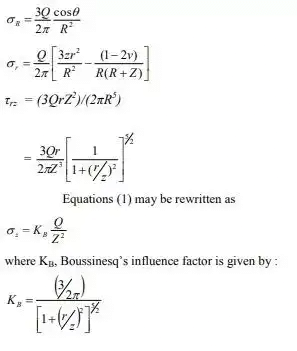
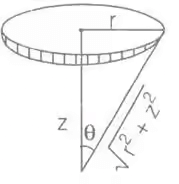
This intensity of vertical stress directly below the point load, on its axis of loading (r = 0) is given by:
σz = 0.4775Q / Z2
The vertical stress on a horizontal plane at depth „Z‟ is given by
σz = KB(Q / Z2)
Z being a specified depth.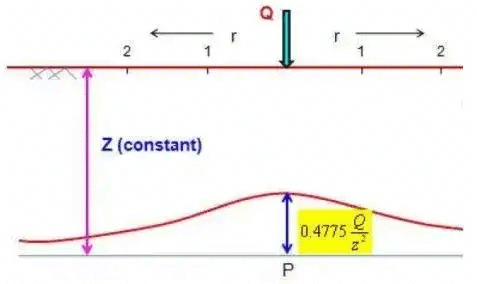
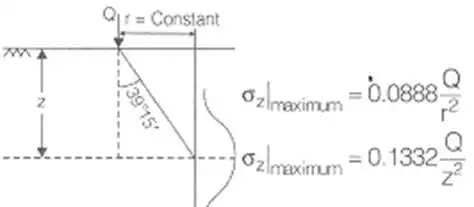
- Boussinesq's Result
σz|max = 0.0888(Q / r2)
σz|max = 0.1332(Q2 / 22)
Westergaard's Theory
(i) 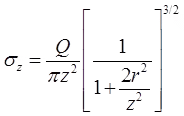
(ii) σz= kW.(Q / z2)
(iii) kw|max = 0.3183
- Westergaard's Results
(i) Vertical Stresss due to Live Loads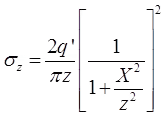
where, σz = Vertical stress of any point having coordinate (x, z)
Load intensity = q' / m
at X = 0
σz = 2q' / πz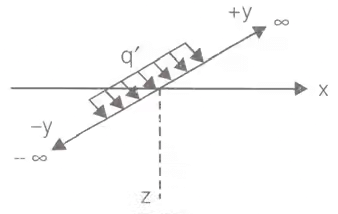 (ii) Vertical Stress due to Strip Loading
(ii) Vertical Stress due to Strip Loading
σz = 2q' / π((X / B)(α) - (sin2β / 2))
where, σz = Vertical stress at point 'p'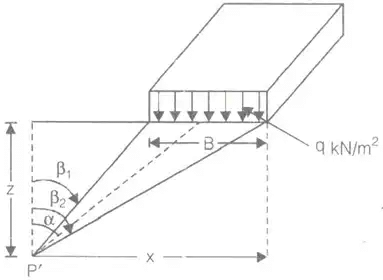 (iii) σz = q / π[β + sinβ]
(iii) σz = q / π[β + sinβ]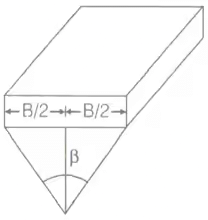 (iv) Vertical stress below uniform load acting on a circular area.
(iv) Vertical stress below uniform load acting on a circular area.
σz = q(1 - cos3 θ)
where, cosθ =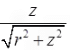
Newmark's Chart Method (Uniform Load on irregular Areas)
- Newmark (1942) constructed influence chart , based on the Boussinesq solution to determine the vertical stress increase at any point below an area of any shape carrying uniform pressure.
- This method is applicable to semi-infinite, homogeneous, isotropic and elastic soil mass. It is not applicable for layered structure.
- The greatest advantage of this method is that it can be applied for a uniformly distributed area of an irregular shape.
- Chart consists of influence areas which have an influence value of 0.005 per unit pressure.
- Position the loaded area on the chart such that the point at which the vertical stress required is at the centre of the chart.
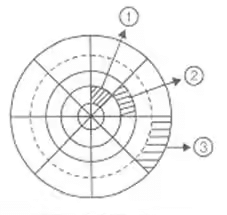
- Newmark's chart is made of concentric circles and radial lines. Normally there are 10 concentric circles and 20 radial lines.
- No. of concentric circle = 10
No. of radial lines = 20
Influence of area (1) = Influence of area (2) = Influence of area (3)
Influence of each area
= 1 / Total no. of sectoral area = 0.005
σz = 0.005qNA
where, NA = Total number of sectorial area of Newmark's chart.
Approximate method
- Equivalent Load Method
σz = σz1 + σz2 + σz3 + ...
where,
σz1 = kB1(Q1/Z2)σz2 = kB2 . (Q22/Z2) ...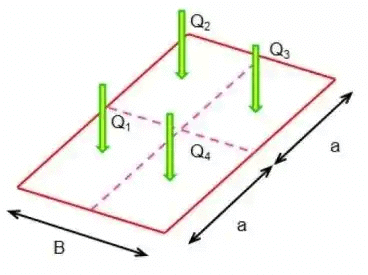
- Trapezoidal Method
σz at depth 'z' = q(BxL) / (B + 2ηz)(L + 2ηz)
For 1H : 1 V
σz = q(BxL) / (B + 2z)(L + 2z)
σz = q(BxL) / (B + 4z)(L + 4z)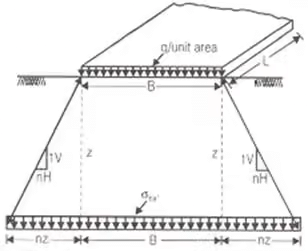
- Stress Isobar Method
Area bounded by 0.2q stress isobar is considered to be stressed by vertical stress on loading.
0.2q = 20% Stress Isobar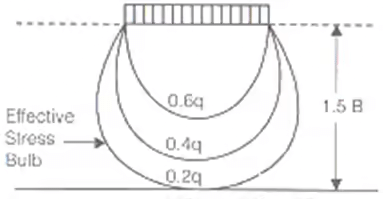
Q. A concentrated load of 22.5 KN acts on thee surface of a homogeneous soil mass of large extent. Find the stress intensity at a depth of 15 metres and (i) directly under the load, and (ii) at a horizontal distance of 7.5 metres. Use Boussinesq's equations.
Ans: According to Boussinesq's theory,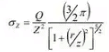
(i) Directly under the load:
r = 0; ∴r/z = 0
z = 15m, Q = 22.5 KN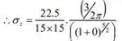
= 47.75 N/m2
(ii) At a horizontal distance of 7.5 metres:
r = 7.5m, z = 15m
r/z = 7.5/15 = 0.5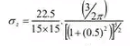
= 27.33 N/m2
|
30 videos|126 docs|74 tests
|
FAQs on Vertical Stress in Ground - Soil Mechanics - Civil Engineering (CE)
| 1. What is vertical stress in ground in civil engineering? |  |
| 2. How is vertical stress in ground calculated in civil engineering? |  |
| 3. What are the factors influencing vertical stress in ground in civil engineering? |  |
| 4. How does vertical stress in ground affect civil engineering projects? |  |
| 5. How can vertical stress in ground be managed in civil engineering? |  |
















