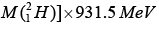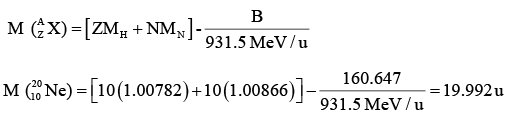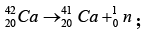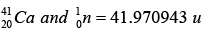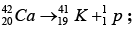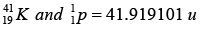Basic Nuclear Properties | Modern Physics PDF Download
| Table of contents |

|
| Introduction |

|
| Size and Density |

|
| Spin and Magnetic Moment |

|
| Angular Momentum of Nucleus |

|
| Stable Nuclei |

|
| Binding Energy |

|
Introduction
An ordinary hydrogen atom has as its nucleus a single proton, whose charge is +e and whose mass is 1836 times that of the electron. All other elements have nuclei that contain neutrons as well as protons. As its name suggests, the neutron is uncharged; its mass is slightly greater than that of the proton. Neutrons and protons are jointly called nucleons. The atomic number of an element is the number of protons in each of its nuclei, which is the same as the number of electrons in a neutral atom of the element. Thus atomic number of hydrogen is 1, of helium 2, of lithium 3, and of uranium 92. All nuclei of a given element do not necessarily have equal numbers of neutrons. For instance, although over 99.9 percent of hydrogen nuclei are just single protons, a few also contain a neutron, and a very few two neutrons, along with the protons. The varieties of an element that differ in the numbers of neutrons their nuclei contain are called isotopes.
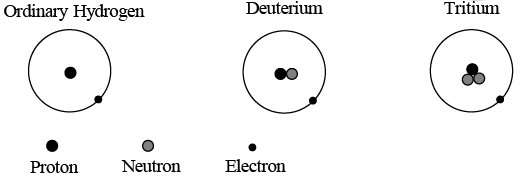 The isotope of hydrogen
The isotope of hydrogen
The conventional symbols for nuclear species, or nuclides, follow the pattern AZ X, where
X = Chemical symbol of the element
Z = Atomic number of the element
= Number of protons in the nucleus
A = Mass number of the nuclide
= Number of nucleons in the nucleus
Nuclear Terminology
- Isotopes
If two nuclei have same atomic number Z (proton), then they are called as isotopes.
Example: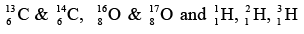
- Isotones
If two nuclei have same neutron number N (proton), then they are called as isotones. Example: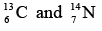
- Isobars
If two nuclei have same mass number A, then they are called as isobars.
Example: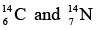
- Mirror nuclei
Nuclei with same mass number A but with proton and neutron number interchanged and their difference is ±1.
Example: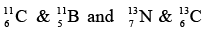
Atomic Masses: Atomic masses refer to the masses of neutral atoms, not of bare nuclei. Thus an atomic mass always includes the masses of Z electrons. Atomic masses are expressed in mass units (u), which are so defined that the mass of a 126 C atom is exactly 12u. The value of mass unit is 1u = 1.66054 x 10-27kg ≈ 931.4 MeV.
Some rest masses in various units are: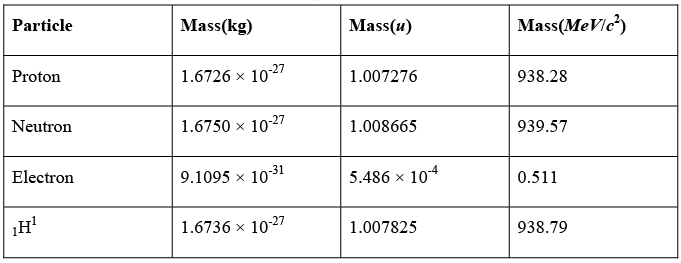
Size and Density
Majority of atomic nuclei have spherical shape and only very few show departure from spherical symmetry. For spherically symmetrical nuclei, nuclear radius is given by
R = R0A1/3
where A is the mass number and R0 = (1.2 ± 0.1) x 10-15 m ≈ 1.2 fm.
R varies slightly from one nucleus to another but is roughly constant for A > 20.
The radius of 126 C nucleus is
R = (1.2)(12)1/3 ≈ 1.2 fm
Example 1: The radius of Ge nucleus is measured to be twice the radius of 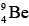 . How many nucleons are there in Ge nucleus?
. How many nucleons are there in Ge nucleus?
R = R0(A)1/3 ⇒ RGe = 2RBe ⇒ R0(A)1/3 = 2R0(9)1/3 ⇒ A = 72
Nuclear Density
Assuming spherical symmetry, volume of nucleus is given by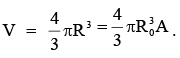
Mass of one proton = 1.67 × 10-27 kg, Nuclear Mass = A × 1.67 × 10-27 kg.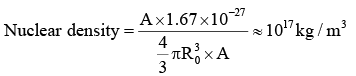
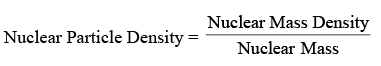
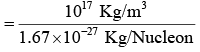
= 1044 Nucleons/m3
Spin and Magnetic Moment
Proton and neutrons, like electrons, are fermions with spin quantum numbers of s = 1/2.
This means they have spin angular momenta 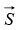 of magnitude
of magnitude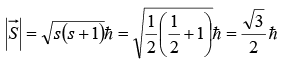
and spin magnetic quantum number of ms = ± 1/2.
As in the case of electrons, magnetic moments are associated with the spins of protons and neutrons. In nuclear physics magnetic moments are expressed in nuclear magnetons, (μN), where
Nuclear magneton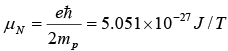
= 3.152 x 10-8 eV/T where mp is the proton mass.
In atomic physics we have defined Bohr magneton 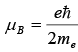 where me is the electron mass.
where me is the electron mass.
The nuclear magneton is smaller than the Bohr magneton by the ratio of the proton mass to the electron mass which is 1836.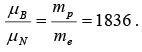
The spin magnetic moments of the proton and neutron have components in any direction of
Proton μpz = ±2.793 μN
Neutron 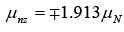
There are two possibilities for the signs of μpz and μnz, depending on whether ms is 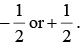 The ± sign is used for μpz because
The ± sign is used for μpz because 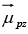 is in the same direction as the spin
is in the same direction as the spin  whereas
whereas 
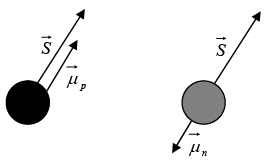 Spin magnetic moment
Spin magnetic moment 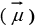 and spin angular momentum
and spin angular momentum 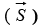 directions for neutron and protons.
directions for neutron and protons.
Note: For neutron, magnetic moment is expected to be zero as e = 0 but 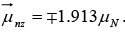
At first glance it seems odd that the neutron, with no net charge, has spin magnetic moment. But if we assume that the neutron contains equal amounts of positive and negative charge, a spin magnetic moment arise if these charges are not uniformly distributed. Thus we can say that neutron has physical significance of negative charges because magnetic moment is opposite to that of its intrinsic spin angular momentum.
Angular Momentum of Nucleus
The hydrogen nucleus 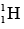 consists of a single proton and its total angular momentum is given by
consists of a single proton and its total angular momentum is given by 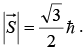 A nucleon in a more complex nucleus may have orbital angular momentum due to motion inside the nucleus as well as spin angular momentum. The total angular momentum of such a nucleus is the vector sum of the spin and orbital angular momenta of its nucleons, as in the analogous case of the electrons of an atom.
A nucleon in a more complex nucleus may have orbital angular momentum due to motion inside the nucleus as well as spin angular momentum. The total angular momentum of such a nucleus is the vector sum of the spin and orbital angular momenta of its nucleons, as in the analogous case of the electrons of an atom.
When a nucleus whose magnetic moment has z component μz is in a constant magnetic field 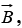 the magnetic potential energy of the nucleus is
the magnetic potential energy of the nucleus is
Magnetic energy
Um = -μzB
The energy is negative when 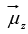 is in the same direction as
is in the same direction as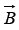 and positive when
and positive when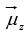 is opposite to
is opposite to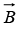 . In a magnetic field, each angular momentum state of the nucleus is therefore split into components, just as in the Zeeman Effect in atomic electron states. Figure below shows the splitting when the angular momentum of the nucleus is due to the spin of a single proton.
. In a magnetic field, each angular momentum state of the nucleus is therefore split into components, just as in the Zeeman Effect in atomic electron states. Figure below shows the splitting when the angular momentum of the nucleus is due to the spin of a single proton.
The energy levels of a proton in a magnetic field are split into spin-up and spin-down sublevels.
The energy difference between the sublevels is
ΔE = 2μpzB
A photon with this energy will be emitted when a proton in the upper state flips its spin to fall to the lower state. A proton in the lower state can be raised to upper one by absorbing a photon of this energy. The photon frequency vL that corresponds to ΔE is
Larmor frequency for photons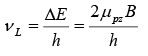
This is equal to the frequency with which a magnetic dipole precesses around a magnetic field.
Stable Nuclei
Not all combination of neutrons and protons form stable nuclei. In general, light nuclei (A < 20) contain equal numbers of neutrons and protons, while in heavier nuclei the proportion of neutrons becomes progressively greater. This is evident in figure as shown below, which is plot of N versus Z for stable nuclides.
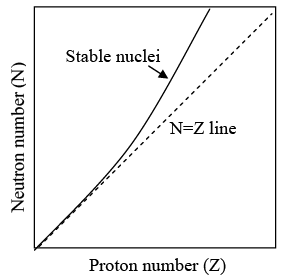 Neutron-proton diagram for stable nuclides.
Neutron-proton diagram for stable nuclides.
The tendency for N to equal Z follows from the existence of nuclear energy levels.
Nucleons, which have spin 1/2, obey exclusion principle. As a result, each energy level can contain two neutrons of opposite spins and two protons of opposite spins. Energy levels in nuclei are filled in sequence, just as energy levels in atoms are, to achieve configurations of minimum energy and therefore maximum stability. Thus the boron isotope 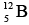 has more energy than the carbon isotope
has more energy than the carbon isotope 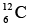 because one of its neutrons is in a higher energy level, and
because one of its neutrons is in a higher energy level, and 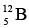 is accordingly unstable. If created in a nuclear reaction, a
is accordingly unstable. If created in a nuclear reaction, a 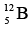 nucleus changes by beta decay into a stable
nucleus changes by beta decay into a stable 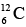 nucleus in a fraction of second.
nucleus in a fraction of second.
 Simplified energy level diagrams of some boron and carbon isotopes.
Simplified energy level diagrams of some boron and carbon isotopes.
The preceding argument is only part of the story. Protons are positively charged and repel one another electrically. This repulsion becomes so great in nuclei with more than 10 protons or so that an excess of neutrons, which produce only attractive forces is required for stability. Thus the curve departs more and more from N = Z line as Z increases.
Sixty percent of stable nuclides have both even Z and even N; these are called “even-even” nuclides. Nearly all the others have either even Z and odd N (“even-odd” nuclides) or odd Z and even N (“odd-even” nuclides) with the numbers of both kinds being about equal. Only five stable odd-odd nuclides are known: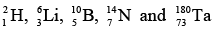
Nuclear abundances follow a similar pattern of favoring even numbers for Z and N.
These observations are consistent with the presence of nuclear energy levels that can each contain two particles of opposite spin. Nuclei with filled levels have less tendency to pick up other nucleons than those with partially filled levels and hence were less likely to participate in the nuclear reactions involved in the formation of elements.
Nuclear forces are limited in range, and as a result nucleons interact strongly only with their nearest neighbors. This effect is referred to as the saturation of nuclear forces. Because the coulomb repulsion of protons is appreciable throughout the entire nucleus, there is a limit to the ability of neutrons to prevent the disruption of large nucleus. This limit is represented by the bismuth isotope 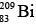 which is the heaviest stable nuclide.
which is the heaviest stable nuclide.
Alpha Decay
All nuclei with Z > 83 and A > 209 spontaneously transform themselves lighter ones through the emission of one or more alpha particles, which are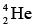 nuclei:
nuclei: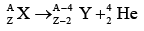
Since an alpha particle consists of two protons and two neutrons, an alpha decay reduces the Z and N of the original nucleus by two each. If the resulting daughter nucleus has either too small or too large a neutron/proton ratio for stability, it may beta-decay to a more appropriate configuration.
Negative beta decay
In negative beta decay, a neutron is transformed into a proton and an electron is emitted:
n0 → p+ + e-
Positron emission
In positive beta decay, a proton becomes a neutron and a positron is emitted:
p+ → n0 + e+
Thus negative beta decay decreases the proportion of neutrons and positive beta decay increases it.
Electron Capture
A process that competes with positron emission is the capture by a nucleus of an electron from its innermost shell. The electron is absorbed by a nuclear proton which is thereby transformed into neutron:
p+ + e- → n0
Figure below shows how alpha and beta decays enable stability to be achieved.
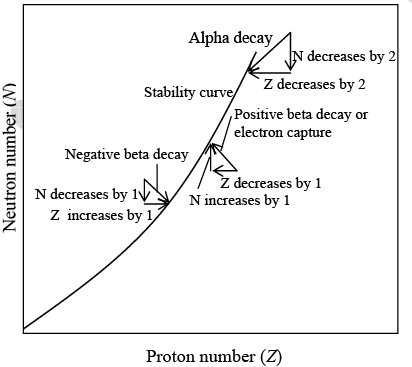 Alpha and beta decays permit an unstable nucleus to reach a stable configuration.
Alpha and beta decays permit an unstable nucleus to reach a stable configuration.
Binding Energy
When nuclear masses are measured, it is found that they are less than the sum of the masses of the neutrons and protons of which they are composed. This is in agreement with Einstein’s theory of relativity, according to which the mass of a system bound by energy B is less than the mass of its constituents by B / c2 (where c is the velocity of light).
The Binding energy B of a nucleus is defined as the difference between the energy of the constituent particles and of the whole nucleus. For a nucleus of atom 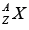
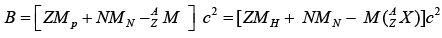
If mass is expressed in atomic mass unit
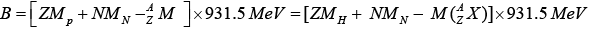
Mp : Mass of free proton,
MN : MN: Mass of free neutron,
MH : mass of hydrogen atom  : mass of the nucleus,
: mass of the nucleus,
Z : Number of proton,
N : Number of neutron,
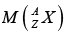 : mass of atom.
: mass of atom.
Binding Energy per Nucleon
The binding energy per nucleon for a given nucleus is found by dividing its total binding energy by the number of nucleon it contains. Thus binding energy per nucleon is
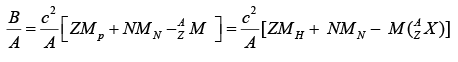
The binding energy per nucleon for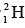 is 2.224/2 = 1.112 MeV / nucleon and for
is 2.224/2 = 1.112 MeV / nucleon and for 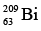
it is 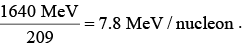
Figure below shows the binding energy per nucleon against the number of nucleons in various atomic nuclei. 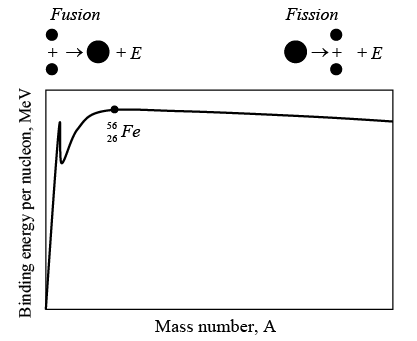 Binding energy per nucleon as function of mass number.
Binding energy per nucleon as function of mass number.
The greater the binding energy per nucleon, the more stable the nucleus is. The graph has the maximum of 8.8 MeV / nucleon when the number of nucleons is 56. The nucleus that has 56 protons and neutrons is 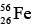 an iron isotope. This is the most stable nucleus of them all, since the most energy is needed to pull a nucleon away from it. Two remarkable conclusions can be drawn from the above graph.
an iron isotope. This is the most stable nucleus of them all, since the most energy is needed to pull a nucleon away from it. Two remarkable conclusions can be drawn from the above graph.
(i) If we can somehow split a heavy nucleus into two medium sized ones, each of the new nuclei will have more binding energy per nucleon than the original nucleus did. The extra energy will be given off, and it can be a lot. For instance, if the uranium nucleus 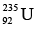 is broken into two smaller nuclei, the binding energy difference per nucleon is about 0.8 MeV. The total energy given off is therefore
is broken into two smaller nuclei, the binding energy difference per nucleon is about 0.8 MeV. The total energy given off is therefore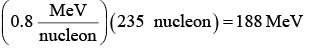
This process is called as nuclear fission.
(ii) If we can somehow join two light nuclei together to give a single nucleus of medium size also means more binding energy per nucleon in the new nucleus. For instance, if two 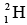 deuterium nuclei combine to form a
deuterium nuclei combine to form a 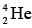 helium nucleus, over 23 MeV is released. Such a process, called nuclear fusion, is also very effective way to obtain energy. In fact, nuclear fusion is the main energy source of the sun and other stars.
helium nucleus, over 23 MeV is released. Such a process, called nuclear fusion, is also very effective way to obtain energy. In fact, nuclear fusion is the main energy source of the sun and other stars.
Example 1: The measured mass of deuteron atom 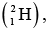 Hydrogen atom
Hydrogen atom 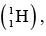 proton and neutron is 2.01649 u ,1.00782 u , 1.00727 u and 1.00866 u. Find the binding energy of the deuteron nucleus (unit MeV / nucleon).
proton and neutron is 2.01649 u ,1.00782 u , 1.00727 u and 1.00866 u. Find the binding energy of the deuteron nucleus (unit MeV / nucleon).
Here A = 2, Z = 1, N = 1
B.E. = ZMH + NMN -
= [1x 1.00782 + 1x 1.00866 - 2.01649] x 931.5 MeV
= [0.00238] x 931.5 MeV = 2.224 MeV
Example: The binding energy of the neon isotope 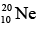 is 160.647 MeV. Find its atomic mass.
is 160.647 MeV. Find its atomic mass.
Here A = 10, Z = 10, N = 10
Example:
(a) Find the energy needed to remove a neutron from the nucleus of the calcium isotop 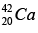
(b) Find the energy needed to remove a proton from this nucleus.
(c) Why are these energies different?
Given: atomic masses of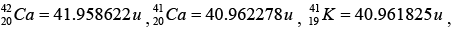 and mass
and mass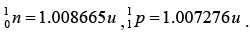
(a)
Total mass of the
Mass defect Δm = 41.970943 - 41.958622 = 0.012321u
So, B.E. of missing neutron Δm x 931.5 = 11.48MeV
(b)
Total mass of the
Mass defect Δm = 41.919101 - 41.958622 = 0.010479u
So, B.E. of missing protron = Δm x 931.5 = 10.27MeV
(c) The neutron was acted upon only by attractive nuclear forces whereas the proton was also acted upon by repulsive electric forces that decrease its binding energy.
Salient Features of Nuclear Forces
Nucleus is bounded by nuclear forces. The basic properties of nuclear forces are
(i) It is a short range attractive force.
(ii) It is in general non-central force.
(iii) They have property of saturation i.e. each nucleon interacts only with its nearest neighbors and not with all the constituents in the nucleus. This is apparent from the fact that average B.E. per nuclear remains approximately constant i.e.
BE . ∝ A
When all interactions are possible then A(A-1)/2 interaction may take place;
B.E. ∝ A2 is not valid.
(iv) They are charge independent i.e. n - n, p - p and n - p have same nuclear force.
(v) They are spin dependent force as shown by deuteron.
(vi) They are exchange forces proposed by Yukawa(Meson Theory).
Despite the strength of the forces that holds nucleons together to form an atomic nucleus, many nuclides are unstable and spontaneously change into other nuclides by radioactive decay.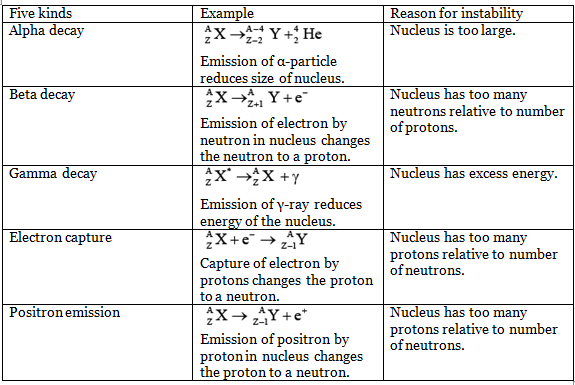
|
37 videos|17 docs|19 tests
|
FAQs on Basic Nuclear Properties - Modern Physics
| 1. What is the size and density of a nucleus? |  |
| 2. What is the spin and magnetic moment of a nucleus? |  |
| 3. What is the angular momentum of a nucleus? |  |
| 4. What are stable nuclei? |  |
| 5. What is binding energy in nuclear physics? |  |