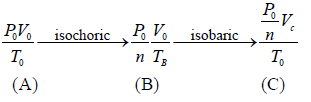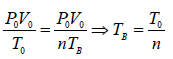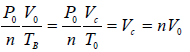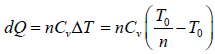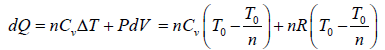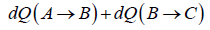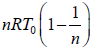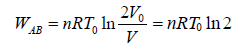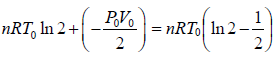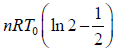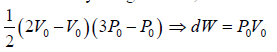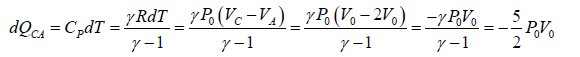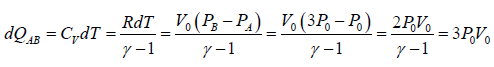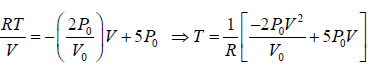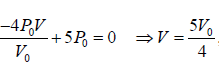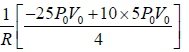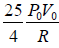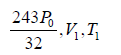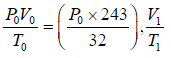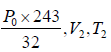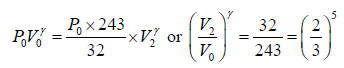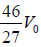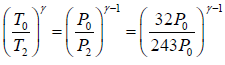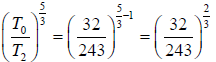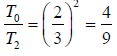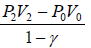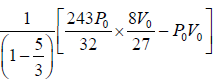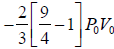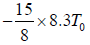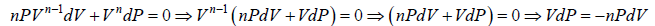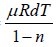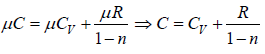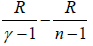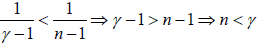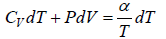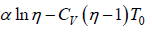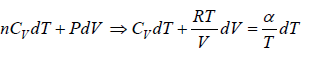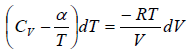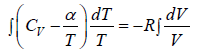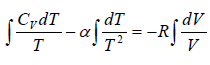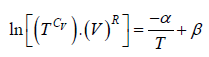Zeroth & First Law of Thermodynamics | Kinetic Theory & Thermodynamics - Physics PDF Download
Zeroth Law of Thermodynamics
If two systems say, A and B are separately in thermal equilibrium with a third system say C, then they must also be in thermal equilibrium with one another.
It is analogous to the transitive property in math (if A = C and B = C , then A = B ).
Another way of stating the zeroth law is that every object has a certain temperature, and
when two objects are in thermal equilibrium, their temperatures are equal.
First Law of Thermodynamics
If ∂Q amount of heat is added to the system and if system will do ∂W amount of work, then change in internal energy is dU , which is given by dU = ∂Q - ∂W . First Law of thermodynamics is law of conservation of energy in which Heat added to system can be divided into mechanical work done by system and increase in internal energy of system .
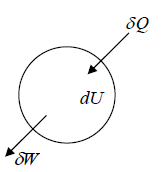
(obviously, heat exchange and work done is dependent on path and internal energy is state function.)
One can also represent first law as ∂Q = dU + ∂W
Different Types of Thermodynamical Process and use of First Law of Thermodynamics.
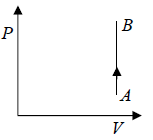
Isochoric Process
When volume remains constant during the process the process is said to be isochoric process.
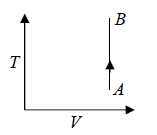
One can also show Isochoric process in T - V Diagram
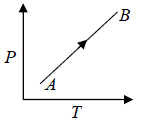
For Ideal Gas Isochoric Process can be shown in P -T diagram which is actually straight line appear to pass through origin which is shown in figure
Work done in isochoric process
dW = PdV
Since, dV = 0 ⇒ dW = 0
In an isochoric process work done during the process is zero.
From first law of thermodynamics,
dU = ∂Q - ∂W
Since, ∂W = 0 ⇒ dU = ∂Q = nCvdT
In isochoric process, change in internal energy is equal to heat exchange, So in isochoric process the Heat exchanged is perfect (Exact) differential, So in isochoric process Heat exchanged is Path independent.
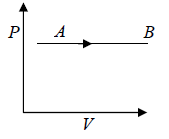
Isobaric Process
When pressure of the system remain constant during the process, the process is set to be isobaric process.
The Isobaric process shown in P - V diagram
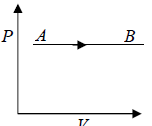
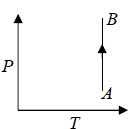
One can also show Isobaric process in T - P Diagram
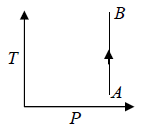
For Ideal Gas Isochoric Process can be shown in P -T diagram which is actually straight line appear to pass through origin which is shown in figure
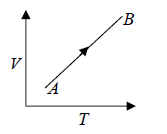
Work done during the process is given by
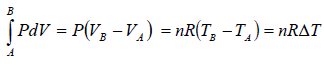
where, ΔT is change in temperature during the process.
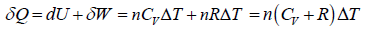
∂Q = nCvΔT [since, Cv + R = CP for the ideal gas]
Heat exchange during the process for n mole of gas is equal to nCvΔT , where CP is specific heat capacity at constant pressure.
∂Q = nCvΔT where CP = 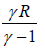
∂Q = 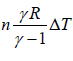 ⇒
⇒ 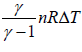 ⇒∂Q =
⇒∂Q = 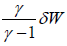
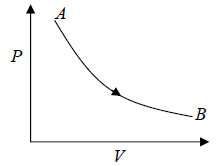
Isothermal Process
When temperature remains constant during the process, then the process is said to be isothermal process.
Isothermal process in P - V diagram shown in figure

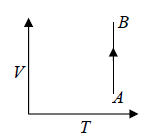
Isothermal process in T - V Diagram shown in figure
Isothermal process in V - T Diagram shown in figure
In isothermal process, change in internal energy is zero because ΔT = 0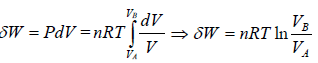
during the isothermal process, heat exchange
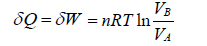
Adiabatic Process
When there is not any heat exchange during the process, then process is said to be adiabatic process.
Adiabatic process is defined by,
PVγ = constant, where γ = Cp/Cv
For Ideal gas, PV = RT for one mole of gas.
Slope of Adiabatic process in P - V diagram
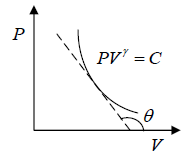
PVγ = C ⇒ 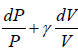 = 0
= 0
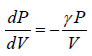 < 0 where γ, P,V > 0
< 0 where γ, P,V > 0
Slope of Adiabatic process in T-V diagram
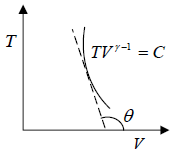
So, TVγ-1 = constant
TVγ-1 = C
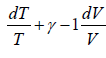 = 0
= 0
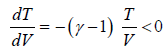 , where γ >1, T > 0, V > 0
, where γ >1, T > 0, V > 0
Slope of adiabatic process in T-P diagram
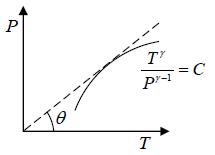
P1-γTγ = constant, where γ = Cp/Cv
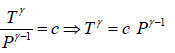
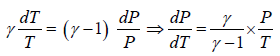
Slope of adiabatic process on P -T diagram is

Work done during adiabatic process
∂W = PdV
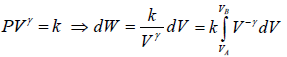
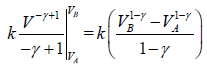
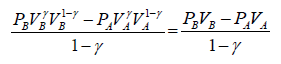
For n mole Ideal gas
W = 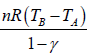
For Adiabatic process ∂Q = dU + ∂W
Since, ∂Q = 0 ⇒dU = - ∂W
For adiabatic process ⇒ ∂W = -dU so work done is perfect (exact) differential .in adiabatic process is work done is path independent.
So, in adiabatic process change in internal energy is equal to negative of work done i.e.
dU = 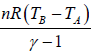
Example 1: n mole of certain ideal gas at temperature T0 were cooled isochorically, so that the gas pressure reduced n times. Then as a result at the isobaric process the gas expanded till its temperature get back to initial value. Find the total amount of heat absorbed by the gas in the process.
Let at state A , the pressure, volume, temperature is P0,V0,T0,n is number of mole.
According to question,
In process A to B
In process B to C
Total heat absorbed by gas,
=
=
Example 2: A sample of an ideal gas is taken through the cyclic process “abcd ” as shown in figure. It absorbed 50Jof heat during part ab, no heat during bc and reject 70J of heat during ca, 40J of work is done on the gas during part bc .
(a) Find the internal energy of the gas at b and c if it is 1500 J at a .
(b) Calculate the work done by the gas during the part ca.
(a) During process a→b , V = constant
ΔQ = dU = Ub -Ua = 50 J
If at ‘ a ’, Ua = 1500J, so at ‘ b’, Ub= 1550J
Work done during process, b→c = -40 J
ΔU = Q - W = 0 - (-40) = 40J
Uc - Ub = 40
Uc = 1550 + 40 = 1590
Ub = 1550J ⇒Uc = 1590(no heat absorbed at bc)
(b) During process, c→a
ΔU = 1500 - 1590 = - 90J
ΔQ = -70J
ΔW = ΔQ - ΔU = -70 + 90 = 20J
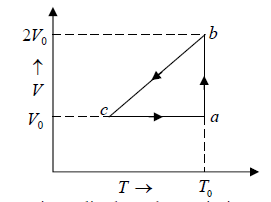
Example 3: A sample of an Ideal gas has pressure P0, volume V0 and temperature T0 . It is isothermally expanded to twice its original volume. It is then compressed at constant pressure to have the original volume V0 . Finally, the gas is heated at constant volume to get the original temperature.
(a) Show the process in VT diagram.
(b) Calculate the heat absorbed in the process.
(a)
(b) Hence the process is cyclic then change in internal energy in cycle is zero. So heat supplied in the cycle is equal to work done.
Work done during isothermal process, A→B is,
Since, process b→c is isobaric, so work done during the process is PdV .
PaVa = PbVb
P0V0 = Pb2V0 ⇒ Pb = P0/2
Wb→c = P0/2(V0 - 2V0) = - P0V0/2
Since, Wc→a is isochoric, so Wc→a = 0
Thus, total work done is given by,
W =
Since, the process is cyclic i.e.,
dU = 0 ⇒ dQ = dW =
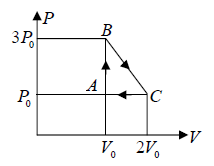
Example 4: One mole of an ideal monatomic 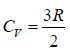 with gas is taken round the cyclic process ABCA as shown in figure. Calculate
with gas is taken round the cyclic process ABCA as shown in figure. Calculate
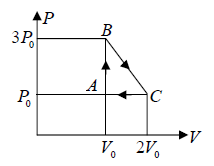
(a) The work done by the gas.
(b) The heat rejected by the gas in the path CA and the heat
absorbed by the gas in the path AB ;
(c) The net heat absorbed by the gas in the path BC ;
(d) The maximum temperature attained by the gas during the cycle.
Number of mole of ideal monatomic gas = n =1 mole
For monatomic gas:
, So γ = Cp/Cv = 5/3
ABCA is cyclic process, so change in internal energy is zero during the cycle
A→B : An isochoric process at constant volume V0
(a) Work done by the gas: dW , which is equivalent to Area of triangle ABC
dW =
(b) The process C → A: is an isobaric compression at constant pressure P0
Heat rejected alone
So magnitude of heat rejected in process C to A is 5/2P0V0 negative sign justify the heat rejection during the process
The process A→B is an isochoric process at constant volume V0
Heat rejected alone
So magnitude of heat rejected in process A to B is 3P0V0 positive sign justify the heat
absorbed during the process
(c) In a cyclic process, dU = 0 , Net heat absorbed along BC :
dQ = dW =
= dW ⇒
= P0V0
the magnitude of heat exchanged is P0V0/2 negative sign justify the rejection of heat in the process.
(d) The maximum temperature of gas: Tmax :
BC is a straight line. The maximum temperature of the gas Tmax will lie on BC at some
point.
For a straight line y = mx + C
For BC, slope m =
, P = mV + C ⇒ P =
will satisfy any of given coordinate on line, so 3P0 =
⇒ C = 5P0
From equation of state P =
and n =1
For T to be maximum, dT/dV = 0, d2T/dV2 = -ve.
Hence,
Tmax =
⇒ Tmax =
⇒ Tmax =
Example 5: The rectangular box shown in figure has a partition which can slide without friction along the length of the box. Initially each of the two chambers of the box has one mole of a mono-atomic ideal gas (γ = 5/3) at a pressure P0 volume V0 and temperature T0. The chamber on the left is slowly heated by an electric heater. The walls of the box and the partition are thermally insulted. Heat loss through the lead wires of the heater is negligible. The gas in the left chamber expands pushing the partition until the final pressure in both chambers becomes 243P0/32 .
Determine:
(i) the final temperature of the gas in each chamber and
(ii) The work done by the gas in the right chamber.
(i) For left chamber, the process of heating is slow. Initially, the parameter is P0,V0,T0 finally, the parameters are
or, T1 =
(i)
Adiabatic compression occurs in the right chamber. The initial quantities are P0,V0, γ,T0 The final qualities are
or V2 =
(ii)
But V1 + V2 = 2V0 given V1 +
= 2V0
or V1 =
From (i) and (iii),
T1 =
(iii)
or T1 =
(iv)
Again for the right chamber, use P - T equation for an adiabatic operation.
Tγ ∞ Pγ-1
so,
⇒
or
or
or
or T2 = 9/4 T0 or T2 = 2.25T0
(ii) Work done by the gas in right chamber.
Adiabatic work done =
W =
or W =
or W =
=
or W = -15.58T0 Joule
Work done is negative as the volume in the right chamber decreases.
Example 6: Find the molar heat capacity of an ideal gas in a polytrophic process
pVn = const if the adiabatic exponent of the gas is equal to γ At what values of the
polytrophic constant n will the heat capacity of the gas be negative?
We know from first law of thermodynamics, dQ = dU + dW
Let us assume C is specific heat related for process so, dQ = μCdT
Cv is specific Heat capacity at constant volume internal energy is path independent so dQ = μCdT which will same for every process for Ideal gas
So first law of thermodynamics can be written as μCdT = μCvdT + PdV
The equation of process is given by PVn = constant
Differentiate equation
Also, the equation of state for Ideal gas is given by PV = μRT
PdV + VdP = μRdT
PdV + nPdV = μRdT ⇒ PdV =
⇒
C =
If C < 0 then
Example: An ideal gas has an adiabatic exponent γ . In some process its molar heat capacity varies as C =α /T , where α is a constant.
(a) What is the work performed by one mole of gas during its heating from the temperature T0 to the temperature η times higher.
(b) Find the that the equation of the process in the variables T,V .
(c) Prove that the equation of the process in the variables P,V is given by PVγ .exp 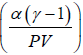 = constant
= constant
(a) C = α/T
The heat exchanged during process is
where n =1 is number of mole
From first law of thermodynamics dQ = dU + dW ⇒ dQ = nCvdT + PdV
dW =
⇒ W =
⇒ W =
(b) From equation of state P = RT/V
From first law of thermodynamics, dQ =
where β is constant
= constant
(c)
= constant
= constant
= constant
= constant
|
6 videos|20 docs|32 tests
|
FAQs on Zeroth & First Law of Thermodynamics - Kinetic Theory & Thermodynamics - Physics
| 1. What are the different types of thermodynamical processes? |  |
| 2. What is the use of the First Law of Thermodynamics? |  |
| 3. What is the Zeroth Law of Thermodynamics? |  |
| 4. What are some frequently asked questions about the Zeroth Law of Thermodynamics? |  |
| 5. How is the First Law of Thermodynamics related to energy conservation? |  |