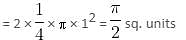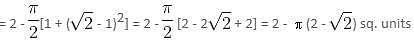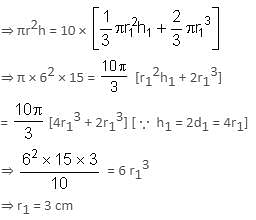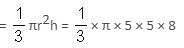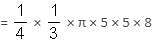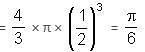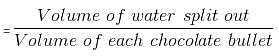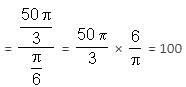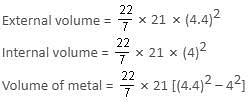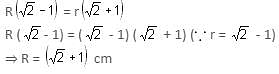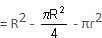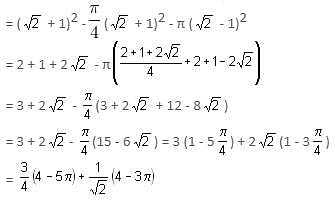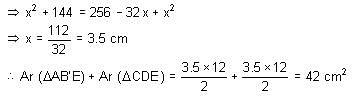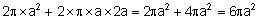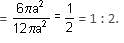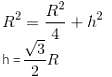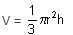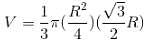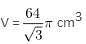This EduRev document offers 10 Multiple Choice Questions (MCQs) from the topic Mensuration (Level - 3). These questions are of Level - 3 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 3: Mensuration
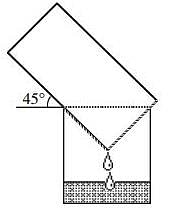
Try yourself:A cylindrical pail containing water drains into a cylindrical tub, 40 cm across and 50 cm deep, while resting at an angle of 45° to the horizontal, as shown in the figure. How deep is the water in the tub when its level reaches the pail?

Explanation
We label the points A, B and C as shown in the diagram. From symmetry, we note that ΔBAC is an isosceles right-angled triangle.
From A, we draw a line perpendicular to BC meeting the line at point D.

This construction allows us to conclude that ΔABD is also a right-angled isosceles triangle and specifically that BD = DA.
Since BD = DA; BD = DC = 20; we find DA = 20 cm.
This makes the depth of the water equal to (50 - 20) cm or 30 cm.
Report a problem
Question for Practice Questions Level 3: Mensuration
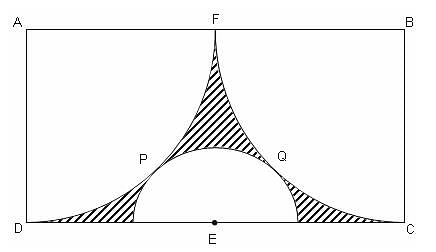
Try yourself:ABCD is a rectangle with AD = 1 unit. DPF and CQF are two equal arcs drawn with A and B as centres respectively. E is the midpoint of CD. Another arc with E as centre touches the two arcs DPF and CQF at P and Q, respectively. What is the area of the shaded portion?

Explanation
Report a problem
Question for Practice Questions Level 3: Mensuration
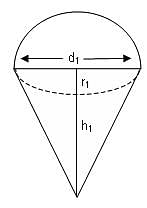
Try yourself:A cylindrical container, whose diameter is 12 cm and height is 15 cm, is filled with ice-cream. The whole ice-cream is distributed among 10 children in equal cones with hemispherical tops. If the height of the conical portion is twice the diameter of its base, find the diameter of the ice-cream cone.
Explanation
Volume of the cylindrical container = 10 × volume of each cone


Diameter of each ice-cream cone = 6 cm
Report a problem
Question for Practice Questions Level 3: Mensuration
Try yourself:A vessel is in the form of an inverted cone, open at the top. Its depth is 8 cm and the diameter is 10 cm. It is filled with water up to the brim. When spherical chocolate bullets of radius 0.5 cm are dropped into the vessel, 1/4 of the water flows out. Find the number of bullets dropped in the vessel.
Explanation
Report a problem
Question for Practice Questions Level 3: Mensuration
Try yourself:What will be the mass of a 21 cm long metallic hollow cylinder with internal diameter 8 cm and thickness of metal being 4 mm, if 1 cm3 of the metal weighs 8 gm?
Explanation
Length of cylinder = 21 cm
Internal radius = 4 cm
External radius = 4.4 cm

= 66 (19.36 – 16) = 221.76 cm3
Hence, Mass of cylinder = Volume of metal × 8 = 221.76 × 8 = 1774 gm
Report a problem
Question for Practice Questions Level 3: Mensuration
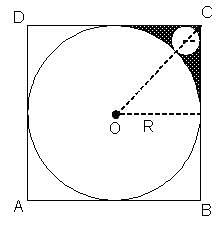
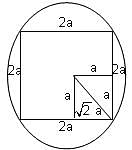
Try yourself:In the above figure, if the radius of the smaller circle is (√2 - 1) cm, then the area of the shaded region will be
Explanation
Report a problem
Question for Practice Questions Level 3: Mensuration
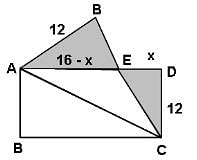
Try yourself:A rectangular piece of paper 16 cm long and 12 cm wide is folded along one of its diagonals. It results in some area of the paper doubling up over the portion of the paper already lying but some portion failing to double up, as it is not a square piece. Determine the area of the paper which has not doubled up.
Explanation
Refer to the figure. △AB'E and △CDE form the area which has not doubled up.

Both triangles are similar since ∠AB'E = ∠CDE = 90°.
∠AEB' = ∠DEC (vertically opposite angles)
Also, since CD and AB', the corresponding sides of the triangles are equal (= 12 cm), the triangles are congruent.
Let and DE = B'E = x and CE = AE = 16 - x
Then, x2 + 122 = (16 - x)2

Report a problem
Question for Practice Questions Level 3: Mensuration
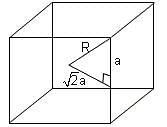
Try yourself:A cube of maximum possible volume is kept inside a sphere. A right circular cylinder of maximum possible volume is kept inside the cube. What is the ratio of the total outer surface area of the cylinder to that of the sphere?
Explanation
Report a problem
Question for Practice Questions Level 3: Mensuration
Try yourself:Ram cuts a semicircle of radius 8 cm from a piece of paper and folds it in such a way that it forms a right circular cone. What is the volume of this cone?
Explanation
Report a problem
Question for Practice Questions Level 3: Mensuration
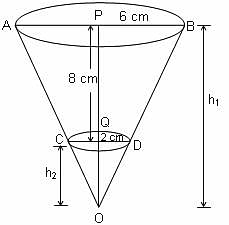
Try yourself:The height of a frustum made from a cone is 8 cm. If the base radii of this frustum are 6 cm and 2 cm, then find the height of the original cone.
Explanation
Height of the frustum (h) = 8 cm

Radii of the base of the frustum are:
r1 = 6 cm and r2 = 2 cm
Height of the original cone = h1 = 8 + h2 … (i)
∵ ΔOPB and ΔOQD are similar
[∵QOD = POB (same angle) and OQD = OPB = 90°]
So, the ratio of their corresponding sides is equal.
Thus, h1/h2 = 6/2 = 3/1 or h1 = 3h2
Putting the value h1 = 3h2 into equation (i), we get
⇒ 3h2 = 8 + h2 or 2h2 = 8 ⇒ h2 = 4 cm
Again putting h2 = 4 into (i), we get
⇒ h1 = 8 + 4 = 12 cm
So, height of the original cone = 12 cm
Report a problem