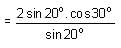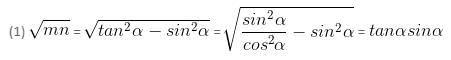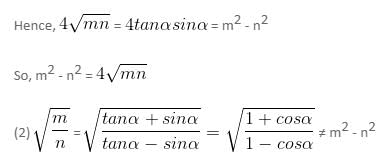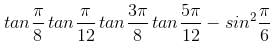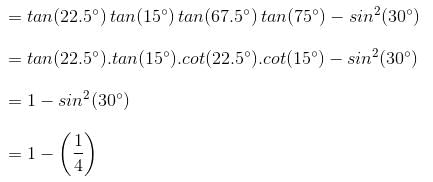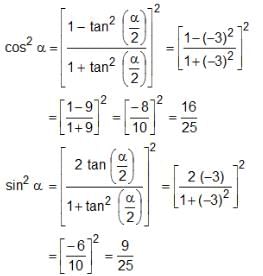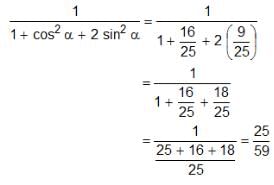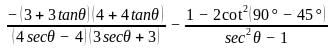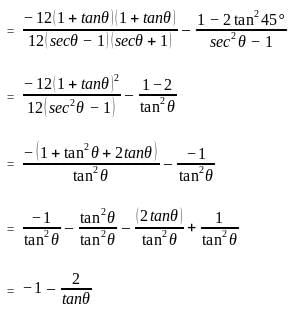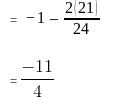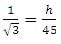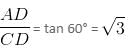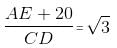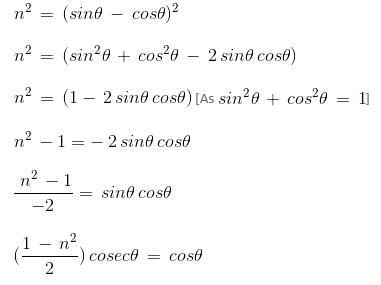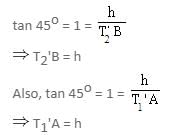This EduRev document offers 15 Multiple Choice Questions (MCQs) from the topic Trigonometry (Level - 2). These questions are of Level - 2 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:If cosθ = 5/21 sinθ, then the value of 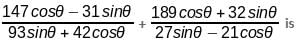
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
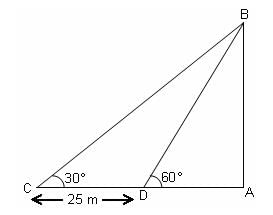
Try yourself:The length of the shadow of a vertical pole on level ground increases by 25 metres when the altitude of the sun changes from 60° to 30°. Calculate the height of the pole.
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
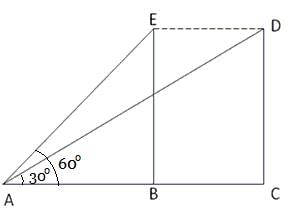
Try yourself:The angles of elevation of a plane flying at a constant altitude of 10000 ft are found to be 60° and 30° at an interval of 1 minute. What is the speed of the plane?
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:If 4 cos2 A + 2 sin2 A = 3, then what is the value of A, given that A lies in the first quadrant?
Explanation
4 cos2 A + 2 (1 - cos2 A) = 3
4 cos2 A + 2 - 2cos2A = 3
2 + 2 cos2A = 3
2(1 + cos2 A) = 3
1 + cos2 A = 3/2
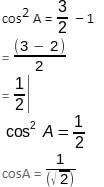
A = 45°
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
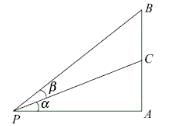
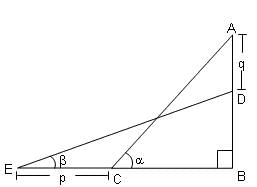
Try yourself:AB is a vertical pole. The end A is on the level ground and C is the middle point of AB. P is a point on the level ground. The portion BC subtends an angle β at P. If AP = nAB, then tan equals
Explanation
AC = AP tan α
⇒ (1/2) AB = n AB tan α
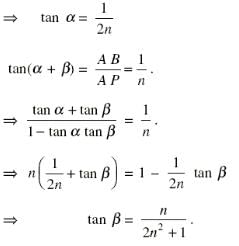
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:A ladder rests against a vertical wall at an inclination α to the horizontal. Its foot is pulled away from the wall through a distance p, such that its upper end slides a distance q down the wall and then the ladder makes an angle β to the horizontal.
What is the value of P/q?
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself: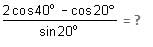
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:If tan α + sin α = m and tan α - sin α = n, then m2 - n2 =
Explanation
m2 - n2 = (m + n)(m - n) = 2 tan α × 2 sin α = 4 tan α sin α
Now, going by options:
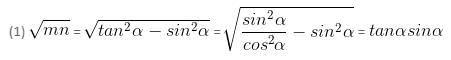
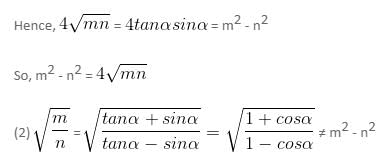
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:Find the value of 
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself: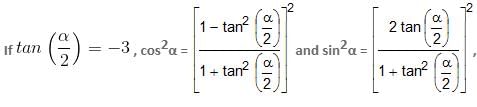
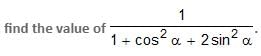
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself: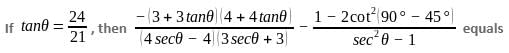
Explanation
Report a problem
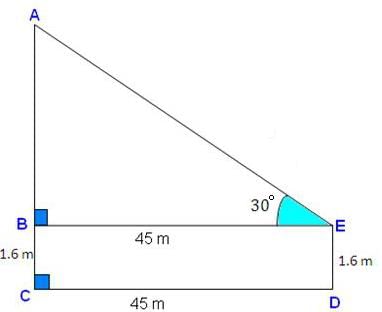
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:A 1.6 m tall observer is 45 meters away from a tower. If the angle of elevation from his eye to the top of the tower is 30°, then the height of the tower in meters is
(Take √3 = 1.732)
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
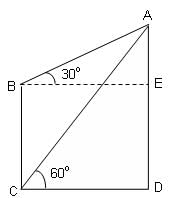
Try yourself:The angle of elevation of the top of a tower from a point on the ground at some distance from its base is 60°. The angle of elevation of the top of the tower from a point 20 m above the same point on the ground is 30°. What is the height of the tower?
Explanation
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:What is the value of cosθ if n = sinθ - cosθ ?
Explanation
n = sinθ - cosθ
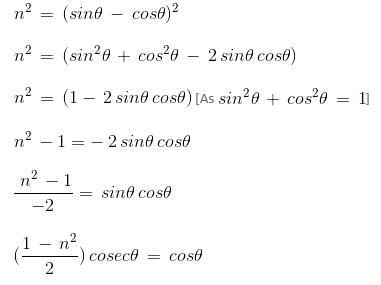
Report a problem
Question for Practice Questions Level 2: Trigonometry - 2
Try yourself:Two vertical poles of equal height are 120 m apart. On the line joining their bases, A and B are two points. Angle of elevation of the top of one pole from A is 45o and that of the other pole from B is also 45o. If AB = 30 m, then the height of each pole is
Explanation
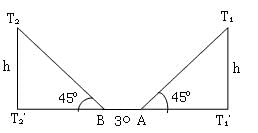
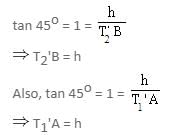
Hence, 120 = h + 30 + h
⇒ h = 45 m
∴ Height of the vertical poles = 45 m
Report a problem
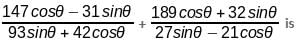
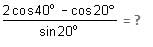

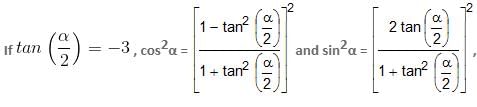
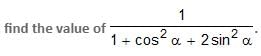
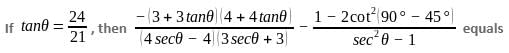

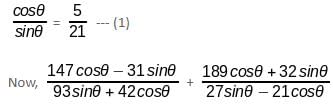
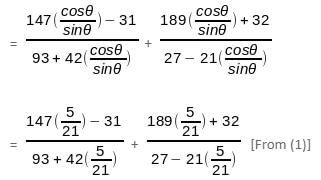
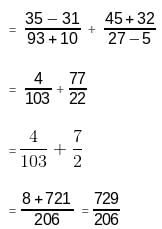
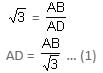

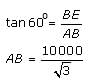
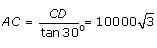
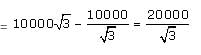
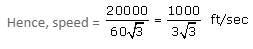
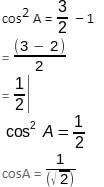

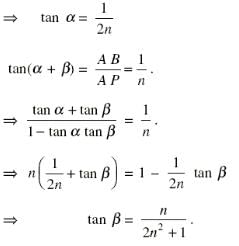
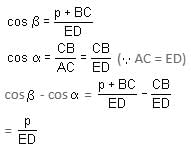
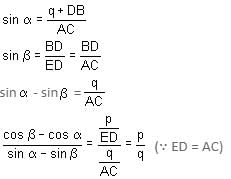
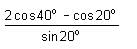
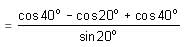
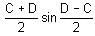
 ]
]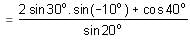
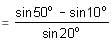
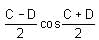 ]
]