Smith Chart | Electromagnetics - Electronics and Communication Engineering (ECE) PDF Download

Introduction

Smith Chart is the invention of two researcher’s Philip Smith and Tosaku who hold individual credits in this chart discovery. This chart was developed in the period 1930s at Bell Telephone’s Research laboratory. All the engineering complications that were raised were resolved by this Smith Chart until the period digital computers came into prominence. When the trademark for Smith chart was expired, MS. Smith sold the trademarks to IEEE MTT Society in the year 2015. Dr. Richard Synder also took part in these trademark discussions along with Ms. Smith. Because of this, it was observed that smith charts have MTT-S backgrounds.

What is a Smith Chart?

• Smith chart is considered as a graphical measuring tool which is constructed mainly for electrical engineers to solve problems related to RF transmission lines and matching devices. At the same time, the device is also used to display various factors like admittance, continual gain outlines, impedance, stability, noise figure, and also shows a detailed analysis of mechanical vibrations.
• The chart will be more common in use internal to the regions that have a radius range of 1 or < 1 such as in the cases of stability performance and oscillator design. As it was known that usage of the smith chart for resolving complicated mathematical problems impose matching issues, but it is still an effective method in explaining how RF frequencies perform at multiple frequency levels. This because most RF circuit software’s consists of smith charts for displaying results.

Types of Smith Charts

Smith charts are plotted in two dimensions on the intricate reflection coefficient plane and the chart is generally standardized in impedance or admittance or even both through multiple colors in order to differentiate between those parameters and depending on this scaling, these are primarily categorized as three types. Those are of:

1. Impedance Smith Chart
These are generally termed as the usual type of smith charts as they correspond to impedance and functions in an excellent way even with huge loads constructed of many series components where these are the crucial components in the impedance matching and for other RF corresponding operations. This impedance of these are the main type where other types are considered as its derivatives. The picture representing impedance smith chart is shown below: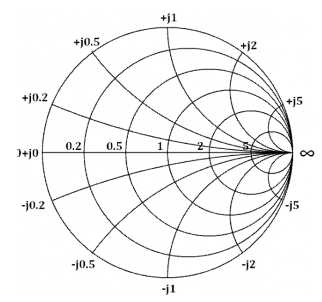
Impedance Smith Chart2.Admittance Smith Chart
The impedance chart is crucially implemented when working on series loads, all the functionality here is to summate the whole increased impedances. Whereas the tricky part here is mathematical calculation when dealing with parallel elements where the elements are of inductors, transmission lines, and capacitors.
So, to minimize the complications involved in impedance charts, these admittance charts were developed. As we all are aware that admittance has revere relation to impedance, this admittance chart works well for complicated parallel scenarios. To do this, one has to clearly analyze the antenna admittance instead of impedance and simply summate all those. The equation which represents the relation between these two factors (impedance and admittance) is:

Here ‘Y’ corresponds to load admittance and ‘Z’ corresponds to impedance.
‘C’ is the Conductance and ‘S’ is the Susceptance.
The equation itself shows that impedance and admittance hold the reverse relationship.
The picture is shown below: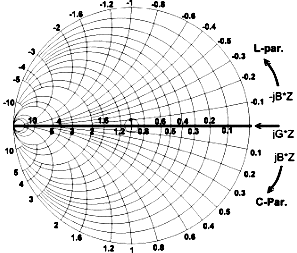
Admittance Smith Chart

3. Immittance Smith Chart
We all have discussed that the impedance smith chart is more useful to deal with series components and the admittance smith chart works well for parallel components. But, in the situation when both the components are implemented, the situation becomes complicated and tricky. The answer to this is the Immittance smith chart. This is an effective solution where it plots impedance and admittance chart one above the other. Below is the picture.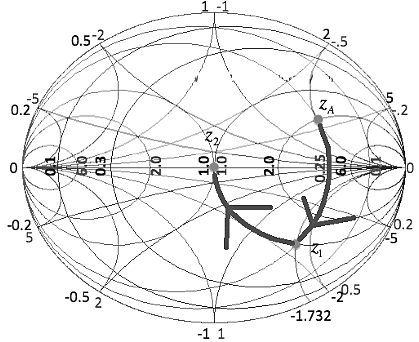
Immittance

This chart is helpful for merging the capability of both the impedance and admittance charts. In the impedance matching operations, it assists in finding out how series or parallel elements influence impedance with minimal effort.

Basics

As it was already stated that Smith chart exhibits intricate reflection coefficients in the polar form for specific load impedance. And we all know that impedance is termed as the sum of reactance and resistance and in the same way, the reflection coefficient is also a complex numeral and so represented as load impedance ‘ZL’ and the reference impedance ‘Z0’.

The mathematical representation of the above statement is that

= (Z_L – Z₀)/ (Z_L + Z₀) = (Z_L – 1)/(Z_L + 1)
Here, ‘Z0’corresponds to transmitters impedance, and ‘ZL’ corresponds to load impedance. It is mainly a graphical representation of exhibiting antenna’s impedance corresponding to frequency might be single or few range of points. This theory corresponds to the basics of smith chart.

Components

• The pictorial representation of this chart seems to be somewhat typical as there are many lines. But when we understand the concept of each line, then the chart is very easy to implement.
• For an impedance smith chart, there are two circles that define the information and design of the smith chart which are constant R circles and constant X circles.

Constant R Circles

Here, the initial set of lines are termed as constant resistance lines where all are in tangential position each other at the right-hand of the horizontal radius. These circles appear when the impedance’s resistance is kept constant and the X value is varied. With this, the entire points on the constant R circle will have similar resistance values.

All these points are highlighted on the horizontal line at the location where their intersection this. This is generally represented as circles’ diameter. The picture is shown below:
Constant Resistance Lines From CirclesFor instance: when the normalized impedance is represented as ZL = R + iX and when R equals to ‘0’ and X equals to any real integral, then
Z_L = 1 + i₀, Z_L = 1 + i₃ and Z_L = 1 + i₄

Constant X Circles

Here, the plot consists of more arcs than that of circles, and all these arcs are in a tangential position to each other at the right-hand edge of the horizontal radius. These circles appear when the impedance’s reactance is kept constant and the X value is varied.

The lines those are in the upper half section signifies a positive value of reactance’s whereas the lines in the lower half section signify the negative value of reactances.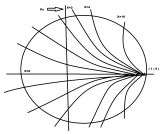
Constant-X-Circles In Smith ChartFor instance, when a curve is represented as ZL =  R + iY and when Y = 1 and maintained at constant and R signifies to real integral which ranges between 0 to infinity, then the constant X circles plot is shown as below. These are the components of the smith chart.

Applications

In any domain of RF engineering, the smith chart has various applications. Few of the foremost applications of the smith chart are:

• It is used in the transmission line is used to calculate impedance provided at any load
• The chart is even employed to calculate admittance values provided at any load
• Used in the measurement of the length of the short-circuited section of the Tx.line in order to offer the required amount of inductive reactance of capacitance
• Used for the purposes of impedance matching
• Employed to know the value of VSWR amongst others.

Application of Smith Chart in Impedance Matching

• To use a smith chart and find out outcomes with it need proper knowledge of alternating circuit and the concept of transmission line where these stand as preconditions for RF engineering. To know more about it, let us consider an example of how to use a smith chart for impedance matching in the case of transmission lines and antennas.
• Here the application of the chart is to find out the components (capacitor or inductor) value which makes sure that the lines are properly matched thus states that the reflection coefficient is ‘0’.
• For instance, when the impedance value is considered as Z = 0.5 – 0.6j. Here, initially, we need to know the resistance circle that has a value 0.5 on the chart. As the impedance value is negative, which implies that we mean a capacitive impedance, we need to move it in the anti-clockwise direction across the 0.5 valued resistance circle to know the point where it touches the negative 0.6 reactance arc. This provides a thought of the component’s value that helps in matching the line to the load.
• Standardized scaling let the smith chart to be implemented in the applications which have any distinctive or system impedance that represents the chart’s focal point. In the case of impedance charts, the generally used value of normalization impedance is 50 ohms where is used for simply tracing the impedance.
• When the result is found through this, we can directly transform in between normalized impedance and the relevant unnormalized value by multiplying with the admittance value. With this reflection, coefficients are easily known. Additionally, these are also employed to solve the complication those arise with a change in frequency values for impedance and admittances.
• It is easy to solve the problems which have one frequency value at a time, whereas in the case of bandwidth applications, it seems to be complicated which works with multiple frequencies. This is solved by smith chart and the outcome is shown as Locus with all the frequency ranges are close to each other. These locus points corresponds that
  • How the inductive or capacitive load varies through the determined frequency range
  • How complicated matching is probable to be at multiple frequency levels
  • How properly a component is matched.

So, this is how smith charts are used for impedance matching.