Simultaneous Equations Examples | Mathematics for Grade 12 PDF Download
Introduction
Simultaneous equations are the system of two or more independent equations which satisfies a common solution. Simultaneous equations could have,
- Linear Equation
- Quadratic Equation
- Polynomial Equations
There are several methods of solving simultaneous equations, and they are:
- Graphical Method – By plotting the given equations on a graph, if they intersect at a unique point, the equations have a unique solution. If the equations coincide with each other, the equations have infinitely many solutions. If the lines are parallel, the equations have no solutions.
- Elimination Method – Eliminate one of the variables, and find the solution for the other.
- Substitution Method – Write one variable in terms of the other and substitute its value in the equation to reduce the equation into one variable form.
- Cross-Multiplication Method – Cross multiply the coefficients to get the solution of the pair of linear equations.
If we are given a pair of linear equations, the existence of the solution is determined as follows: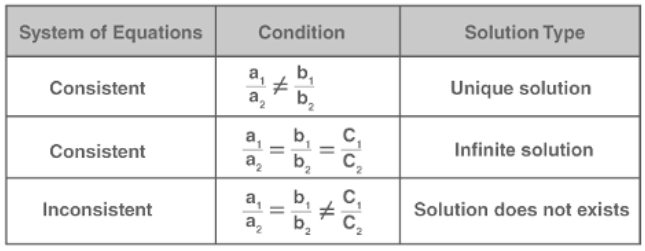
Simultaneous Equations Examples
Example 1: Solve the following:
2x + 3y = –2
5x + 4y + 2 = 0
Given,
2x + 3y = –2 ….(i)
5x + 4y = –2 ….(ii)
Multiply (i) by 5 and (ii) 2 on both sides, subtracting (ii) from (i), we get
10x + 15y = –10
10x + 8y = –4
(–)_ (–)___(+)___
7y = –6
⇒ y = –6/7
Substituting the value of y in (i), we get
x = 2/7
∴ the solution of the given simultaneous equation is (2/7, –6/7)
Example 2: Solve the following equations:
2a – b = 4 and 2b – 3a = 2
Given,
2a – b = 4 …..(i)
2b – 3a = 2
⇒ –3a + 2b = 2 ….(ii)
From (i),
a = ½ (b + 4)
Putting this value in (ii)
–3/2(b + 4) + 2b = 2
⇒ –3b – 12 + 4b = 4
⇒ b = 16
Thus, a = ½(16 + 4) = 10
∴ the solution of the given simultaneous equation is a = 10 and b = 16.
Example 3: Solve the following simultaneous equations:
7m – 9n = 3 and 2m – 3n = 1
Given,
7m – 9n = 3 ….(i)
2m – 3n = 1 ….(ii)
Multiplying equation (ii) by 3 on both sides, then subtracting from (i), we get,
(7m – 6m) + ( –9n + 9n) = 3 – 3
⇒ m = 0
Consequently, n = –⅓
∴ the solution of the given simultaneous equation is m = 0 and n = –⅓.
Example 4: Solve the following simultaneous equations:
a2 + 2b = 9 and b – a = 3
Given,
a2 + 2b = 9 …(i)
b – a = 3 …(ii)
From (ii) b = 3 + a
Substituting the value of b in (i), we get
a2 + 2(3 + a) = 9
⇒ a2 + 2a + 6 = 9
⇒ a2 + 2a + – 3 = 0
⇒ (a + 3)(a – 1) = 0
⇒ a = 1 and –3
When a = 1, b = 4, and when a = –3, b = 0
∴ the solution of the given simultaneous equation is a = 1, b = 4 and a = –3, b = 0.
Also, learn about solving simultaneous equations by Cramer’s rule.
Example 5: Solve the following simultaneous equations:
a2 – b = 14 and 2b – 4 = 12a
Given,
a2 – b = 14 ….(i)
2b – 4 = 12a
⇒ 12a – 2b = –4
⇒ 6a – b = –2
⇒ b = 6a + 2
Substituting the value of b in (i), we get
a2 – 6a – 2 = 14
⇒ a2 – 6a – 16 = 0
⇒ (a – 8)(a + 2) = 0
⇒ a = 8 and –2
When a = 8, b = 50 and when a = –2, b = 10
∴ the solution of the given simultaneous equation is a = 8, b = 50 and a = –5, b = 11.
Example 6: Solve the following simultaneous equations:
8q + p + r = 0
q + 2p + r = 0
q + p – 1600 = 0
Given,
8q + p + r = 0 …(i)
q + 2p + r = 0 …(ii)
q + p – 1600 = 0 …(iii)
Subtracting (ii) from (i), we get
7q – p = 0 ….(iv)
Solving (iii) and (iv), we get,
q = 200 and p = 1400
And from (i), we get r = –3000
Example 7: Solve the following equations:
(m + n – 8)/2 = (m + 2n – 14)/3 = (3m + n – 12)/11
Taking the first two equations:
(m + n – 8)/2 = (m + 2n – 14)/3
⇒ 3m + 3n – 24 = 2m + 4n – 28
⇒ m – n = – 28 + 24
⇒ m – n = –4 ….(i)
Taking the last two equations:
(m + 2n – 14)/3 = (3m + n – 12)/11
⇒ 11m + 22n – 154 = 9m + 3n – 36
⇒ 2m + 19n = 154 – 36
⇒ 2m + 19n = 118 ….(ii)
Multiplying both sides of (i) by 2 and then subtracting from (ii), we get
19n + 2n = 118 + 8
⇒ 21n = 126
⇒ n = 126/21 = 6
And m = 2
∴ the solution of the given simultaneous equation is m = 2 and n = 6.
The other methods of solving simultaneous equations are:
- Gaussian Elimination
- Jacobi and Gauss-Seidel Iterative Methods
Example 8: A man can row 16 km downstream and 8 km upstream in 6 hours. He can row 6 km upstream and 24 km downstream in 6 hours. Find the speed of man in still water.
Let the speed of a man rowing a boat in still water be x km/hr and the speed of the stream be y km/hr.
Speed of rowing in downstream = x + y = 1/u (let)
Speed of rowing in upstream = x – y = 1/v
Now, given
16/(x + y) + 8/(x – y) = 6
⇒ 16u + 8v = 6
⇒ 8u + 4v = 3 ….(i)
And 24/(x + y) + 6/(x – y) = 6
⇒ 24u + 6v = 6
⇒ 4u + v = 1 ….(ii)
Solving (i) and (ii) we get, u = ⅛ ⇒ x + y = 8 and v = ½ ⇒ x – y = 2
Thus, we get x = 5km/hr and y = 3km/hr.
∴ The speed of the man rowing the boat in still water is 5 km/hr.
Example 9: If (sin 𝜃, cos 𝜃) satisfies the system of equations mx + ny + a + b = a – b and nx + my + 2b = 0, then find the value of 𝜃 where 0≤ 𝜃 ≤ 90o.
Given equations,
mx + ny + a + b = a – b
⇒ mx + ny + 2b = 0 …..(i)
And nx + my + 2b = 0 ….(ii)
Multiply (i) by n and (ii) by m on both sides, subtracting (ii) from (i), we get,
(n2 – m2)y + 2nb – 2mb = 0
y = –2/(m + n)
Similarly, x = –2/(m + n)
That is, x = y ⇒ sin 𝜃 = cos 𝜃, for 𝜃 = 45o, the value of sin 𝜃 is equal to the value of cos 𝜃.
Example 10: A sum of ₹ 400 was distributed among the students of a class. Each boy received ₹ 8, and each girl received ₹ 4. If each girl had received ₹ 10, then each boy would have received ₹ 5. Find the total number of students in the class.
Let the number of boys be x, and the number of girls be y. Then
8x + 4y = 400 and 5x + 10y = 400
⇒ 2x + y = 100 and x + 2y = 80
Multiplying the second equation by 2 on both sides, then subtracting from the first equation, we get,
–3y = –60 ⇒ y = 20 and x = 40
∴ there are 40 boys and 20 girls in the classroom.
|
174 videos|103 docs|119 tests
|




















