Decimals and Operations | Mathematics for Grade 10 PDF Download
| Table of contents |

|
| Adding Decimals |

|
| Adding and Subtracting Decimals |

|
| Dividing Decimals |

|
| Relationship Between Fractions and Decimals |

|
Decimals are used to express the whole number and fraction together. Here, we will separate the whole number from the fraction by inserting a ".", which is called a decimal point. For example, let's say you are going to take a cone of ice cream. The vendor tells you that the price of ice cream is $2 and 50 cents. Now, if you want to express this amount in one figure, you will say that the price of the ice cream cone is $2.50. There are many such real-life situations in which you might be using decimals without even realizing it.
What are Decimals?
Decimals are a set of numbers lying between integers on a number line. They are just another way to represent fractions in mathematics. With the help of decimals, we can write more precise values of measurable quantities like length, weight, distance, money, etc. The numbers to the left of the decimal point are the integers or whole numbers and the numbers to the right of the decimal point are decimal fractions. If we go right from ones place, the next place will be (1/10) times smaller, which will be (1/10)th or tenth place value. For an instance, observe the place value chart of decimals given below for the number 12.45.
Decimals Place Value Chart
In the case of decimals, for the whole number part, the place value system is the same as the whole number. But after the decimal point, there is a different world of numbers going on in which we use decimal fractions to represent the value. When we are going towards the left, each place is ten times greater than the previous place value. So, to the right of ones place, we have tenths (1/10) and to the right of tenths, we have hundredths (1/100), and so on. Let us look at some examples of decimal place values for more clarity. The place values of each digit of the numbers 73.789, 8.350, and 45.08 are shown.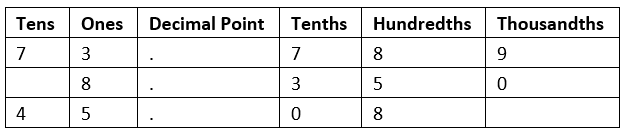
Reading Decimal Numbers: There are two ways to read a decimal number. The first way is to simply read the whole number followed by "point", then to read the digits in the fractional part separately. It is a more casual way to read decimals. For example, we read 85.64 as eighty-five point six-four. The second way is to read the whole number part followed by "and", then to read the fractional part in the same way as we read whole numbers but followed by the place value of the last digit. For example, we can also read 85.64 as eighty-five and sixty-four hundredths.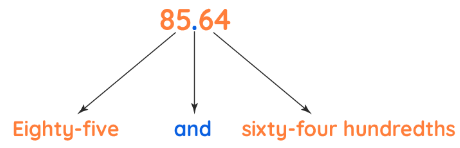
Decimals in Expanded Form
Decimals can also be written in expanded form just like whole numbers. As you know that to write any number in expanded form, we have to write the face value multiplied by the place value of all the digits in the number combined together with an addition sign in between. For writing decimals in expanded form, we will be doing the same. For example, let us write the expanded form of 23.758. The first step is to write the digits of the given number in the place value chart of decimals, as shown below.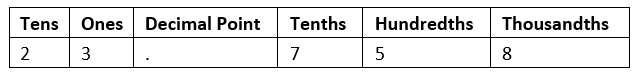
As we can observe, the place values are clearly marked along with the face values of each of the digits of the number 23.758. So, the expanded form of 23.758 can be expressed in the following way:
23.758 = 2 × 10 + 3 × 1 + 7 × 1/10 + 5 × 1/100 + 8 × 1/1000
OR
23.758 = 20 + 3 + 0.7 + 0.05 + 0.008
Rounding Decimals to the Nearest Tenths
Rounding a decimal number to the nearest tenths is done by taking the digit at the hundredth place into consideration. The digit in this hundredth place can have two variations. First, if that number is 4 or less, just remove all the digits to the right of the tenth place digit and the remaining portion is our desired result. But if the digit at the hundredths place is 5 or greater, we need to increase the tenths place digit by 1, and then remove all the digits on the right of the tenths place digit.
For example, let us try rounding 765.27446 to the tenth place. As we can see, the hundredth place digit in 765.27446 is 7. Now, since 7>5, therefore, to round this number to the nearest tenths place, we add 1 to the tenths place digit and ignore the rest. Hence, when we round off 765.27446 to the nearest tenth place, the result will be 765.3.
Comparing Decimals
In order to compare the decimals, keep the following two things in mind. First, compare the whole number part of the given decimals. The decimal number with the greater whole number will be greater than the other number, and vice-versa. Second, if the digits before the decimal point are equal to each other then we compare the first digit after the decimal point which is the tenth place digit, and examine which is greater or smaller. We repeat this process and keep on comparing digits to the right until we get the unequal digits.
For example, let's compare 23.789 with 23.759. Here we see digits before the decimal point are equal which is 23 = 23. Now moving on to the tenth place digits to compare. i.e., 23.789 with 23.759, we get 7 = 7. Both of them are again equal. Now we move to the next term to the right of the tenth place digit which is the hundredth digit. i.e., 23.789 with 23.759. Now, since 8>5, then we can say that 23.789 > 23.759. ∴ 23.789 > 23.759. This is how we compare decimal numbers.
Types of Decimals
Decimals can be divided into different categories depending upon what type of digits occur after the decimal point. It will depend upon whether the digits are repeating, non-repeating, or terminating. Let us have a look at how the decimals are categorized based on their type here.
- Terminating decimals: Terminating decimals mean it does not reoccur and end after a finite number of decimal places. For example: 543.534234, 27.2, etc.
- Non-terminating decimals: It means that the decimal numbers have infinite digits after the decimal point. For example, 54543.23774632439473747..., 827.79734394723... etc. The non-terminating decimal numbers can be further divided into 2 parts:
- Recurring decimal numbers: In recurring decimal numbers, digits repeat after a fixed interval. For example, 94346.374374374..., 573.636363... etc.
- Non-recurring decimal numbers: In non-recurring decimal numbers, digits never repeat after a fixed interval. For example 743.872367346.., 7043927.78687564... and so on.
Interesting Facts and Notes on Decimals
Below given are some interesting facts and notes on decimals. This will help you in understanding the topic faster.
- The prefix "deci" in the word "decimal" means ten.
- Always remember to add the decimal point after the ones place so that we know from where the fraction begins.
- To compare the decimal or fractional part of any decimal number, consider one digit at a time from the right of the decimal point. For example, 4.109 < 4.2, as at the tenths place 1 < 2.
Decimal Example
Example 1: Raven bought 100 apples from a nearby fruit vendor but later found out that 5 of them were rotten. Can you tell the fraction as well as decimals of the rotten apples to the total apples bought by Raven?
Solution: Here, we have 5 rotten apples out of 100.
So our fraction becomes, (5/100) or 1/20.
Now, how do we write it as decimals? Such problems are solved by dividing the numerator by the denominator.
Here, we need to divide 5 by 100.
To divide 5 by 100, we will simply shift the decimal point by 2 places towards the left.
The number of decimal places we can shift in the numerator depends upon the trailing zeroes the whole number in the denominator has.
Thus, after division, we get: 5/100 = 0.05.
Therefore, rotten apples to fresh apples are 0.05 in decimal form.
Adding Decimals
Adding decimals means finding the sum of two or more decimal numbers. Although adding decimals is similar to the regular addition of whole numbers, there are certain rules that need to be followed while adding them.
How to Add Decimals?
Adding decimals is similar to the usual addition of whole numbers. We know that a decimal number is a number with a whole number part and a fractional part which is separated by a decimal point. However, this does not change the rules for the addition of decimal numbers. For this, we need to align the decimal numbers according to their place values one below the other, keeping the decimal point intact, and then add the numbers. We should remember that the decimal place value chart has some extra place values, like, the place value just after the decimal point is called tenths, followed by hundredths, thousandths and so on.
Rules for Adding Decimals
While adding decimals, we need to remember some rules that would be helpful and make the addition easier.
- Align the decimal numbers in such a way that they are placed in the correct place according to their place values.
- Always convert the decimal numbers to like decimals, wherever needed.
- Add zeros in the places wherever the length of the decimal numbers is not the same.
- Now, add the numbers and place the decimal point aligned with the given decimal points.
Adding Decimals with Different Decimal Places
Sometimes, the given numbers are different in their length, that is, they do not have an equal number of decimal digits after the decimal point. In such cases, we convert the given decimal numbers to like decimals by adding the required number of zeros to the right of the decimal. This is done to make the process of addition easier.
Like decimals have an equal number of digits after the decimal, for example, 0.14 and 2.35 are like decimals, whereas, unlike decimals have different number of digits after the decimal, for example, 6.32 and 6.324 are unlike decimals. So, after the given decimals are changed to like decimals, they are added and the sum is obtained.
Adding Like Decimals
Example: Add: 2.53 + 1.14
Solution: If we observe the given decimals, we see that they are like decimals, so, we will place them in order and do the regular addition.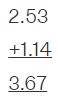
Therefore, after adding the decimals, we get the sum as 3.67
Adding Unlike Decimals
Example: Add: 6.3 + 2.54
Solution: We can see that the given decimals are unlike, so we will convert them to like decimals by adding the required number of zeros.
We will count the number of digits after the decimal point in both the numbers and identify the higher one in them.
In this case, 6.3 has one digit after the decimal, and 2.54 has two digits after the decimal.
So, will make it 6.30 so that it becomes of the same length as 2.54.
Now, we will add the decimals numbers.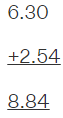
Therefore, the sum of the given decimals is 8.84
Adding Decimals with Whole Numbers
Adding decimals with whole numbers is simple to understand. We place a decimal point after the whole number and add the required number of zeros so as to make both the addends of the same length. This is done in such a way that it aligns with the number of digits in the other number. In other words, we convert them to like decimals and then add them.
Example: Add 4 to 6.54
Solution: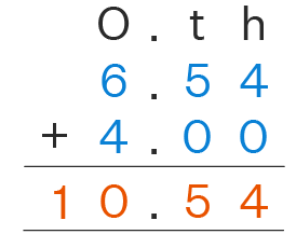
- Step 1: We can see that 4 is a whole number and 6.54 is a decimal number. So, let us make both the numbers of equal length.
- Step 2: Since there are two digits after the decimal in 6.54, we will place a decimal after 4 and add two zeros after it so that it becomes a decimal number too.
- Step 3: Now, we will add the numbers and the sum of the given numbers will be 10.54
Adding Decimals by Regrouping
Adding decimals by regrouping is similar to the regrouping that is done in the addition of whole numbers. Regrouping is also termed as carrying over. In addition, if the sum of the addends is greater than 9 in any of the columns, we regroup it by carrying over the extra digit to the preceding column. Let us understand how to add decimals by regrouping with the following example.
Example: Add 14.62 + 12.63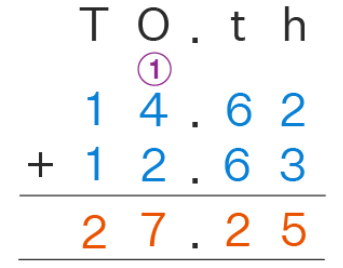 Let us see how to add the given decimals by regrouping.
Let us see how to add the given decimals by regrouping.
- Step 1: Align the given numbers according to their corresponding place value columns and change them to like decimals, if needed. Ensure that the decimal points are aligned with each other.
- Step 2: Start adding digits in each column individually, starting from the right and move to the left in the same way as we do for whole numbers. Add the numbers given in the hundredths column (h). 2 + 3 = 5 and write 5 below the column.
- Step 3: Move to the tenths column (t) and add 6 + 6 = 12. Now, we cannot place '12' in this column since we can write only one digit below each column, so we regroup the number 12. For this, we write 2 in this column and carry 1 to the preceding column, that is, the ones column (O). This 1 (carry-over) is added along with the numbers in the ones column. Remember to place the decimal point below the decimal point.
- Step 4: Now, let us add the numbers under the ones column. Here, after the carry-over is placed in this column, it is added along with the other addends. This will be, 4 + 2 + 1 (carry-over) = 7.
- Step 5: Finally, add the numbers given in the tens column (T), that is, 1 + 1 = 2.
Thus, the sum of the numbers 14.62 + 12.63 is 27.25
Adding and Subtracting Decimals
Adding and subtracting decimals is the same as the addition and subtraction of whole numbers keeping in mind that the decimal point needs to be in place. The length of the decimal numbers can be adjusted by adding or removing zeros from the decimal part.
What is Addition and Subtraction of Decimals?
The addition and subtraction of decimals involves the usual addition and subtraction rules. The only points to be taken care of are the decimal places after the decimal point. The numbers need to be written in columns according to their place values before and after the decimal point. The decimal place value chart shows that the place values before the decimal point start from ones tens, hundreds, and so on; whereas, the place values after the decimal point start from tenths, followed by hundredths, and so on. Let us understand this with the help of the addition of the decimal numbers explained in the following section.
Addition of Decimals
The addition of decimals is done by starting from the right-hand side and then we move on to the left adding each column. For example, let us add 12.5 + 14.9 using the following steps.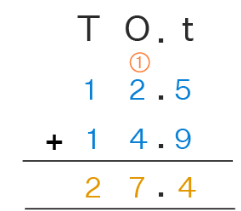
- Step 1: Write the numbers one below the other such that they are aligned as per their place values and the decimal point is placed one below the other.
- Step 2: Now add the decimal numbers to get the sum. In this case, 12.5 + 14.9 = 27.4
Now let us understand the subtraction of decimals in the following section.
Subtraction of Decimals
The subtraction of decimals is done in the same way as the subtraction of whole numbers. We just need to write the numbers correctly according to their place values. Let us understand this with the help of the example given below.
Example: Subtract the given decimals: 15.8 - 2.7
Solution: Let us subtract these decimals using the following steps.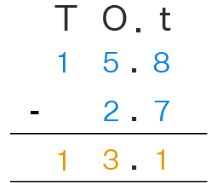
- Step 1: Write the numbers one below the other such that the larger number is on top and the smaller number is written below it.
- Step 2: Now subtract the decimal numbers starting from the tenths column, moving on to the ones column, and then the tens column. Copy the decimal as it comes. In this case, 15.8 - 2.7 = 13.1
Adding and Subtracting Unlike Decimals
'Unlike decimals' are those decimal numbers that do not have the same number of digits after the decimal point. For example, 3.45 and 7.831 are 'unlike decimals' because 3.45 has two numbers after the decimal point and 7.831 has three numbers after the decimal point. In other words, unlike decimals are said to be of different lengths.
The operation of addition and subtraction can be conveniently performed even with these unlike decimals. We just need to convert the 'unlike decimals' to 'like decimals' by writing zeros in the places wherever the length of decimal numbers is not the same. In this way, the decimal digits in each of the numbers becomes equal and it is easier to add or subtract the numbers. Let us understand this with the help of the following example.
Example: Add the decimal numbers 24, 32.1, 0.08, 0.5, and 4.003
Solution: Let us add these numbers using the following steps.
- Step 1: Here, each of the numbers needs to have an equal number of decimal digits.
- Step 2: For this, we rewrite the numbers as follows: 24.000, 32.100, 0.080, 0.500, and 4.003. Therefore, the sum of the given decimal numbers is 60.683
How to Add and Subtract Decimals with Whole Numbers?
For adding or subtracting a decimal and a whole number, the whole number is changed into a decimal number. This is done by placing a decimal after the whole number and then writing the required number of zeros after the decimal point. For example, the whole number 5 is written in the decimal form as 5.0. Let us understand this with the help of the following examples.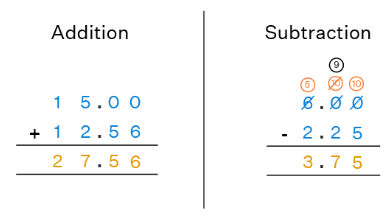 Example: Add 15 + 12.56
Example: Add 15 + 12.56
- Step 1: Refer to the figure given above and place a decimal after 15 and write two zeros after it so that both the numbers become like decimals.
- Step 2: Now add the decimal numbers and find the sum using the usual rules of addition. Therefore, the 15 + 12.56 = 27.56
Let us understand this using subtraction.
Example: Subtract 6 - 2.25
- Step 1: Refer to the figure given above and place a decimal after 6 and write two zeros after it so that both the numbers become like decimals.
- Step 2: Now subtract the decimal numbers and find the difference using the usual rules of subtraction. Therefore, 6 - 2.25 = 3.75
Important Points on Addition and Subtraction of Decimals
- The value of a decimal does not change on placing a zero after the decimal digits. For example, 5 can be written as 5.00
- Even though the time and angle measure is represented in decimal format, they cannot be added or subtracted as decimals.
Dividing Decimals
Dividing Decimals is similar to dividing whole numbers, keeping in mind the position of the decimal point. While dividing decimal numbers, we need to follow a certain set of rules but the basic process of division remains the same.
How to Divide Decimals?
The process of dividing decimals is similar to the normal division process, but we just need to keep in mind the decimal point which should be correctly placed in the quotient. A decimal number has a whole number part and a fractional part separated by a dot which is the decimal point.
The digits after the decimal point have a value smaller than 1. For example, 24.15 is a decimal number in which 24 is the whole number part and 15 is the fractional part. For dividing decimal numbers, we can come across 2 scenarios - one in which we need to divide decimals by a whole number, and the other in which we divide decimals by decimals. Let us read more about this in the following sections.
Dividing Decimals by Whole Numbers
Dividing decimals by whole numbers is similar to normal division. Here, the dividend is a decimal number and the divisor is a whole number, so the decimal point in the quotient will be placed according to the decimal point of the dividend. We can understand this with the help of the long division of decimals.
Long Division of Decimals
The long division of decimals can be easily done like the normal long division. Let us understand this using an example.
Example: Divide 338.56 ÷ 23
Solution: 
- Step 1: First, write the division in the standard form. Start by dividing the whole number part by the divisor.
- Step 2: Place the decimal point in the quotient above the decimal point of the dividend. Bring down the tenth digit.
- Step 3: Divide and bring down the other digit in sequence. Divide until 0 is obtained in the remainder. Thus, the decimal in the quotient is placed according to the decimal in the dividend.
Dividing Decimals by Decimals
For dividing decimals by another decimal, we need to convert the divisor into a whole number and then continue the division. Let us understand the conditions and rules for this method using an example.
Example: Divide 48.65 ÷ 3.5
Solution: In this division, the dividend and the divisor are decimals, so we need to convert the divisor to a whole number using the following steps.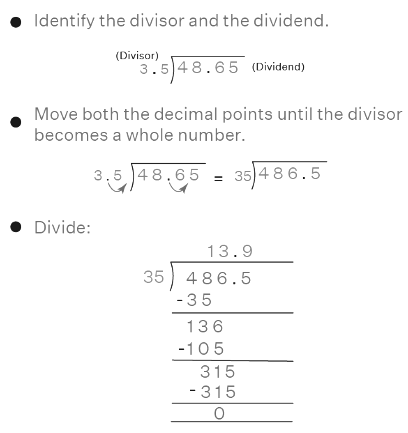
- Step 1: The dividend is 48.65 and the divisor is 3.5. We need to change the divisor to a whole number and so we will multiply it by 10 so that the decimal point shifts to the right and it becomes a whole number. This means, 3.5 × 10 = 35.
- Step 2: We need to treat the dividend in the same way as we had treated the divisor. So, we will multiply the dividend by 10 as well. This means it will be 48.65 × 10 = 486.5. In other words, we need to move both the decimal points to the right until the divisor becomes a whole number.
- Step 3: Now, we have 486.5 as the dividend and 35 as the divisor. This can be divided as we do the usual division and we get 13.9 as the quotient.
Important Tips on Dividing Decimals
The following tips that are helpful while dividing decimals.
- Convert the divisor to a whole number by multiplying by the powers of 10. Multiply the dividend by the same powers of 10.
- In order to divide a decimal number by 10, move the decimal point to the left by one place. For example, if we need to divide 45.67 ÷ 10, then it can be easily done by shifting the decimal point to the left and the answer will be 4.567
- In order to divide a decimal number by 100, move the decimal point to the left by two places. For example, if we need to divide 324.6 ÷ 100, then it can be easily done by shifting the decimal point to the left and the answer will be 3.246
- In order to divide a decimal number by 1000, move the decimal point to the left by three places. For example, if we need to divide 8934.5 ÷ 1000, then it can be easily done by shifting the decimal point to the left and the answer will be 8.9345
Relationship Between Fractions and Decimals
The relationship between fractions and decimals is very important to understand to develop a strong base in arithmetic. When a number is represented in form of p/q, where p and q belong to whole numbers and q is not equal to 0, it is termed as a fraction and can be converted into a decimal form by either converting the denominator to a power of 10 or by long division method.
What is the Relationship Between Fractions and Decimals?
Both fractions and decimals are just two ways to represent numbers. Fractions are written in the form of p/q, where q≠0, while in decimals, the whole number part and fractional part are connected through a decimal point, for example, 0.5. Fractions and decimals represent the relationship of part by whole. In both fractions and decimals, we represent the whole by 1.
Let us look at some examples to understand the relationship between fractions and decimals. Consider a full-thin crust pizza with 6 slices. Your mother gave half of it, i.e., 3 slices then in fractional form, we write it as 1/2, and in the decimal form, we write it as 0.5.
Let us consider another example. Emma divides her garden into 12 equal parts. She grows flowers of different colors in each part of the garden. Amongst the 12 slots, she reserved 8 equal portions for red flowers, 2 portions for yellow color flowers, and 2 for blue color flowers. Let us write the portion given to flowers of each color in fraction and in decimal.
- Red flowers are grown in the 8/12 or 0.666 part of the garden.
- Yellow flowers are grown in the 2/12 or 0.1666 part of the garden.
- Blue flowers are also grown in the 2/12 or 0.1666 part of the garden.
The fraction and decimal representation given in the chart below to have more clarity about the fractions and decimals relationship.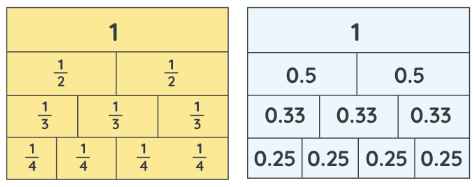
Converting Fraction into Decimal
We can convert a fraction to its decimal form by the following two methods.
- Long Division Method
- Convert the denominator of the fraction to multiples of 10 like 10, 100, 1000, etc.
Converting Fraction to Decimal by Long Division Method
When a number is present in a fraction form i.e., p/q, to convert it into the decimal form we use the long division method. The steps for converting fractions into decimals are given below. Let us understand these steps of the long division with the help of an example.
Convert 3/8 into decimals.
Step 1: Treat the numerator digit of the fraction as a dividend and the denominator as the divisor. In this case, the numerator is lesser than the denominator.
Step 2: Make the dividend greater than the numerator by placing 0 next to the digit and to the quotient. Now we have 30 as a new dividend. (30>8).
Step 3: In the quotient, place decimal after 0 and start the division.
Step 4: Multiply 8 with a number so that the product is less than equal to 30. 8 times 3 is 24. Here now the digit in the quotient is 3, the remainder is 6. After introducing decimal in the quotient we can attach one 0 at each step of division.
Step 5: Now the new dividend is 60. Multiply 8 with a number so that the product is less than equal to 60. 8 times 7 is 56. Here now quotient is 7, the remainder is 4.
Step 6: Now the new dividend is 40. Multiply 8 with a number so that the product is less than equal to 40. 8 times 5 is 40.
Step 7: The final remainder is 0 and the quotient is 0.375.
3/8 = 0.375.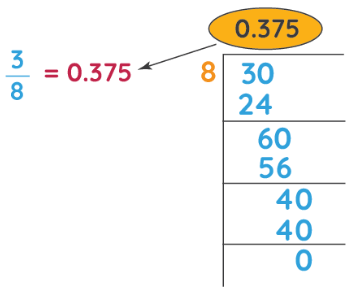
Convert the Denominator
Another method to convert the fraction to a decimal is by converting the denominator of the fraction to powers of 10 like 10, 100, 1000, etc. Let us understand this with the steps given below. We will take an example to proceed with the given steps.
Convert 3/4 to decimals.
Step1: Think of a number by which we can easily multiply the denominator and numerator to get the power of 10.
Step2: Here denominator is 4. 4 times 25 is 100.
Step 3: Multiply the numerator also with the same number
Step 4: By multiplying the numerator of the fraction by 25 we get (3 × 25) = 75
Step 5: Now we have a denominator in terms of powers of 10.
Step 6: 75/100 = 0.75.
The decimal place of the final answer depends upon the number of trailing zeros present in the digit of the denominator.
Converting Decimal into Fraction
Every decimal number can be expressed in the form of a fraction. Steps to convert a decimal number to the fractional form are stated below:
- Rewrite the number by ignoring the decimal point.
- Divide the number by the power of 10 such that the number of zeros in that should be equal to the number of decimal places in the given number.
- Simplify the fraction.
Look at this example for a deeper understanding.
Decimal form = 6.5 = 65/10 = 13/2 (Fraction form of 6.5)
Important Notes:
- Fractions represent a ratio between two numbers, so they show finite value. For example, 1/3, or we can say 1 out of 3 parts.
- Decimals can also represent infinite values along with finite values. For example, if we convert the above fraction to decimal, we get 0.33333333 and it goes on up to infinity.
|
138 videos|67 docs|41 tests
|

|
Explore Courses for Grade 10 exam
|

|
















