Grade 10 Exam > Grade 10 Notes > Mathematics for Grade 10 > Quartiles Quartiles
Quartiles Quartiles | Mathematics for Grade 10 PDF Download
Quartile Formula
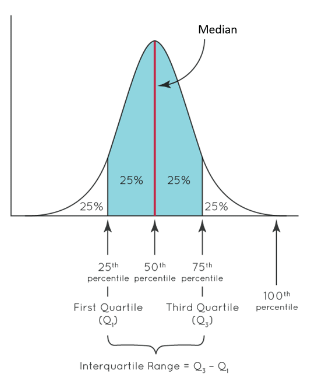
The quartile formula helps in calculating the value that divides a list of numbers into quarters. The data is firstly arranged into ascending order and is then divided into quartiles. Median divides a given data into two equal parts while quartiles divide it into four parts. Using the quartile formula we can find the first quartile, second quartile, and third quartile.
What Is Quartile Formula?
The quartile formula helps to divide a set of observations into 4 equal parts. The first quartile lies in the middle of the first term and the median. The median is the second quartile. The middle value lying between the median and the last term is the third quartile. Mathematically, they are represented as follows,
When the set of observations are arranged in ascending order the quartiles are represented as,
- First Quartile(Q1) = ((n + 1)/4)th Term
- Second Quartile(Q2) = ((n + 1)/2)th Term
- Third Quartile(Q3) = (3(n + 1)/4)th Term
1st quartile is also known as the lower quartile. 2nd quartile is the same as the median dividing data into 2 equal parts. 3rd quartile is also called the upper quartile.
The interquartile range is calculated as Upper Quartile – Lower Quartile.
Solved Example
Example 1: Calculate the median, lower quartile, upper quartile, and interquartile range of the following data set of values: 20, 19, 21, 22, 23, 24, 25, 27, 26
Arranging the values in ascending order: 19, 20, 21, 22, 23, 24, 25, 26, 27
Putting the values in the formulas above we get,
Median(Q2) = 5th Term = 23
Lower Quartile (Q1) = Mean of 2nd and 3rd term = (20 + 21)/2 = 20.5
Upper Quartile(Q3) = Mean of 7th and 8th term = (25 + 26)/2 = 25.5
IQR = Upper Quartile−Lower Quartile
IQR = 25.5 – 20.5
IQR = 5
Example 2: What will be the upper quartile for the following set of numbers?
26, 19, 5, 7, 6, 9, 16, 12, 18, 2, 1.
The formula for the upper quartile formula is Q3 = ¾(n + 1)th Term.
The formula instead of giving the value for the upper quartile gives us the place. For example, 8th place, 10th place, etc.
So firstly we put your numbers in ascending order: 1, 2, 5, 6, 7, 9, 12, 16, 18, 19, 26. There are a total of 11 numbers, so:
Q3 = ¾(n + 1)th Term.
Q3 = ¾(12)th Term. = 9th Term.
The upper quartile (18) is the 9th term or on the 9th place from the left.
Example 3: Find the 3rd quartile in the following data set: 4, 5, 8, 7, 11, 9, 9
Let us first arrange our array in ascending order and it becomes 4, 5, 7, 8, 9, 9, 11
The median of our data is 8.
In order to find the 3rd quartile, we have to deal with the data points that are greater than the median that is 9, 9, 10.
In order to find the 3rd quartile, we have to find the median of the data points that are greater than the median that is 9, 9, 10.
The document Quartiles Quartiles | Mathematics for Grade 10 is a part of the Grade 10 Course Mathematics for Grade 10.
All you need of Grade 10 at this link: Grade 10
|
138 videos|67 docs|41 tests
|
Related Exams
About this Document
|
Nov 22, 2024 Last updated |
Document Description: Quartiles Quartiles for Grade 10 2024 is part of Mathematics for Grade 10 preparation.
The notes and questions for Quartiles Quartiles have been prepared according to the Grade 10 exam syllabus. Information about Quartiles Quartiles covers topics
like Quartile Formula, What Is Quartile Formula? , Solved Example and Quartiles Quartiles Example, for Grade 10 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Quartiles Quartiles.
Introduction of Quartiles Quartiles in English is available as part of our Mathematics for Grade 10
for Grade 10 & Quartiles Quartiles in Hindi for Mathematics for Grade 10 course.
Download more important topics related with notes, lectures and mock test series for Grade 10
Exam by signing up for free. Grade 10: Quartiles Quartiles | Mathematics for Grade 10
Description
Full syllabus notes, lecture & questions for Quartiles Quartiles | Mathematics for Grade 10 - Grade 10 | Plus excerises question with solution to help you revise complete syllabus for Mathematics for Grade 10 | Best notes, free PDF download
Information about Quartiles Quartiles
In this doc you can find the meaning of Quartiles Quartiles defined & explained in the simplest way possible. Besides explaining types of
Quartiles Quartiles theory, EduRev gives you an ample number of questions to practice Quartiles Quartiles tests, examples and also practice Grade 10
tests
|
Explore Courses for Grade 10 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
past year papers
,Quartiles Quartiles | Mathematics for Grade 10
,Important questions
,video lectures
,shortcuts and tricks
,MCQs
,ppt
,Quartiles Quartiles | Mathematics for Grade 10
,Extra Questions
,practice quizzes
,Previous Year Questions with Solutions
,Summary
,Objective type Questions
,Quartiles Quartiles | Mathematics for Grade 10
,Exam
,Free
,Semester Notes
,Sample Paper
,mock tests for examination
,Viva Questions
,study material
;
Additional Information about Quartiles Quartiles for Grade 10 Preparation
Quartiles Quartiles Free PDF Download
The Quartiles Quartiles is an invaluable resource that delves deep into the core of the Grade 10 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Quartiles Quartiles now and kickstart your journey towards success in the Grade 10 exam.
Importance of Quartiles Quartiles
The importance of Quartiles Quartiles cannot be overstated, especially for Grade 10 aspirants.
This document holds the key to success in the Grade 10 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Quartiles Quartiles Notes
Quartiles Quartiles Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Quartiles Quartiles.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Quartiles Quartiles Notes on EduRev are your ultimate resource for success.
Quartiles Quartiles Grade 10 Questions
The "Quartiles Quartiles Grade 10 Questions" guide is a valuable resource for all aspiring students preparing for the
Grade 10 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Quartiles Quartiles on the App
Students of Grade 10 can study Quartiles Quartiles alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Quartiles Quartiles,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Quartiles Quartiles is prepared as per the latest Grade 10 syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup