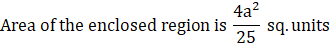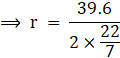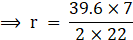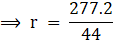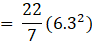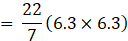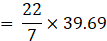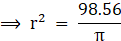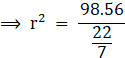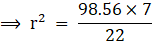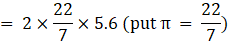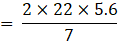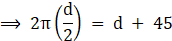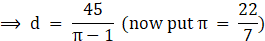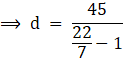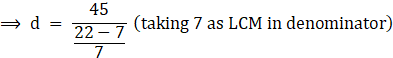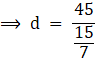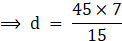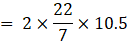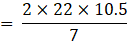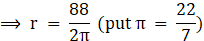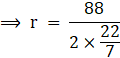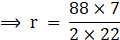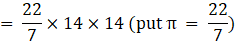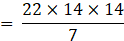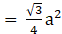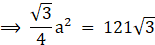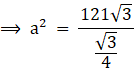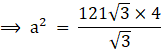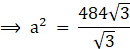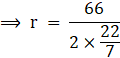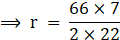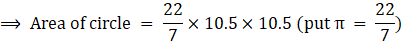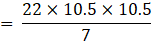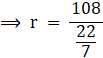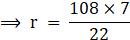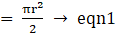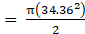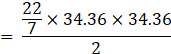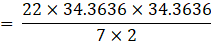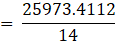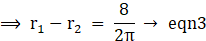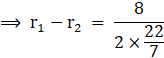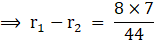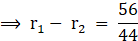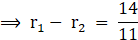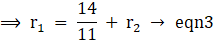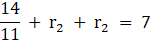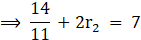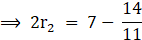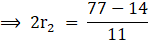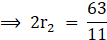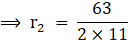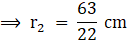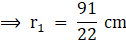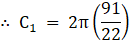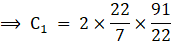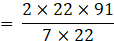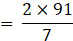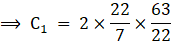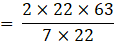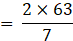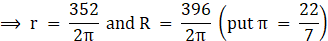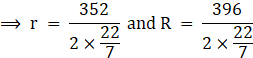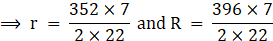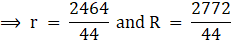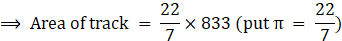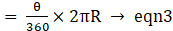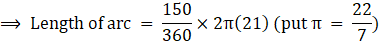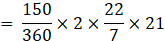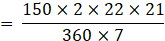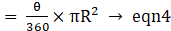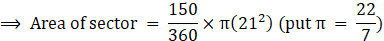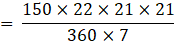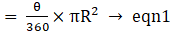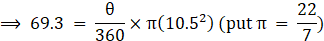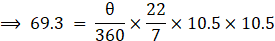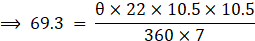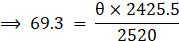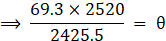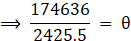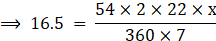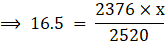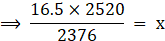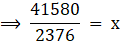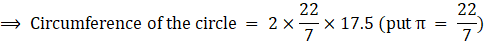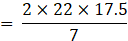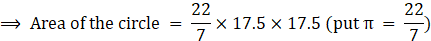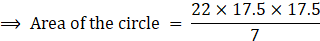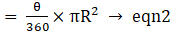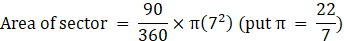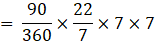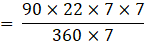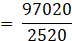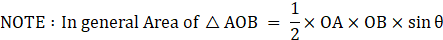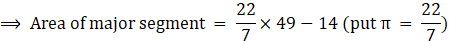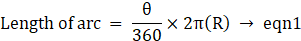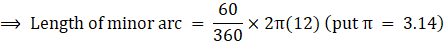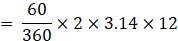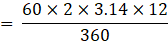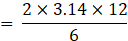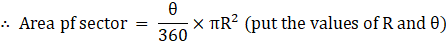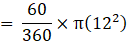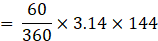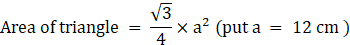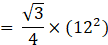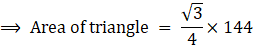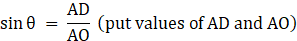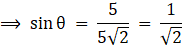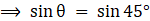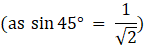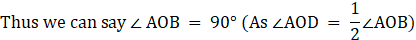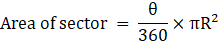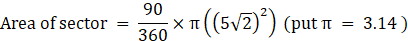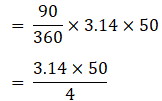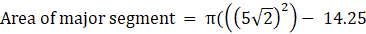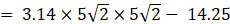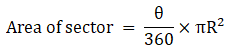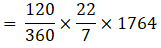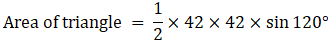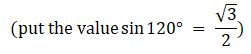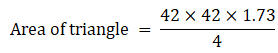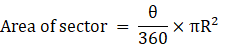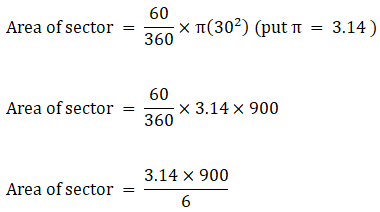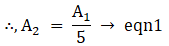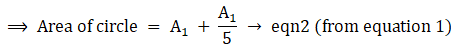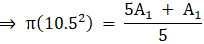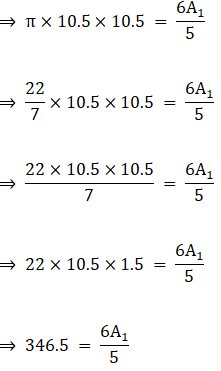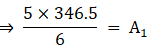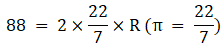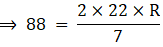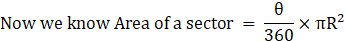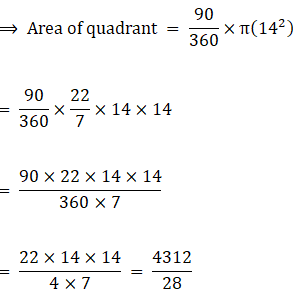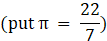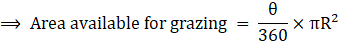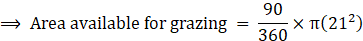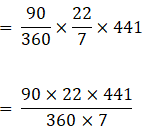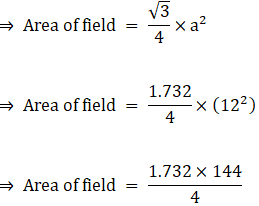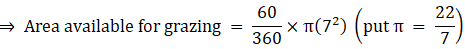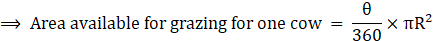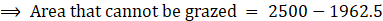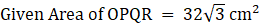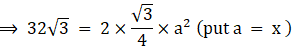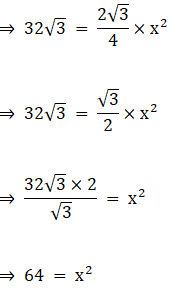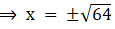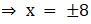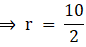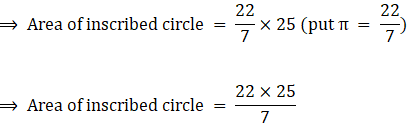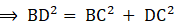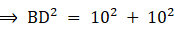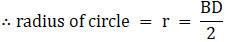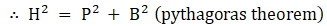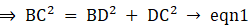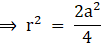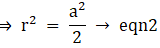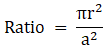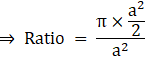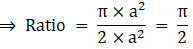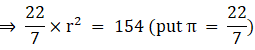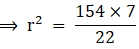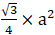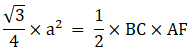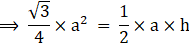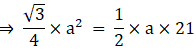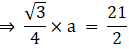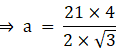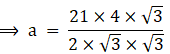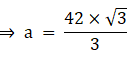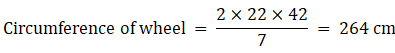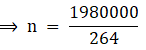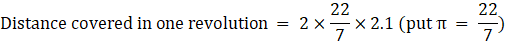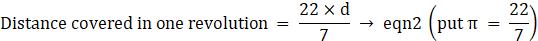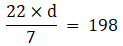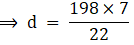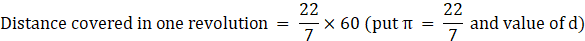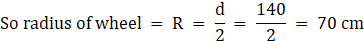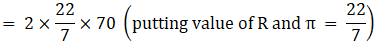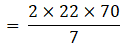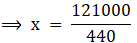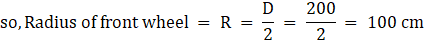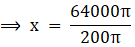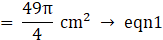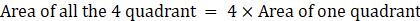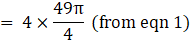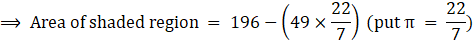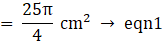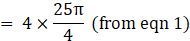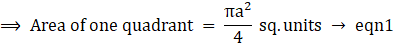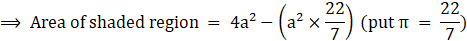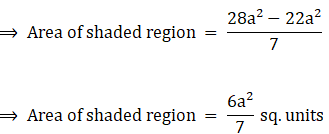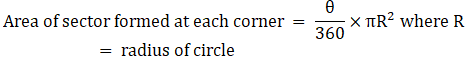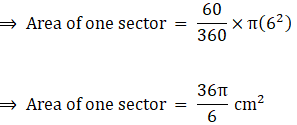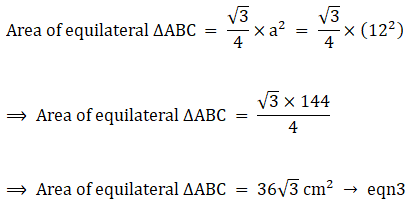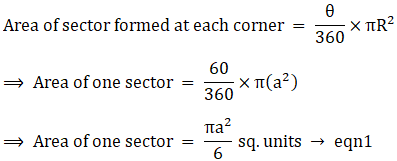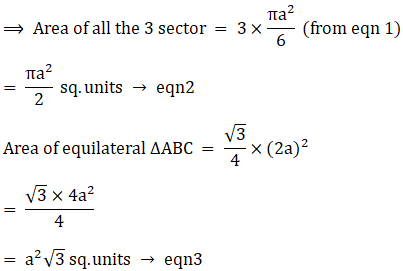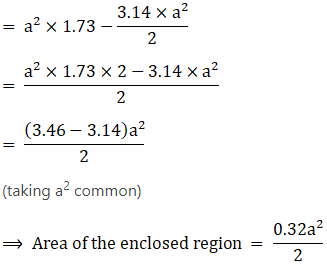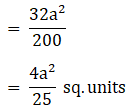RS Aggarwal Solutions: Area of Circle, Sector and Segment- 2 - Class 10 PDF Download
Exercise: 18b
Q.1. The circumference of a circle is 39.6 cm. Find its area.
In order to solve such type of questions we basically need to find the radius of the give circle and simply use it to find the area of the given circle.
Given the circumference or perimeter of the circle = 39.6 cm.
And we know, Perimeter or circumference of circle = 2πr
Where, r = Radius of the circle
Therefore, 2πr = 39.6
⇒ r = 39.6/2π
(put value of π = 22/7)
On rearranging we get,
⇒ r = 6.3 cm
So, the radius of the circle = 6.3 cm
And we also know, Area of the circle = πr2
Where, r = radius of the circle
⇒ Area of the circle = π(6.3)2
(putting value of r)
= 22×5.67
= 124.74 cm2
The area of the circle = 124.74 cm2.
Q.2. The area of a circle is 98.56 cm2. Find its circumference.
In order to solve such type of questions we basically need to find the radius of the give circle and simply use it to find the are circumference or perimeter of the given circle.
Given the area of the circle = 98.56 cm2
And we also know, Area of the circle = πr2
Therefore, πr2 = 98.56
(put value of π = 22/7)
On rearranging we get,
⇒ r2 = 689.92/22
⇒ r2 = 31.36
⇒ r = √31.36
⇒ r = 5.6 cm
So, the radius of the circle = 5.6 cm
And we know, Perimeter of circle = 2πr
(put value of r)
⇒ Circumference or Perimeter of circle = 2π(5.6)
= 246.4/7
= 35.2 cm
The circumference or perimeter of the circle is 35.2 cm.
Q.3. The circumference of a circle exceeds its diameter by 45 cm. Find the circumference of the circle.
Given, the circumference of a circle exceeds its diameter by 45 cm.
⇒ Circumference of circle = Diameter of circle + 45
Let ‘d’ = diameter of the circle
⇒ Circumference = d + 45 → eqn1
And we know, Circumference of a circle = 2πr → eqn2
Where r = radius of circle
Also, we know that the radius of the circle is half of its diameter.
⇒ r = d/2 → eqn3
Put value of circumference in equation 1 from equation 2
⇒ 2πr = d + 45 → eqn4
Put value of r in equation 4 from equation 3
⇒ πd = d + 45
⇒ πd – d = 45
⇒ (π – 1)d = 45 (taking d common from L.H.S)
On rearranging, we get
⇒ d = 315/15
⇒ d = 21 cm
Therefore, the diameter of the circle is 21 cm.
Thus, the radius of the circle r = d/2(from equation 3)
∴ r = 21/2
⇒ r = 10.5 cm
Now put the value of r in equation 2, we get
= 462/7 = 66 cm
The circumference of the circle is 66 cm.
Q.4. A copper wire when bent in the form of a square encloses an area of 484 cm2. The same wire is now bent in the form of a circle. Find the area enclosed by the circle.
In this question the wire is first bent in the shape of square and then same wire is bent to form a circle. The point to be noticed is that the same wire is used both the times which implies that the perimeter of square and that of circle will be equal.

Let the square be of side ‘a’ cm and radius of the circle be ‘r’
Given the area enclosed by the square = 484 cm2
Also, we know that Area of square = Side × Side
Area of the square = a2
⇒ a2 = 484
⇒ a = √484
⇒ a = 22 cm
Therefore, side of square, ‘a’ is 22 cm.
Also, circumference of the circle = Perimeter of square → eqn1
Perimeter of square = 4 × side
Perimeter of square = 4×22
⇒ Perimeter of square = 88 cm → eqn2
Also, we know, Circumference of circle = 2πr → eqn3
Put values in equation 1 from equation 2 & 3, we get
2πr = 88
On rearranging,⇒ r = 616/44
⇒ r = 14 cm
So, the radius ‘r’ of the circle is 14 cm.
Area of circle = πr2
Where r = radius of the circle
= π(142)
= 4312/7
= 616 cm2
Area of the circle is 616 cm2.
Q.5. A wire when bent in the form of an equilateral triangle encloses an area of 121√3 cm2. The same wire is bent to form a circle. Find the area enclosed by the circle.
In this question the wire is first bent in the shape of equilateral triangle and then same wire is bent to form a circle. The point to be noticed is that the same wire is used both the times which implies that the perimeter of equilateral triangle and that of circle will be equal.
Let the equilateral triangle be of side ‘a’ cm and radius of the circle be ‘r’.
Given: Area enclosed by equilateral triangle = 123√3 cm2
Also, we know that Area of equilateral triangle =
Where ‘a’ = side of equilateral triangle
⇒ a = √484
⇒ a = 22 cm
Therefore, side of equilateral triangle, ‘a’ is 22 cm.
Also, circumference of the circle = Perimeter of equilateral triangle → eqn1
Perimeter of equilateral triangle = 3 × side
= 3 × 22
= 66 cm → eqn2
Also, we know Circumference of circle = 2πr → eqn3
Put values in equation 1 from equation 2 & 3, we get
2πr = 66
⇒ r = 66/2π
(put π = 22/7)
On rearranging,
⇒ r = 462/44
⇒ r = 10.5 cm
So, the radius ‘r’ of the circle is 10.5 cm.
Area of circle = πr2
Where r = radius of the circle
⇒ Area of circle = π(10.52)
= 2425.5/7
= 346.5 cm2
Area of the circle is 346.5 cm2.
Q.6. The length of a chain used as the boundary of a semicircular park is 108 m. Find the area of the park.
In this question the length of chain used as boundary of the semicircular park is the perimeter of the semicircular park. By using this we will first calculate the radius of the semicircular park and then area of semicircle consequently.
Length of chain = 108 m
Length of chain = Perimeter or circumference of semicircle
Therefore, Circumference or Perimeter of semicircle = 108 m
Also, Circumference or Perimeter of semicircle = πr
Where r = radius of semicircle
⇒ πr = 108
⇒ r = 108/π
(put π = 22/7)
On rearranging,
⇒ r = 756/22
⇒ r = 34.46 m
Therefore, radius of semicircle is 34.36 m
As, Area of semicircle
Put value of ‘r’ in equation 1, we get
Area of semicircle
(put π = 22/7)
On rearranging,
= 1855.63 m2
The area of the semicircular park is 1855.63 m2.
Q.7. The sum of the radii of two circles is 7 cm, and the difference of their circumferences is 8 cm. Find the circumferences of the circles.
Given Sum of the radius of the circles = 7 cm
the difference of their circumference = 8 cm
Let the radius one circle be ‘r1’ cm and other be ‘r2’ cm and circumference be ‘C1’ and ‘C2’ respectively.
Also, circumference of circle = 2πr
Where r = radius of the circle
C1 = 2πr1 and C2 = 2πr2
r1 + r2 = 7 → eqn1
C1 – C2 = 8 → eqn2
(Note: Her it is considered that r1>r2)
We can rewrite equation 2 as,
2πr1 – 2πr2 = 8
⇒ 2π(r1 – r2) = 8
(taking 2π common from L.H.S)
Put the value of r1 from equation 3 in equation 1
(taking 11 as LCM on R.H.S)
Put value of r2 in equation 3(from equation 3)
(taking 22 as LCM on R.H.S)
(by putting value of r1)
= 182/7
= 26 cm(by putting value of r2)
= 126/7
= 18 cm
The circumference of circles are 26 cm and 18 cm.
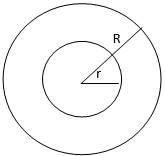
Q.8. Find the area of a ring whose outer and inner radii are respectively 23 cm and 12 cm.
Consider the ring as shown in the figure below,
The inner radius of ring is ‘r’ and the outer radius is ‘R’.
Area of inner Circle = πr2 and Area of outer Circle = πR2
Where r = 12 cm and R = 23 cm
Area of ring = Area of outer circle – Area of inner circle
Area o ring = πR2 – πr2 (put values of r & R)
⇒ Area of ring = π(232) – π(122)
⇒ Area of ring = π(232 – 122) (taking π common from R.H.S)
⇒ Area of ring = π(529 – 144)
= 8470/7
= 1210 cm2
Area of ring is 1210 cm2.
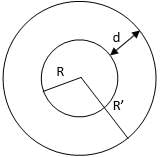
Q.9. A path of 8 m width runs around the outside of a circular park whose radius is 17 m. Find the area of the path.
Given radius of circular park = R = 17 m
Width of the circular path outside the park = d = 8 m
Therefore, the radius of the outer circle = R’ = R + d
Outer radius = R’ = 17 + 8
R’ = 25 m
Area of inner circle = πR2 and,
Area of outer circle = πR’2
Area of path = Area of outer circle – Area of inner circle
= πR’2 – πR2 (put values of R’ & R)
= π(252) – π(172)
= π(252 – 172) (taking π common from R.H.S)
= π(625 – 289)
(put π = 22/7)
= 7392/7
= 1056 m2
The area of the path is 1056 m2.
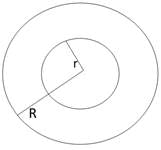
Q.10. A racetrack is in the form of a ring whose inner circumference is 352 m and outer circumference is 396 m. Find the width and the area of the track.
Consider the race track as shown below,
The inner and outer radius of track is ‘r’ cm and ‘R’ cm respectively.
Let inner and outer circumference be ‘C1’ and C2’ respectively.
C1 = 352 m and C2 = 396 m.
We know,
Circumference of circle = 2πr
Where r = radius of the circle
C1 = 2πr and C2 = 2πR
⇒ 2πr = 352 and 2πR = 396
On rearranging,
⇒ r = 56 m and R = 63 m
So, the width of the race track = R – r,
⇒ Width of the race track = 63 – 56
⇒ Width of the race track = 7 m
Area of race track = area of outer circle – area of inner circle
⇒ Area of track = πR2 – πr2 (put values of r and R)
⇒ Area of track = π(632) – π(562)
⇒ Area of track = π(632 – 562) (taking π common from R.H.S)
⇒ Area of track = π(3969 – 3136)
⇒ Area of track = π×833
= 22×119
= 2618 m2
The width of tack is 7 m and area of track is 2618 m2.
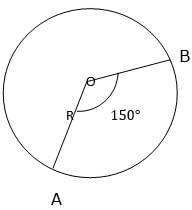
Q.11. A sector is cut from a circle of radius 21 cm. The angle of the sector is 150°. Find the length of the arc and the area of the sector.
Consider the circle shown above,
Given radius of the circle = R = 21 cm → eqn1
And angle of the sector = θ = 150o→ eqn2
Length of arc of a sector
Where ‘R’ = radius of sector (or circle)
θ = angle subtended by the arc on the centre of the circle
Put the values of R and θ from equation 1 and 2 in equation 3
= 138600/2520
= 55 cm
Area of a sector
Where ‘R’ = radius of sector (or circle)
θ = angle subtended by the arc on the centre of the circle
Put the values of R and θ from equation 1 and 2 in equation 3
= 1455300/2520
= 577.5 cm2
The length of arc is 55 cm and area of sector is 577.5 cm2.
Q.12. The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
Consider the circle shown above,
We know , Area of sector
Where R = radius of the circle and θ = central angle
Given R = 10.5 cm and Area of sector = 69.3 cm2
Let the angle subtended at centre = θ
Put the values of R and area of sector in equation 1
⇒ θ = 72°
The central angle of the sector is 72°.
Q.13. The length of an arc of a circle, subtending an angle of 54° at the centre is 16.5 cm. Calculate the radius, circumference and area of the circle.
Consider the Circle shown above,
We know, Length of arc of sector
Where R = radius of circle and θ = central angle of the sector
Given, Length of arc = ℓ = 16.5 cm and θ = 54o. Let the radius be x cm
Put the values of ℓ and θ in equation 1
On rearranging
⇒ x = 17.5 cm
Also, we know circumference of the circle = 2πR
⇒ Circumference of the circle = 2πx (put value of x in this equation)
⇒ Circumference of the circle = 2π(17.5)
= 770/7
⇒ Circumference of the circle = 110 cm
Also, we know Area of the circle = πR2
⇒ Area of the circle = πx2
⇒ Are of the circle = π(17.52)
⇒ Area of the circle = 962.5 cm2
The radius of circle is 17.5 cm, circumference is 110 cm and area is 962.5 cm2
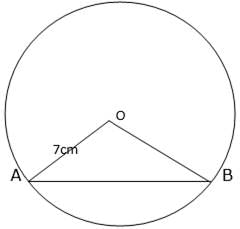
Q.14. The radius of a circle with centre O is 7 cm. Two radii OA and OB are drawn at right angles to each other. Find the areas of minor and major segments.
Consider the above figure,
From here we can conclude that the portion or the segment below the chord AB is the minor segment and the segment above AB is major segment.
Also we know,
Area of minor segment = Area of sector – Area of ∆AOB → eqn1
Now, Area of sector
Where R = radius of the circle and θ = central angle of the sector
Given, R = 7 cm and θ = 90°
Putting these values in the equation 2, we get
⇒ Area of sector = 38.5 cm2→ eqn3
Area of △AOB = 1/2 × base × height
As triangle is isosceles therefore height and base both are 7 cm.
⇒ Area of △AOB = 1/2×7×7 = 49/2
= 24.5 cm2→ eqn4
Putting values of equation 2 and 4 in equation 1 we get
Area of minor segment = 38.5 – 24.5
⇒ Area of minor segment = 14 cm2
Area of major segment = πR2 – Area of minor segment → eqn5
Put the value of R, and Area of minor segment in equation 5
= π(72) – 14
= 49π - 14
= (22×7) - 14
= 154 - 14
= 140 cm2
Area of minor segment is 14 cm2 and of major segment is 140 cm2.
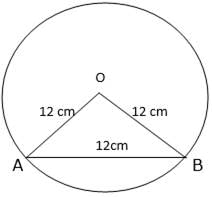
Q.15. Find the lengths of the arcs cut off from a circle of radius 12 cm by a chord 12 cm long. Also, find the area of the minor segment. [Take π = 3.14 and √3 = 1.73.]
Consider the figure shown above.
In this, the triangle AOB is an equilateral triangle as all the sides are equal; therefore, it is obvious that the central angle of the sector is 60 degrees. Now by simply applying the formula of length of an arc, we can easily calculate the length of arc of the sector AOB.
Given Radius of circle = R = 12 cm,
Length of chord AB = 12 cm
∴ Central angle = θ = 60° (∵ ∆AOB is an equilateral triangle)
Where R = radius of the circle and θ = central angle of the sector
Put the values of R and θ in equation 1
= 2×3.14×2
= 12.56 cm
Now, Length of major arc = 2πR – Length of minor arc
⇒ Length of major arc = 2π(12) – 12.56 (put π = 3.14)
⇒ Length of major arc = (2×3.14×12) – 12.56
⇒ Length of major arc = 75.36 – 12.56
⇒ Length of major arc = 62.8 cm
Now, Area of minor segment = Area of sector – Area of triangle → eqn1
= 75.36 cm2→ eqn2
⇒ Area of triangle = 1.73×36
⇒ Area of triangle = 62.28 cm2→ eqn3
Put the values of equation 2 and 3 in equation 1,
∴ Area of minor segment = 75.36 – 62.28
= 13.08 cm2
Length of major arc is 62.8 cm and of minor arc is 12.56 cm and area of minor segment is 13.08 cm2.
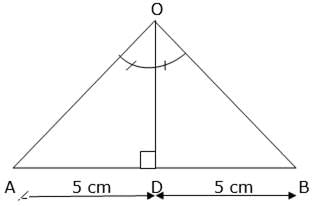
Q.16. A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the areas of both the segments. [Take π = 3.14.]
Consider the figure shown above.
In this, the triangle AOB is an isosceles triangle. So here we will construct a perpendicular bisector from O on AB and as this triangle is isosceles therefore this perpendicular will also act as median and angle bisector.
Therefore,
Draw a perpendicular bisector from O which meets AB at D and bisects AB, as ABO is an isosceles triangle therefore OD acts as a median.
So, consider right angle triangle AOD right angled at D
Let ∠AOD = θ ⇒ Perpendicular = AD and Hypotenuse = AO = R
Given Radius of circle = R = 5√2 cm
Length of chord AB = 10 cm, AD = 5 cm
⇒ θ = 45°
∴ ∠AOD = 45°
Area of minor segment = Area of sector – Area of right angle triangle
→ eqn1
Where R = radius of the circle and θ = central angle of the sector
∴ Area of sector = 39.25 cm2
Area of right angle triangle = 1/2 × base × height
As this is isosceles right-angle triangle
∴ height = base = 5√2 cm
Area of right angle triangle = 1/2 ×5√2×5√2 = 50/2 = 25 cm2
Put the value of area of sector and area of right angle triangle in equation 1,
⇒ Area of minor segment = 39.25 -25
= 14.25 cm2
Area of major segment = πR2 – area of minor segment
⇒ Area of major segment = 157 – 14.25 = 142.75 cm2
Area of major segment is 142.75 cm2 and of minor segment is 14.25 cm 2.
Q.17. Find the area of both the segments of a circle of radius 42 cm with central angle 120°. [Given, sin 120° = √3/2 and √3 = 1.73.]
Given R = 42 cm and central angle of sector = 120°
Area of minor segment = Area of sector – Area of triangle → eqn1
Where R = radius of the circle and θ = central angle of the sector
∴ Area of sector = 1848 cm2
Area of right angle triangle = 1/2×base×height×sin θ
Where θ = central angle of the sector
Area of triangle = 1/2×42×42×√3/2
Area of triangle = (42×42×√3)/4
(put √3 = 1.73)
= 762.93 cm2
Put the values of area of triangle and area of sector in equation 1
⇒ Area of minor segment = 1848 – 762.93
= 1085.07 cm2
Area of major segment = πR2 – Area of minor segment
Put the value of area of minor segment and R in above equation
= π(422) – 1085.07
⇒Area of major segment = 22/7×42×42-1085.07
(put π = 22/7)
⇒ Area of major segment = 5544 – 1085.07
∴ Area of major segment = 4458.93 cm2
Area of major segment is 4458.93 cm2 and of minor segment is 1085.07 cm2.
Q.18. A chord of a circle of radius 30 cm makes an angle of 60° at the centre of the circle. Find the areas of the minor and major segments. [Take π = 3.14 and √3 = 1.732.]
Area of minor segment = Area of sector – Area of triangle → eqn1
Where R = radius of the circle and θ = central angle of the sector
∴ Area of sector = 471 cm2
Where a = side of the triangle
Area of triangle = √3/4×30×30
Area of triangle = √3/4×900
Area of triangle = (900×√(3 ))/4
(put √3 = 1.732)
Area of triangle = (1.732×900)/4
∴ Area of triangle = 389.7 cm2
Put the values of area of triangle and area of sector in equation 1
Area of minor segment = 471 – 389.7
⇒ Area of minor segment = 81.3 cm2
Area of major segment = πR2 – Area of minor segment
Put the value of area of minor segment and R in above equation
⇒ Area of major segment = π×(302) – 81.3 (put π = 3.14)
⇒ Area of major segment = 3.14×30×30 – 81.3
⇒ Area of major segment = 2826 – 81.3
= 2744.7 cm2
Area of major segment is 2744.7cm2 and of minor segment is 81.3 cm2.
Q.19. In a circle of radius 10.5 cm, the minor arc is one-fifth of the major arc. Find the area of the sector corresponding to the major arc.
Given radius of circle = R = 10.5 cm
Let the area of major sector be ‘A1’ and that of minor sector be ‘A2’
We know, Area of circle = Area of major sector + Area of minor sector
⇒ Area of circle = A1 + A2
We also know, Area of circle = πR2
Where R = radius of circle, put value of area of circle in equation 2.
(taking 5 as L.C.M on R.H.S)
= 288.75 cm2
The area of major sector is 288.75 cm2.
Q.20. The short and long hands of a clock are 4 cm and 6 cm long respectively. Find the sum of distances travelled by their tips in 2 days. [Take π = 3.14.]
In an hour the minute hand completes one rotation therefore in 24 hours the minute hand will complete 24 rotations similarly the hour hand completes one rotation in 12 hours therefore in 24 hours it will complete 2 rotations. Now we have to just calculate the perimeter of the circle traced by minute hand and hour hand and multiply it with the number of rotations of minute hand and hour hand in 2 days respectively.
Length of short/hour hand = r = 4 cm
Length of long/minute hand = R = 6 cm
∴ The perimeter of circle traced by short hand = p = 2πr → eqn1
∴ The perimeter of circle traced by Long hand = P = 2πR → eqn2
Now put the value of ‘r’ and ‘R’ in the equation 1 and 2 respectively.
⇒ p = 2π(4) & P = 2π(6) (put π = 3.14)
⇒ p = 2×3.14×4 & P = 2×3.14×6
∴ p = 25.12 cm & P = 37.68 cm
Therefore, distance covered by short hand in one rotation = 25.12 cm
Distance covered by long hand in one rotation = 37.68 cm
Number of rotation of short hand in one day = 2
Number of rotation of long hand in one day = 24
Therefore number of rotation of small hand in two days = 4
Number of rotation of long hand in two days = 48
Total distance covered by long hand in 2 days = P × no. of rotations in 2 days
⇒ Total distance covered by long hand in 2 days = 37.68×48
⇒ Total distance covered by long hand in 2 days = 1808.64 cm → eqn3
Total distance covered by short hand in 2 days = p × no. of rotations in 2 days
⇒ Total distance covered by short hand in 2 days = 25.12×24
⇒ Total distance covered by short hand in 2 days = 100.48 cm → eqn4
Now total distance covered by tip of both hands in 2 days = eqn3 + eqn4
⇒ Total distance covered by both hands in 2 days = 1808.64 + 100.48
⇒ Total distance covered by both hands in 2 days = 1909.12 cm
The distance covered by both hands tip in 2 days is 1909.12 cm
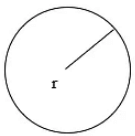
Q.21. Find the area of a quadrant of a circle whose circumference is 88 cm.
Quadrant is a sector in which the central angle is 90 degrees, and this is the key to solve this question. As we know the central angle of the sector so we can easily calculate the area of quadrant by first calculating the radius of the circle as the circumference of the circle is given and then applying the formula of area of sector.
So, we know Circumference of a circle = 2πR → eqn1
Where R = radius of the circle
Given Circumference of the circle = 88 cm, θ = 90°
Put the given values in equation 1
⇒ 88 = (44×R)/7
⇒ 88 = 44R/7
⇒ (88×7)/44 = R
⇒ 616/44 = R
⇒ R = 14 cm
Put the values of R and θ in the above equation
= 154 cm2.
The area of quadrant is 154 cm2.
Q.22. A rope by which a cow is tethered is increased from 16 m to 23 m. How much additional ground does it have now to graze?
Here the increase in the length of the rope simply means that there is increase in the radius of the circle within which cow can graze. Now to find the additional area available for grazing can be easily be found by simply subtracting the initial area available for grazing from the new area available.
Initial radius = r = 16 cm
Increased radius = R = 23 cm
Additional ground available = Area of new ground – Initial area → eqn1
Initial area of ground = π(r2)
⇒ Initial area of ground = π(162)
⇒ Initial area of ground = 256π → eqn2
Area of new ground = πR2
⇒ Area of new ground = π(232)
⇒ Area of new ground = 529π → eqn3
Now put the values of equation 2 and 3 in equation 1
⇒ Additional area of ground available = 529π – 256π
⇒ Additional area available = (529 – 256)π (Taking π common)
⇒ Additional ground available = 273π
= (22×273)/7
= 6006/7
= 858 cm2
The additional ground available is 858 cm2.
Q.23. A horse is placed for grazing inside a rectangular field 70 m by 52 m. It is tethered to one corner by a rope 21 m long. On how much area can it graze? How much area is left ungrazed?
Here the horse is tethered to one corner implies or means that the area available for grazing is a quadrant of radius 21 m. Now we need to find the area of this quadrant to find out the area available for grazing and then subtract it from the total area of the rectangular field to obtain the area left ungrazed.
Given length of rectangular field = ℓ = 70 m
Breadth of rectangular field = b = 52 m
∴ Area of the field = ℓ × b
⇒ Area of the field = 70×52
⇒ Area of the field = 3640 m2
We know in a rectangle all the angles are 90 degrees.
∴ Area available for grazing = area of quadrant
Where R = radius of circle & θ = central angle
Given R = 21 m and θ = 90°
Put the given values in the above equation,
(put π = 22/7)
= (22×63)/4
= 1386/4
⇒ Area available for grazing = 346.5 m2
Area left ungrazed = Area of field – Area available for grazing
⇒ Area left ungrazed = 3640 – 346.5
⇒ Area left ungrazed = 3293.5 m2
The area available for grazing is 346.5 m2 and area left ungrazed is 3293.5 m2.
Q.24. A horse is tethered to one corner of a field which is in the shape of an equilateral triangle of side 12 m. If the length of the rope is 7 m, find the area of the field which the horse cannot graze. Take √3 = 1.732. Write the answer correct to 2 places of decimal.
Here the horse is tethered to one corner implies or means that the area available for grazing is a sector of radius 21 m with central angle as 60 degrees as the field is in shape of equilateral triangle . Now we need to find the area of this sector to find out the area available for grazing and then subtract it from the total area of the triangular field to obtain the area left ungrazed.
Given the side of field = a = 12 m
∴ Area of field = Area of equilateral triangle
⇒ Area of field = 62.352 m2
We know in an equilateral triangle all the angles are 60 degrees.
∴ Area available for grazing = Area of the sector
Where R = radius of circle and θ = central angle of sector
Given R = 7 m and θ = 60°
Put the given values in the above equation,
⇒ Area available for grazing = 25.666 m2
Area that cannot be grazed = Area of field – Area available for grazing
⇒ Area that cannot be grazed = 62.352 – 25.666
⇒ Area that cannot be grazed = 36.686 m2
The area that cannot be grazed is 36.656 m2.
Q.25. Four cows are tethered at the four corners of a square field of side 50 m such that each can graze the maximum unshared area. What area will be left ungrazed? [Take π = 3.14.]
Here the 4 cows are tethered to each corner implies or means that the area available for grazing is a quadrant of radius 25 m with central angle as 60 degrees as the field is in shape of square . Now we need to find the area of this sector to find out the area available for grazing for all the cows and then subtract it from the total area of the square field to obtain the area left ungrazed.
The reason why we have taken the radius as 25 m is , basically we have considered that each cow is tethered to a rope which is equal to half of the side of the square as we had to maximize the area each cow gets to graze without sharing thus the maximum radius within which a cow can graze maximum unshared area is simply the half of the side of square.
Given the side of field which is in shape of square = a = 50 m
∴ Area of the field = Area of Square
⇒ Area of field = a2
⇒ Area of field = (502)
⇒ Area of field = 2500 m2
We know in an square all the angles are 90 degrees.
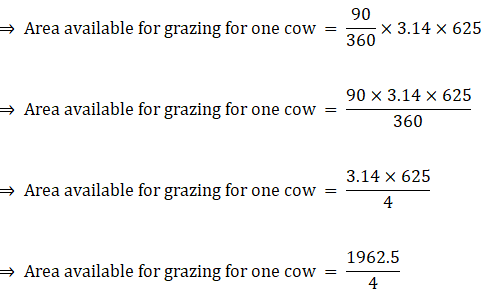
∴ Area available for grazing for one cow = area of sector/quadrant
Where R = radius of circle & θ = central angle of sector
Given R = 25 m & θ = 90°
Put the given values in the above equation,
⇒ Area available for grazing for one cow = 490.625 m2
⇒ Area available for 4 cows = 4 × Area available for one cow
⇒ Area available for 4 cows = 4 × 490.625
⇒ Area available for 4 cows = 1962.5 m2
Area left ungrazed = Area of field – Area available for grazing for 4 cows
⇒ Area that cannot be grazed = 2500 – 1962.5
⇒ Area that cannot be grazed = 537.5 m2
The area left ungrazed is 537.5 m2.
Q.26. In the given figure, OPQR is a rhombus, three of whose vertices lie on a circle with centre O. If the area of the rhombus is 32√3 cm2, find the radius of the circle.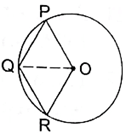
Here in the given figure ‘O’ is the centre of circle on which three vertices of rhombus lie, this implies that OP, OR are both radius of the circle. Also we know that in rhombus all the 4 sides are equal in length. Thus OP, OR, PQ, RQ, they all are radii of circle. Also OQ is equal to radius of circle. Now rhombus being a parallelogram therefore diagonal OQ will divide the rhombus into two equal halves this means that the area of triangle OQR will be equal to half of the area of rhombus. Also we can see that triangle OQR is an equilateral triangle and hence we can easily calculate its area in terms of radius of circle and equate it to half of the area of rhombus and calculate the radius of given circle.
Let the radius of the circle = x cm
Now join OQ
Consider ∆OQR,
OQ = OR = RQ = x cm
⇒ ∆OQR is an equilateral triangle
Where a = side of equilateral triangle
Also we know OQ is a diagonal of rhombus OPQR and as in a parallelogram diagonal divides it into two equal area or halves , similarly OQ is also dividing the rhombus into two equal areas therefore,
⇒ Area of ∆OQR = Area of ∆OPQ → eqn2
Area of OPQR = Area of ∆OQR + Area of ∆OPQ
Area of OPQR = 2 × Area of ∆OQR (from eqn2) → eqn3
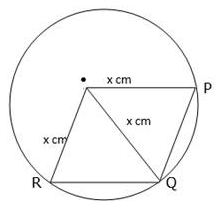
Put the values of area of OPQR and equation 1 in equation 3
As every quadratic equation has two roots, similarly x2 = 64 also have two roots i.e. x = 8 and x = -8. As we know that ‘x’ represents radius of circle therefore it cannot be a negative value, hence we discard the negative root.
Therefore radius of the circle = x = 8 cm.
The radius of circle is 8 cm.
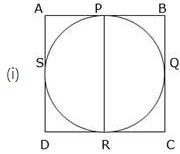
Q.27. The side of a square is 10 cm. Find
(i) The area of the inscribed circle, and
(ii) The area of the circumscribed circle. [Take π = 3.14.]
Consider the above figure, Join PR,
Now PR = Diameter of the inscribed circle
Also, PR = BC = 10 cm.
So, PR = 10 cm
⇒ r = 5 cm
∴ Area of inscribed circle = πr2 (put value of r in this equation)
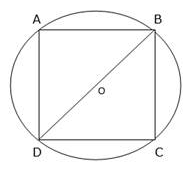
⇒ Area of inscribed circle = π(52)
⇒ Area of inscribed circle = 78.57 cm2
The area of inscribed circle is 78.57 cm2.
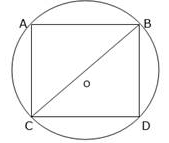
(ii)
Consider the above figure, O is the centre of circle and ABCD is a square inscribed. Now OB and OD are radii of circle.
Consider ∆DBC right angled at c (as C is a vertex of square)
∴ Apply Pythagoras theorem in triangle DBC
Hypotenuse2 = Perpendicular2 + Base2
In triangle DBC, hypotenuse = DB,
perpendicular = BC and
base = DC
Put the values of BC and DC i.e. 10 cm
⇒ BD2 = 200
⇒ BD = √200
⇒ BD = 10√2 cm
Now radius of circle = half of BD
⇒ r = (10√2)/2
⇒ r = 5√2 cm
Hence Area of circumscribing circle = πr2
⇒ Area of circumscribing circle = 3.14×5√2×5√2
(put π = 3.14 and r = 5√2 cm)
⇒ Area of circumscribing circle = 3.14 × 50
⇒ Area of circumscribing circle = 157 cm2
Area of circumscribing circle is 157 cm2.
Q.28. If a square is inscribed in a circle, find the ratio of the areas of the circle and the square.
Consider the figure shown below where O is centre of circle, join BC which passes through O, let the side of square be ‘a’ and radius of circle be ‘r’.
Now we know OB and OC are radius of circle
So, OB = OC = r
Consider ∆BDC right angled at D
And we know BC = OC + OB
BC = 2r and BD = DC = a (put these values in eqn1)
⇒ (2r)2 = a2 + a2
⇒ 4r2 = 2a2
Area of inscribed square = side × side
Areaa of inscribed square = a × a
Area of inscribed square = a2→ eqn3
Area of circumscribing circle = πR2 where R = radius of circle
⇒ Area of circumscribing circle = πr2→ eqn4
Put the values from equation 3 & 4 in above equation
(from eqn 2)
So, Ratio is π : 2
The ratio is π:2.
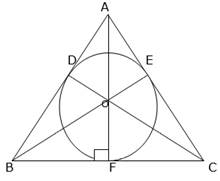
Q.29. The area of a circle inscribed in an equilateral triangle is 154 cm2. Find the perimeter of the triangle. [Take √3 = 1.73.]
Consider the figure shown above, AF, BE and CD are perpendicular bisector.
Now we know that the point at which all three perpendiculars meet is called incentre, so O is the incentre, thus O divides all three perpendiculars in a ratio 2:1.
Let AB = BC = CA = a cm
Therefore let AF = h cm
⇒ ∠AFC = 90° and OF = 1/3 × AF
⇒ OF = h/3 cm (putting value of OF)
⇒ h = 3×OF → eqn1
And we can see from figure that OF = radius of circle
Now let radius of circle be = r cm
∴ Area of circle = πR2
where R = radius of circle
Given area of circle = 154 cm2
⇒ πr2 = 154
⇒ r2 = 49
⇒ r = 7 cm
Therefore OF = 7 cm
⇒ h = 3×7 (from eqn 1)
⇒ h = 21 cm
we know area of an equilateral triangle =
where a = side of triangle
Also, Area of triangle = 1/2 ×base×height
Equating both the areas we get,
Put the values of BC and AF
(putting value of h = 21 cm)
(rationalize it)
⇒ a = 14√3 cm
∴ Perimeter of equilateral triangle = 3×side of triangle
⇒ Perimeter of ∆ ABC = 3×14√3 (put √3 = 1.73)
⇒ Perimeter of ∆ABC = 42×1.73
⇒ Perimeter of ∆ABC = 72.66 cm
The perimeter of triangle is 72.66 cm
Q.30. The radius of the wheel of a vehicle is 42 cm. How many revolutions will it complete in a 19.8-km-long journey?
In one revolution a wheel will cover a distance equal to its circumference, so in order to find the number of revolutions we have to first calculate the circumference of the wheel and then divide it with the total distance covered to find out the total number of revolutions
Given radius of wheel = r = 42 cm
Circumference of wheel = 2πR where R = radius of the wheel
= 2π(42) (putting value of r)
Therefore distance covered in one revolution = 264 cm
Total distance covered = 19.8 km = 1980000 cm
Total number of revolutions = n
Distance covered on 1 revolution ×no. of revolutions = Total distance
264×n = 1980000
⇒ n = 7500
Total number of revolutions is 7500.
Q.31. The wheels of the locomotive of a train are 2.1 m in radius. They make 75 revolutions in one minute. Find the speed of the train in km per hour.
Given radius of wheel = R = 2.1m
Number of revolutions in one minute = 75
Number of revolutions in 1 hour = 75×60
Number of revolutions in 1 hour = 45000
Distance covered in one revolution = Circumference of wheel
Distance covered in 1 revolution = 2πR (where R = radius of wheel)
Distance covered n 1 revolution = 2π(2.1)
= 13.2 m
So, distance covered in 4500 revolutions = 4500×distance covered in 1
Distance covered in 4500 revolution = 4500× 13.2
Distance covered in 4500 revolutions = 59400 m = 59.4 km
∴ Distance covered in 1 hour = 59.4 km
Hence speed of the locomotive = 59.4 km/hr
The speed of locomotive is 59.4 km/hr
Q.32. The wheels of a car make 2500 revolutions in covering a distance of 4.95 km. Find the diameter of a wheel.
Let the diameter of the wheel be ‘d’ cm
Total distance covered in 250 revolutions = 49.5 km = 495000 m
⇒ Distance covered in one revolution = 198 cm → eqn1
Also, Distance covered in one revolution = circumference of wheel
∴ Distance covered in one revolution = πD where d = diameter of wheel
Equate equation 1 and 2 we get,
⇒ d = 9×7
⇒ d = 63 cm
The diameter of the wheel is 63 cm.
Q.33. A boy is cycling in such a way that the wheels of his bicycle are making 140 revolutions per minute. If the diameter of a wheel is 60 cm, calculate the speed (in km/h) at which the boy is cycling.
Given diameter of wheel = d = 60 cm
Number of revolutions in one minute = 140
Number of revolutions in one hour = 140×60
Number of revolutions in one hour = 8400
Distance covered in one revolution = circumference of wheel
⇒ Distance covered in one revolution = πd
= 188.57 cm
Distance covered in one hour = Distance in 1 revolution × no. of revolutions
⇒ Total distance covered in one hour = 188.57× 8400
⇒ Total distance covered in one hour = 1583988 cm = 15.839 km
∴ speed with which boy is cycling = 15.839 km/hr
The speed with which boy is cycling is 15.839 km/hr
Q.34. The diameter of the wheels of a bus is 140 cm. How many revolutions per minute do the wheels make when the bus is moving at a speed of 72.6 km per hour?
Given diameter of wheel of bus = d = 140 cm
Speed of bus = 72.6 km/hr
∴ Distance covered by bus in one hour = 72.6 km = 7260000 cm
Distance covered in one minute = 121000 cm → eqn1
Let the number of revolutions made by wheel per minute = x
Distance covered by wheel in one revolution = circumference of wheel = 2πR
Distance covered by wheel in one revolution = 2π(70)
(putting value of R)
= 2×22×10 = 440 cm
∴ Total distance = No. of revolution× Distance covered in1 revolution
On putting the required values we get,
121000 = 440×(x)
⇒ x = 275
Number of revolutions made per minute is 275.
Q.35. The diameters of the front and rear wheels of a tractor are 80 cm and 2 m respectively. Find the number of revolutions that a rear wheel makes to cover the distance which the front wheel covers in 800 revolutions.
Given diameter of front wheel = d = 80 cm
so, Radius of front wheel = r = d/2 = 80/2 = 40 cm
Diameter of rear wheel = D = 2 m = 200 cm
Distance covered by wheel in 1 revolution = Circumference of wheel
⇒ Distance covered by front wheel = 2πr = 2π(40)
⇒ Distance covered by front wheel = 80π
∴ Distance covered by front wheel in 800 revolutions = 80π×800
⇒ Distance covered by front wheel in 800 revolutions = 6400π → eqn1
Similarly
⇒ Distance covered by rear wheel = 2πR = 2π(100)
⇒ Distance covered by rear wheel = 200π → eqn2
Let the number of revolutions made by rear wheel to cover 6400π cm be “x”
∴ (x)×200π = 6400π (from eqn1 and eqn2)
⇒ x = 64000/200
⇒ x = 320
Number of revolution made by rear wheel to cover the distance covered by front wheel in 800 revolutions is 320.
Q.36. Four equal circles are described about the four corners of a square so that each touches two of the others, as shown in the figure. Find the area of the shaded region, if each side of the square measures 14 cm.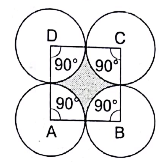
Here the distance between the center of circles touching each other is equal to the side of the square. Therefore, we can say that the radius of ach circle is equal to the half of the side of the square. Now by simply calculating the area of the 4 quadrants and then subtracting it from the area of the square we can easily calculate the area of the shaded region.
Given side of square = a = 14 cm
Central angle of each sector formed at corner = θ = 90°
So, radius of 4 equal circles = r = a/2 = 14/2
∴ Radius of 4 circles = r = 7 cm
where R = radius of circle
⇒ Area of all 4 quadrants = 49π → eqn2
Also, Area of square = side×side = a×a = a2 = 142 (putting value of side of square)
⇒ Area of square = 196 cm2→ eqn3
∴ Area of shaded region = Area of square – Area of all 4 quadrants
⇒ Area of shaded region = 196 – 49π (fromeqn3 and eqn2)
= 196 – (7×22)
= 196 – 154
= 42 cm2
The area of shaded region is 42 cm2.
Q.37. Four equal circles, each of radius 5 cm, touch each other, as shown in the figure. Find the area included between them. [Take π = 3.14]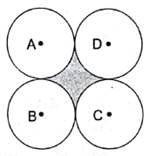
Here, first we join the center of all adjacent circles then the distance between the center of circles touching each other is equal to the side of the square formed by joining the center of adjacent circles. Therefore, we can say that the side of the square equal to the twice of the radius of circle. Now by simply calculating the area of the 4 quadrants and then subtracting it from the area of the square we can easily calculate the area of the shaded region.
Given radius of each circle = r = 5 cm
Central angle of each sector formed at corner = θ = 90°
Side of square ABCD = a = 2×r = 2×5 = 10 cm
where R = radius of circle
(putting value of r and θ)
Area of all 4 quadrants = 4×Area of one quadrant
⇒ Area of all 4 quadrants = 25π → eqn2
Area of square = side×side = a×a = a2
⇒ Area of square = 102 (putting value of side of square)
⇒ Area of square = 100 cm2→ eqn3
Area of shaded region = Area of square – Area of all 4 quadrants
Area of shaded region = 100 – 25π (from eqn3 and eqn2)
= 100 – (25×3.14) (put π = 3.14)
= 100 – 78.5
= 21.5 cm2
The area of shaded region is 21.5 cm2.
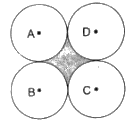
Q.38. Four equal circles, each of radius a units, touch each other. Show that the area between them is 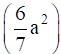 sq units.
sq units.
Here, first we join the centre of all adjacent circles then the distance between the centre of circles touching each other is equal to the side of the square formed by joining the centre of adjacent circles. Therefore, we can say that the side of the square equal to the twice of the radius of circle. Now by simply calculating the area of the 4 quadrants and then subtracting it from the area of the square we can easily calculate the area of the shaded region.
Given radius of each circle = “a” units
Central angle of each sector formed at corner = θ = 90°
Side of square ABCD = 2×a units
where R = radius of circle
∴ Area all 4 quadrants = 4×Area of one quadrant
= πa2 sq. units → eqn2
Area of square = side × side = 2a×2a = 4a2
⇒ Area of square = 4a2 sq. units → eqn3
Area of shaded region = Area of square – Area of all 4 quadrants
⇒ Area of shaded region = 4a2 – πa2 (from eqn3 and eqn2)
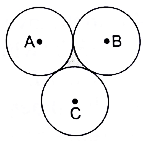
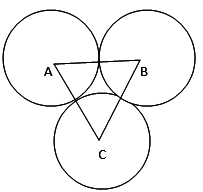
Q.39. Three equal circles, each of radius 6 cm, touch one another as shown in the figure. Find the area enclosed between them. [Take π = 3.14 and √3 = 1.732.]
Consider the above figure,
Here, first we join the center of all adjacent circles then the distance between the center of circles touching each other is equal to the side of an equilateral triangle formed by joining the center of adjacent circles. Therefore, we can say that the side of the equilateral triangle is equal to the twice of the radius of circle. Now by simply calculating the area of the 3 sectors and then subtracting it from the area of the equilateral triangle we can easily calculate the area of the enclosed region.
Given radius of each circle = r = 6 cm
Central angle of each sector = θ = 60° (∵ ∆ABC is equilateral)
Side of equilateral ∆ABC = a = 2×r = 2×6
∴ Side of equilateral ∆ABC = a = 12 cm
⇒ Area of one sector = 6π cm2→ eqn1
Area of all the 3 sector = 3×Area of one sector
= 3×6π (from eqn1)
= 18π cm2→ eqn2
Area of enclosed region = Area of equilateral ∆ABC – Area of all 3 sectors
⇒ Area of enclosed region = 36√3-18π (from eqn 3 and eqn 2)
⇒ Area of enclosed region = (36×1.732)-(18×3.14)
(put π = 3.14 &√3 = 1.732)
= 62.352 - 56.52
= 5.832 cm2
The area of enclosed region is 5.832 cm2.
Q.40. If three circles of radius a each, are drawn such that each touches the 4 other two, prove that the area included between them is equal to 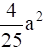 [Take √3 = 1.73 and π = 3.14.]
[Take √3 = 1.73 and π = 3.14.]
Consider the figure shown below
Here, first we join the center of all adjacent circles then the distance between the center of circles touching each other is equal to the side of an equilateral triangle formed by joining the center of adjacent circles. Therefore, we can say that the side of the equilateral triangle is equal to the twice of the radius of circle. Now by simply calculating the area of the 3 sectors and then subtracting it from the area of the equilateral triangle we can easily calculate the area of the enclosed region.
Given radius of each circle = “a” units
Central angle of each sector = θ = 60° (∵ ∆ABC is equilateral)
Side of equilateral ∆ABC = 2×a units
∴ Area of all 3sectors = 3×Area of one sector
Area of enclosed region = Area of equilateral ∆ABC – Area of all 3 sectors
⇒ Area of enclosed region