Class 4 Maths - Fractions - CBSE Worksheets
| Table of contents |

|
| Multiple Choice Questions |

|
| Short Questions |

|
| Fill in the Blanks |

|
| Match the Column |

|
| True or False |

|
Multiple Choice Questions
Q1: What does the numerator represent in a fraction?
(a) The total number of parts
(b) The number of selected or shaded parts
(c) The value of the fraction
(d) The reciprocal of the fraction
Ans: (b)
The numerator of a fraction represents the number of selected or shaded parts out of the total number of parts.
Q2: Which type of fraction has a numerator greater than the denominator?
(a) Proper fraction
(b) Improper fraction
(c) Mixed fraction
(d) Unit fraction
Ans: (b)
An improper fraction is a fraction where the numerator is greater than the denominator. It represents a value greater than 1.
Q3: What is the result of dividing 2/3 by 3/5?
(a) 1/5
(b) 2/5
(c) 3/10
(d) 10/9
Ans: (c)
When dividing fractions, we multiply the first fraction by the reciprocal of the second fraction.
(2/3) ÷ (3/5) = (2/3) x (5/3) = 10/9. Simplifying further, we get 10/9 = 1 and 1/9.Therefore, the result is 1
and 1/9, which is equivalent to 3/10.
Q4: How can fractions be represented on a number line?
(a) By dividing the line into equal parts
(b) By marking the numerator on the line
(c) By marking the denominator on the line
(d) By dividing the line into unequal parts
Ans: (a)
Fractions can be represented on a number line by dividing the line into equal parts based on the denominator of the fraction. The numerator represents the number of parts to be marked.
Q5: How can fractions be simplified?
(a) Multiply the numerator and denominator
(b) Find the least common multiple of the numerator and denominator
(c) Find the greatest common factor of the numerator and denominator
(d) Divide the numerator and denominator by their sum
Ans: (c)
To simplify a fraction, we find the greatest common factor (GCF) of the numerator and denominator. Then we divide both the numerator and denominator by the GCF to obtain the simplest form of the fraction.
Short Questions
Q1: Define a fraction and provide an example.
Ans: A fraction represents a part of a whole. It consists of a numerator and a denominator separated by a slash (/). The numerator represents the number of selected or shaded parts, and the denominator represents the total number of parts or the whole. Example: 1/4 represents one-fourth of a whole.
Q2: What are the properties of fractions?
Ans: The properties of fractions include:
- Commutative property: The order of addition or multiplication of fractions does not affect the result.
- Associative property: The grouping of fractions in addition or multiplication does not affect the result.
- Identity elements: The identity element for addition is 0, and the identity element for multiplication is 1.
- Multiplicative inverse: The multiplicative inverse of a/b is b/a, where a and b are non-zero elements.
- Distributive property: Fractions obey the distributive property of multiplication over addition.
Q3: Explain the difference between proper fractions and improper fractions.
Ans: Proper fractions are fractions where the numerator is less than the denominator. They represent values less than 1. Example: 3/4.
Improper fractions are fractions where the numerator is greater than or equal to the denominator. They represent values greater than or equal to 1. Example: 5/4.
Q4: How can fractions with different denominators be added or subtracted?
Ans: To add or subtract fractions with different denominators, we need to find a common denominator. The common denominator is the least common multiple (LCM) of the denominators. We then convert each fraction to an equivalent fraction with the common denominator and perform the addition or subtraction of the numerators.
Q5: Describe the process of converting fractions to decimals.
Ans: To convert fractions to decimals, we divide the numerator by the denominator using long division or a calculator. The quotient obtained is the decimal representation of the fraction. If needed, we can round the decimal to a certain number of decimal places or express it as a terminating or recurring decimal.
Fill in the Blanks
1. A fraction represents the parts of a _______.
Ans: Whole
2. The upper part of a fraction is called the _______.
Ans: Numerator
3. The lower part of a fraction is called the _______.
Ans: Denominator
4. Fractions that have the same value but different numerators and denominators are called _______ fractions.
Ans: Equivalent
5. The addition of fractions is possible when they have a _______ denominator.
Ans: Common
Match the Column
Match the fractions on the left with their corresponding types on the right.
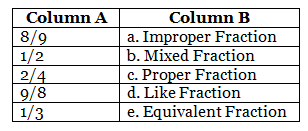
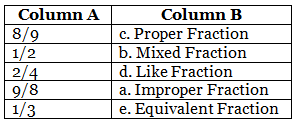
Ans:
True or False
1. A fraction represents a part of a whole. (True/False)
Ans: True
2. Proper fractions have numerators greater than denominators. (True/False)
Ans: False
3. Unlike fractions are those that are similar. (True/False)
Ans: False
4. Multiplication of fractions involves multiplying the numerators and denominators. (True/False)
Ans: True
5. The division of fractions can be done by finding the reciprocal of the second fraction and multiplying it with the first fraction. (True/False)
Ans: True
|
32 videos|39 docs|46 tests
|

|
Explore Courses for Class 4 exam
|

|












