Worksheet Solutions: Cube - 2 - Class 7 PDF Download
Q1: Find the cube roots by prime factorization method
(i) 15625
(ii) 2744
(iii) 125/2197
(iv) 5832
(v) 64000
(vi) 10648
(vii) 1331
(viii) 1728
Ans:
Q2: Is 256 a perfect cube? If not, find the smallest number by which it should be divided to get a perfect cube.
Ans: 256 = 28 = 23 × 23 × 22
Therefore it is not a perfect cube
So, it must be divided by 4 to become perfect cube
Q3: Three numbers are in the ratio 1:2:3 and the sum of their cubes is 4500. Find the numbers.
Ans: Let the number be x,2x,3x
Now x3 + 8x3 + 27x3 = 4500
36x3 = 4500 or x3 = 125
x = 5
So numbers are 5,10,15
Q4: Find the cubes of these numbers.
(i) .4
(ii) 8
(iii) 15
(iv) .02
(v) 1.1
(vi) .011
Ans:
(i) .43 = .046
(ii) 83 = 512
(iii)153 = 3375
(iv) .023 = .000008
(v) 1.13 = 1.331
(vi) .0113 = .000001331
Q5: if 6n+2 = 1296 , then 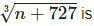
Ans: 6n+2 = 1296
6n+2 = 64
n + 2 = 4 or n = 2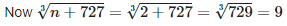
Q6: (i)The least number by which 72 be multiplied to make it a perfect cube is _____________
(ii) If 8x3 = 216, then x ix ____
(iii) The cube of .5 is _____
(iv) There are _________ perfect cubes between 1 and 1000.
(v) The digit at the ones place of 233 is ____
(vi) The cube of the even natural number is ____
Ans:
(i) 3
(ii) 3
(iii) .125
(iv) 8
(v) 7
(vi) even
Q7: (i) 648 is not perfect cube?
(ii) 999 is a perfect cube
(iii) Square of a number is positive, so the cube of that number will also be positive
(iv) 125 × 8 × 27 is a perfect cube
(v) For an integer p, p3 is always greater than p2
(vi) .83 = 5.12
Ans: (i)True
(ii) false
(iii) false
(iv) True
(v)False
(vi) False
Q8: Cube root of the expression 1252
(a) 5
(b) 25
(c) 10
(d) 125
Ans: (b)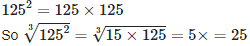
Q9: Which of these is a perfect cube
(a) 216
(b) 392
(b) 8640
(d) 243
Ans: (a)
Q10: Which of these is not a perfect cube
(a) 729
(b) 2197
(c) -1331
(d) 169
Ans: (d)













