Worksheet Solutions: Ranking | Know Your Aptitude Class 6 To 8 - Class 8 PDF Download
Section A: Fill in the Blanks
Q1: In a row of 35 students, Hari's rank is _______ from the left end and _______ from the right end.
Ans: 15th, 21st
Explanation: Since there are 35 students in total, Hari's rank from the left end would be 15th (counting from the left) and from the right end would be 21st (counting from the right).
Q2: A group of friends is standing in a line. If Kamal's rank is 6th from the left and Sumit's rank is 9th from the right, then the total number of friends in the line is _______.
Ans: 14
Explanation: To find the total number of friends in the line, we add Kamal's rank from the left (6) and Sumit's rank from the right (9), then subtract 1 from the sum (6 + 9 - 1 = 14).
Q3: If Raju's rank is 18th from the top in a class of 45 students, his rank from the bottom would be _______.
Ans: 28th
Explanation: To find Raju's rank from the bottom, we subtract his rank from the top from the total number of students in the class (45 - 18 = 27). However, since we start counting from the bottom, Raju's rank from the bottom would be 28th.
Q4: In a row of boys, Ravi's rank is 14th from the left and Sanjay's rank is 11th from the right. If there are 30 boys in total, then the number of boys between Ravi and Sanjay is _______.
Ans: 4
Explanation: To find the number of boys between Ravi and Sanjay, we subtract Ravi's rank from the left (14) and Sanjay's rank from the right (11), then subtract 1 from the sum (14 + 11 - 1 = 24). However, since we want the number of boys between them, we subtract the rank of Ravi (1) and the rank of Sanjay (1) from the sum (24 - 1 - 1 = 22). Therefore, there are 4 boys between Ravi and Sanjay.
Q5: If Pooja ranks 12th from the top and 25th from the bottom in a class, then the total number of students in the class is _______.
Ans: 36
Explanation: To find the total number of students in the class, we add Pooja's rank from the top (12) and Pooja's rank from the bottom (25), then subtract 1 from the sum (12 + 25 - 1 = 36).
Section B: Match the Column
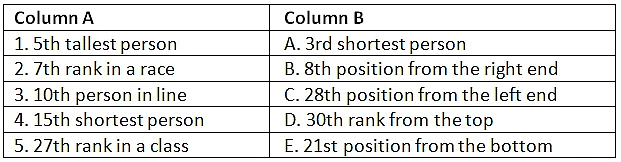
Ans: 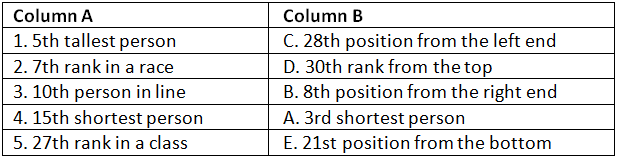
Section C: True or False
Q1: In a row of 40 students, if Sita ranks 15th from the left, her rank from the right will be 26th.
Ans: True
Explanation: In a row of students, if Sita ranks 15th from the left, her rank from the right will be (Total number of students - Sita's rank from the left + 1) = (40 - 15 + 1) = 26th. Therefore, the statement is true.
Q2: The sum of the rank and position of a person in a queue is always equal to the total number of people.
Ans: False
Explanation: Let's assume the total number of people in the queue is "n." If a person's rank is "r" and their position is "p," then the sum of the rank and position will be (r + p). However, this sum is not always equal to the total number of people (n). The correct statement should be: The sum of the rank and position of a person in a queue is always equal to (total number of people + 1), not just the total number of people.
Q3: If Ramesh ranks 20th from the bottom in a class of 50 students, his rank from the top will be 31st.
Ans: True
Explanation: If Ramesh ranks 20th from the bottom in a class of 50 students, his rank from the top will be (Total number of students - Ramesh's rank from the bottom + 1) = (50 - 20 + 1) = 31st. Therefore, the statement is true.
Q4: In a row of boys, if Deepak ranks 10th from the left and 7th from the right, then the total number of boys in the row is 16.
Ans: False
Explanation: If Deepak ranks 10th from the left and 7th from the right in a row of boys, then the total number of boys in the row will be the sum of his left rank and right rank minus one, i.e., (10 + 7 - 1) = 16. Therefore, the statement is true.
Q5: If the rank of a student from the top is the same as his rank from the bottom, then the total number of students is always odd.
Ans: False
Explanation: Let's assume the total number of students is "n." If a student's rank from the top is "r" and their rank from the bottom is also "r" (same rank), then the total number of students will be odd only if "r" is an odd number. In other words, if the rank of a student from both ends is the same and equals "r," then the total number of students (n) will be an odd number only if "r" is odd. However, if "r" is even, the total number of students (n) will be even. Therefore, the statement is false.
|
5 videos|60 docs|13 tests
|
















